Deck 9: Infinite Series
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/199
Play
Full screen (f)
Deck 9: Infinite Series
1
Let 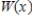 be the Taylor expansion for
be the Taylor expansion for 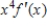 about
about 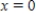 .Find the general term for
.Find the general term for  and find
and find 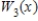 .
.
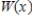 be the Taylor expansion for
be the Taylor expansion for 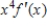 about
about 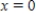 .Find the general term for
.Find the general term for 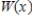 and find
and find 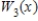 .
. 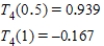
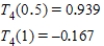
2
If 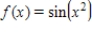 ,the first three terms of the Taylor series expansion about
,the first three terms of the Taylor series expansion about 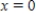 for
for  are
are
A)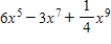
B)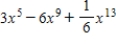
C)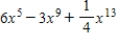
D)
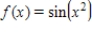 ,the first three terms of the Taylor series expansion about
,the first three terms of the Taylor series expansion about 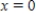 for
for  are
are A)
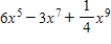
B)
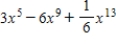
C)
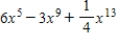
D)

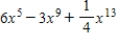
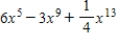
3
Which of the following series is conditionally convergent?
A)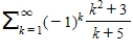
B)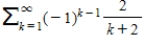
C)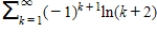
D)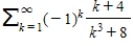
A)
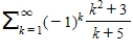
B)
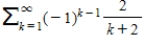
C)
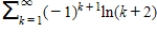
D)
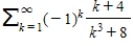
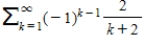
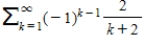
4
Let 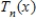 represent the Taylor Polynomial of degree n about
represent the Taylor Polynomial of degree n about 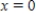 for
for 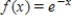 .If
.If 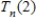 is used to approximate the value of
is used to approximate the value of 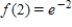 ,then which of the following expressions is NOT less than
,then which of the following expressions is NOT less than  ?
?
A)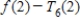
B)
C)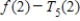
D)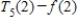
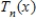 represent the Taylor Polynomial of degree n about
represent the Taylor Polynomial of degree n about 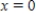 for
for 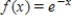 .If
.If 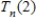 is used to approximate the value of
is used to approximate the value of 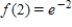 ,then which of the following expressions is NOT less than
,then which of the following expressions is NOT less than  ?
? A)
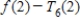
B)

C)
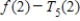
D)
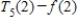

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
5
Find a geometric power series for the function 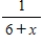 centered at 0.
centered at 0.
A)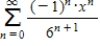
B)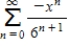
C)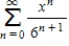
D)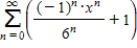
E)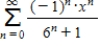
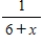 centered at 0.
centered at 0. A)
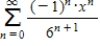
B)
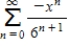
C)
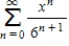
D)
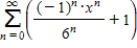
E)
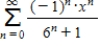

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
6
The radius of convergence of the power series 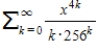 .
.
A)3
B)5
C)4
D)6
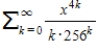 .
. A)3
B)5
C)4
D)6

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
7
The sixth degree term of the Taylor series expansion for  about
about  has coefficient
has coefficient
A)
B)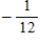
C)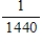
D)
 about
about  has coefficient
has coefficient A)

B)
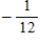
C)
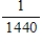
D)


Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
8
Which of the following series diverge? 
A)I only
B)II only
C)I and II only
D)I and III only

A)I only
B)II only
C)I and II only
D)I and III only

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
9
The Maclaurin series for 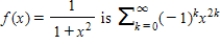 .What is the Maclaurin series for
.What is the Maclaurin series for 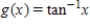 ?
?
A)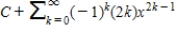
B)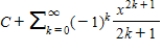
C)
D)
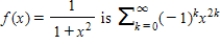 .What is the Maclaurin series for
.What is the Maclaurin series for 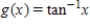 ?
? A)
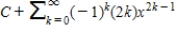
B)
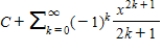
C)

D)


Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
10
For function 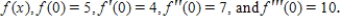 Using the Taylor series expansion for
Using the Taylor series expansion for 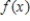 about
about 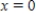 ,the second degree estimate for
,the second degree estimate for 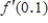 is
is
A)4.335
B)4.750
C)4.420
D)5.240
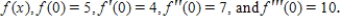 Using the Taylor series expansion for
Using the Taylor series expansion for 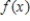 about
about 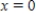 ,the second degree estimate for
,the second degree estimate for 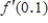 is
is A)4.335
B)4.750
C)4.420
D)5.240

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
11
The power series 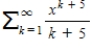 converges for which values of x?
converges for which values of x?
A)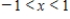
B)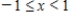
C)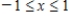
D) is any real number.
is any real number.
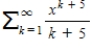 converges for which values of x?
converges for which values of x? A)
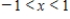
B)
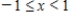
C)
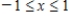
D)
 is any real number.
is any real number.
Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
12
Which of the following series is absolutely convergent?
A)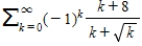
B)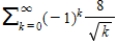
C)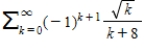
D)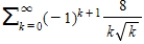
A)
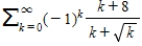
B)
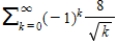
C)
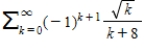
D)
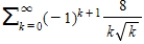

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
13
The Maclaurin series 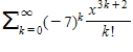 represents which expression below?
represents which expression below?
A)
B)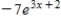
C)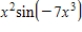
D)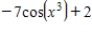
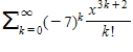 represents which expression below?
represents which expression below? A)

B)
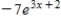
C)
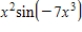
D)
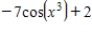

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
14
Which of the following series is the power series expansion for 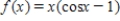 .
.
A)
B)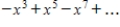
C)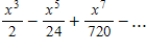
D)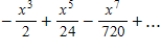
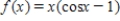 .
.A)

B)
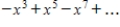
C)
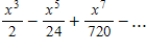
D)
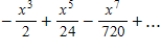

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
15
What are all values of a for which the series 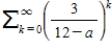 converges?
converges?
A)
B)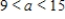
C)
D)
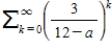 converges?
converges? A)

B)
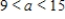
C)

D)


Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
16
Find a geometric power series for the function 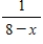 centered at 0.
centered at 0.
A)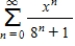
B)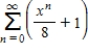
C)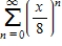
D)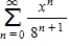
E)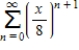
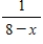 centered at 0.
centered at 0. A)
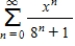
B)
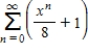
C)
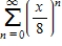
D)
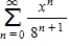
E)
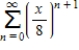

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
17
The Maclaurin series 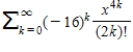 represents which function below?
represents which function below?
A)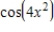
B)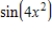
C)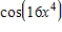
D)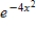
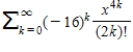 represents which function below?
represents which function below? A)
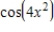
B)
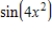
C)
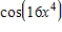
D)
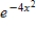

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
18
Which value is smaller, 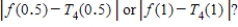 Give a reason for your answer.
Give a reason for your answer.
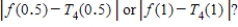 Give a reason for your answer.
Give a reason for your answer.
Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
19
Let  be a function that is differentiable for all
be a function that is differentiable for all  .The Taylor expansion for
.The Taylor expansion for 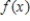 about
about 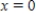 is given by
is given by 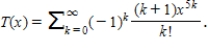 The first four nonzero terms of
The first four nonzero terms of 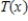 are given by
are given by 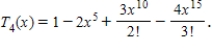
Show that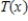 converges for all
converges for all  .
.
 be a function that is differentiable for all
be a function that is differentiable for all  .The Taylor expansion for
.The Taylor expansion for 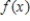 about
about 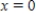 is given by
is given by 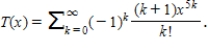 The first four nonzero terms of
The first four nonzero terms of 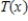 are given by
are given by 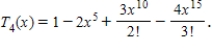
Show that
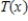 converges for all
converges for all  .
.
Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
20
If the first five terms of the Taylor expansion for 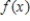 about
about 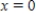 are
are 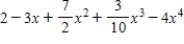 ,then
,then 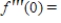
A)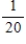
B)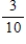
C)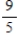
D)
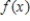 about
about 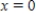 are
are 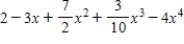 ,then
,then 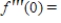
A)
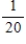
B)
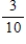
C)
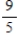
D)


Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
21
Find a power series for the function 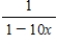 centered at 0.
centered at 0.
A)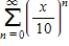
B)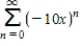
C)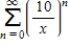
D)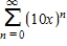
E)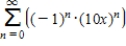
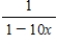 centered at 0.
centered at 0. A)
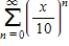
B)
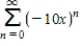
C)
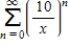
D)
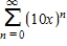
E)
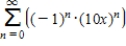

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
22
Find a power series for the function  centered at 0.
centered at 0.
A)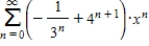
B)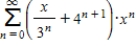
C)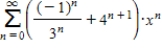
D)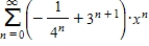
E)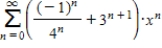
 centered at 0.
centered at 0. A)
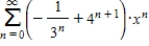
B)
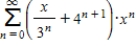
C)
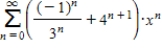
D)
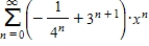
E)
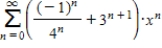

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
23
Find a power series for the function 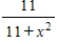 centered at 0.
centered at 0.
A)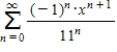
B)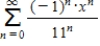
C)
D)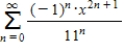
E)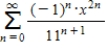
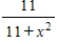 centered at 0.
centered at 0. A)
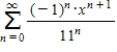
B)
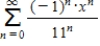
C)

D)
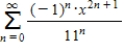
E)

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
24
Find a power series for the function  centered at 1.
centered at 1.
A)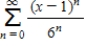
B)
C)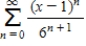
D)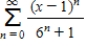
E)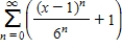
 centered at 1.
centered at 1. A)
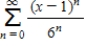
B)

C)
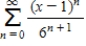
D)
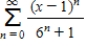
E)
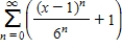

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
25
Use the power series 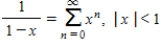 to determine a power series for the function
to determine a power series for the function 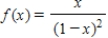 .
.
A)
B)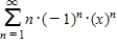
C)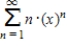
D)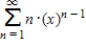
E)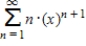
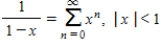 to determine a power series for the function
to determine a power series for the function 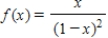 .
. A)

B)
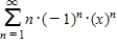
C)
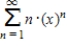
D)
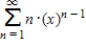
E)
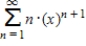

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
26
Find the radius of convergence of the power series. 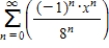
A)
B)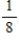
C)8
D)∞
E)64
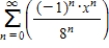
A)

B)
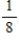
C)8
D)∞
E)64

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
27
Find a geometric power series for the function centered at 0, (i)by the technique shown in Examples 1 and 2 and (ii)by long division. 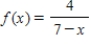
A)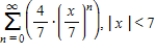
B)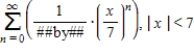
C)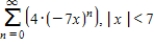
D)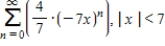
E)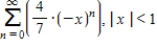
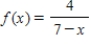
A)
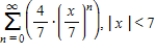
B)
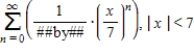
C)
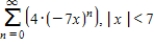
D)
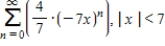
E)
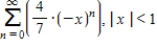

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
28
Explain how to use the geometric series  to find the series for the function
to find the series for the function 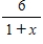 .
.
A)replace x with
B)replace x with and multiply the series by
and multiply the series by
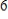
C)replace x with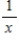 and divide the series by
and divide the series by
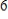
D)replace x with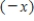 and divide the series by
and divide the series by
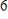
E)replace x with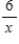
 to find the series for the function
to find the series for the function 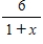 .
. A)replace x with

B)replace x with
 and multiply the series by
and multiply the series by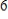
C)replace x with
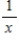 and divide the series by
and divide the series by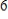
D)replace x with
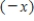 and divide the series by
and divide the series by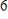
E)replace x with
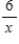

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
29
Use the power series 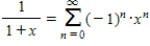 to determine a power series centered at 0 for the function
to determine a power series centered at 0 for the function 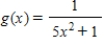 . .
. .
A)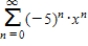
B)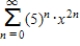
C)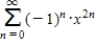
D)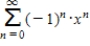
E)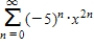
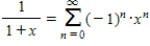 to determine a power series centered at 0 for the function
to determine a power series centered at 0 for the function 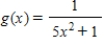 . .
. .A)
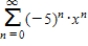
B)
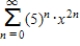
C)
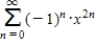
D)
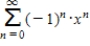
E)
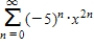

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
30
Identify the interval of convergence of a power series 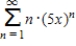 .
.
A)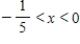
B)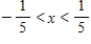
C)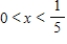
D)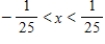
E)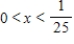
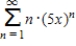 .
. A)
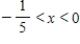
B)
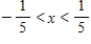
C)
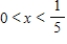
D)
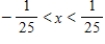
E)
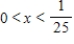

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
31
Find the sum of the convergent series 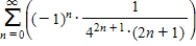 by using a well-known function.Round your answer to four decimal places.
by using a well-known function.Round your answer to four decimal places.
A)0.1419
B)0.2450
C)0.8961
D)0.1651
E)0.1974
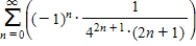 by using a well-known function.Round your answer to four decimal places.
by using a well-known function.Round your answer to four decimal places. A)0.1419
B)0.2450
C)0.8961
D)0.1651
E)0.1974

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
32
Find the sum of the convergent series 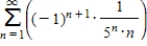 by using a well-known function.Round your answer to four decimal places.
by using a well-known function.Round your answer to four decimal places.
A)0.3365
B)0.8755
C)1.6487
D)0.1823
E)1.6094
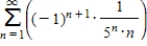 by using a well-known function.Round your answer to four decimal places.
by using a well-known function.Round your answer to four decimal places. A)0.3365
B)0.8755
C)1.6487
D)0.1823
E)1.6094

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
33
State where the power series 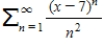 is centered.
is centered.
A)0
B)-2
C)7
D)2
E)-7
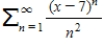 is centered.
is centered. A)0
B)-2
C)7
D)2
E)-7

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
34
Identify the interval of convergence of a power series 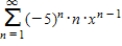 .
.
A)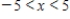
B)
C)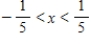
D)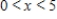
E)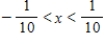
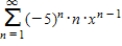 .
. A)
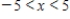
B)

C)
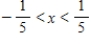
D)
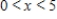
E)
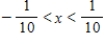

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
35
Use the series 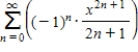 for
for 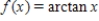 to approximate the value of
to approximate the value of  using
using 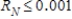 .Round your answer to three decimal places.
.Round your answer to three decimal places.
A)0.245
B)0.195
C)0.328
D)0.161
E)0.281
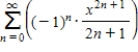 for
for 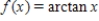 to approximate the value of
to approximate the value of  using
using 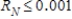 .Round your answer to three decimal places.
.Round your answer to three decimal places. A)0.245
B)0.195
C)0.328
D)0.161
E)0.281

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
36
Find the interval of convergence of the power series.(Be sure to include a check for convergence at the endpoints of the interval. ) 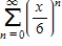
A)
B)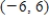
C)
D)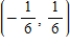
E)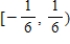
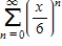
A)

B)
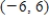
C)

D)
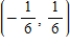
E)
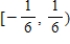

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
37
Find a power series for the function 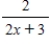 centered at 0.
centered at 0.
A)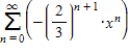
B)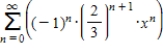
C)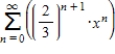
D)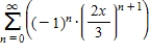
E)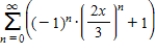
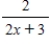 centered at 0.
centered at 0. A)
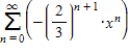
B)
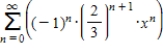
C)
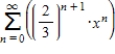
D)
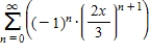
E)
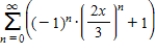

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
38
Identify the interval of convergence of a power series 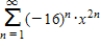 .
.
A)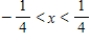
B)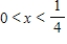
C)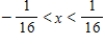
D)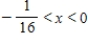
E)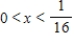
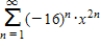 .
. A)
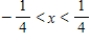
B)
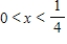
C)
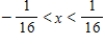
D)
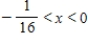
E)
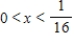

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
39
Find the radius of convergence of the power series. 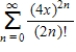
A)
B)8
C)16
D)0
E)4
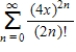
A)

B)8
C)16
D)0
E)4

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
40
Use the power series 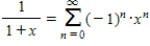 to determine a power series centered at 0 for the function
to determine a power series centered at 0 for the function 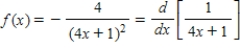 .
.
A)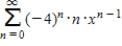
B)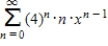
C)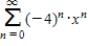
D)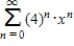
E)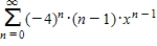
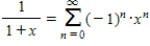 to determine a power series centered at 0 for the function
to determine a power series centered at 0 for the function 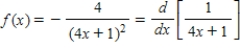 .
. A)
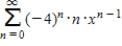
B)
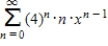
C)
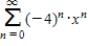
D)
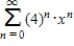
E)
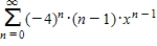

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
41
Find the interval of convergence of the power series.(Be sure to include a check for convergence at the endpoints of the interval. ) 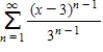
A)
B)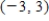
C)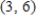
D)
E)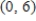
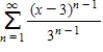
A)

B)
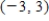
C)
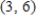
D)

E)
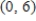

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
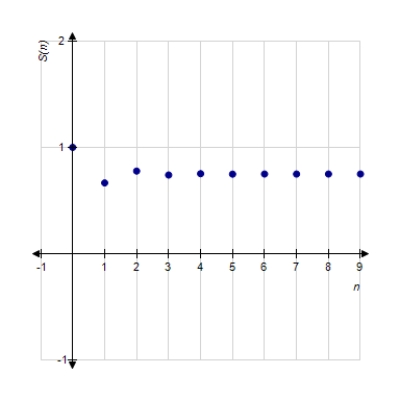
42
Identify the graph of the first 10 terms of the sequence of partial sum of the series 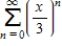 for
for 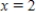 .
.
A)
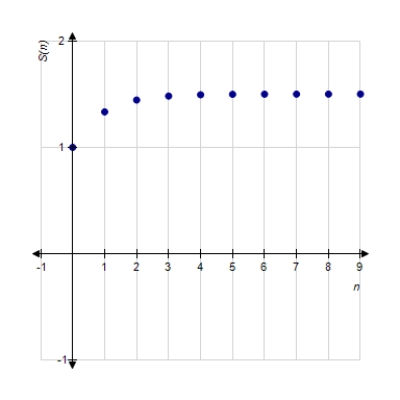
B)

C)
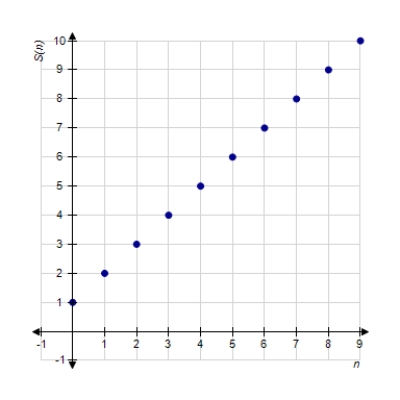
D)
E)

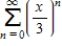 for
for 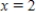 .
. A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
43
Consider the function given by 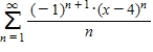 .Find the interval of convergence for
.Find the interval of convergence for 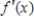 .
.
A)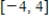
B)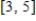
C)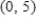
D)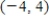
E)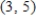
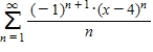 .Find the interval of convergence for
.Find the interval of convergence for 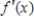 .
. A)
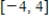
B)
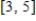
C)
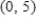
D)
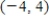
E)
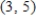

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
44
Consider the function given by 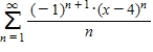 .Find the interval of convergence for
.Find the interval of convergence for 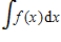 .
.
A)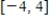
B)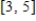
C)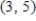
D)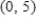
E)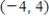
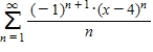 .Find the interval of convergence for
.Find the interval of convergence for 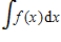 .
. A)
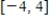
B)
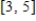
C)
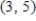
D)
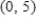
E)
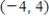

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
45
Write an equivalent series of the series 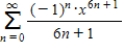 with the index of summation beginning at
with the index of summation beginning at 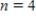 .
.
A)
B)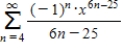
C)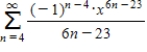
D)
E)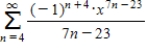
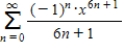 with the index of summation beginning at
with the index of summation beginning at 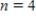 .
. A)

B)
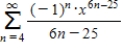
C)
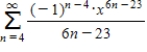
D)

E)
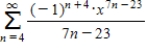

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
46
Find the differential equation having the solution 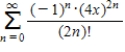 .
.
A)
B)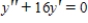
C)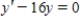
D)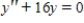
E)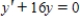
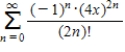 .
. A)

B)
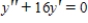
C)
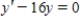
D)
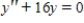
E)
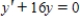

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
47
What is P1 a first-degree polynomial function whose value and slope agree with the value and slope of 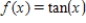 at
at 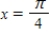 ?
?
A)P1 is the tangent line to the curve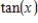 at the point
at the point
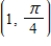 .
.
B)P1 is the tangent line to the curve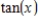 at the point
at the point
 .
.
C)P1 is the tangent line to the curve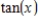 at the point
at the point
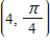 .
.
D)P1 is the tangent line to the curve at the point
at the point
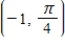 .
.
E)P1 is the tangent line to the curve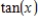 at the point
at the point
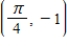 .
.
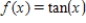 at
at 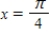 ?
? A)P1 is the tangent line to the curve
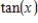 at the point
at the point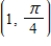 .
.B)P1 is the tangent line to the curve
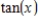 at the point
at the point .
.C)P1 is the tangent line to the curve
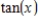 at the point
at the point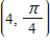 .
.D)P1 is the tangent line to the curve
 at the point
at the point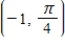 .
.E)P1 is the tangent line to the curve
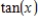 at the point
at the point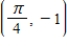 .
.
Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
48
Find the Maclaurin polynomial of degree 4 for the function. 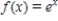
A)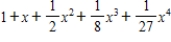
B)
C)
D)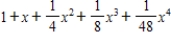
E)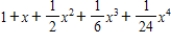
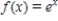
A)
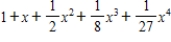
B)

C)

D)
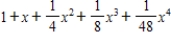
E)
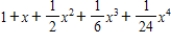

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
49
Consider the function 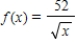 and its second-degree polynomial
and its second-degree polynomial 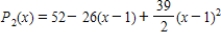 at
at 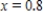 .Compute the value of
.Compute the value of 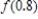 and
and  Round your answer to four decimal places.
Round your answer to four decimal places.
A)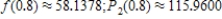
B)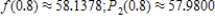
C)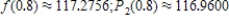
D)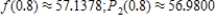
E)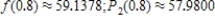
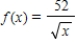 and its second-degree polynomial
and its second-degree polynomial 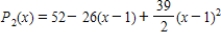 at
at 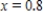 .Compute the value of
.Compute the value of 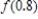 and
and  Round your answer to four decimal places.
Round your answer to four decimal places. A)
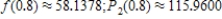
B)
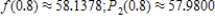
C)
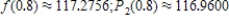
D)
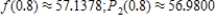
E)
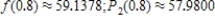

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
50
Consider the function given by  .Find the interval of convergence for
.Find the interval of convergence for 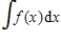 .
.
A)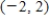
B)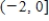
C)
D)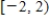
E)
 .Find the interval of convergence for
.Find the interval of convergence for 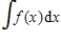 .
. A)
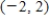
B)
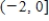
C)

D)
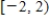
E)


Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
51
Find a first-degree polynomial function P1 whose value and slope agree with the value and slope of 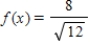 at
at 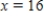 .
.
A)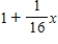
B)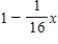
C)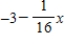
D)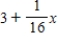
E)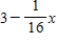
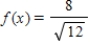 at
at 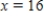 .
. A)
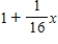
B)
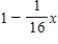
C)
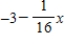
D)
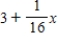
E)
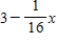

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
52
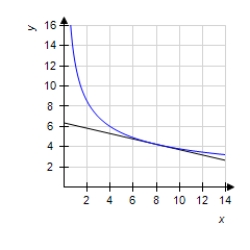
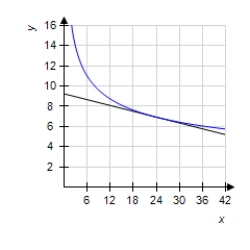
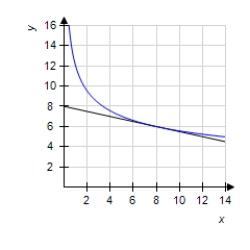
Use a graphing utility to graph 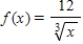 and P1,a first-degree polynomial function whose value and slope agree with the value and slope of f at
and P1,a first-degree polynomial function whose value and slope agree with the value and slope of f at 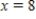 .
.
A)
B)

C)
D)
E)
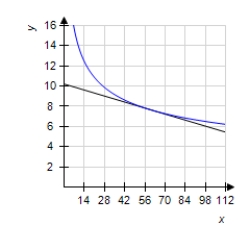
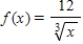 and P1,a first-degree polynomial function whose value and slope agree with the value and slope of f at
and P1,a first-degree polynomial function whose value and slope agree with the value and slope of f at 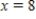 .
. A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
53
Find the interval of convergence of the power series.(Be sure to include a check for convergence at the endpoints of the interval. ) 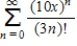
A)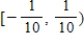
B)
C)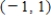
D)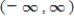
E)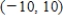
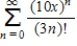
A)
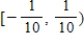
B)

C)
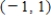
D)
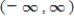
E)
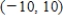

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
54
Consider the function given by 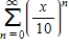 .Find the interval of convergence for
.Find the interval of convergence for 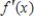 .
.
A)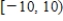
B)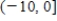
C)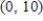
D)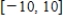
E)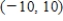
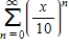 .Find the interval of convergence for
.Find the interval of convergence for 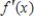 .
. A)
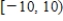
B)
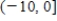
C)
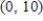
D)
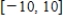
E)
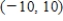

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
55
Find the interval of convergence of the power series  .(Be sure to include a check for convergence at the endpoints of the interval. )
.(Be sure to include a check for convergence at the endpoints of the interval. )
A)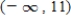
B)
C)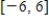
D)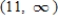
E)
 .(Be sure to include a check for convergence at the endpoints of the interval. )
.(Be sure to include a check for convergence at the endpoints of the interval. ) A)
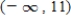
B)

C)
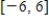
D)
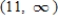
E)


Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
56
Find a first-degree polynomial function P1 whose value and slope agree with the value and slope of f at  .What is P1 called?
.What is P1 called? 
A)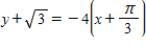 ;tangent line to
;tangent line to 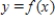 at
at
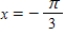
B)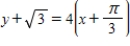 ;secant line to
;secant line to 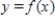 at
at
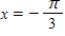
C)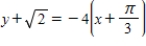 ;tangent line to
;tangent line to 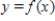 at
at
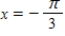
D)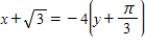 ;differential of
;differential of  at
at

E)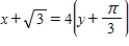 ;tangent line to
;tangent line to 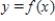 at
at

 .What is P1 called?
.What is P1 called? 
A)
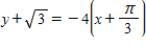 ;tangent line to
;tangent line to 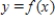 at
at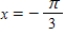
B)
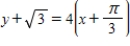 ;secant line to
;secant line to 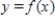 at
at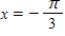
C)
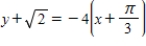 ;tangent line to
;tangent line to 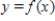 at
at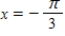
D)
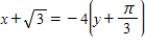 ;differential of
;differential of  at
at
E)
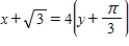 ;tangent line to
;tangent line to 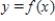 at
at

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
57
Find the interval of convergence of the power series 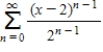 .(Be sure to include a check for convergence at the endpoints of the interval. )
.(Be sure to include a check for convergence at the endpoints of the interval. )
A)
B)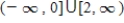
C)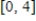
D)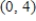
E)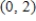
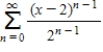 .(Be sure to include a check for convergence at the endpoints of the interval. )
.(Be sure to include a check for convergence at the endpoints of the interval. ) A)

B)
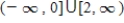
C)
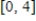
D)
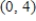
E)
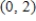

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
58
Write an equivalent series of the series 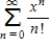 with the index of summation beginning at
with the index of summation beginning at 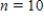 .
.
A)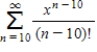
B)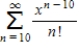
C)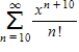
D)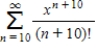
E)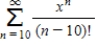
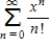 with the index of summation beginning at
with the index of summation beginning at 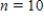 .
. A)
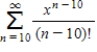
B)
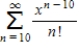
C)
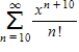
D)
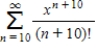
E)
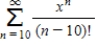

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
59
Find the interval of convergence of the power series.(Be sure to include a check for convergence at the endpoints of the interval. ) 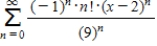
A)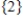
B)
C)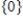
D)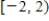
E)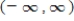
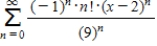
A)
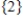
B)

C)
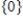
D)
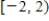
E)
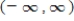

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
60
Find a first-degree polynomial function P1 whose value and slope agree with the value and slope of f at  .What is P1 called?
.What is P1 called? 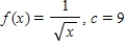
A)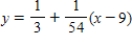 tangent line to
tangent line to  at
at
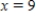
B)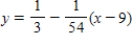 secant line to
secant line to 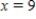
C)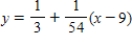 secant line to
secant line to 
D) secant line to
secant line to 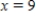
E)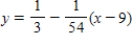 tangent line to
tangent line to 
 .What is P1 called?
.What is P1 called? 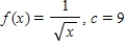
A)
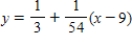 tangent line to
tangent line to  at
at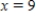
B)
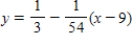 secant line to
secant line to 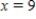
C)
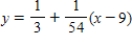 secant line to
secant line to 
D)
 secant line to
secant line to 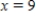
E)
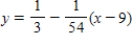 tangent line to
tangent line to 

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
61
Find the fourth degree Taylor polynomial centered at 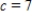 for the function.
for the function. 
A)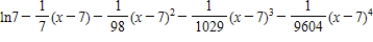
B)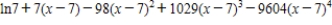
C)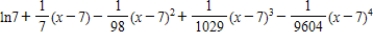
D)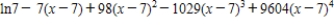
E)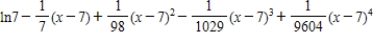
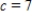 for the function.
for the function. 
A)
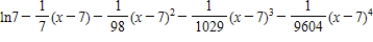
B)
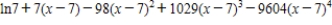
C)
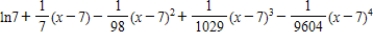
D)
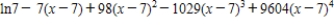
E)
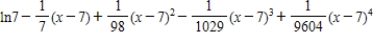

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
62
Use the Root Test to determine the convergence or divergence of the series 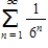 .
.
A)converges
B)diverges
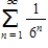 .
. A)converges
B)diverges

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
63
Find the fourth degree Maclaurin polynomial for the function. 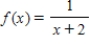
A)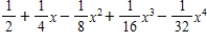
B)
C)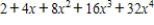
D)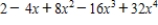
E)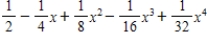
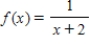
A)
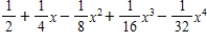
B)

C)
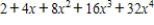
D)
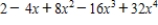
E)
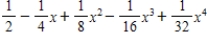

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
64
Find the third Taylor polynomial for 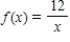 expanded about
expanded about  .
.
A)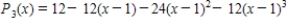
B)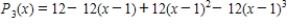
C)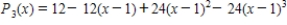
D)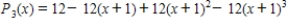
E)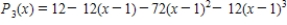
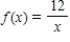 expanded about
expanded about  .
. A)
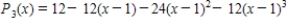
B)
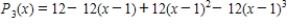
C)
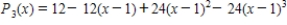
D)
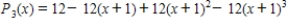
E)
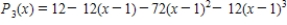

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
65
Use the Root Test to determine the convergence or divergence of the series. 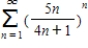
A)converges
B)diverges
C)Root Test inconclusive
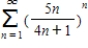
A)converges
B)diverges
C)Root Test inconclusive

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
66
Determine the degree of the Maclaurin polynomial required for the error in the approximation of the function 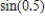 to be less than 0.001.
to be less than 0.001.
A)3
B)2
C)5
D)1
E)4
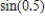 to be less than 0.001.
to be less than 0.001. A)3
B)2
C)5
D)1
E)4

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
67
Find the Maclaurin polynomial of degree two for the function  .
.
A)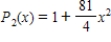
B)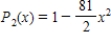
C)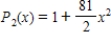
D)
E)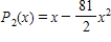
 .
. A)
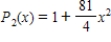
B)
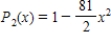
C)
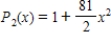
D)

E)
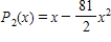

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
68
Find the Maclaurin polynomial of degree 5 for the function. 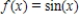
A)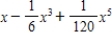
B)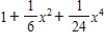
C)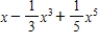
D)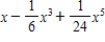
E)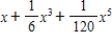
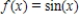
A)
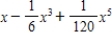
B)
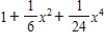
C)
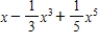
D)
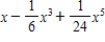
E)
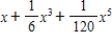

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
69
Determine the values of x for which the function 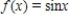 can be replaced by the Taylor polynomial
can be replaced by the Taylor polynomial 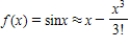 if the error cannot exceed 0.004.Round your answer to four decimal places.
if the error cannot exceed 0.004.Round your answer to four decimal places.
A)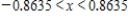
B)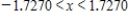
C)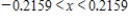
D)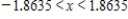
E)
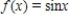 can be replaced by the Taylor polynomial
can be replaced by the Taylor polynomial 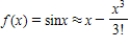 if the error cannot exceed 0.004.Round your answer to four decimal places.
if the error cannot exceed 0.004.Round your answer to four decimal places. A)
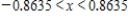
B)
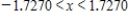
C)
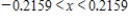
D)
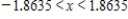
E)


Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
70
Use the Ratio Test to determine the convergence or divergence of the series. 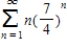
A)Ratio Test inconclusive
B)converges
C)diverges
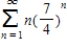
A)Ratio Test inconclusive
B)converges
C)diverges

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
71
Use the Root Test to determine the convergence or divergence of the series. 
A)Root Test inconclusive
B)converges
C)diverges

A)Root Test inconclusive
B)converges
C)diverges

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
72
Use the Root Test to determine the convergence or divergence of the series 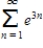 .
.
A)converges
B)diverges
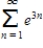 .
. A)converges
B)diverges

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
73
Use the Ratio Test to determine the convergence or divergence of the series 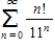 .
.
A)converges
B)diverges
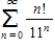 .
. A)converges
B)diverges

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
74
Use the Ratio Test to determine the convergence or divergence of the series. 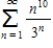
A)converges
B)diverges
C)Ratio Test inconclusive
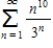
A)converges
B)diverges
C)Ratio Test inconclusive

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
75
Use the Root Test to determine the convergence or divergence of the series. 
A)Root Test inconclusive
B)converges
C)diverges

A)Root Test inconclusive
B)converges
C)diverges

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
76
Find the third degree Taylor polynomial centered at 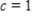 for the function.
for the function. 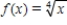
A)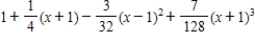
B)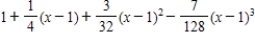
C)
D)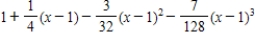
E)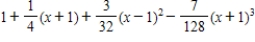
 for the function.
for the function. 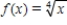
A)
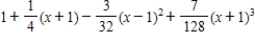
B)
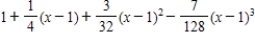
C)

D)
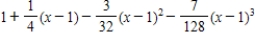
E)
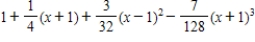

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
77
Use the Ratio Test to determine the convergence or divergence of the series 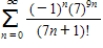 .
.
A)diverges
B)converges
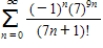 .
. A)diverges
B)converges

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
78
Find the Maclaurin polynomial of degree 3 for the function. 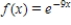
A)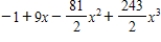
B)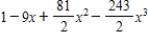
C)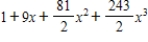
D)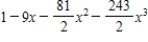
E)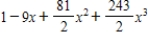
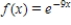
A)
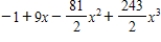
B)
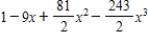
C)
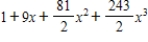
D)
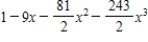
E)
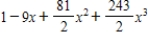

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
79
Use the Ratio Test to determine the convergence or divergence of the series. 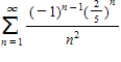
A)converges
B)Ratio Test inconclusive
C)diverges
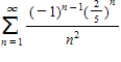
A)converges
B)Ratio Test inconclusive
C)diverges

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck
80
Find the Maclaurin polynomial of degree 4 for the function. 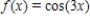
A)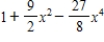
B)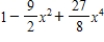
C)
D)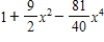
E)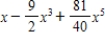
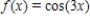
A)
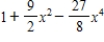
B)
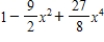
C)

D)
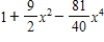
E)
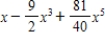

Unlock Deck
Unlock for access to all 199 flashcards in this deck.
Unlock Deck
k this deck


