Deck 8: Rotational Motion
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/76
Play
Full screen (f)
Deck 8: Rotational Motion
1
Calculate the linear speed due to the Earth's rotation for a person at a point on its surface located at 30o N latitude. The radius of the Earth is 6.40 *106 m.
A) 299 m/s
B) 233 m/s
C) 403 m/s
D) 465 m/s
A) 299 m/s
B) 233 m/s
C) 403 m/s
D) 465 m/s
403 m/s
2
Which of the first units given below can be units for angular acceleration while the second units can be units for angular velocity?
A)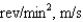
B)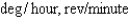
C)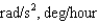
D)
A)
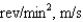
B)
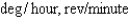
C)
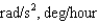
D)

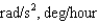
3
A bank vault is opened by applying a force of 300 N perpendicular to the plane of the door, 1.2 m from the hinges. Find the torque due to this force about an axis through the hinges.
A) 180 N·m
B) 240 N·m
C) 300 N·m
D) 360 N·m
A) 180 N·m
B) 240 N·m
C) 300 N·m
D) 360 N·m
360 N·m
4
What is the angular speed about the rotational axis of the Earth for a person standing on the surface?
A) 7.3 *10-5 rad/s
B) 3.6 *10-5 rad/s
C) 6.28 *10-5 rad/s
D) 3.14 *10-5 rad/s
A) 7.3 *10-5 rad/s
B) 3.6 *10-5 rad/s
C) 6.28 *10-5 rad/s
D) 3.14 *10-5 rad/s

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
5
Two equal magnitude forces are in opposite directions, and their lines of action are separated by distance d. These two forces are applied to a solid disk, which is mounted on a frictionless axle. If d is half the radius r of the disk, which of the following positions for the forces would give the most torque?
A) One force on a line touching the circumference of the disk and the other on a line halfway to the center.
B) One force on a line at a distance d from the center and the other on a line through the center of the disk.
C) One force on a line at a distance d/2 from the center of the disk and the other on a line at a distance d/2 on the opposite side of the center of the disk.
D) Since all the above orientations give the same torque, choose this answer.
A) One force on a line touching the circumference of the disk and the other on a line halfway to the center.
B) One force on a line at a distance d from the center and the other on a line through the center of the disk.
C) One force on a line at a distance d/2 from the center of the disk and the other on a line at a distance d/2 on the opposite side of the center of the disk.
D) Since all the above orientations give the same torque, choose this answer.

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
6
A 0.60-kg rock is swung in a circular path and in a vertical plane on a 0.25-m length string. At the top of the path, the angular speed is 12.0 rad/s. What is the tension in the string at that point?
A) 7.9 N
B) 16 N
C) 22 N
D) 6.5 N
A) 7.9 N
B) 16 N
C) 22 N
D) 6.5 N

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
7
A 0.200-kg object is swung in a circular path and in a vertical plane on a 0.500-m length string. If the angular speed at the bottom is 8.00 rad/s, what is the tension in the string when the object is at the bottom of the circle?
A) 8.36 N
B) 10.5 N
C) 16.7 N
D) 6.40 N
A) 8.36 N
B) 10.5 N
C) 16.7 N
D) 6.40 N

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
8
A 3.0-m rod is pivoted about its left end. A force of 10 N is applied perpendicular to the rod at a distance of 1.2 m from the pivot causing a ccw torque, and a force of 5.2 N is applied at the end of the rod 3.0 m from the pivot. The 5.2-N force is at an angle of 30o to the rod and causes a cw torque. What is the net torque about the pivot? (Take ccw as positive.)
A) "18 N·m"
B) "-18 N·m"
C) "4.2 N·m"
D) "-4.2 N·m"
A) "18 N·m"
B) "-18 N·m"
C) "4.2 N·m"
D) "-4.2 N·m"

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
9
Calculate the linear speed due to the Earth's rotation for a person at the equator of the Earth. The radius of the Earth is 6.40 *106 m.
A) 74.0 m/s
B) 233 m/s
C) 465 m/s
D) 73.0 m/s
A) 74.0 m/s
B) 233 m/s
C) 465 m/s
D) 73.0 m/s

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
10
Consider a point on a bicycle wheel as the wheel turns about a fixed axis, neither speeding up nor slowing down. Compare the linear and angular accelerations of the point.
A) Both are zero.
B) Only the angular acceleration is zero.
C) Only the linear acceleration is zero.
D) Neither is zero.
A) Both are zero.
B) Only the angular acceleration is zero.
C) Only the linear acceleration is zero.
D) Neither is zero.

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
11
A ceiling fan is turned on and reaches an angular speed of 120 rev/min in 20 s. It is then turned off and coasts to a stop in an additional 60 s. The ratio of the average angular acceleration for the first 20 s to that for the last 60 s is which of the following?
A) 3
B) -3
C) 0.3
D) -0.3
A) 3
B) -3
C) 0.3
D) -0.3

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
12
The torque caused by a force F applied at a distance r from the axis of rotation, is given by 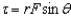 , where
, where  is the angle between
is the angle between 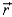 and
and 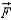 . If the magnitudes r and F remain the same, what other angle
. If the magnitudes r and F remain the same, what other angle 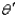 will produce the same torque as was produced at angle
will produce the same torque as was produced at angle  if
if  was less than 90°?
was less than 90°?
A)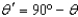
B)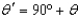
C)
D) None of the above.
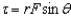 , where
, where  is the angle between
is the angle between 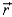 and
and 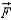 . If the magnitudes r and F remain the same, what other angle
. If the magnitudes r and F remain the same, what other angle 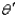 will produce the same torque as was produced at angle
will produce the same torque as was produced at angle  if
if  was less than 90°?
was less than 90°?A)
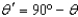
B)
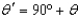
C)

D) None of the above.

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
13
A circular disk is rotating about a central axis with an angular velocity which is increasing at a constant rate. Point 1 on the disk is located at a distance  from the center of the disk and point 2 on the disk is located at a distance
from the center of the disk and point 2 on the disk is located at a distance 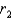 from the center of the disk. If
from the center of the disk. If  , which of the following ratios is the largest? 1 and 2 refer to being at points 1 and 2 in the subscripts below, all values occurring at the same time.
, which of the following ratios is the largest? 1 and 2 refer to being at points 1 and 2 in the subscripts below, all values occurring at the same time.
A)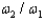
B)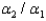
C)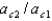
D) All the ratios are 1 so none is larger.
 from the center of the disk and point 2 on the disk is located at a distance
from the center of the disk and point 2 on the disk is located at a distance 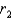 from the center of the disk. If
from the center of the disk. If  , which of the following ratios is the largest? 1 and 2 refer to being at points 1 and 2 in the subscripts below, all values occurring at the same time.
, which of the following ratios is the largest? 1 and 2 refer to being at points 1 and 2 in the subscripts below, all values occurring at the same time.A)
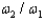
B)
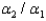
C)
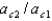
D) All the ratios are 1 so none is larger.

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
14
Suppose a car has a 100,000 mile 10 year warranty on the wheels and axles of a car. How may turns will the wheels of 0.80-m diameter make if both the mileage and time warranties run out simultaneously and what will be the average angular velocities of the wheel? Assume that a mile = 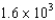 m and that a year is
m and that a year is 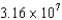 s.
s.
A) ,
, 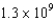 rad/y
rad/y
B) , 13 rad/s
, 13 rad/s
C)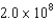 , 0.51 rad/s
, 0.51 rad/s
D)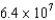 , 1.3 rad/s
, 1.3 rad/s
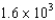 m and that a year is
m and that a year is 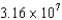 s.
s.A)
 ,
, 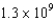 rad/y
rad/yB)
 , 13 rad/s
, 13 rad/sC)
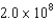 , 0.51 rad/s
, 0.51 rad/sD)
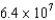 , 1.3 rad/s
, 1.3 rad/s
Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
15
Consider a point on a bicycle wheel as the wheel turns about a fixed axis, neither speeding up nor slowing down. Compare the linear and angular velocities of the point.
A) Both are constant.
B) Only the angular velocity is constant.
C) Only the linear velocity is constant.
D) Neither is constant.
A) Both are constant.
B) Only the angular velocity is constant.
C) Only the linear velocity is constant.
D) Neither is constant.

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
16
Consider a point on a bicycle wheel as the wheel makes exactly four complete revolutions about a fixed axis. Compare the linear and angular displacements of the point.
A) Both are zero.
B) Only the angular displacement is zero.
C) Only the linear displacement is zero.
D) Neither is zero.
A) Both are zero.
B) Only the angular displacement is zero.
C) Only the linear displacement is zero.
D) Neither is zero.

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
17
A grindstone spinning at the rate of 12 rev/s has what approximate angular speed?
A) 3.2 rad/s
B) 26 rad/s
C) 52 rad/s
D) 75 rad/s
A) 3.2 rad/s
B) 26 rad/s
C) 52 rad/s
D) 75 rad/s

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
18
Two points on a merry-go-round are located at distances from the center r1 and r2, where r1 < r2. While the merry-go-round is in the process of speeding up to operational speed, which of the following equations involving magnitudes of angular speed, angular acceleration, and linear speed for these points is incorrect?
A)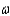 1 =
1 = 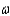 2
2
B) a1 = a2
C) v1 = v2
D) All of the equations are correct.
A)
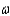 1 =
1 = 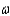 2
2B) a1 = a2
C) v1 = v2
D) All of the equations are correct.

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
19
A spool of thread has an average radius of 1.00 cm. If the spool contains 126 m of thread, how many turns of thread are on the spool? "Average radius" allows us to not need to treat the layering of threads on lower layers.
A) 100
B) 1000
C) 2000
D) 62,800
A) 100
B) 1000
C) 2000
D) 62,800

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
20
A 0.12-m radius grinding wheel takes 2.1 s to speed up from 2.0 rad/s to 11.0 rad/s. What is the wheel's average angular acceleration?
A) 9.6 rad/s2
B) 4.3 rad/s2
C) 1.6 rad/s2
D) 0.33 rad/s2
A) 9.6 rad/s2
B) 4.3 rad/s2
C) 1.6 rad/s2
D) 0.33 rad/s2

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
21
A rod of length L is pivoted about its left end and has a force F applied perpendicular to the other end. The force F is now removed and another force F' is applied at the midpoint of the rod. If F' is at an angle of 30o with respect to the rod, what is its magnitude if the resulting torque is the same as when F was applied?
A) F
B) 2F
C) 3F
D) 4F
A) F
B) 2F
C) 3F
D) 4F

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
22
A meterstick is supported by a knife-edge at the 50-cm mark. Doug hangs masses of 0.40 and 0.60 kg from the 35-cm and 80-cm marks, respectively. Where should Doug hang a third mass of 0.30 kg to keep the stick balanced?
A) 20 cm
B) 70 cm
C) 30 cm
D) 10 cm
A) 20 cm
B) 70 cm
C) 30 cm
D) 10 cm

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
23
The quantity "moment of inertia" (in terms of the fundamental quantities of mass, length, and time) is equivalent to:
A) ML2T-2.
B) ML.
C) ML2.
D) ML-1T-2.
A) ML2T-2.
B) ML.
C) ML2.
D) ML-1T-2.

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
24
Tasha has mass 20 kg and wants to use a 6.0-m board of mass 10 kg as a seesaw. Her friends are busy, so Tasha seesaws by herself by putting the support at the system's center of gravity when she sits on one end of the board. How far is she from the support point?
A) 2.0 m
B) 1.0 m
C) 0.67 m
D) 0.33 m
A) 2.0 m
B) 1.0 m
C) 0.67 m
D) 0.33 m

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
25
A uniform bridge span weighs 70.0 *103 N and is 40.0 m long. An automobile weighing 15.0 *103 N is parked with its center of gravity located 12.0 m from the right pier. What upward support force does the right pier provide?
A) 24.5 *103 N
B) 35.5 *103 N
C) 65.0 *103 N
D) 45.5*103 N
A) 24.5 *103 N
B) 35.5 *103 N
C) 65.0 *103 N
D) 45.5*103 N

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
26
An 800-N billboard worker stands on a 4.0-m scaffold supported by vertical ropes at each end. If the scaffold weighs 500 N and the worker stands 1.0 m from one end, what is the tension in the rope farthest from the worker?
A) 450 N
B) 500 N
C) 800 N
D) 850 N
A) 450 N
B) 500 N
C) 800 N
D) 850 N

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
27
A uniform meterstick balances on a fulcrum placed at the 40-cm mark when a weight W is placed at the 20-cm mark. What is the weight of the meterstick?
A) W
B) 2W
C) W/2
D) 0.4W
A) W
B) 2W
C) W/2
D) 0.4W

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
28
A uniform 1.0-N meterstick is suspended horizontally by vertical strings attached at each end. A 2.0-N weight is suspended from the 10-cm position on the stick, another 2.0-N weight is suspended from the 50-cm position, and a 3.0-N weight is suspended from the 60-cm position. What is the tension in the string attached at the 100-cm end of the stick?
A) 1.9 N
B) 3.0 N
C) 3.5 N
D) 4.0 N
A) 1.9 N
B) 3.0 N
C) 3.5 N
D) 4.0 N

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
29
A uniform rod of length L and weight W is placed on a fulcrum a distance of 0.4L from the left end. On the left end is placed a mass of weight 2W and on the right end a vertical force F is applied to keep the system in balance. What are the magnitude and direction of the force F?
A)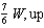
B)
C)
D)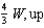
A)
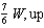
B)

C)

D)
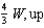

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
30
An 80-kg man is one fourth of the way up a 10-m ladder that is resting against a smooth, frictionless wall. If the ladder has a mass of 30 kg and it makes an angle of 60 with the ground, find the force of friction of the ground on the foot of the ladder.
A) 7.8 * 102 N
B) 2.0 * 102 N
C) 50 N
D) 1.7 * 102 N
A) 7.8 * 102 N
B) 2.0 * 102 N
C) 50 N
D) 1.7 * 102 N

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
31
A 100-N uniform ladder, 8.0 m long, rests against a smooth vertical wall. The coefficient of static friction between ladder and floor is 0.30. What minimum angle can the ladder make with the floor before it slips?
A) 22
B) 51
C) 18
D) 59
A) 22
B) 51
C) 18
D) 59

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
32
A 4.0-kg mass is placed at (3.0, 4.0) m, and a 7.0-kg mass is placed at (3.0, -4.0) m. What is the moment of inertia of this system of masses about the y axis?
A) 275 kg·m2
B) 90 kg·m2
C) 176 kg·m2
D) 99 kg·m2
A) 275 kg·m2
B) 90 kg·m2
C) 176 kg·m2
D) 99 kg·m2

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
33
A 8.4-kg mass is placed at (3.0, 4.0) m. Where can a 4.2-kg mass be placed so that the moment of inertia about the z axis is zero?
A) (-3.0, -4.0) m
B) (-6.0, -8.0) m
C) (-1.5, -2.0) m
D) There is no position giving this result.
A) (-3.0, -4.0) m
B) (-6.0, -8.0) m
C) (-1.5, -2.0) m
D) There is no position giving this result.

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
34
A bucket filled with water has a mass of 23 kg and is attached to a rope, which in turn, is wound around a 0.080-m radius cylinder at the top of a well. What torque does the weight of water and bucket produce on the cylinder if the cylinder is not permitted to rotate? (g = 9.8 m/s2)
A) 34 N·m
B) 18 N·m
C) 11 N·m
D) 36 N·m
A) 34 N·m
B) 18 N·m
C) 11 N·m
D) 36 N·m

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
35
An 800-N billboard worker stands on a 4.0-m scaffold weighing 500 N and supported by vertical ropes at each end. How far would the worker stand from one of the supporting ropes to produce a tension of 770 N in that rope?
A) 1.4 m
B) 2.0 m
C) 2.5 m
D) 2.7 m
A) 1.4 m
B) 2.0 m
C) 2.5 m
D) 2.7 m

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
36
A uniform, horizontal beam of length 6.0 m and weight 140 N is attached at one end to a wall by a pin connection (so that it may rotate). A cable attached to the wall above the pin supports the opposite end. The cable makes an angle of 60 with the horizontal. What is the tension in the cable needed to maintain the beam in equilibrium?
A) 81 N
B) 69 N
C) 70 N
D) 140 N
A) 81 N
B) 69 N
C) 70 N
D) 140 N

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
37
A woman who weighs 500 N stands on an 8.0 m long board that weighs 100 N. The board is supported at each end. The support force at the right end is 2 times the support force at the left end. How far from the right end is the woman standing?
A) 4.0 m
B) 2.4 m
C) 2.7 m
D) 1.6 m
A) 4.0 m
B) 2.4 m
C) 2.7 m
D) 1.6 m

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
38
Two children seat themselves on a seesaw. The one on the left has a weight of 400 N while the one on the right weighs 300 N. The fulcrum is at the midpoint of the seesaw. If the child on the right is not at the end but is 1.50 m from the fulcrum and the seesaw is balanced, what is the torque provided by the weight of the child on the left? (Take ccw as positive.)
A) 600 N·m
B) 450 N·m
C) -600 N·m
D) -450 N·m
A) 600 N·m
B) 450 N·m
C) -600 N·m
D) -450 N·m

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
39
A bucket of water with total mass 23 kg is attached to a rope, which in turn, is wound around a 0.080-m radius cylinder at the top of a well. A crank with a turning radius of 0.25 m is attached to the end of the cylinder. What minimum force directed perpendicular to the crank handle is required to just raise the bucket? (Assume the rope's mass is negligible, that the cylinder turns on frictionless bearings, and that g = 9.8 m/s2.)
A) 45 N
B) 68 N
C) 72 N
D) 135 N
A) 45 N
B) 68 N
C) 72 N
D) 135 N

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
40
A 4.0-kg mass is placed at (3.0, 4.0) m, and a 7.0-kg mass is placed at (3.0, -4.0) m. What is the moment of inertia of this system of masses about the x axis?
A) 275 kg·m2
B) 90 kg·m2
C) 176 kg·m2
D) 99 kg·m2
A) 275 kg·m2
B) 90 kg·m2
C) 176 kg·m2
D) 99 kg·m2

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
41
A fan blade, initially at rest, rotates with a constant acceleration of 0.025 rad/s2. What is the time interval required for it to reach a 10-rad displacement after starting from rest?
A) 28 s
B) 2.0 s
C) 56 s
D) 18 s
A) 28 s
B) 2.0 s
C) 56 s
D) 18 s

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
42
A fan blade, initially at rest, rotates with a constant acceleration of 0.025 rad/s2. What is its angular speed at the instant it goes through an angular displacement of 10 rad?
A) 0.025 rad/s
B) 0.11 rad/s
C) 0.46 rad/s
D) 0.71 rad/s
A) 0.025 rad/s
B) 0.11 rad/s
C) 0.46 rad/s
D) 0.71 rad/s

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
43
A ceiling fan is turned on and reaches an angular speed of 60 rev/min in 20 s. It is then turned off and coasts to a stop in 40 s. In the one minute of rotation, through how many revolutions did the fan turn?
A) 30
B) 60
C) 0
D) 600
A) 30
B) 60
C) 0
D) 600

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
44
A baseball pitcher loosens up his pitching arm. He tosses a 0.20-kg ball using only the rotation of his forearm, 0.32 m in length, to accelerate the ball. What is the moment of inertia of the ball alone as it moves in a circular arc with a radius of 0.32 m?
A) 1.5 *10-2 kg·m2
B) 2.0 *10-2 kg·m2
C) 4.0 *10-2 kg·m2
D) 7.6 *10-2 kg·m2
A) 1.5 *10-2 kg·m2
B) 2.0 *10-2 kg·m2
C) 4.0 *10-2 kg·m2
D) 7.6 *10-2 kg·m2

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
45
A Ferris wheel starts at rest and builds up to a final angular speed of 0.70 rad/s while rotating through an angular displacement of 2.5 rad. What is its average angular acceleration?
A) 0.098 rad/s2
B) 0.05 rad/s2
C) 1.8 rad/s2
D) 0.60 rad/s2
A) 0.098 rad/s2
B) 0.05 rad/s2
C) 1.8 rad/s2
D) 0.60 rad/s2

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
46
A bucket of water with total mass 23 kg is attached to a rope, which in turn is wound around a 0.050-m radius cylinder at the top of a well. The bucket is raised to the top of the well and released. The bucket is moving with a speed of 6.0 m/s upon hitting the water surface in the well. What is the angular speed of the cylinder at this instant?
A) 39 rad/s
B) 79 rad/s
C) 120 rad/s
D) 160 rad/s
A) 39 rad/s
B) 79 rad/s
C) 120 rad/s
D) 160 rad/s

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
47
A Ferris wheel, rotating initially at an angular speed of 0.16 rad/s, accelerates over a 7.0-s interval at a rate of 0.050 rad/s2. What is its angular speed after this 7.0-s interval?
A) 0.46 rad/s
B) 0.85 rad/s
C) 0.51 rad/s
D) 1.2 rad/s
A) 0.46 rad/s
B) 0.85 rad/s
C) 0.51 rad/s
D) 1.2 rad/s

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
48
An object consists of a rod (of length 3.0 m and negligible moment of inertia) to which four small 2.0-kg masses are attached, one at each end and one at each point on the rod 1.0 m from each end. (The masses are one meter apart.) The moment of inertia of this object about an axis perpendicular to the rod and through one of the end masses:
A) is 28 kg·m2.
B) is 12 kg·m2.
C) is 4 kg·m2.
D) cannot be uniquely determined until it is stated which inner mass the axis goes through.
A) is 28 kg·m2.
B) is 12 kg·m2.
C) is 4 kg·m2.
D) cannot be uniquely determined until it is stated which inner mass the axis goes through.

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
49
A majorette takes two batons and fastens them together in the middle at right angles to make an "x" shape. Each baton was 0.90 m long and each ball on the end is 0.20 kg. (Ignore the mass of the rods.) What is the moment of inertia if the arrangement is spun around an axis through the center perpendicular to both rods?
A) 0.064 kg·m2
B) 0.096 kg·m2
C) 0.075 kg·m2
D) 0.16 kg·m2
A) 0.064 kg·m2
B) 0.096 kg·m2
C) 0.075 kg·m2
D) 0.16 kg·m2

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
50
A bowling ball has a mass of 7.0 kg, a moment of inertia of 2.8 * 10-2 kg·m2, and a radius of 0.10 m. If it rolls down the lane without slipping at a linear speed of 5.0 m/s, what is its angular speed?
A) 0.80 rad/s
B) 50 rad/s
C) 0.050 rad/s
D) 40 rad/s
A) 0.80 rad/s
B) 50 rad/s
C) 0.050 rad/s
D) 40 rad/s

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
51
A non-uniform rod of length L and mass M is pivoted about one end. The moment of inertia of this rod about its pivoted end is 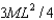 . Attached to the rod is a mass M at its midpoint and at the end opposite the pivot is another mass 2M. What is the moment of inertia of this configuration about the pivot?
. Attached to the rod is a mass M at its midpoint and at the end opposite the pivot is another mass 2M. What is the moment of inertia of this configuration about the pivot?
A)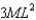
B)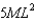
C)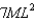
D) None of the above answers is correct.
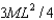 . Attached to the rod is a mass M at its midpoint and at the end opposite the pivot is another mass 2M. What is the moment of inertia of this configuration about the pivot?
. Attached to the rod is a mass M at its midpoint and at the end opposite the pivot is another mass 2M. What is the moment of inertia of this configuration about the pivot?A)
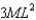
B)
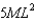
C)
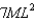
D) None of the above answers is correct.

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
52
A uniform rod of length L and mass M is pivoted about one end. Attached to the rod is a mass M at its midpoint and at the end opposite the pivot is another mass M. What is the moment of inertia of this configuration about the pivot?
A)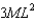
B)
C)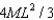
D)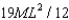
A)
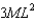
B)

C)
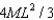
D)
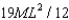

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
53
Starting from rest, a wheel undergoes constant angular acceleration for a period of time T. At what time after the start of rotation does the wheel reach an angular speed equal to its average angular speed for this interval?
A) 0.25T
B) 0.50T
C) 0.67T
D) 0.71T
A) 0.25T
B) 0.50T
C) 0.67T
D) 0.71T

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
54
Two hoops or rings (I = MR2) are centered, lying on a turntable. The smaller ring has radius = 0.050 m; the larger has radius = 0.10 m. Both have a mass of 6.0 kg. What is the total moment of inertia as the turntable spins? Ignore the mass of the turntable.
A) 0.030 kg·m2
B) 0.0075 kg·m2
C) 0.038 kg·m2
D) 0.075 kg·m2
A) 0.030 kg·m2
B) 0.0075 kg·m2
C) 0.038 kg·m2
D) 0.075 kg·m2

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
55
A 4.0-kg mass is placed at (3.0, 4.0) m, and a 7.0 kg-mass is placed at (3.0, -.0) m. What is the moment of inertia of this system of masses about the z axis?
A) 275 kg·m2
B) 90 kg·m2
C) 250 kg·m2
D) 180 kg·m2
A) 275 kg·m2
B) 90 kg·m2
C) 250 kg·m2
D) 180 kg·m2

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
56
Starting from rest, a wheel undergoes constant angular acceleration for a period of time T. At which of the following times does the average angular acceleration equal the instantaneous angular acceleration?
A) 0.50 T
B) 0.67 T
C) 0.71 T
D) all of the above
A) 0.50 T
B) 0.67 T
C) 0.71 T
D) all of the above

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
57
The Earth moves about the Sun in an elliptical orbit. As the Earth moves closer to the Sun, which of the following best describes the Earth-Sun system's moment of inertia?
A) decreases
B) increases
C) remains constant
D) None of the above choices are valid.
A) decreases
B) increases
C) remains constant
D) None of the above choices are valid.

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
58
A Ferris wheel, rotating initially at an angular speed of 0.500 rad/s, accelerates over a 7.00-s interval at a rate of 0.0500 rad/s2. What angular displacement does the Ferris wheel undergo in this 7.00-s interval?
A) 4.48 rad
B) 2.50 rad
C) 4.73 rad
D) 0.500 rad
A) 4.48 rad
B) 2.50 rad
C) 4.73 rad
D) 0.500 rad

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
59
A 0.30-m radius automobile tire rotates how many rad after starting from rest and accelerating at a constant 3.0 rad/s2 over a 5.0-s interval?
A) 12 rad
B) 25 rad
C) 37 rad
D) 0.50 rad
A) 12 rad
B) 25 rad
C) 37 rad
D) 0.50 rad

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
60
A majorette takes two batons and fastens them together in the middle at right angles to make an "x" shape. Each baton was 0.90 m long and each ball on the end is 0.20 kg. (Ignore the mass of the rods.) What is the moment of inertia if the arrangement is spun around an axis formed by one of the batons?
A) 0.081 kg·m2
B) 0.064 kg·m2
C) 0.19 kg·m2
D) 0.32 kg·m2
A) 0.081 kg·m2
B) 0.064 kg·m2
C) 0.19 kg·m2
D) 0.32 kg·m2

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
61
A disk has a moment of inertia of 3.0 *10-4 kg·m2 and rotates with an angular speed of 3.5 rad/sec. What net torque must be applied to bring it to rest within 3.7 s?
A) 4.6 * 10-4 N·m
B) 7.5 * 10-4 N·m
C) 3.5 *10-4 N·m
D) 2.8 * 10-4 N·m
A) 4.6 * 10-4 N·m
B) 7.5 * 10-4 N·m
C) 3.5 *10-4 N·m
D) 2.8 * 10-4 N·m

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
62
A bucket in an old well is hoisted upward by a rope which winds up on a cylinder having a radius of 0.050 m. How many rev/s must the cylinder turn if the bucket is raised at a speed of 0.20 m/s?
A) 3.0 rev/s
B) 1.5 rev/s
C) 0.48 rev/s
D) 0.64 rev/s
A) 3.0 rev/s
B) 1.5 rev/s
C) 0.48 rev/s
D) 0.64 rev/s

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
63
A 0.40-m radius automobile tire accelerates from rest at a constant 2.0 rad/s2. What is the centripetal acceleration of a point on the outer edge of the tire after 5.0 s?
A) 4.0 m/s2
B) 40 m/s2
C) 44 m/s2
D) 400 m/s2
A) 4.0 m/s2
B) 40 m/s2
C) 44 m/s2
D) 400 m/s2

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
64
A ventilation fan with a moment of inertia of 0.034 kg·m2 has a net torque of 0.14 N·m applied to it. What angular acceleration does it experience?
A) 5.3 rad/s2
B) 4.1 rad/s2
C) 3.2 rad/s2
D) 0.31 rad/s2
A) 5.3 rad/s2
B) 4.1 rad/s2
C) 3.2 rad/s2
D) 0.31 rad/s2

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
65
A ventilation fan has blades 0.25 m in radius rotating at 30 rpm. What is the tangential speed of each blade tip?
A) 0.02 m/s
B) 0.52 m/s
C) 0.79 m/s
D) 20 m/s
A) 0.02 m/s
B) 0.52 m/s
C) 0.79 m/s
D) 20 m/s

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
66
If a nonzero torque is applied to an object, that object will experience:
A) a constant angular speed.
B) an angular acceleration.
C) a constant moment of inertia.
D) an increasing moment of inertia.
A) a constant angular speed.
B) an angular acceleration.
C) a constant moment of inertia.
D) an increasing moment of inertia.

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
67
A solid sphere of radius R is placed on an incline that makes an angle  with the horizontal. The sphere rolls down the incline without slipping. What is its acceleration?
with the horizontal. The sphere rolls down the incline without slipping. What is its acceleration?
A)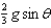
B)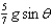
C)
D)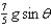
 with the horizontal. The sphere rolls down the incline without slipping. What is its acceleration?
with the horizontal. The sphere rolls down the incline without slipping. What is its acceleration?A)
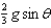
B)
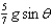
C)

D)
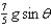

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
68
An automobile accelerates from zero to 30 m/s in 6.0 s. The wheels have a diameter of 1.0 m. What is the average angular acceleration of each wheel?
A) 5.0 rad/s2
B) 10 rad/s2
C) 25 rad/s2
D) 40 rad/s2
A) 5.0 rad/s2
B) 10 rad/s2
C) 25 rad/s2
D) 40 rad/s2

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
69
A solid cylinder (I = MR2/2) has a string wrapped around it many times. When I release the cylinder, holding on to the string, the cylinder falls and spins as the string unwinds. What is the downward acceleration of the cylinder as it falls?
A) 0
B) 4.9 m/s2
C) 6.5 m/s2
D) 9.8 m/s2
A) 0
B) 4.9 m/s2
C) 6.5 m/s2
D) 9.8 m/s2

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
70
A bucket of water with total mass 30 kg is attached to a rope, which in turn is wound around a 0.050 m radius cylinder at the top of a well. A crank with a turning radius of 0.25 m is attached to the end of the cylinder and the moment of inertia of cylinder and crank is 0.12 kg*m2. If the bucket is raised to the top of the well and released, what is the acceleration of the bucket as it falls toward the bottom of the well? (Assume rope's mass is negligible, that cylinder turns on frictionless bearings and that g = 9.8 m/s2.)
A) 3.2 m/s2
B) 3.8 m/s2
C) 7.4 m/s2
D) 9.8 m/s2
A) 3.2 m/s2
B) 3.8 m/s2
C) 7.4 m/s2
D) 9.8 m/s2

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
71
A baseball pitcher loosens up his pitching arm. He tosses a 0.15-kg ball using only the rotation of his forearm, 0.32 m in length, to accelerate the ball. If the ball starts at rest and is released with a speed of 24 m/s in a time of 0.40 s, what torque is applied to the ball while being held by the pitcher's hand to produce the angular acceleration?
A) 1.1 N·m
B) 11 N·m
C) 2.9 N·m
D) 1.4 N·m
A) 1.1 N·m
B) 11 N·m
C) 2.9 N·m
D) 1.4 N·m

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
72
A baseball pitcher, loosening up his arm before a game, tosses a 0.15-kg ball using only the rotation of his forearm, 0.32 m in length, to accelerate the ball. If the ball starts at rest and is released with a speed of 24 m/s in a time of 0.40 s, what is the average angular acceleration of the arm and ball?
A) 0.067 rad/s2
B) 94 rad/s2
C) 15 rad/s2
D) 190 rad/s2
A) 0.067 rad/s2
B) 94 rad/s2
C) 15 rad/s2
D) 190 rad/s2

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
73
A rod of length L is hinged at one end. The moment of inertia as the rod rotates around that hinge is ML2/3. Suppose a 2.50 m rod with a mass of 3.00 kg is hinged at one end and is held in a horizontal position. The rod is released as the free end is allowed to fall. What is the angular acceleration as it is released?
A) 3.70 rad/s2
B) 7.35 rad/s2
C) 5.88 rad/s2
D) 4.90 rad/s2
A) 3.70 rad/s2
B) 7.35 rad/s2
C) 5.88 rad/s2
D) 4.90 rad/s2

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
74
A 0.20-m radius grinding wheel starts at rest and develops an angular speed of 12.0 rad/s in 4.0 s. What is the average tangential acceleration of a point on the wheel's edge?
A) 0.45 m/s2
B) 0.60 m/s2
C) 28 m/s2
D) 14 m/s2
A) 0.45 m/s2
B) 0.60 m/s2
C) 28 m/s2
D) 14 m/s2

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
75
A 0.40-m radius automobile tire accelerates from rest at a constant 2.0 rad/s2 over a 5.0 s interval. What is the tangential component of acceleration for a point on the outer edge of the tire during the 5.0-s interval?
A) 33 m/s2
B) 0.80 m/s2
C) 0.60 m/s2
D) 0.30 m/s2
A) 33 m/s2
B) 0.80 m/s2
C) 0.60 m/s2
D) 0.30 m/s2

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck
76
According to Newton's second law, the angular acceleration experienced by an object is directly proportional to:
A) its moment of inertia.
B) the net applied torque.
C) the object's size.
D) choices a and b above which are both valid.
A) its moment of inertia.
B) the net applied torque.
C) the object's size.
D) choices a and b above which are both valid.

Unlock Deck
Unlock for access to all 76 flashcards in this deck.
Unlock Deck
k this deck


