Deck 14: Analysis of Variance

Question 1

In a two-factor ANOVA, there are 4 levels for factor A and 5 levels for factor B, and two observations within each cell. The number of treatments in this experiment is 40.

Answer 1

False

Question 2

In a two-factor ANOVA, where a is the number of factor A levels and b is the number of factor B levels, the number of degrees of freedom for the interaction term is

A)(a - 1)(b - 1)
B)ab - 1
C)(a - 1) + (b - 1)
D)Unknown; need to know the number of replicates.

Answer 2

(a - 1)(b - 1)

Question 3

A balanced experiment requires that the sample size for each treatment be equal.

Answer 3

True

Question 4

A complete 3*2 factorial experiment is called balanced if:

A)data is collected at all three levels of factor A.
B)data is collected at both levels of factor B.
C)the number of replicates is the same for each of the 6 treatments.
D)None of these choices.

Question 5

In the two-factor ANOVA where a is the number of factor A levels, b is the number of factor B levels, and r is the number of replicates, the number of degrees of freedom for interaction is:

A)(a-1)(b - 1)
B)abr - 1
C)(a - 1)(r - 1)
D)n - ab

Question 6

In a two-factor ANOVA, always test for ____________________ first.

Question 7

In a two-factor ANOVA, the sum of squares due to both factors, the interaction sum of squares, and the error sum of squares must all add up to the total sum of squares.

Question 8

In a two-factor ANOVA, there are 4 levels for factor A, 5 levels for factor B, and 3 observations for each combination of factor A and factor B levels. The number of treatments in this experiment equals:

A)16
B)20
C)25
D)60

Question 9

A complete factorial experiment is an experiment in which the number of replicates is the same for each treatment on which data is collected.

Question 10

In a two-factor ANOVA, there are 5 levels for factor A, 4 levels for factor B, and 3 observations for each combination of factor A and factor B levels. The number of treatments in this experiment equals 20.

Question 11

Each observation for particular combination of treatments is called a(n) ________________.

Question 12

When the effect of a level for one factor depends on which level of another factor is present, the most appropriate ANOVA design to use in this situation is the:

A)One-way ANOVA with 2 treatments.
B)Two-factor ANOVA with interaction.
C)Two-factor ANOVA with no interaction.
D)None of these choices.

Question 13

If there is enough evidence to conclude that there is interaction in a two-factor ANOVA, do not proceed to conduct the F-tests for each factor individually.

Question 14

In a two-factor ANOVA, there are 4 levels for factor A, 5 levels for factor B, and 3 observations for each combination of factor A and factor B levels. The total number of observations in this experiment equals:

A)60
B)25
C)20
D)16

Question 15

A(n) ____________________ experiment requires that the sample size for each treatment be equal.

Question 16

In a two-factor ANOVA, always test for interaction last.

Question 17

Each observation for a particular combination of treatments is called a replicate.

Question 18

The equation: SS(Total) = SS(A) + SS(B) + SS(AB) + SSE applies to which ANOVA model?

A)One-way ANOVA with 2 treatments.
B)Two-factor ANOVA with interaction.
C)Two-factor ANOVA with no interaction.
D)None of these choices.

Question 19

In a(n) ____________________ factorial experiment, data for all possible combinations of the levels of the factors are gathered.

Question 20

In the two-factor ANOVA where a is the number of factor A levels, b is the number of factor B levels, r is the number of replicates, and n is the total number of observations, the number of degrees of freedom for error is:

A)(a - 1)(b - 1)
B)abr - 1
C)r(a - 1)(b -1)
D)n - ab

Question 21

A randomized block design with 4 treatments and 5 blocks produced the following sum of squares values: SS(Total) = 2000, SST = 400, SSE = 200. The value of MSB must be 350.

Question 22

NARRBEGIN: MigraineTreatments
Migraine Treatments
The following data were generated from a 2 * 2 factorial experiment with 3 replicates, where factor A levels represent two different injection procedures of an anesthetic to the occipital nerve (located in the back of the neck), and factor B levels represent two different drugs, which physicians recommend to increase the effectiveness of the injections. Three migraine patients were randomly selected for each combination of injection and drug. $$\begin{array} { l } \begin{array} { | c | c c | } &\text { Factor B } \\\hline \text { Factor A } & 1 & 2 \\\hline 1 & 7 & 13 \\& 10 & 11 \\& 8 & 12 \\2 & 10 & 10 \\& 11 & 15 \\& 0 & 11 \\\hline\end{array}\end{array}$$ NARREND

-{Migraine Treatments Narrative} Test at the 5% significance level to determine if differences exist among the levels of factor B.

Question 23

A randomized block experiment having five treatments and six blocks produced the following values: SST = 252, SS(Total) = 1,545, SSE = 198. The value of SSB must be 1095.

Question 24

When the problem objective is to compare more than two populations, the experimental design that is the counterpart of the matched pairs experiment is called the randomized block design.

Question 25

In the randomized block design for ANOVA, where k is the number of treatments and b is the number of blocks, the number of degrees of freedom for error is:

A)k - b
B)kb - 1
C)n - k - b + 1
D)None of these choices.

Question 26

One example of a blocking variable is the dosage level that each subject is assigned to in a randomized experiment.

Question 27

NARRBEGIN: MigraineTreatments
Migraine Treatments
The following data were generated from a 2 * 2 factorial experiment with 3 replicates, where factor A levels represent two different injection procedures of an anesthetic to the occipital nerve (located in the back of the neck), and factor B levels represent two different drugs, which physicians recommend to increase the effectiveness of the injections. Three migraine patients were randomly selected for each combination of injection and drug. $$\begin{array} { l } \begin{array} { | c | c c | } &\text { Factor B } \\\hline \text { Factor A } & 1 & 2 \\\hline 1 & 7 & 13 \\& 10 & 11 \\& 8 & 12 \\2 & 10 & 10 \\& 11 & 15 \\& 0 & 11 \\\hline\end{array}\end{array}$$ NARREND

-{Migraine Treatments Narrative} Test at the 5% significance level to determine if differences exist among the four treatment means.

Question 28

NARRBEGIN: Keyboard Configuration an
Keyboard Configuration and Size
The data shown below were taken from a 2 * 3 factorial experiment to examine the effects of factor A (keyboard configuration, 3 levels) and factor B (keyboard size, 2 levels) on typing speed. Each cell consists of the times needed for each of 4 randomly assigned keyboardists to type a standard document under each set of conditions (in minutes). $$\begin{array}{l}                   \text { Factor } B\\\begin{array} { | c | c c | } \hline \text { Factor } A & 1 & 2 \\\hline 1 & 26 & 24 \\& 19 & 21 \\& 20 & 20 \\ & 21 & 23 \\2& 30 & 33 \\& 24 & 27 \\& 25 & 31 \\& 29 & 29 \\3& 26 & 31 \\& 22 & 23 \\& 27 & 24 \\& 17 & 26 \\\hline\end{array}\end{array}$$ NARREND

-{Keyboard Configuration and Size Narrative}
a.Create the ANOVA table.
b.Is there sufficient evidence at the 5% significance level to infer that factors A and B interact?

Question 29

The F-test of the randomized block design of the analysis of variance has the same requirements as the independent samples design; that is, the random variable must be normally distributed and the population variances must be equal.

Question 30

When the variation associated with blocks is removed from the data, SSE increases.

Question 31

NARRBEGIN: MigraineTreatments
Migraine Treatments
The following data were generated from a 2 * 2 factorial experiment with 3 replicates, where factor A levels represent two different injection procedures of an anesthetic to the occipital nerve (located in the back of the neck), and factor B levels represent two different drugs, which physicians recommend to increase the effectiveness of the injections. Three migraine patients were randomly selected for each combination of injection and drug. $$\begin{array} { l } \begin{array} { | c | c c | } &\text { Factor B } \\\hline \text { Factor A } & 1 & 2 \\\hline 1 & 7 & 13 \\& 10 & 11 \\& 8 & 12 \\2 & 10 & 10 \\& 11 & 15 \\& 0 & 11 \\\hline\end{array}\end{array}$$ NARREND

-{Migraine Treatments Narrative}
a.Create the ANOVA table.
b.Test at the 5% significance level to determine if factors A and B interact.

Question 32

SSE in the independent samples design is equal to the sum of SSB and SSE in the randomized block design.

Question 33

In a two-factor ANOVA, the total sum of squares is broken down into the sum of SS(A) + SS(B) + SSE + SS(____________________).

Question 34

NARRBEGIN: Keyboard Configuration an
Keyboard Configuration and Size
The data shown below were taken from a 2 * 3 factorial experiment to examine the effects of factor A (keyboard configuration, 3 levels) and factor B (keyboard size, 2 levels) on typing speed. Each cell consists of the times needed for each of 4 randomly assigned keyboardists to type a standard document under each set of conditions (in minutes). $$\begin{array}{l}                   \text { Factor } B\\\begin{array} { | c | c c | } \hline \text { Factor } A & 1 & 2 \\\hline 1 & 26 & 24 \\& 19 & 21 \\& 20 & 20 \\ & 21 & 23 \\2& 30 & 33 \\& 24 & 27 \\& 25 & 31 \\& 29 & 29 \\3& 26 & 31 \\& 22 & 23 \\& 27 & 24 \\& 17 & 26 \\\hline\end{array}\end{array}$$ NARREND

-{Keyboard Configuration and Size Narrative} Test at the 5% significance level to determine if time differences exist among the different keyboard configurations.

Question 35

In employing the randomized block design, the primary interest lies in reducing sum of squares for blocks (SSB).

Question 36

NARRBEGIN: MigraineTreatments
Migraine Treatments
The following data were generated from a 2 * 2 factorial experiment with 3 replicates, where factor A levels represent two different injection procedures of an anesthetic to the occipital nerve (located in the back of the neck), and factor B levels represent two different drugs, which physicians recommend to increase the effectiveness of the injections. Three migraine patients were randomly selected for each combination of injection and drug. $$\begin{array} { l } \begin{array} { | c | c c | } &\text { Factor B } \\\hline \text { Factor A } & 1 & 2 \\\hline 1 & 7 & 13 \\& 10 & 11 \\& 8 & 12 \\2 & 10 & 10 \\& 11 & 15 \\& 0 & 11 \\\hline\end{array}\end{array}$$ NARREND

-{Migraine Treatments Narrative} Test at the 5% significance level to determine if differences exist among the levels of factor A.

Question 37

The required conditions for a two-factor ANOVA are that the distribution of the response is ____________________ distributed; the variance for each treatment is ____________________; and the samples are ____________________.

Question 38

The purpose of designing a randomized block experiment is to reduce the between-treatments variation (SST) to more easily detect differences between the treatment means.

Question 39

To test for interaction between factors A and B in a two-factor ANOVA, you use the F statistic that equals ____________________ divided by ____________________.

Question 40

NARRBEGIN: Keyboard Configuration an
Keyboard Configuration and Size
The data shown below were taken from a 2 * 3 factorial experiment to examine the effects of factor A (keyboard configuration, 3 levels) and factor B (keyboard size, 2 levels) on typing speed. Each cell consists of the times needed for each of 4 randomly assigned keyboardists to type a standard document under each set of conditions (in minutes). $$\begin{array}{l}                   \text { Factor } B\\\begin{array} { | c | c c | } \hline \text { Factor } A & 1 & 2 \\\hline 1 & 26 & 24 \\& 19 & 21 \\& 20 & 20 \\ & 21 & 23 \\2& 30 & 33 \\& 24 & 27 \\& 25 & 31 \\& 29 & 29 \\3& 26 & 31 \\& 22 & 23 \\& 27 & 24 \\& 17 & 26 \\\hline\end{array}\end{array}$$ NARREND

-{Keyboard Configuration and Size Narrative} Test at the 5% significance level to determine if time differences exist among the keyboard sizes.

Question 41

Which of the following is false regarding SSB?

A)SSB stands for sum of squares for blocks.
B)SSB can help to reduce SSE.
C)SSB can help make it easier to determine whether differences exist between the treatment means.
D)All of these choices are true.

Question 42

The test of whether the block means differ uses an F-test whose test statistic is ____________________ divided by ____________________.

Question 43

NARRBEGIN: Acid Reflux
Acid Reflux
A partial ANOVA table in a randomized block design is shown below, where the treatments refer to different acid reflux medicines, and the blocks refer to groups of men with similar levels of stomach acid.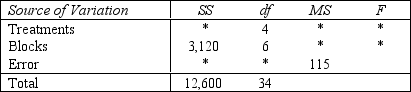 NARREND
{Acid Reflux Narrative} Fill in the missing values (identified by asterisks) in the above ANOVA Table.

Question 44

In the randomized block design ANOVA, the sum of squares for error equals:

A)SS(Total) - SST
B)SS(Total) - SSB
C)SS(Total) - SST - SSB
D)None of these choices.

Question 45

NARRBEGIN: Food Irradiation
Food Irradiation
In recent years the irradiation of food to reduce bacteria and preserve the food longer has become more common. A company that performs this service has developed four different methods of irradiating food. To determine which is best, it conducts an experiment where different foods are irradiated and the bacteria count is measured. As part of the experiment the following foods are irradiated: meat, poultry, veal, tuna, and yogurt. The results are shown below. $$\begin{array}{l}\text { Bactaria Count }\\\begin{array} { | l | c c c c | } \hline \text { Food } & \text { Method 1 } & \text { Method 2 } & \text { Method 3 } & \text { Method 4 } \\\hline \text { Meat } & 47 & 53 & 36 & 68 \\\text { Poulty } & 53 & 61 & 48 & 75 \\\text { Veal } & 68 & 85 & 55 & 45 \\\text { Tuna } & 25 & 24 & 20 & 27 \\\text { Yogurt } & 44 & 48 & 38 & 46 \\\hline\end{array}\end{array}$$ NARREND

-{Food Irradiation Narrative} Set up the ANOVA Table. Use $\alpha$ = 0.01 to determine the critical values.

Question 46

When the objective is to compare more than two populations, the experimental design that is the counterpart of the matched pairs experiment is called a:

A)completely randomized design.
B)one-way ANOVA design.
C)randomized block design.
D)None of these choices.

Question 47

In employing the randomized block design, the primary interest lies in reducing sum of squares for ____________________.

Question 48

When we perform a blocked experiment by using the same subject for each treatment, this is called a(n) ____________________ design.

Question 49

If our analysis includes all possible levels of a factor, the technique for analyzing the data is called a(n) ____________________-effects ANOVA.

Question 50

NARRBEGIN: Motorcycle Repair Cost
Motorcycle Repair Cost
Motorcycle insurance appraisers examine motorcycles that have been involved in accidental collisions and estimate the cost of repairs. An insurance executive claims that there are significant differences in the estimates from different appraisers. To support his claim he takes a random sample of six motorcycles that have recently been damaged in accidents. Three appraisers then estimate the repair costs of all six motorcycles. The data are shown below. $$\begin{array}{l}\text { Bstimatad Repair Cost }\\\begin{array} { | c | c c c | } \hline \text { Motorcycles } & \text { Appraiser 1 } & \text { Appraiser 2 } & \text { Appraiser 3 } \\\hline 1 & 650 & 600 & 750 \\2 & 930 & 910 & 1010 \\3 & 440 & 450 & 500 \\4 & 750 & 710 & 810 \\5 & 1190 & 1050 & 1250 \\6 & 1560 & 1270 & 1450 \\\hline\end{array}\end{array}$$ NARREND

-{Motorcycle Repair Cost Narrative} Set up the ANOVA Table. Use $\alpha$ = 0.05 to determine the critical values.

Question 51

The randomized block design with exactly two treatments is equivalent to a two-tail:

A)independent samples z-test.
B)independent samples equal-variances t-test.
C)independent samples unequal-variances t-test.
D)matched pairs t-test.

Question 52

SSE in the independent samples design is equal to the sum of SS____________________ and SS____________________ in the randomized block design.

Question 53

The F-test of a randomized block design of the analysis of variance has the same requirements as the independent samples design; that is, the random variable must be ____________________ distributed and the population ____________________ must be equal.

Question 54

NARRBEGIN: Food Irradiation
Food Irradiation
In recent years the irradiation of food to reduce bacteria and preserve the food longer has become more common. A company that performs this service has developed four different methods of irradiating food. To determine which is best, it conducts an experiment where different foods are irradiated and the bacteria count is measured. As part of the experiment the following foods are irradiated: meat, poultry, veal, tuna, and yogurt. The results are shown below. $$\begin{array}{l}\text { Bactaria Count }\\\begin{array} { | l | c c c c | } \hline \text { Food } & \text { Method 1 } & \text { Method 2 } & \text { Method 3 } & \text { Method 4 } \\\hline \text { Meat } & 47 & 53 & 36 & 68 \\\text { Poulty } & 53 & 61 & 48 & 75 \\\text { Veal } & 68 & 85 & 55 & 45 \\\text { Tuna } & 25 & 24 & 20 & 27 \\\text { Yogurt } & 44 & 48 & 38 & 46 \\\hline\end{array}\end{array}$$ NARREND

-{Food Irradiation Narrative} Can the company infer at the 1% significance level that differences in the bacteria count exist among the four irradiation methods?

Question 55

When the problem objective is to compare more than two populations, the experimental design that is the counterpart of the matched pairs experiment is called the randomized ____________________ design.

Question 56

A randomized block design experiment produced the following data. $$\begin{array}{l}\quad\quad\quad\quad\text { Treatment }\\\begin{array} { | c | c c c | } \hline \text { Block } & 1 & 2 & 3 \\\hline 1 & 25 & 27 & 25 \\2 & 19 & 18 & 17 \\3 & 15 & 20 & 16 \\4 & 23 & 27 & 20 \\5 & 30 & 31 & 28 \\\hline\end{array}\end{array}$$
a.Set up the ANOVA Table. Use $\alpha$ = 0.05 to determine the critical values.
b.Test to determine whether the treatment means differ. (Use $\alpha$ = 0.05.)
c.Test to determine whether the block means differ. (Use $\alpha$ = 0.05.)

Question 57

Which of the following statements is true?

A)A fixed-effects ANOVA refers to the analysis which includes all possible levels of a factor.
B)A random-effects ANOVA refers to the analysis where the levels included in the study represent a random sample of all levels that exist.
C)A multifactor experiment is one where there are two or more factors that define the treatments.
D)All of these choices are true.

Question 58

NARRBEGIN: Motorcycle Repair Cost
Motorcycle Repair Cost
Motorcycle insurance appraisers examine motorcycles that have been involved in accidental collisions and estimate the cost of repairs. An insurance executive claims that there are significant differences in the estimates from different appraisers. To support his claim he takes a random sample of six motorcycles that have recently been damaged in accidents. Three appraisers then estimate the repair costs of all six motorcycles. The data are shown below. $$\begin{array}{l}\text { Bstimatad Repair Cost }\\\begin{array} { | c | c c c | } \hline \text { Motorcycles } & \text { Appraiser 1 } & \text { Appraiser 2 } & \text { Appraiser 3 } \\\hline 1 & 650 & 600 & 750 \\2 & 930 & 910 & 1010 \\3 & 440 & 450 & 500 \\4 & 750 & 710 & 810 \\5 & 1190 & 1050 & 1250 \\6 & 1560 & 1270 & 1450 \\\hline\end{array}\end{array}$$ NARREND

-{Motorcycle Repair Cost Narrative} Can we infer at the 5% significance level that the executive's claim is true?

Question 59

A randomized block design with 4 treatments and 5 blocks produced the following sum of squares values: SS(Total) = 1,951, SST = 349, SSE = 188 . The value of SSB must be:

A)537
B)1,763
C)1,414
D)1,602

Question 60

The primary interest of designing a randomized block experiment is to:

A)reduce the within-treatments variation to more easily detect differences among the treatment means.
B)increase the between-treatments variation to more easily detect differences among the treatment means.
C)reduce the variation among blocks.
D)None of these choices.

Question 61

Fisher's least significant difference (LSD) multiple comparison method is flawed because

A)it will increase $\beta$ ; the probability of committing a Type II error
B)it will increase $\alpha$ ; the probability of committing a Type I error
C)it will increase both $\alpha$ and $\beta$ , the probabilities of committing Type I and Type II errors, respectively.
D)None of these choices.

Question 62

____________________ multiple comparison method is based on the Studentized range statistic.

Question 63

Tukey's multiple comparison method is ____________________ (more/less) powerful than Fisher's LSD method.

Question 64

The Bonferroni adjustment decreases the experimentwise Type I error rate, but it increases the probability of a Type II error.

Question 65

Which of the following statements about multiple comparison methods is false?

A)They are to be use once the F-test in ANOVA has been rejected.
B)They are used to determine which particular population means differ.
C)There are many different multiple comparison methods but all yield the same conclusions.
D)All of these choices are true.

Question 66

Tukey's multiple comparison method is based on the Studentized range statistic.

Question 67

The ____________________ adjustment used with the LSD method decreases the experimentwise Type I error rate.

Question 68

The Bonferroni adjustment to Fisher's Least Significant Difference (LSD) multiple comparison method is made by dividing the specified experimentwise Type I error rate by the number of pairs of population means.

Question 69

Multiple comparison methods are used after it is found that H₀ from ANOVA has been ____________________.

Question 70

Fisher's least significant difference method (LSD) substitutes the pooled variance estimator from the equal variances t-test with the MSE from ANOVA.

Question 71

When is the Tukey multiple comparison method used?

A)To test for normality.
B)To test for differences in pairwise means.
C)To test for equality of a group of population means.
D)To test equal variances.

Question 72

If you plan to compare all possible combinations of pairs of population means, the multiple comparison method to use is ____________________ method.

Question 73

NARRBEGIN: Acid Reflux
Acid Reflux
A partial ANOVA table in a randomized block design is shown below, where the treatments refer to different acid reflux medicines, and the blocks refer to groups of men with similar levels of stomach acid. $$\begin{array} { | l | c c c c | } \hline \text { Source of Variation } & \mathbf { S S } & \boldsymbol { df } & \boldsymbol { M S } & \boldsymbol { F } \\\hline \text { Treatments } & * & 4 & * & * \\\text { Blocks } & 3,120 & 6 & * & * \\\text { Error } &* &*&115 & \\\hline \text { Total } &12,600&34 & & \\\hline\end{array}$$ NARREND

-{Acid Reflux Narrative} Can we infer at the 5% significance level that the block means differ?

Question 74

Which of the following is not true of Tukey's Multiple Comparison Method?

A)It is based on the studentized range statistic to obtain the critical value needed to construct individual confidence intervals.
B)It theoretically requires that all sample sizes be equal, or at least similar.
C)It is more powerful than the LSD method.
D)All of these choices are true.

Question 75

The techniques used to detect which population means differ are called multiple ____________________ methods.

Question 76

Tukey's multiple comparison method is more powerful than Fisher's LSD Method at finding differences in pairwise population means.

Question 77

Multiple comparison methods are used to determine whether or not any differences occur amongst a group of population means.

Question 78

NARRBEGIN: Health Inspectors' Ages
Health Inspectors' Ages
In order to examine the differences in ages of health inspectors among five counties, a Health Department statistician took random samples of six inspectors' ages in each county. The data are listed below. An F-test using ANOVA showed that average age differs for at least two counties. $$\begin{array}{l}\text { Ages of hipectors among Five sehoal Districts }\\\begin{array} { | c c c c c | } \hline 1 & 2 & 3 & 4 & 5 \\\hline 41 & 39 & 36 & 45 & 53 \\53 & 48 & 28 & 37 & 55 \\28 & 41 & 29 & 46 & 49 \\45 & 51 & 33 & 48 & 56 \\40 & 49 & 27 & 51 & 48 \\59 & 50 & 26 & 49 & 61 \\\hline\end{array}\end{array}$$ NARREND

-{Health Inspectors' Ages Narrative} Use Tukey's multiple comparison method to determine which means differ.

Question 79

In Fisher's least significant difference (LSD) multiple comparison method, the LSD value will be the same for all pairs of means if:

A)all sample sizes are the same.
B)all sample means are the same.
C)all population means are the same.
D)None of these choices.

Question 80

NARRBEGIN: Acid Reflux
Acid Reflux
A partial ANOVA table in a randomized block design is shown below, where the treatments refer to different acid reflux medicines, and the blocks refer to groups of men with similar levels of stomach acid. $$\begin{array} { | l | c c c c | } \hline \text { Source of Variation } & \mathbf { S S } & \boldsymbol { df } & \boldsymbol { M S } & \boldsymbol { F } \\\hline \text { Treatments } & * & 4 & * & * \\\text { Blocks } & 3,120 & 6 & * & * \\\text { Error } &* &*&115 & \\\hline \text { Total } &12,600&34 & & \\\hline\end{array}$$ NARREND

-{Acid Reflux Narrative} Can we infer at the 5% significance level that the treatment means differ?