Deck 6: Linear Programming
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/161
Play
Full screen (f)
Deck 6: Linear Programming
1
Write down (without solving) the dual linear programming problem. Minimize subject to the following.
A)Maximize subject to the following.
B) Maximize subject to the following.
C) Maximize subject to the following.
D) Maximize subject to the following.
E) Maximize subject to the following.
A)Maximize subject to the following.
B) Maximize subject to the following.
C) Maximize subject to the following.
D) Maximize subject to the following.
E) Maximize subject to the following.
Maximize subject to the following.
2
Solve the standard minimization problem using duality.
Minimize
Subject to
A) , ,
B) , ,
C) , ,
D) , ,
E) , ,
Minimize
Subject to
A) , ,
B) , ,
C) , ,
D) , ,
E) , ,
, ,
3
Solve the standard minimization problem using duality.
If you have several solutions, select one of them.
A) , , ,
B) , , ,
C) , , ,
D) , , ,
E) , , ,
If you have several solutions, select one of them.
A) , , ,
B) , , ,
C) , , ,
D) , , ,
E) , , ,
, , ,
4
Solve the standard minimization problem using duality.
Minimize
Subject to
A) , ,
B) , ,
C) , ,
D) , ,
E) , ,
Minimize
Subject to
A) , ,
B) , ,
C) , ,
D) , ,
E) , ,

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
5
Solve the games with the given payoff matrix.
A) , ,
B) , ,
C) , ,
D) , ,
E) , ,
A) , ,
B) , ,
C) , ,
D) , ,
E) , ,

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
6
Solve the standard minimization problem using duality.
Minimize
Subject to
A) , , ,
B) , , ,
C) , , ,
D) , , ,
E) , , ,
Minimize
Subject to
A) , , ,
B) , , ,
C) , , ,
D) , , ,
E) , , ,

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
7
Solve the games with the given payoff matrix.
A) , ,
B) , ,
C) , ,
D) , ,
E) , ,
A) , ,
B) , ,
C) , ,
D) , ,
E) , ,

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
8
Minimize subject to the following constraints.
A) , ,
B) , ,
C) , ,
D) , ,
E) , ,
A) , ,
B) , ,
C) , ,
D) , ,
E) , ,

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
9
Oz makes lion food out of giraffe and gazelle meat. Giraffe meat has 18 grams of protein and 36 grams of fat per pound, while gazelle meat has 36 grams of protein and 18 grams of fat per pound. A batch of lion food must contain at least 36,000 grams of protein and 54,000 grams of fat. Giraffe meat costs $4/pound and gazelle meat costs $8/pound. How many pounds of each should go into each batch of lion food in order to minimize costs What are the shadow costs of protein and fat Use the rounded numbers of pounds in your calculations. ?
A)1,333 pounds of giraffe meat and 333 pounds of gazelle, 22 cents per gram of protein and 15 cents per gram of fat
B) 333 pounds of giraffe meat and 333 pounds of gazelle, 15 cents per gram of protein and 15 cents per gram of fat
C) 1,333 pounds of giraffe meat and 333 pounds of gazelle, 15 cents per gram of protein and 22 cents per gram of fat
D) 1,333 pounds of giraffe meat and 333 pounds of gazelle, 22 cents per gram of protein and 22 cents per gram of fat
E) 333 pounds of giraffe meat and 1,333 pounds of gazelle, 22 cents per gram of protein and 15 cents per gram of fat
A)1,333 pounds of giraffe meat and 333 pounds of gazelle, 22 cents per gram of protein and 15 cents per gram of fat
B) 333 pounds of giraffe meat and 333 pounds of gazelle, 15 cents per gram of protein and 15 cents per gram of fat
C) 1,333 pounds of giraffe meat and 333 pounds of gazelle, 15 cents per gram of protein and 22 cents per gram of fat
D) 1,333 pounds of giraffe meat and 333 pounds of gazelle, 22 cents per gram of protein and 22 cents per gram of fat
E) 333 pounds of giraffe meat and 1,333 pounds of gazelle, 22 cents per gram of protein and 15 cents per gram of fat

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
10
Solve the standard minimization problem using duality.
A) , , ,
B) , , ,
C) , , ,
D) , , ,
E) , , ,
A) , , ,
B) , , ,
C) , , ,
D) , , ,
E) , , ,

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
11
Write down (without solving) the dual LP problem.
Minimize subject to
A)maximize subject to
B) maximize subject to
C) maximize subject to
D) maximize subject to
E) maximize subject to
Minimize subject to
A)maximize subject to
B) maximize subject to
C) maximize subject to
D) maximize subject to
E) maximize subject to

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
12
Solve the standard minimization problem using duality.
Minimize
Subject to
A) , ,
B) , ,
C) , ,
D) , ,
E) , ,
Minimize
Subject to
A) , ,
B) , ,
C) , ,
D) , ,
E) , ,

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
13
The Enormous State University's Business School is buying computers. The school has two models to choose from. Each first model comes with 400 MB of memory and 80 GB of disk space, while each second model has 300 MB of memory and 100 GB of disk space. For reasons related to its accreditation, the school would like to be able to say that it has a total of at least 48,000 MB of memory and at least 12,800 GB of disk space. If both models cost $4,400 each, how many of each should the school buy to keep the cost as low as possible What are the shadow costs of memory and disk space
A)80 first models and 60 second models, $ per MB and $ per GB
B) 60 first models and 80 second models, $ per MB and $ per GB
C) 60 first models and 80 second models, $ per MB and $ per GB
D) 60 first models and 80 second models, $ per MB and $ per GB
E) 80 first models and 60 second models, $ per MB and $ per GB
A)80 first models and 60 second models, $ per MB and $ per GB
B) 60 first models and 80 second models, $ per MB and $ per GB
C) 60 first models and 80 second models, $ per MB and $ per GB
D) 60 first models and 80 second models, $ per MB and $ per GB
E) 80 first models and 60 second models, $ per MB and $ per GB

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
14
We are to minimize subject to the following constraints.
After using the simplex method for dual problem to arrive at the tableau below, what is the maximum value for
X y s t p
A)9
B) 3
C) 5.5
D)
E)
After using the simplex method for dual problem to arrive at the tableau below, what is the maximum value for
X y s t p
A)9
B) 3
C) 5.5
D)
E)

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
15
Solve the games with the given payoff matrix.
A) , ,
B) , ,
C) , ,
D) , ,
E) , ,
A) , ,
B) , ,
C) , ,
D) , ,
E) , ,

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
16
Ruff makes dog food out of chicken and grain. Chicken has 10 grams of protein and 5 grams of fat/ounce, and grain has 2 grams of protein and 2 grams of fat per ounce. A bag of dog food must contain at least 200 grams of protein and at least 50 grams of fat. If chicken costs 15 cents per ounce and grain costs 2 cents per ounce, how many ounces of each should Ruff use in each bag of dog food in order to minimize cost What are the shadow costs of protein and fat ?
A)100 oz of chicken and no grain, 1 cents per gram of protein and 1 cents per gram of fat
B) 200 oz of chicken and no grain, 0 cents per gram of protein and 1 cents per gram of fat
C) 100 oz of chicken and no grain, 0.5 cents per gram of protein and 0.5 cents per gram of fat
D) 200 oz of grain and no chicken, 0.5 cents per gram of protein and 0.5 cents per gram of fat
E) 100 oz of grain and no chicken, 1 cents per gram of protein and 0 cents per gram of fat
A)100 oz of chicken and no grain, 1 cents per gram of protein and 1 cents per gram of fat
B) 200 oz of chicken and no grain, 0 cents per gram of protein and 1 cents per gram of fat
C) 100 oz of chicken and no grain, 0.5 cents per gram of protein and 0.5 cents per gram of fat
D) 200 oz of grain and no chicken, 0.5 cents per gram of protein and 0.5 cents per gram of fat
E) 100 oz of grain and no chicken, 1 cents per gram of protein and 0 cents per gram of fat

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
17
Solve the standard minimization problem using duality.
Minimize
Subject to
A)
B)
C)
D)
E)
Minimize
Subject to
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
18
Solve the games with the given payoff matrix.
A) , ,
B) , ,
C) , ,
D) , ,
E) , ,
A) , ,
B) , ,
C) , ,
D) , ,
E) , ,

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
19
We are to minimize subject to the following constraints.
What is the dual problem in matrix form
A)
B)
C)
D)
E)
What is the dual problem in matrix form
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
20
Write down (without solving) the dual LP problem.
Maximize subject to
A)minimize subject to
B) minimize subject to
C) minimize subject to
D) minimize subject to
E) minimize subject to
Maximize subject to
A)minimize subject to
B) minimize subject to
C) minimize subject to
D) minimize subject to
E) minimize subject to

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
21
Federal Rent-a-Car is putting together a new fleet. It is considering package offers from three car manufacturers. Fred Motors is offering 5 small cars, 5 medium cars, and 10 large cars for $500,000. Admiral Motors is offering 5 small, 10 medium, and 5 large cars for $400,000. Chrysalis is offering 10 small, 5 medium, and 5 large cars for $300,000. Federal would like to buy at least 600 small cars, at least 500 medium cars, and at least 600 large cars. How many packages should it buy from each car maker to keep the total cost as small as possible What will be the total cost ?
A)15 packages of Fred Motors, 35 packages of Admiral Motors, and 15 packages of Chrysalis, the total cost is $26,000,000
B) 35 packages of Fred Motors, 15 packages of Admiral Motors, and 35 packages of Chrysalis, the total cost is $34,000,000
C) 35 packages of Fred Motors, 35 packages of Admiral Motors, and 15 packages of Chrysalis, the total cost is $36,000,000
D) 15 packages of Fred Motors, 35 packages of Admiral Motors, and 35 packages of Chrysalis, the total cost is $32,000,000
E) 15 packages of Fred Motors, 15 packages of Admiral Motors, and 35 packages of Chrysalis, the total cost is $24,000,000
A)15 packages of Fred Motors, 35 packages of Admiral Motors, and 15 packages of Chrysalis, the total cost is $26,000,000
B) 35 packages of Fred Motors, 15 packages of Admiral Motors, and 35 packages of Chrysalis, the total cost is $34,000,000
C) 35 packages of Fred Motors, 35 packages of Admiral Motors, and 15 packages of Chrysalis, the total cost is $36,000,000
D) 15 packages of Fred Motors, 35 packages of Admiral Motors, and 35 packages of Chrysalis, the total cost is $32,000,000
E) 15 packages of Fred Motors, 15 packages of Admiral Motors, and 35 packages of Chrysalis, the total cost is $24,000,000

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
22
Write down (without solving) the dual LP problem.
Maximize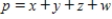 subject to
subject to
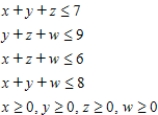
Use the letters s, t, u, and v in the indicated order.
Minimize __________ subject to the constraints.
__________ subject to the constraints.
Please enter the inequalities, separated by commas.
Maximize
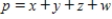 subject to
subject to
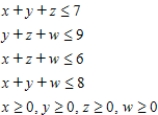
Use the letters s, t, u, and v in the indicated order.
Minimize
 __________ subject to the constraints.
__________ subject to the constraints.
Please enter the inequalities, separated by commas.

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
23
The Enormous State University's Business School is buying computers. The school has two models to choose from. Each first model comes with 400 MB of memory and 80 GB of disk space, while each second model has 300 MB of memory and 100 GB of disk space. For reasons related to its accreditation, the school would like to be able to say that it has a total of at least 48,000 MB of memory and at least 12,800 GB of disk space. If both models cost $800 each, how many of each should the school buy to keep the cost as low as possible
__________ first model(s) and __________ second model(s).
What are the shadow costs of memory and disk space
Please, enter first the shadow cost of MB and then the shadow cost of GB as improper fractions of integers without units. Separate your answers by a comma.
__________ first model(s) and __________ second model(s).
What are the shadow costs of memory and disk space
Please, enter first the shadow cost of MB and then the shadow cost of GB as improper fractions of integers without units. Separate your answers by a comma.

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
24
Solve the standard minimization problem using duality.
Minimize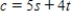
subject to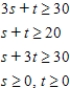
 __________
__________
 __________
__________
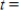 ___________
___________
Minimize
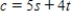
subject to
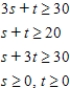
 __________
__________
 __________
__________
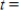 ___________
___________
Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
25
Solve the standard minimization problem using duality.
Minimize
subject to
After using the simplex method for dual problem to arrive at the tableau below, what is the maximum value for c
Minimize
subject to
After using the simplex method for dual problem to arrive at the tableau below, what is the maximum value for c

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
26
Ruff makes dog food out of chicken and grain. Chicken has 10 grams of protein and 5 grams of fat per ounce, and grain has 2 grams of protein and 2 grams of fat per ounce. A bag of dog food must contain at least 200 grams of protein and at least 100 grams of fat. If chicken costs 25 cents/ounce and grain costs 1 cents/ounce, how many ounces of each should Ruff use in each bag of dog food in order to minimize cost
__________ oz of chicken and __________ oz of grain.
What are the shadow costs of protein and fat
__________ cent(s) per gram of protein and __________ cent(s) per gram of fat.
__________ oz of chicken and __________ oz of grain.
What are the shadow costs of protein and fat
__________ cent(s) per gram of protein and __________ cent(s) per gram of fat.

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
27
Solve the standard minimization problem using duality.
Minimize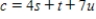
subject to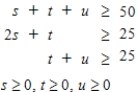
 _________
_________
 _________
_________
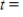 __________
__________
 __________
__________
Minimize
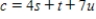
subject to
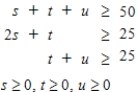
 _________
_________
 _________
_________
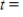 __________
__________
 __________
__________
Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
28
Oz makes lion food out of giraffe and gazelle meat. Giraffe meat has 18 grams of protein and 36 grams of fat per pound, while gazelle meat has 36 grams of protein and 18 grams of fat per pound. A batch of lion food must contain at least 36,000 grams of protein and 54,000 grams of fat. Giraffe meat costs $2/pound and gazelle meat costs $4/pound. How many pounds of each should go into each batch of lion food in order to minimize costs Please round the answers to the nearest pound.
__________ pound(s) of giraffe meat and __________ pound(s) of gazelle.
What are the shadow costs of protein and fat Please round the answers to the nearest cent.
__________ cents per gram of protein and __________ cents per gram of fat.
__________ pound(s) of giraffe meat and __________ pound(s) of gazelle.
What are the shadow costs of protein and fat Please round the answers to the nearest cent.
__________ cents per gram of protein and __________ cents per gram of fat.

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
29
Solve the standard minimization problem using duality.
Minimize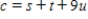
subject to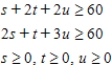
 __________
__________
 __________
__________
 __________
__________
 __________
__________
Minimize
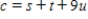
subject to
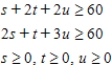
 __________
__________
 __________
__________
 __________
__________
 __________
__________
Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
30
Each serving of Gerber Mixed Cereal for Baby contains 60 calories and no Vitamin C. Each serving of Gerber Mango Tropical Fruit Dessert contains 80 calories and 45 percent of the U.S. Recommended Daily Allowance (RDA) of Vitamin C for infants. Each serving of Gerber Apple Banana Juice contains 60 calories and 120 percent of the RDA of Vitamin C for infants. The cereal costs 10 cents per serving, the dessert costs 53 cents per serving, and the juice costs 27 cents per serving. If you want to provide your child with at least 120 calories and at least 120 percent of the RDA of Vitamin C, how can you do so at the least cost ?
A)1 serving of cereal, 1 serving of juice, and no dessert
B) 1 serving of dessert, 1 serving of juice, and no cereal
C) 1 serving of cereal, 3 servings of dessert, and no juice
D) 3 servings of dessert, 1 serving of juice, and no cereal
E) 1 serving of cereal, 1 serving of dessert, and no juice
A)1 serving of cereal, 1 serving of juice, and no dessert
B) 1 serving of dessert, 1 serving of juice, and no cereal
C) 1 serving of cereal, 3 servings of dessert, and no juice
D) 3 servings of dessert, 1 serving of juice, and no cereal
E) 1 serving of cereal, 1 serving of dessert, and no juice

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
31
Write down (without solving) the dual LP problem.
Minimize subject to
subject to
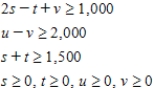
Use the letters x, y, z, and w in the indicated order. Please, use the numbers in the expression for p in order they come in the given LP problem.
Maximize __________ subject to the constraints.
__________ subject to the constraints.
Please enter the inequalities, separated with commas.
Minimize
 subject to
subject to
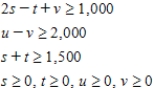
Use the letters x, y, z, and w in the indicated order. Please, use the numbers in the expression for p in order they come in the given LP problem.
Maximize
 __________ subject to the constraints.
__________ subject to the constraints.
Please enter the inequalities, separated with commas.

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
32
Solve the standard minimization problem using duality.
Minimize
subject to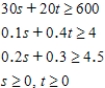
 __________
__________
 __________
__________
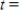 __________
__________
Minimize

subject to
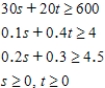
 __________
__________
 __________
__________
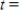 __________
__________
Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
33
Solve the standard minimization problem using duality.
Minimize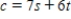
subject to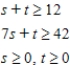
 __________
__________
 __________
__________
 __________
__________
Minimize
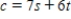
subject to
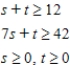
 __________
__________
 __________
__________
 __________
__________
Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
34
Solve the standard minimization problem using duality.
Minimize
subject to

Minimize

subject to



Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
35
Solve the standard minimization problem using duality. Minimize 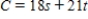 subject to the following.
subject to the following.
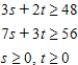
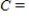 __________
__________
 __________
__________
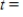 __________
__________
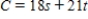 subject to the following.
subject to the following.
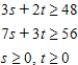
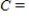 __________
__________
 __________
__________
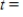 __________
__________
Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
36
The Miami Beach City Council has offered to subsidize hotel development in Miami Beach, and it is hoping for at least two hotels with a total capacity of at least 2,600. Suppose that you are a developer interested in taking advantage of this offer by building a small group of hotels in Miami Beach. You are thinking of three prototypes: a convention-style hotel with 500 rooms costing $100 million, a vacation-style hotel with 200 rooms costing $20 million, and a small motel with 50 rooms costing $4 million. The City Council will approve your plans, provided you build at least one convention-style hotel and no more than two small motels. How many of each type of hotel should you build to satisfy the city council's wishes and stipulations while minimizing your total cost ?
A)1 convention-style hotel, 13 vacation-style hotels, and 2 small motels
B) 1 convention-style hotel, 1 vacation-style hotel, and 2 small motels
C) 2 convention-style hotels, 10 vacation-style hotels, and 1 small motel
D) 2 convention-style hotels, 1 vacation-style hotel, and 1 small motel
E) 1 convention-style hotel, 10 vacation-style hotels, and 2 small motels
A)1 convention-style hotel, 13 vacation-style hotels, and 2 small motels
B) 1 convention-style hotel, 1 vacation-style hotel, and 2 small motels
C) 2 convention-style hotels, 10 vacation-style hotels, and 1 small motel
D) 2 convention-style hotels, 1 vacation-style hotel, and 1 small motel
E) 1 convention-style hotel, 10 vacation-style hotels, and 2 small motels

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
37
Write down ( without solving ) the dual LP problem.
Minimize subject to
What is the dual problem in matrix form
Minimize subject to
What is the dual problem in matrix form

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
38
Solve the standard minimization problem using duality.
Minimize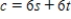 subject to
subject to 
 __________
__________
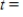 __________
__________
 __________
__________
Minimize
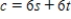 subject to
subject to 
 __________
__________
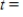 __________
__________
 __________
__________
Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
39
We return to your exploits coordinating distribution for the Tubular Ride Boogie Board Company. You will recall that the company has manufacturing plants in Tucson, Arizona and Toronto, Ontario, and you have been given the job of coordinating distribution of their latest model, the Gladiator, to their outlets in Honolulu and Venice Beach. The Tucson plant can manufacture up to 620 boards per week, while the Toronto plant, beset by labor disputes, can produce no more than 410 Gladiator boards per week. The outlet in Honolulu orders 480 Gladiator boards per week, while Venice Beach orders 510 boards per week. Transportation costs are as follows: Tucson to Honolulu: $10 per board; Tucson to Venice Beach: $5 per board; Toronto to Honolulu: $20 per board; Toronto to Venice Beach: $10 per board. Your manager has said that you are to be sure to fill all orders and ship the boogie boards at a minimum total transportation cost. But you have just been notified that workers at the Toronto boogie board plant have gone on strike, resulting in a total work stoppage. You are to come up with a revised delivery schedule by tomorrow with the understanding that the Tucson plant can push production to a maximum of 880 boards per week. Can you devise a delivery schedule with these accommodations in mind ?
A)no
B) yes
A)no
B) yes

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
40
Solve the standard minimization problem using duality.
Minimize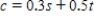
subject to
 __________
__________
 __________
__________
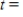 __________
__________
Minimize
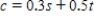
subject to

 __________
__________
 __________
__________
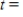 __________
__________
Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
41
Minimize 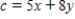 subject to the following (using the simplex method).
subject to the following (using the simplex method).
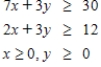
 __________
__________
 __________
__________
 __________
__________
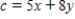 subject to the following (using the simplex method).
subject to the following (using the simplex method).
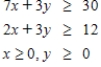
 __________
__________
 __________
__________
 __________
__________
Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
42
Minimize
Subject to
A) , , , ,
B) , , , ,
C) , , , ,
D) , , , ,
E) , , , ,
Subject to
A) , , , ,
B) , , , ,
C) , , , ,
D) , , , ,
E) , , , ,

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
43
Minimize
Subject to
A) , ,
B) , ,
C) , ,
D) , ,
E) no solution
Subject to
A) , ,
B) , ,
C) , ,
D) , ,
E) no solution

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
44
Minimize
Subject to
A) , , ,
B) , , ,
C) , , ,
D) , , ,
E) no solution
Subject to
A) , , ,
B) , , ,
C) , , ,
D) , , ,
E) no solution

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
45
Maximize

subject to

If there is no solution, do not fill the fields.
 __________
__________
 __________
__________
 __________
__________
 __________
__________
 __________
__________

subject to

If there is no solution, do not fill the fields.
 __________
__________
 __________
__________
 __________
__________
 __________
__________
 __________
__________
Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
46
Maximize
Subject to
A) , , , ,
B) , , , ,
C) , , , ,
D) , , , ,
E) no solution
Subject to
A) , , , ,
B) , , , ,
C) , , , ,
D) , , , ,
E) no solution

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
47
Your small farm encompasses 100 acres, and you are planning to grow tomatoes, lettuce, and carrots in the coming planting season. Fertilizer costs per acre are: $5 for tomatoes, $4 for lettuce, and $2 for carrots. Based on past experience, you estimate that each acre of tomatoes will require an average of 4 hours of labor per week, while tending to lettuce and carrots will each require an average of 2 hours per week. You estimate a profit of $2,000 for each acre of tomatoes, $1,500 for each acre of lettuce and $500 for each acre of carrots. You would like to spend at least $300 on fertilizer (your niece owns the company that manufactures it) and your farm laborers can supply up to 500 hours per week. How many acres of each crop should you plant to maximize total profits In this event, will you be using all 100 acres of your farm ?
A)plant 100 acres of tomatoes and no other crops, you will be using all 100 acres of your farm
B) plant 60 acres of tomatoes and no other crops, you will not be using all 100 acres of your farm
C) plant 100 acres of lettuce and no other crops, you will be using all 100 acres of your farm
D) plant 40 acres of carrots and no other crops, you will be using all 100 acres of your farm
E) plant 20 acres of lettuce and no other crops, you will be using all 100 acres of your farm
A)plant 100 acres of tomatoes and no other crops, you will be using all 100 acres of your farm
B) plant 60 acres of tomatoes and no other crops, you will not be using all 100 acres of your farm
C) plant 100 acres of lettuce and no other crops, you will be using all 100 acres of your farm
D) plant 40 acres of carrots and no other crops, you will be using all 100 acres of your farm
E) plant 20 acres of lettuce and no other crops, you will be using all 100 acres of your farm

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
48
Minimize
Subject to
Round all answers to two decimal places.
A) , , ,
B) , , ,
C) , , ,
D) , , ,
E) , , ,
Subject to
Round all answers to two decimal places.
A) , , ,
B) , , ,
C) , , ,
D) , , ,
E) , , ,

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
49
Maximize
Subject to
A) , ,
B) , ,
C) , ,
D) , ,
E) no solution
Subject to
A) , ,
B) , ,
C) , ,
D) , ,
E) no solution

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
50
Minimize
Subject to
A) , , ,
B) , , ,
C) , , ,
D) , , ,
E) no solution
Subject to
A) , , ,
B) , , ,
C) , , ,
D) , , ,
E) no solution

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
51
Maximize
Subject to
A) , ,
B) , ,
C)
D) , ,
E) no solution
Subject to
A) , ,
B) , ,
C)
D) , ,
E) no solution

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
52
Minimize
Subject to
A) , , , ,
B) , , , ,
C) , , , ,
D) , , , ,
E) no solution
Subject to
A) , , , ,
B) , , , ,
C) , , , ,
D) , , , ,
E) no solution

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
53
Fancy Pineapple produces pineapple juice and canned pineapple rings. This year the company anticipates a demand of at least 10,000 pints of pineapple juice and 500 cans of pineapple rings. Each pint of pineapple juice requires 2 pineapples, and each can of pineapple rings requires 1 pineapple. The company anticipates using at least 5,000 pineapples for these products. Each pint of pineapple juice costs the company 20 cents to produce, and each can of pineapple rings costs 50 cents to produce. How many pints of pineapple juice and cans of pineapple rings should Fancy Pineapple produce to meet the demand and minimize total costs ?
A)25,000 pints of pineapple juice and 500 cans of pineapple rings
B) 500 pints of pineapple juice and 10,000 cans of pineapple rings
C) 1,000 pints of pineapple juice and 25,000 cans of pineapple rings
D) 10,000 pints of pineapple juice and 500 cans of pineapple rings
E) 25,000 pints of pineapple juice and 1,000 cans of pineapple rings
A)25,000 pints of pineapple juice and 500 cans of pineapple rings
B) 500 pints of pineapple juice and 10,000 cans of pineapple rings
C) 1,000 pints of pineapple juice and 25,000 cans of pineapple rings
D) 10,000 pints of pineapple juice and 500 cans of pineapple rings
E) 25,000 pints of pineapple juice and 1,000 cans of pineapple rings

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
54
Maximize subject to the following (using the simplex method).
A) , ,
B) , ,
C) , ,
D) , ,
E) no solution
A) , ,
B) , ,
C) , ,
D) , ,
E) no solution

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
55
Maximize  subject to the following (using the simplex method).
subject to the following (using the simplex method).
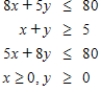
 __________
__________
 __________
__________
 __________
__________
 subject to the following (using the simplex method).
subject to the following (using the simplex method).
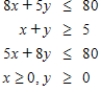
 __________
__________
 __________
__________
 __________
__________
Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
56
The political pollster Canter is preparing for a national election. It would like to poll at least 2,400 Democrats and 2,400 Republicans. Each mailing to the East Coast gets responses from 100 Democrats and 50 Republicans. Each mailing to the Midwest gets responses from 100 Democrats and 100 Republicans. And each mailing to the West Coast gets responses from 50 Democrats and 100 Republicans. Mailings to the East Coast cost $40 each to produce and mail, mailings to the Midwest cost $60 each, and mailings to the West Coast cost $50 each. How many mailings should Canter send to each area of the country to get the responses it needs at the least possible cost What will it cost ?
A)16 mailings to the East Coast, none to the Midwest, 16 to the West Coast, the cost is $1,440
B) No mailings to the East Coast, 24 to the Midwest, none to the West Coast, the cost is $1,440
C) 24 mailings to the East Coast, none to the Midwest, 24 to the West Coast, another solution resulting in the same cost is no mailings to the East Coast, 16 to the Midwest, none to the West Coast, the cost is $1,440
D) 16 mailings to the East Coast, none to the Midwest, 16 to the West Coast, another solution resulting in the same cost is no mailings to the East Coast, 24 to the Midwest, none to the West Coast, the cost is $1,440
E) No mailings to the East Coast, 16 to the Midwest, none to the West Coast, the cost is $1,440
A)16 mailings to the East Coast, none to the Midwest, 16 to the West Coast, the cost is $1,440
B) No mailings to the East Coast, 24 to the Midwest, none to the West Coast, the cost is $1,440
C) 24 mailings to the East Coast, none to the Midwest, 24 to the West Coast, another solution resulting in the same cost is no mailings to the East Coast, 16 to the Midwest, none to the West Coast, the cost is $1,440
D) 16 mailings to the East Coast, none to the Midwest, 16 to the West Coast, another solution resulting in the same cost is no mailings to the East Coast, 24 to the Midwest, none to the West Coast, the cost is $1,440
E) No mailings to the East Coast, 16 to the Midwest, none to the West Coast, the cost is $1,440

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
57
Minimize
Subject to
A) , , ,
B) , , ,
C) , , ,
D) , , ,
E) no solution
Subject to
A) , , ,
B) , , ,
C) , , ,
D) , , ,
E) no solution

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
58
Maximize
Subject to
Round all answers to two decimal places.
A) , , , ,
B) , , , ,
C) , , , ,
D) , , , ,
E) , , , ,
Subject to
Round all answers to two decimal places.
A) , , , ,
B) , , , ,
C) , , , ,
D) , , , ,
E) , , , ,

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
59
Minimize
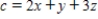
subject to
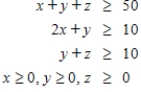
If there is no solution, do not fill the fields.
 __________
__________
 __________
__________
 __________
__________
 __________
__________
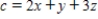
subject to
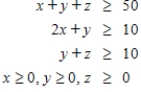
If there is no solution, do not fill the fields.
 __________
__________
 __________
__________
 __________
__________
 __________
__________
Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
60
Maximize
Subject to
A) , , ,
B) , , ,
C) , , ,
D) , , ,
E) no solution
Subject to
A) , , ,
B) , , ,
C) , , ,
D) , , ,
E) no solution

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
61
The political pollster Canter is preparing for a national election. It would like to poll at least 900 Democrats and 900 Republicans. Each mailing to the East Coast gets responses from 100 Democrats and 50 Republicans. Each mailing to the Midwest gets responses from 100 Democrats and 100 Republicans. And each mailing to the West Coast gets responses from 50 Democrats and 100 Republicans. Mailings to the East Coast cost $40 each to produce and mail, mailings to the Midwest cost $60 each, and mailings to the West Coast cost $50 each. How many mailings should Canter send to each area of the country to get the responses it needs at the least possible cost
__________ mailings to the East Coast, none to the Midwest, and __________ to the West Coast.
Another solution resulting in the same cost is no mailings to the East Coast, __________ to the Midwest, and none to the West Coast.
What will it cost
$__________
__________ mailings to the East Coast, none to the Midwest, and __________ to the West Coast.
Another solution resulting in the same cost is no mailings to the East Coast, __________ to the Midwest, and none to the West Coast.
What will it cost
$__________

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
62
You have $600,000 and are considering investing in three municipal bond funds, Pimco, Fidelity Spartan, and Columbia Oregon. You have the following data: Your broker has made the following suggestions:
At least 50 percent of your total investment should be in CMBFX.
No more than 10 percent of your total investment should be in PNF.
How much should you invest in each fund to maximize your anticipated returns while following your broker's advice
A)$60,000 in PNF, $240,000 in FDMMX, and $300,000 in CMBFX
B) $240,000 in PNF, $300,000 in FDMMX, and $60,000 in CMBFX
C) $300,000 in PNF, $240,000 in FDMMX, and $60,000 in CMBFX
D) $300,000 in PNF, $60,000 in FDMMX, and $240,000 in CMBFX
E) $60,000 in PNF, $300,000 in FDMMX, and $240,000 in CMBFX
At least 50 percent of your total investment should be in CMBFX.
No more than 10 percent of your total investment should be in PNF.
How much should you invest in each fund to maximize your anticipated returns while following your broker's advice
A)$60,000 in PNF, $240,000 in FDMMX, and $300,000 in CMBFX
B) $240,000 in PNF, $300,000 in FDMMX, and $60,000 in CMBFX
C) $300,000 in PNF, $240,000 in FDMMX, and $60,000 in CMBFX
D) $300,000 in PNF, $60,000 in FDMMX, and $240,000 in CMBFX
E) $60,000 in PNF, $300,000 in FDMMX, and $240,000 in CMBFX

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
63
The Miami Beach City Council has offered to subsidize hotel development in Miami Beach, and it is hoping for at least two hotels with a total capacity of at least 1,800. Suppose that you are a developer interested in taking advantage of this offer by building a small group of hotels in Miami Beach. You are thinking of three prototypes: a convention-style hotel with 500 rooms costing $100 million, a vacation-style hotel with 200 rooms costing $20 million, and a small motel with 50 rooms costing $4 million. The City Council will approve your plans, provided you build at least one convention-style hotel and no more than two small motels. How many of each type of hotel should you build to satisfy the city council's wishes and stipulations while minimizing your total cost
__________ convention-style hotel(s), __________ vacation-style hotel(s), and __________ small motel(s).
Now assume that the city council will give developers 20 percent of the cost of building new hotels in Miami Beach, up to $70 million. Will the city's $70 million subsidy be sufficient to cover 20 percent of your total costs
__________ convention-style hotel(s), __________ vacation-style hotel(s), and __________ small motel(s).
Now assume that the city council will give developers 20 percent of the cost of building new hotels in Miami Beach, up to $70 million. Will the city's $70 million subsidy be sufficient to cover 20 percent of your total costs

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
64
Each serving of Gerber Mixed Cereal for Baby contains 60 calories and no Vitamin C. Each serving of Gerber Mango Tropical Fruit Dessert contains 80 calories and 45 percent of the U.S. Recommended Daily Allowance (RDA) of Vitamin C for infants. Each serving of Gerber Apple Banana Juice contains 60 calories and 120 percent of the RDA of Vitamin C for infants. The cereal costs 10 cents per serving, the dessert costs 53 cents per serving, and the juice costs 27 cents per serving. If you want to provide your child with at least 360 calories and at least 240 percent of the RDA of Vitamin C, how can you do so at the least cost
?
__________ serving(s) of cereal, __________ serving(s) of dessert, and __________ serving(s) of juice.
?
__________ serving(s) of cereal, __________ serving(s) of dessert, and __________ serving(s) of juice.

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
65
Minimize
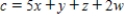
subject to

If there is no solution, do not fill the fields.
 __________
__________
 __________
__________
 __________
__________
 __________
__________
 __________
__________
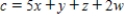
subject to

If there is no solution, do not fill the fields.
 __________
__________
 __________
__________
 __________
__________
 __________
__________
 __________
__________
Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
66
Minimize
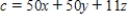
subject to
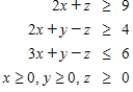
If there is no solution, do not fill the fields.
 __________
__________
 __________
__________
 __________
__________
 __________
__________
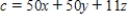
subject to
If there is no solution, do not fill the fields.
 __________
__________
 __________
__________
 __________
__________
 __________
__________
Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
67
Maximize

subject to
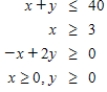
Please enter your answer as three numbers, separated by commas in the order p, x, y. If there is no solution, enter no solution.

subject to
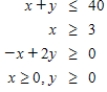
Please enter your answer as three numbers, separated by commas in the order p, x, y. If there is no solution, enter no solution.

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
68
Minimize
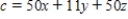
subject to
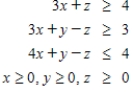
If there is no solution, do not fill the fields.
 __________
__________
 __________
__________
 __________
__________
 __________
__________
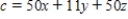
subject to
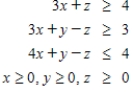
If there is no solution, do not fill the fields.
 __________
__________
 __________
__________
 __________
__________
 __________
__________
Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
69
We return to your exploits coordinating distribution for the Tubular Ride Boogie Board Company. You will recall that the company has manufacturing plants in Tucson, Arizona and Toronto, Ontario, and you have been given the job of coordinating distribution of their latest model, the Gladiator, to their outlets in Honolulu and Venice Beach. The Tucson plant can manufacture up to 620 boards per week, while the Toronto plant, beset by labor disputes, can produce no more than 410 Gladiator boards per week. The outlet in Honolulu orders 470 Gladiator boards per week, while Venice Beach orders 500 boards per week. Transportation costs are as follows: Tucson to Honolulu: $10 per board; Tucson to Venice Beach: $5 per board; Toronto to Honolulu: $20 per board; Toronto to Venice Beach: $10 per board. Your manager has said that you are to be sure to fill all orders and ship the boogie boards at a minimum total transportation cost. But you have just been notified that workers at the Toronto boogie board plant have gone on strike, resulting in a total work stoppage. You are to come up with a revised delivery schedule by tomorrow with the understanding that the Tucson plant can push production to a maximum of 810 boards per week. What should you do

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
70
Federal Rent-a-Car is putting together a new fleet. It is considering package offers from three car manufacturers. Fred Motors is offering 5 small cars, 5 medium cars, and 10 large cars for $500,000. Admiral Motors is offering 5 small, 10 medium, and 5 large cars for $400,000. Chrysalis is offering 10 small, 5 medium, and 5 large cars for $300,000. Federal would like to buy at least 750 small cars, at least 600 medium cars, and at least 750 large cars. How many packages should it buy from each car maker to keep the total cost as small as possible
__________ package(s) of Fred Motors, __________ package(s) of Admiral Motors, and __________ package(s) of Chrysalis.
What will be the total cost
$__________
__________ package(s) of Fred Motors, __________ package(s) of Admiral Motors, and __________ package(s) of Chrysalis.
What will be the total cost
$__________

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
71
Maximize
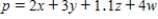
subject to

Round answers to two decimal places, if necessary.
If there is no solution, do not fill the fields.
 __________
__________
 __________
__________
 __________
__________
 __________
__________
 __________
__________
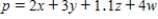
subject to

Round answers to two decimal places, if necessary.
If there is no solution, do not fill the fields.
 __________
__________
 __________
__________
 __________
__________
 __________
__________
 __________
__________
Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
72
Minimize

subject to
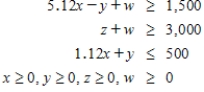
If there is no solution, do not fill the fields.
 __________
__________
 __________
__________
 __________
__________
 __________
__________
 __________
__________

subject to
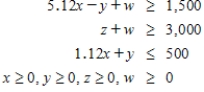
If there is no solution, do not fill the fields.
 __________
__________
 __________
__________
 __________
__________
 __________
__________
 __________
__________
Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
73
Use the simplex method to maximize , subject to the following.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
74
Fancy Pineapple produces pineapple juice and canned pineapple rings. This year the company anticipates a demand of at least 15,000 pints of pineapple juice and 2,500 cans of pineapple rings. Each pint of pineapple juice requires 2 pineapples, and each can of pineapple rings requires 1 pineapple. The company anticipates using at least 10,000 pineapples for these products. Each pint of pineapple juice costs the company 20 cents to produce, and each can of pineapple rings costs 50 cents to produce. How many pints of pineapple juice and cans of pineapple rings should Fancy Pineapple produce to meet the demand and minimize total costs
__________ pints of pineapple juice and __________ cans of pineapple rings.
__________ pints of pineapple juice and __________ cans of pineapple rings.

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
75
Minimize
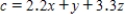
subject to
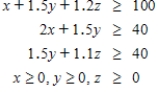
Round answers to two decimal places, if necessary.
If there is no solution, do not fill the fields.
 __________
__________
 __________
__________
 __________
__________
 __________
__________
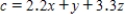
subject to
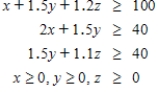
Round answers to two decimal places, if necessary.
If there is no solution, do not fill the fields.
 __________
__________
 __________
__________
 __________
__________
 __________
__________
Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
76
Your publishing company is about to start a promotional blitz for its new book, Physics for the Liberal Arts. You have 35 salespeople stationed in Chicago and 10 in Denver. You would like to fly at most 20 into Los Angeles and at most 20 into New York. A round-trip plane flight from Chicago to LA costs $195; from Chicago to NY costs $182; from Denver to LA costs $395; and from Denver to NY costs $166. You want to spend at most $7,380 on plane flights. How many salespeople should you fly from each of Chicago and Denver to each of LA and NY to have the most salespeople on the road ?
A)20 people from Chicago to LA, 10 people from Chicago to NY, no people from Denver to LA, and 10 people from Denver to NY
B) 10.0000000000 people from Chicago to LA, 10.0000000000 people from Chicago to NY, 20.0000000000 people from Denver to LA, and no people from Denver to NY
C) 10.0000000000 people from Chicago to LA, no people from Chicago to NY, 10.0000000000 people from Denver to LA, and 20.0000000000 people from Denver to NY
D) 10 people from Chicago to LA, 20 people from Chicago to NY, no people from Denver to LA, and 10 people from Denver to NY
E) 10 people from Chicago to LA, no people from Chicago to NY, 10 people from Denver to LA, and 10 people from Denver to NY
A)20 people from Chicago to LA, 10 people from Chicago to NY, no people from Denver to LA, and 10 people from Denver to NY
B) 10.0000000000 people from Chicago to LA, 10.0000000000 people from Chicago to NY, 20.0000000000 people from Denver to LA, and no people from Denver to NY
C) 10.0000000000 people from Chicago to LA, no people from Chicago to NY, 10.0000000000 people from Denver to LA, and 20.0000000000 people from Denver to NY
D) 10 people from Chicago to LA, 20 people from Chicago to NY, no people from Denver to LA, and 10 people from Denver to NY
E) 10 people from Chicago to LA, no people from Chicago to NY, 10 people from Denver to LA, and 10 people from Denver to NY

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
77
Maximize
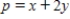
subject to
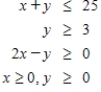
Please enter your answer as three numbers, separated by commas in the order p, x, y. If there is no solution, enter no solution.
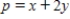
subject to
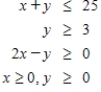
Please enter your answer as three numbers, separated by commas in the order p, x, y. If there is no solution, enter no solution.

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
78
The University's employee credit union has $4 million available for loans in the coming year. As VP in charge of finances, you must decide how much capital to allocate to each of four different kinds of loans, as shown in the table. State laws and credit union policies impose the following restrictions:
Signature loans may not exceed 10 percent of the total investment of funds.
Furniture loans plus other secured loans may not exceed automobile loans.
How much should you allocate to each type of loan to maximize the annual return
A)allocate $1,800,000 to automobile loans, $400,000 to signature loans, $1,800,000 to furniture loans and no allocation to other secured loans
B) allocate $1,800,000 to automobile loans, $400,000 to signature loans, no allocation to furniture loans and $1,800,000 other secured loans
C) allocate $400,000 to automobile loans, $1,800,000 to signature loans, and $1,800,000 to any combination of furniture loans and other secured loans
D) allocate $1,800,000 to automobile loans, $400,000 to signature loans, and $1,800,000 to any combination of furniture loans and other secured loans
E) allocate $1,800,000 to automobile loans, $400,000 to signature loans, and $400,000 to any combination of furniture loans and other secured loans
Signature loans may not exceed 10 percent of the total investment of funds.
Furniture loans plus other secured loans may not exceed automobile loans.
How much should you allocate to each type of loan to maximize the annual return
A)allocate $1,800,000 to automobile loans, $400,000 to signature loans, $1,800,000 to furniture loans and no allocation to other secured loans
B) allocate $1,800,000 to automobile loans, $400,000 to signature loans, no allocation to furniture loans and $1,800,000 other secured loans
C) allocate $400,000 to automobile loans, $1,800,000 to signature loans, and $1,800,000 to any combination of furniture loans and other secured loans
D) allocate $1,800,000 to automobile loans, $400,000 to signature loans, and $1,800,000 to any combination of furniture loans and other secured loans
E) allocate $1,800,000 to automobile loans, $400,000 to signature loans, and $400,000 to any combination of furniture loans and other secured loans

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
79
Your small farm encompasses 150 acres, and you are planning to grow tomatoes, lettuce, and carrots in the coming planting season. Fertilizer costs per acre are: $5 for tomatoes, $4 for lettuce, and $2 for carrots. Based on past experience, you estimate that each acre of tomatoes will require an average of 4 hours of labor per week, while tending to lettuce and carrots will each require an average of 2 hours per week. You estimate a profit of $2,000 for each acre of tomatoes, $1,500 for each acre of lettuce and $500 for each acre of carrots. You would like to spend at least $600 on fertilizer (your niece owns the company that manufactures it) and your farm laborers can supply up to 900 hours per week. How many acres of each crop should you plant to maximize total profits
Plant __________ acres of tomatoes, __________ acres of lettuce, and __________ acres of carrots.
In this event, will you be using all 150 acres of your farm
Plant __________ acres of tomatoes, __________ acres of lettuce, and __________ acres of carrots.
In this event, will you be using all 150 acres of your farm

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck
80
If x dollars is invested in a company that controls, say, 30 percent of the market with 5 brand-names, then is a measure of market exposure and is a measure of brand-name exposure. Now suppose you are a broker at a large securities firm, and one of your clients would like to invest up to $100,000 in recording industry stocks. You decide to recommend a combination of stocks in four of the world's largest companies: Warner Music, Universal Music, Sony, and EMI.
You wanted your client to maximize his total market exposure but limit his brand-name exposure to 2 million or less (representing an average of 20 labels or fewer per company), and still invest at least 20 percent of the total in Universal. How much should you advise your client to invest in each company
A)$20,000 in Warner Music, $80,000 in Sony, and no investment in Universal Music and EMI
B) $80,000 in Warner Music, $20,000 in EMI, and no investment in Universal Music and Sony
C) $20,000 in Universal Music, $80,000 in Sony, and no investment in Warner Music and EMI
D) $80,000 in Warner Music, $20,000 in Sony, and no investment in Universal Music and EMI
E) $80,000 in Universal Music, $20,000 in Sony, and no investment in Warner Music and EMI
You wanted your client to maximize his total market exposure but limit his brand-name exposure to 2 million or less (representing an average of 20 labels or fewer per company), and still invest at least 20 percent of the total in Universal. How much should you advise your client to invest in each company
A)$20,000 in Warner Music, $80,000 in Sony, and no investment in Universal Music and EMI
B) $80,000 in Warner Music, $20,000 in EMI, and no investment in Universal Music and Sony
C) $20,000 in Universal Music, $80,000 in Sony, and no investment in Warner Music and EMI
D) $80,000 in Warner Music, $20,000 in Sony, and no investment in Universal Music and EMI
E) $80,000 in Universal Music, $20,000 in Sony, and no investment in Warner Music and EMI

Unlock Deck
Unlock for access to all 161 flashcards in this deck.
Unlock Deck
k this deck


