Deck 11: Estimation: Describing a Single Population
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/127
Play
Full screen (f)
Deck 11: Estimation: Describing a Single Population
1
Which of the following statements is (are) correct?
A) The sample mean is a biased estimator of the population mean.
B) The sample proportion is an unbiased estimator of the population proportion.
C) The sample mean is not a consistent estimator.
D) All of these choices are correct.
A) The sample mean is a biased estimator of the population mean.
B) The sample proportion is an unbiased estimator of the population proportion.
C) The sample mean is not a consistent estimator.
D) All of these choices are correct.
B
2
Which of the following best describes an unbiased estimator?
A) Every estimator is an unbiased estimator.
B) An interval estimator is an unbiased estimator.
C) An unbiased estimator of a population parameter is an estimator whose expected value is equal to that parameter.
D) None of these choices are correct.
A) Every estimator is an unbiased estimator.
B) An interval estimator is an unbiased estimator.
C) An unbiased estimator of a population parameter is an estimator whose expected value is equal to that parameter.
D) None of these choices are correct.
C
3
Which of the following is the width of the confidence interval for the population mean?
A) Upper confidence limit + Lower confidence limit
B) (Upper confidence limit - Lower confidence)/2
C) Upper confidence limit - Lower confidence limit
D) None of these choices are correct.
A) Upper confidence limit + Lower confidence limit
B) (Upper confidence limit - Lower confidence)/2
C) Upper confidence limit - Lower confidence limit
D) None of these choices are correct.
C
4
Which of the following best describes an interval estimator?
A) An interval estimator is the same as a point estimator.
B) An interval estimator is an interval that draws inferences about a population based on a sample statistic.
C) An interval estimator can only be done for the population mean.
D) An interval estimator can only be done for the population proportion.
A) An interval estimator is the same as a point estimator.
B) An interval estimator is an interval that draws inferences about a population based on a sample statistic.
C) An interval estimator can only be done for the population mean.
D) An interval estimator can only be done for the population proportion.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
5
The width of a confidence interval estimate of the population mean widens when the:
A) level of confidence increases.
B) sample size increases.
C) population standard deviation decreases.
D) Sample mean gets further from the population mean.
A) level of confidence increases.
B) sample size increases.
C) population standard deviation decreases.
D) Sample mean gets further from the population mean.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
6
A point estimate is defined as:
A) the average of the sample values.
B) the average of the population values.
C) a single value of an estimator.
D) an interval within which the population parameter is believed to lie .
A) the average of the sample values.
B) the average of the population values.
C) a single value of an estimator.
D) an interval within which the population parameter is believed to lie .

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
7
The problem with relying on a point estimate of a population parameter is that:
A) it has no variance.
B) it might be unbiased.
C) it might not be relatively efficient.
D) it does not tell us how close or far the point estimate might be from the parameter.
A) it has no variance.
B) it might be unbiased.
C) it might not be relatively efficient.
D) it does not tell us how close or far the point estimate might be from the parameter.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
8
Which of the following statistical distributions is used when estimating the population mean when the population variance is unknown?
A) Student t distribution
B) Standard normal distribution
C) Chi-square distribution
D) None of these choices are correct.
A) Student t distribution
B) Standard normal distribution
C) Chi-square distribution
D) None of these choices are correct.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
9
In developing an interval estimate at 87.4% for a population mean, the value of z to use is:
A) 1.15.
B) 0.32.
C) 1.53.
D) 0.16.
A) 1.15.
B) 0.32.
C) 1.53.
D) 0.16.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
10
Which of the following statements are correct?
A) If there are two unbiased estimators of a parameter, the one whose variance is larger is said to be relatively efficient.
B) If there are two unbiased estimators of a parameter, the one whose mean is larger is said to be relatively efficient.
C) If there are two unbiased estimators of a parameter, the one whose mean is smaller is said to be relatively efficient.
D) If there are two unbiased estimators of a parameter, the one whose variance is smaller is said to be relatively efficient.
A) If there are two unbiased estimators of a parameter, the one whose variance is larger is said to be relatively efficient.
B) If there are two unbiased estimators of a parameter, the one whose mean is larger is said to be relatively efficient.
C) If there are two unbiased estimators of a parameter, the one whose mean is smaller is said to be relatively efficient.
D) If there are two unbiased estimators of a parameter, the one whose variance is smaller is said to be relatively efficient.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
11
Which of the following statistical distributions are used to find a confidence interval for the population proportion?
A) Student t distribution
B) Standard normal distribution
C) Chi-square distribution
D) None of these choices are correct.
A) Student t distribution
B) Standard normal distribution
C) Chi-square distribution
D) None of these choices are correct.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
12
In developing an interval estimate for a population mean, the interval estimate was 62.84 to 69.46. The population standard deviation was assumed to be 6.50, and a sample of 100 observations was used. The mean of the sample was:
A) 56.34.
B) 62.96.
C) 13.24.
D) 66.15.
A) 56.34.
B) 62.96.
C) 13.24.
D) 66.15.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
13
If the Student t distribution is incorrectly used instead of the Standard normal distribution when finding the confidence interval for the population mean, and the population variance was known, what will happen to the width of the confidence interval?
A) The confidence interval would have the same width.
B) The confidence interval would be wider than it should be, as t is flatter than Z.
C) The confidence interval will be narrower than it should be as t is flatter than Z.
D) None of these choices are correct.
A) The confidence interval would have the same width.
B) The confidence interval would be wider than it should be, as t is flatter than Z.
C) The confidence interval will be narrower than it should be as t is flatter than Z.
D) None of these choices are correct.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
14
In developing an interval estimate for a population mean, the population standard deviation was assumed to be 10. The interval estimate was 50.92 ± 2.14. Had equaled 20, the interval estimate would have been:
A) 60.92 ± 2.14.
B) 50.92 ± 12.14.
C) 101.84 ± 4.28.
D) 50.92 ± 4.28.
A) 60.92 ± 2.14.
B) 50.92 ± 12.14.
C) 101.84 ± 4.28.
D) 50.92 ± 4.28.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
15
A 95% confidence interval estimate for a population mean is determined to be 43.78 to 52.19. If the confidence level is decreased to 90%, the confidence interval :
A) becomes wider.
B) remains the same.
C) becomes narrower.
D) None of these choices are correct.
A) becomes wider.
B) remains the same.
C) becomes narrower.
D) None of these choices are correct.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
16
Which of the following statements is (are) true?
A) The sample mean is relatively more efficient than the sample median.
B) The sample median is relatively more efficient than the sample mean.
C) The sample variance is relatively more efficient than the sample variance.
D) All of these choices are correct.
A) The sample mean is relatively more efficient than the sample median.
B) The sample median is relatively more efficient than the sample mean.
C) The sample variance is relatively more efficient than the sample variance.
D) All of these choices are correct.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
17
The degrees of freedom used to find the t/2 for a confidence interval for the population mean?
A) The degrees of freedom are n − 2.
B) The degrees of freedom are n − 3.
C) The degrees of freedom are n − 1.
D) The degrees of freedom are n.
A) The degrees of freedom are n − 2.
B) The degrees of freedom are n − 3.
C) The degrees of freedom are n − 1.
D) The degrees of freedom are n.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
18
A 90% confidence interval estimate of the population mean can be interpreted to mean that:
A) if we repeatedly draw samples of the same size from the same population, 90% of the values of the sample means will result in a confidence interval that includes the population mean .
B) there is a 90% probability that the population mean will lie between the lower confidence limit (LCL) and the upper confidence limit (UCL).
C) we are 90% confident that we have selected a sample whose range of values does not contain the population mean .
D) We are 90% confident that 10% the values of the sample means will result in a confidence interval that includes the population mean .
A) if we repeatedly draw samples of the same size from the same population, 90% of the values of the sample means will result in a confidence interval that includes the population mean .
B) there is a 90% probability that the population mean will lie between the lower confidence limit (LCL) and the upper confidence limit (UCL).
C) we are 90% confident that we have selected a sample whose range of values does not contain the population mean .
D) We are 90% confident that 10% the values of the sample means will result in a confidence interval that includes the population mean .

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
19
Which of the following statistical distributions is used when estimating the population mean when the population variance is known?
A) Student t distribution
B) Standard normal distribution
C) Chi-square distribution
D) None of these choices are correct.
A) Student t distribution
B) Standard normal distribution
C) Chi-square distribution
D) None of these choices are correct.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
20
The z value for a 95% confidence interval estimate is:
A) 2.12.
B) 1.82.
C) 2.00.
D) 1.96.
A) 2.12.
B) 1.82.
C) 2.00.
D) 1.96.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
21
Under which of the following circumstances is it impossible to construct a confidence interval for the population mean?
A) A non-normal population with a large sample and an unknown population variance.
B) A normal population with a large sample and a known population variance.
C) A non-normal population with a small sample and an unknown population variance.
D) A normal population with a small sample and an unknown population variance.
A) A non-normal population with a large sample and an unknown population variance.
B) A normal population with a large sample and a known population variance.
C) A non-normal population with a small sample and an unknown population variance.
D) A normal population with a small sample and an unknown population variance.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
22
A random sample of size 20 taken from a normally distributed population resulted in a sample variance of 32. The lower limit of a 90% confidence interval for the population variance would be:
A) 52.185.
B) 20.375.
C) 20.170.
D) 54.931.
A) 52.185.
B) 20.375.
C) 20.170.
D) 54.931.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
23
The use of the standard normal distribution for constructing a confidence interval estimate for the population proportion p requires that:
A) n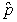 and n(1 -
and n(1 -  ) are both greater than 5, where
) are both greater than 5, where  denotes the sample proportion.
denotes the sample proportion.
B) np and n(1 - p) are both greater than 5.
C) n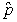 and n(p +
and n(p + 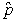 ) are both greater than 5.
) are both greater than 5.
D) the sample size is greater than 5.
A) n
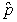 and n(1 -
and n(1 -  ) are both greater than 5, where
) are both greater than 5, where  denotes the sample proportion.
denotes the sample proportion.B) np and n(1 - p) are both greater than 5.
C) n
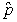 and n(p +
and n(p + 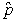 ) are both greater than 5.
) are both greater than 5.D) the sample size is greater than 5.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
24
Which of the following statements is false?
A) The t-distribution is symmetric about zero.
B) The t-distribution is more spread out than the standard normal distribution.
C) As the number of degrees of freedom gets smaller, the t-distribution's dispersion gets smaller.
D) The t-distribution is mound-shaped.
A) The t-distribution is symmetric about zero.
B) The t-distribution is more spread out than the standard normal distribution.
C) As the number of degrees of freedom gets smaller, the t-distribution's dispersion gets smaller.
D) The t-distribution is mound-shaped.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
25
The sample variance is an unbiased estimator of the population variance when the denominator of is:
A) n.
B) n - 1.
C)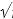 n.
n.
D) n + 1.
A) n.
B) n - 1.
C)
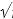 n.
n.D) n + 1.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
26
Which of the following is not a part of the formula for constructing a confidence interval estimate of the population mean?
A) A point estimate of the population mean.
B) The standard error of the sampling distribution of the sample mean.
C) The confidence level.
D) The value of the population mean.
A) A point estimate of the population mean.
B) The standard error of the sampling distribution of the sample mean.
C) The confidence level.
D) The value of the population mean.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
27
In selecting the sample size to estimate the population proportion p, if we have no knowledge of even the approximate values of the sample proportion 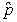 , we:
, we:
A) take another sample and estimate p.
B) take two more samples and find the average of their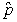 values.
values.
C) let = 0.50.
= 0.50.
D) let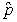 = 0.95.
= 0.95.
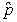 , we:
, we:A) take another sample and estimate p.
B) take two more samples and find the average of their
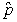 values.
values.C) let
 = 0.50.
= 0.50.D) let
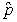 = 0.95.
= 0.95.
Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
28
The smaller the level of confidence used in constructing a confidence interval estimate of the population mean, the:
A) more likely that the confidence interval will contain the population mean.
B) wider the confidence interval.
C) narrower the confidence interval.
D) larger the sample required to estimate the population mean to within a certain error bound.
A) more likely that the confidence interval will contain the population mean.
B) wider the confidence interval.
C) narrower the confidence interval.
D) larger the sample required to estimate the population mean to within a certain error bound.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
29
A random sample of size 15 taken from a normally distributed population revealed a sample mean of 75 and a sample variance of 25. The upper limit of a 95% confidence interval for the population mean would equal:
A) 77.769.
B) 72.231.
C) 72.727.
D) 77.273.
A) 77.769.
B) 72.231.
C) 72.727.
D) 77.273.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
30
For a sample of size 30 taken from a normally distributed population with standard deviation equal to 5, a 95% confidence interval for the population mean would require the use of:
A) z = 1.96.
B) z = 1.645.
C) t = 2.045.
D) t = 1.699.
A) z = 1.96.
B) z = 1.645.
C) t = 2.045.
D) t = 1.699.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
31
In constructing a confidence interval for the population mean when the population variance is unknown, which of the following assumptions is required when using the following formula? 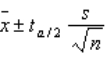
A) The sample size is greater than 30.
B) The population variance is known.
C) The population is normal.
D) The sample is drawn from a positively skewed distribution.
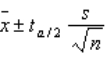
A) The sample size is greater than 30.
B) The population variance is known.
C) The population is normal.
D) The sample is drawn from a positively skewed distribution.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
32
Which of the following is true about the t-distribution?
A) It approaches the normal distribution as the number of degrees of freedom increases.
B) It assumes that the population is normally distributed.
C) It is more spread out than the standard normal distribution.
D) All of these choices are correct.
A) It approaches the normal distribution as the number of degrees of freedom increases.
B) It assumes that the population is normally distributed.
C) It is more spread out than the standard normal distribution.
D) All of these choices are correct.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
33
A confidence interval is defined as:
A) a point estimate plus or minus a specific level of confidence.
B) a lower and upper confidence limit associated with a specific level of confidence.
C) an interval that has a 95% probability of containing the population parameter.
D) a lower and upper confidence limit that has a 95% probability of containing the population parameter.
A) a point estimate plus or minus a specific level of confidence.
B) a lower and upper confidence limit associated with a specific level of confidence.
C) an interval that has a 95% probability of containing the population parameter.
D) a lower and upper confidence limit that has a 95% probability of containing the population parameter.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
34
A robust estimator is one that:
A) is unbiased and symmetrical about zero.
B) is consistent and is also mound-shaped.
C) is efficient and less spread out.
D) is not sensitive to moderate departure from the assumption of normality in the population.
A) is unbiased and symmetrical about zero.
B) is consistent and is also mound-shaped.
C) is efficient and less spread out.
D) is not sensitive to moderate departure from the assumption of normality in the population.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
35
The objective of estimation is to determine the approximate value of:
A) a sample statistic.
B) a population parameter.
C) the sample mean.
D) the sample variance.
A) a sample statistic.
B) a population parameter.
C) the sample mean.
D) the sample variance.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
36
Which of the following assumptions must be true in order to use the formula  to find a confidence interval estimate of the population mean?
to find a confidence interval estimate of the population mean?
A) The population variance is known.
B) The population mean is known.
C) The population is normally distributed.
D) The confidence level is greater than 90%.
 to find a confidence interval estimate of the population mean?
to find a confidence interval estimate of the population mean?A) The population variance is known.
B) The population mean is known.
C) The population is normally distributed.
D) The confidence level is greater than 90%.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
37
For statistical inference about the mean of a single population when the population standard deviation is unknown, the number of degrees for freedom for the t-distribution is equal to n - 1 because we lose one degree of freedom by using the:
A) sample mean as an estimate of the population mean.
B) sample standard deviation as an estimate of the population standard deviation.
C) sample proportion as an estimate of the population proportion.
D) sample size as an estimate of the population size.
A) sample mean as an estimate of the population mean.
B) sample standard deviation as an estimate of the population standard deviation.
C) sample proportion as an estimate of the population proportion.
D) sample size as an estimate of the population size.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
38
As the number of degrees of freedom for a t-distribution increases:
A) the dispersion of the distribution decreases.
B) the shape of the distribution becomes narrower and taller.
C) the t-distribution becomes more and more similar to the standard normal distribution.
D) All of these choices are correct.
A) the dispersion of the distribution decreases.
B) the shape of the distribution becomes narrower and taller.
C) the t-distribution becomes more and more similar to the standard normal distribution.
D) All of these choices are correct.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
39
The student t-distribution approaches the normal distribution as the:
A) number of degrees of freedom increases.
B) number of degrees of freedom decreases.
C) sample size decreases.
D) population size increases.
A) number of degrees of freedom increases.
B) number of degrees of freedom decreases.
C) sample size decreases.
D) population size increases.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
40
In the formula 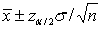 , the
, the  refers to:
refers to:
A) the probability that the confidence interval will contain the population mean.
B) the probability that the confidence interval will not contain the population mean.
C) the area in the lower tail or upper tail of the sampling distribution of the sample mean.
D) the level of confidence.
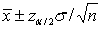 , the
, the  refers to:
refers to:A) the probability that the confidence interval will contain the population mean.
B) the probability that the confidence interval will not contain the population mean.
C) the area in the lower tail or upper tail of the sampling distribution of the sample mean.
D) the level of confidence.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
41
The upper limit of a confidence interval at the 99% level of confidence for the population proportion if a sample of size 100 had 40 successes is:
A) 0.3040.
B) 0.4047.
C) 0.4960.
D) 0.4806.
A) 0.3040.
B) 0.4047.
C) 0.4960.
D) 0.4806.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
42
An interval estimate is a range of values within which the actual value of a population parameter falls.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
43
The lower and upper limits of the 68.26% confidence interval for the population mean 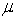 , given that n = 64,
, given that n = 64,  = 110 and
= 110 and 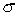 = 8, are 109 and 111, respectively.
= 8, are 109 and 111, respectively.
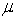 , given that n = 64,
, given that n = 64,  = 110 and
= 110 and 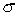 = 8, are 109 and 111, respectively.
= 8, are 109 and 111, respectively.
Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
44
An unbiased estimator is said to be consistent if the difference between the estimator and the parameter grows smaller as the sample size grows larger.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
45
The probability that a confidence interval includes the parameter of interest is either 1 or 0.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
46
Knowing that an estimator is unbiased only assures us that its expected value equals the parameter, but it does not tell us how close the estimator is to the parameter.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
47
A random sample of size n has been selected from a normally distributed population whose standard deviation is s. In estimating an interval for the population mean, the t-distribution should be used instead of the z-test if:
A) n ?
30 and is known.
B) is unknown and estimated by s, and the population is normal.
C) Population is normal and is known.
D) None of these choices are correct.
A) n ?
30 and is known.
B) is unknown and estimated by s, and the population is normal.
C) Population is normal and is known.
D) None of these choices are correct.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
48
A sample of 250 observations is to be selected at random from an infinite population. Given that the population proportion is 0.25, the standard error of the sampling distribution of the sample proportion is:
A) 0.0274.
B) 0.50.
C) 0.0316.
D) 0.0548.
A) 0.0274.
B) 0.50.
C) 0.0316.
D) 0.0548.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
49
An unbiased estimator of a population parameter is an estimator whose expected value is equal to the population parameter to be estimated.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
50
The sample mean  is a consistent estimator of the population mean
is a consistent estimator of the population mean 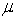 .
.
 is a consistent estimator of the population mean
is a consistent estimator of the population mean 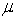 .
.
Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
51
An interval estimate is an estimate of the range for a population parameter.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
52
If the standard error of the sampling distribution of the sample proportion is 0.0337 for samples of size 200, then the population proportion must be:
A) 0.25.
B) 0.75.
C) either 0.20 or 0.80.
D) either 0.35 or 0.65.
E) either 0.30 or 0.70.
A) 0.25.
B) 0.75.
C) either 0.20 or 0.80.
D) either 0.35 or 0.65.
E) either 0.30 or 0.70.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
53
The sample standard deviation is an unbiased estimator of the population standard deviation.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
54
The width of a confidence interval increases as the level of significance increases.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
55
A sample of size 200 is to be taken at random from an infinite population. Given that the population proportion is 0.60, the probability that the sample proportion will be greater than 0.58 is:
A) 0.281.
B) 0.719.
C) 0.580.
D) 0.762.
A) 0.281.
B) 0.719.
C) 0.580.
D) 0.762.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
56
The sample proportion is a biased estimator of the population proportion.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
57
A confidence interval is an interval estimate for which there is a specified degree of certainty that the actual value of the population parameter will fall within the interval.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
58
The upper limit of the 90% confidence interval for  , given that n = 64,
, given that n = 64, 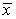 = 70 and
= 70 and  = 20, is 65.89.
= 20, is 65.89.
 , given that n = 64,
, given that n = 64, 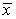 = 70 and
= 70 and  = 20, is 65.89.
= 20, is 65.89.
Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
59
The sample proportion 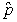 is a consistent estimator of the population proportion p because it is unbiased and the variance of
is a consistent estimator of the population proportion p because it is unbiased and the variance of 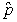 is p(1 - p)/n, which grows smaller as n grows larger.
is p(1 - p)/n, which grows smaller as n grows larger.
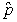 is a consistent estimator of the population proportion p because it is unbiased and the variance of
is a consistent estimator of the population proportion p because it is unbiased and the variance of 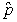 is p(1 - p)/n, which grows smaller as n grows larger.
is p(1 - p)/n, which grows smaller as n grows larger.
Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
60
A sample of size 300 is to be taken at random from an infinite population. Given that the population proportion is 0.70, the probability that the sample proportion will be smaller than 0.75 is:
A) 0.9706.
B) 0.4772.
C) 0.4706.
D) 0.9772.
A) 0.9706.
B) 0.4772.
C) 0.4706.
D) 0.9772.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
61
If there are two unbiased estimators of a parameter, the one whose variance is smaller is said to be relatively efficient.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
62
The difference between the sample statistic and actual value of the population parameter is the percentage of the confidence interval.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
63
A confidence interval becomes narrower as the sample size increases, for the same percentage of confidence.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
64
Suppose that a 90% confidence interval for 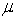 is given by
is given by 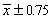 . This notation means that we are 90% confident that
. This notation means that we are 90% confident that  falls between
falls between 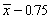 and
and 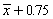 .
.
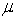 is given by
is given by 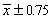 . This notation means that we are 90% confident that
. This notation means that we are 90% confident that  falls between
falls between 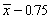 and
and 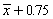 .
.
Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
65
In developing an interval estimate for a population mean, the population standard deviation 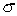 was assumed to be 8. The interval estimate was 50.0 ± 2.50. Had
was assumed to be 8. The interval estimate was 50.0 ± 2.50. Had 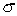 equalled 16, the interval estimate would have been 100 ± 5.0.
equalled 16, the interval estimate would have been 100 ± 5.0.
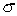 was assumed to be 8. The interval estimate was 50.0 ± 2.50. Had
was assumed to be 8. The interval estimate was 50.0 ± 2.50. Had 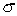 equalled 16, the interval estimate would have been 100 ± 5.0.
equalled 16, the interval estimate would have been 100 ± 5.0.
Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
66
The sample variance 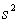 is an unbiased estimator of the population variance
is an unbiased estimator of the population variance 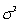 when the denominator of
when the denominator of 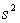 is n - 1.
is n - 1.
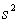 is an unbiased estimator of the population variance
is an unbiased estimator of the population variance 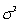 when the denominator of
when the denominator of 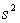 is n - 1.
is n - 1.
Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
67
When constructing confidence interval for a parameter, we generally set the confidence level  close to 1 (usually between 0.90 and 0.99) because we would like to be reasonably confident that the interval includes the actual value of the population parameter.
close to 1 (usually between 0.90 and 0.99) because we would like to be reasonably confident that the interval includes the actual value of the population parameter.
 close to 1 (usually between 0.90 and 0.99) because we would like to be reasonably confident that the interval includes the actual value of the population parameter.
close to 1 (usually between 0.90 and 0.99) because we would like to be reasonably confident that the interval includes the actual value of the population parameter.
Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
68
A 95% confidence interval estimate for a population mean 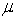 is determined to be 75 to 85. If the confidence level is reduced to 80%, the confidence interval for
is determined to be 75 to 85. If the confidence level is reduced to 80%, the confidence interval for 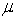 becomes narrower.
becomes narrower.
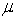 is determined to be 75 to 85. If the confidence level is reduced to 80%, the confidence interval for
is determined to be 75 to 85. If the confidence level is reduced to 80%, the confidence interval for 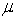 becomes narrower.
becomes narrower.
Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
69
It is possible to construct a confidence interval estimate of the population mean if the population variance is unknown.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
70
The percentage of the confidence interval relies on the significance level.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
71
In general, decreasing the confidence level ( 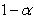 ) will narrow the interval.
) will narrow the interval.
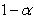 ) will narrow the interval.
) will narrow the interval.
Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
72
The range of a confidence interval is a measure of the expected sampling error.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
73
The width of the confidence interval estimate of the population mean  is a function of only two quantities, the population standard deviation
is a function of only two quantities, the population standard deviation 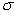 and the sample size n.
and the sample size n.
 is a function of only two quantities, the population standard deviation
is a function of only two quantities, the population standard deviation 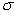 and the sample size n.
and the sample size n.
Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
74
At a given sample size and level of confidence, the smaller the population standard deviation 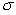 , the wider and thus the less precise the confidence interval estimate of
, the wider and thus the less precise the confidence interval estimate of 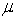 is.
is.
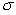 , the wider and thus the less precise the confidence interval estimate of
, the wider and thus the less precise the confidence interval estimate of 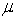 is.
is.
Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
75
The larger the level of confidence used in constructing a confidence interval, the wider the confidence interval.

Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
76
Suppose that a 95% confidence interval for  is given by
is given by  . This notation means that if we repeatedly draw samples of the same size from the same population, 95% of the values of
. This notation means that if we repeatedly draw samples of the same size from the same population, 95% of the values of 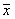 will be such that
will be such that 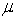 would lie somewhere between
would lie somewhere between 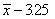 and
and 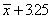 .
.
 is given by
is given by  . This notation means that if we repeatedly draw samples of the same size from the same population, 95% of the values of
. This notation means that if we repeatedly draw samples of the same size from the same population, 95% of the values of 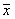 will be such that
will be such that 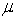 would lie somewhere between
would lie somewhere between 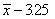 and
and 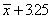 .
.
Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
77
In the formula 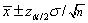 , the subscript
, the subscript 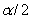 refers to the area in the lower tail or upper tail of the sampling distribution of the sample mean.
refers to the area in the lower tail or upper tail of the sampling distribution of the sample mean.
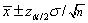 , the subscript
, the subscript 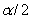 refers to the area in the lower tail or upper tail of the sampling distribution of the sample mean.
refers to the area in the lower tail or upper tail of the sampling distribution of the sample mean.
Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
78
The sample mean is an unbiased estimator of the population mean 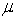 , and (when sampling from a normal population) the sample median is also an unbiased estimator of
, and (when sampling from a normal population) the sample median is also an unbiased estimator of 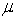 . However, the sample mean is relatively more efficient than the sample median.
. However, the sample mean is relatively more efficient than the sample median.
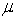 , and (when sampling from a normal population) the sample median is also an unbiased estimator of
, and (when sampling from a normal population) the sample median is also an unbiased estimator of 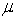 . However, the sample mean is relatively more efficient than the sample median.
. However, the sample mean is relatively more efficient than the sample median.
Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
79
When constructing a confidence interval estimate of  , doubling the sample size n reduces the width %of the interval by half.
, doubling the sample size n reduces the width %of the interval by half.
 , doubling the sample size n reduces the width %of the interval by half.
, doubling the sample size n reduces the width %of the interval by half.
Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck
80
We cannot interpret the confidence interval estimate of 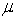 as a probability statement about
as a probability statement about 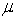 , simply because the population mean is a fixed but unknown quantity.
, simply because the population mean is a fixed but unknown quantity.
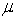 as a probability statement about
as a probability statement about 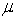 , simply because the population mean is a fixed but unknown quantity.
, simply because the population mean is a fixed but unknown quantity.
Unlock Deck
Unlock for access to all 127 flashcards in this deck.
Unlock Deck
k this deck


