Deck 13: Factoring
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/56
Play
Full screen (f)
Deck 13: Factoring
1
Factor completely:
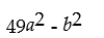
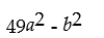
(7a - b)(7a + b)
2
Factor the special product: 
A) (x-8)²
B) (x-8)(x-8)
C) (x+8)²
D) (x+8)(x-8)

A) (x-8)²
B) (x-8)(x-8)
C) (x+8)²
D) (x+8)(x-8)
(x+8)(x-8)
3
Factor: 
A) (x+10)(x - 10)
B)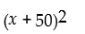
C)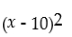
D) (x +50)(x - 50)

A) (x+10)(x - 10)
B)
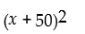
C)
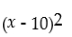
D) (x +50)(x - 50)
(x+10)(x - 10)
4
Factor completely: 
A) x(x +2)(x-2)
B)
C) x(x +4)
D) (x +2)(x-2)

A) x(x +2)(x-2)
B)

C) x(x +4)
D) (x +2)(x-2)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
5
Factor completely: 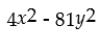
A) 4(x +9y)(x - 9y)
B) (4% +9y)(4x - 9y)
C) (x +9y)(2x - %)
D)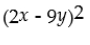
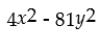
A) 4(x +9y)(x - 9y)
B) (4% +9y)(4x - 9y)
C) (x +9y)(2x - %)
D)
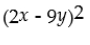

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
6
Factor the special product: 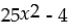
A) 25(x +2)(x - 2)
B) (5x +2)(5x - 2)
C) (x-2/5)(x+2/5)
D)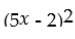
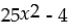
A) 25(x +2)(x - 2)
B) (5x +2)(5x - 2)
C) (x-2/5)(x+2/5)
D)
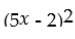

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
7
Factor completely: 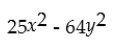
A)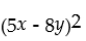
B) (5xy +8)(5xy - 8)
C) (5x +8y)(5x - 8y)
D) 25(x + 8y)(x - 8y)
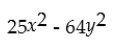
A)
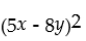
B) (5xy +8)(5xy - 8)
C) (5x +8y)(5x - 8y)
D) 25(x + 8y)(x - 8y)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
8
Factor completely: 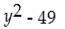
A) (y-7)(y-7)
B)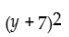
C)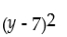
D) (y+7)(y-7)
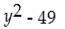
A) (y-7)(y-7)
B)
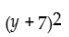
C)
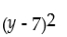
D) (y+7)(y-7)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
9
Factor completely: 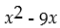
A)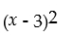
B) 9(x-1)
C) x(x-9)
D) (x+3)(x -3)
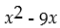
A)
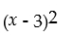
B) 9(x-1)
C) x(x-9)
D) (x+3)(x -3)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
10
Factor the special product: 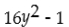
A) (4y +1)(4y - 1)
B) (4y - 1)²
C) (8y +1)(8y - 1)
D) (4y -1)(4y -1)
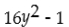
A) (4y +1)(4y - 1)
B) (4y - 1)²
C) (8y +1)(8y - 1)
D) (4y -1)(4y -1)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
11
Factor the special product: 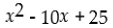
A) (x-5)²
B) (x+25)(x+1)
C) (x+5)(x-5)
D)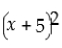
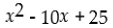
A) (x-5)²
B) (x+25)(x+1)
C) (x+5)(x-5)
D)
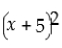

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
12
Factor the special product: 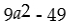
A) (3a+7)(3a-7)
B)
C) (3a-7)(3a-7)
D)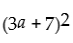
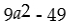
A) (3a+7)(3a-7)
B)

C) (3a-7)(3a-7)
D)
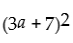

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
13
Factor the polynomial completely: 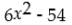
A) 6x(x -9)
B) 6(x - 1)(x +9)
C) (6x +1)(x -9)
D) 6(x +3)(x -3)
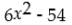
A) 6x(x -9)
B) 6(x - 1)(x +9)
C) (6x +1)(x -9)
D) 6(x +3)(x -3)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
14
Factor completely: 6ax +8a
A)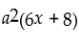
B)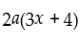
C) 6(ax +2a)
D) 20(3x +4a)
A)
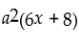
B)
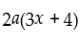
C) 6(ax +2a)
D) 20(3x +4a)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
15
Factor the special product: 
A) 36(x - y)(x - y)
B) (x +6y)(x - 6y)
C)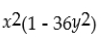
D)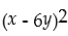

A) 36(x - y)(x - y)
B) (x +6y)(x - 6y)
C)
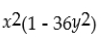
D)
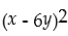

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
16
Factor the polynomial completely: 9x3-25x
A)(3X2+5)(3X-5)
B)X(9X+5)(9X-5)
C)X(3X+5)(3X-5)
D)9X(X+5)(X-5)
A)(3X2+5)(3X-5)
B)X(9X+5)(9X-5)
C)X(3X+5)(3X-5)
D)9X(X+5)(X-5)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
17
Factor completely:
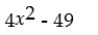
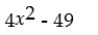

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
18
Factor completely: 
A)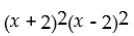
B)
C)
D)

A)
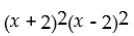
B)

C)

D)


Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
19
Factor completely:
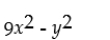
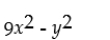

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
20
Factor completely:
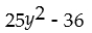
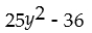

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
21
Factor completely: 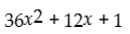
A)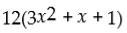
B)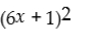
C) (6x +1)(6x - 1)
D) prime
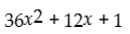
A)
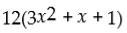
B)
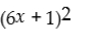
C) (6x +1)(6x - 1)
D) prime

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
22
Factor completely:
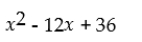
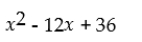

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
23
Factor: 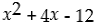
A) (x +2)(x-6)
B) (x +4)(x-3)
C) (x-2)(x +6)
D)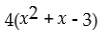
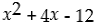
A) (x +2)(x-6)
B) (x +4)(x-3)
C) (x-2)(x +6)
D)
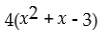

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
24
Factor the special product: 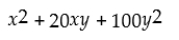

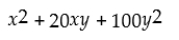


Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
25
Factor: 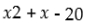
A)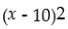
B) (x +4)(x - 5)
C) (x-4)(x +5)
D) (y - 1)(x +20)
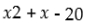
A)
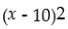
B) (x +4)(x - 5)
C) (x-4)(x +5)
D) (y - 1)(x +20)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
26
Factor the special product: 
A)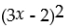
B)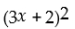
C)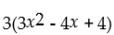
D) (3x +2)(3x - 2)

A)
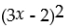
B)
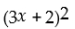
C)
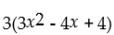
D) (3x +2)(3x - 2)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
27
Factor completely: 
A)(x - 2)(x - 3)
B)(x + 2)(x - 3)
C)(x + 1)(x - 6)
D)(x - 1)(x - 6)

A)(x - 2)(x - 3)
B)(x + 2)(x - 3)
C)(x + 1)(x - 6)
D)(x - 1)(x - 6)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
28
Factor: 
A) (x +2)(x +5)
B) (x-2)(x +5)
C) (x-2)(x-5)
D) (x +2)(x - 5)

A) (x +2)(x +5)
B) (x-2)(x +5)
C) (x-2)(x-5)
D) (x +2)(x - 5)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
29
Factor completely:
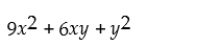
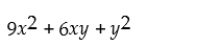

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
30
Factor the special product: 
A) (x+4)(x-4)
B)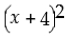
C) (x-4)²
D)

A) (x+4)(x-4)
B)
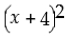
C) (x-4)²
D)


Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
31
Factor completely: 
A)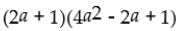
B)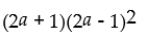
C)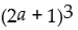
D)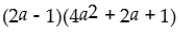

A)
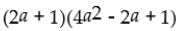
B)
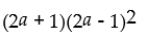
C)
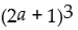
D)
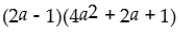

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
32
Factor completely: 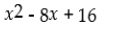
A)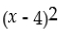
B)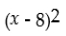
C)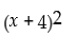
D)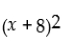
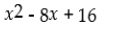
A)
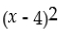
B)
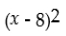
C)
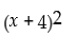
D)
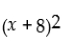

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
33
Simplify and factor: 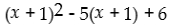
A) (x-2)(x-1)
B) x(x +7)
C) (x+4)(x-1)
D) (x-2)(x-3)
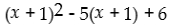
A) (x-2)(x-1)
B) x(x +7)
C) (x+4)(x-1)
D) (x-2)(x-3)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
34
Factor the special product: 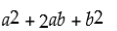
A)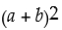
B)
C) (a+b)(a-b)
D)
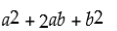
A)
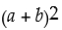
B)

C) (a+b)(a-b)
D)


Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
35
Factor: 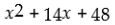
A) (x+6)(x +8)
B) (x - 2)(x +24)
C)(x+4)(x+12)
D) (x-6)(x-8)
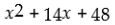
A) (x+6)(x +8)
B) (x - 2)(x +24)
C)(x+4)(x+12)
D) (x-6)(x-8)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
36
Factor completely: 
A) (x +3)(x -5)
B) (x -2)(x +5)
C) (x-3)(x-5)
D) (x +3)(x +5)

A) (x +3)(x -5)
B) (x -2)(x +5)
C) (x-3)(x-5)
D) (x +3)(x +5)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
37
Factor completely: 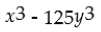
A)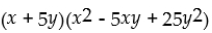
B)
C)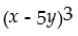
D)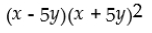
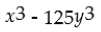
A)
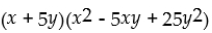
B)

C)
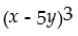
D)
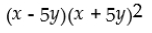

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
38
Factor: 
A) (y +3)(y +-15)
B) (y +5)(y +0)
C) (y+-9)(y-5)
D) (y -3)(y +15)

A) (y +3)(y +-15)
B) (y +5)(y +0)
C) (y+-9)(y-5)
D) (y -3)(y +15)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
39
Factor:
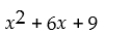
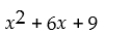

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
40
Factor completely:
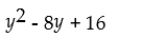
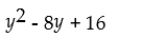

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
41
Factor completely 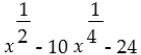 by comparing it to
by comparing it to 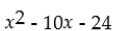
A)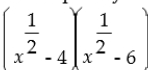
B)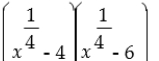
C)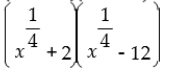
D)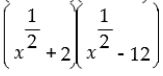
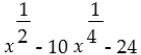 by comparing it to
by comparing it to 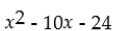
A)
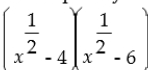
B)
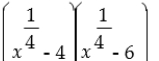
C)
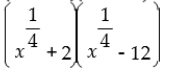
D)
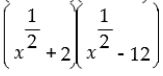

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
42
Factor completely: 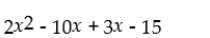
A)(x - 3)(2x - 5)
B)(x - 5)(2x + 3)
C)(x + 3)(2x - 5)
D)(x - 5)(2x - 3)
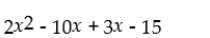
A)(x - 3)(2x - 5)
B)(x - 5)(2x + 3)
C)(x + 3)(2x - 5)
D)(x - 5)(2x - 3)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
43
Factor: 
A) (x +4)(x-6)
B) (X - 4)(x - 6)
C) (x-3)(x-8)
D) (x-3)(x +8)

A) (x +4)(x-6)
B) (X - 4)(x - 6)
C) (x-3)(x-8)
D) (x-3)(x +8)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
44
Factor: 
A)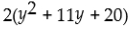
B) (y +2)(y +20)
C) (y +4)(y +10)
D) (y - 2)(y - 20)

A)
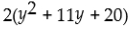
B) (y +2)(y +20)
C) (y +4)(y +10)
D) (y - 2)(y - 20)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
45
Factor: 
A) (x +3)(x +10)
B) (x +3)(x - 10)
C) (x +10)(x - 3)
D) prime

A) (x +3)(x +10)
B) (x +3)(x - 10)
C) (x +10)(x - 3)
D) prime

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
46
Factor completely:
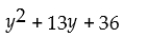
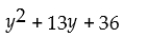

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
47
Factor: 
A) (x+5)(x+8)
B) (x +4)(x +10)
C) (x +3)(x +10)
D) (x +2)(x +20)

A) (x+5)(x+8)
B) (x +4)(x +10)
C) (x +3)(x +10)
D) (x +2)(x +20)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
48
Factor: x2 + 11x + 10
A)(x - 1)(x - 10)
B)(x + 2)(x + 5)
C)(x + 1)(x + 10)
D)(x + 5)(x + 6)
A)(x - 1)(x - 10)
B)(x + 2)(x + 5)
C)(x + 1)(x + 10)
D)(x + 5)(x + 6)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
49
Factor completely:
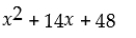
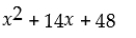

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
50
Factor completely:
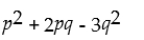
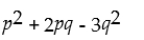

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
51
Factor completely:



Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
52
Factor completely: 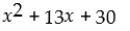
A)(x + 2)(x + 15)
B)(x + 3)(x + 10)
C)(x - 3)(x - 10)
D)(x - 2)(x + 15)
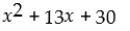
A)(x + 2)(x + 15)
B)(x + 3)(x + 10)
C)(x - 3)(x - 10)
D)(x - 2)(x + 15)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
53
Factor: x2 - 7x - 18
A)(x + 3)(x - 6)
B)(x - 3)(x - 6)
C)(x + 2)(x - 9)
D)(x - 2)(x + 9)
A)(x + 3)(x - 6)
B)(x - 3)(x - 6)
C)(x + 2)(x - 9)
D)(x - 2)(x + 9)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
54
Rewrite the trinomial as a polynomial with 4 terms that may be grouped: 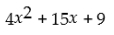
A)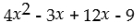
B)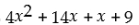
C)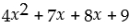
D)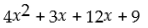
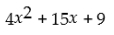
A)
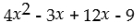
B)
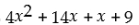
C)
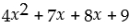
D)
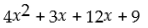

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
55
Factor completely: 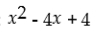
A)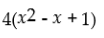
B)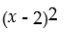
C) (x +2)(x-2)
D)
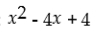
A)
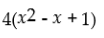
B)
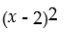
C) (x +2)(x-2)
D)


Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
56
Factor completely: 3ax + 6ay - 2x - 4y
A)(x + 2y)(3a - 2)
B)(x - 2y)(3x - 2)
C)-6a(x + 2y)
D)(x + 2y)(x - 2y)(3a - 2)
A)(x + 2y)(3a - 2)
B)(x - 2y)(3x - 2)
C)-6a(x + 2y)
D)(x + 2y)(x - 2y)(3a - 2)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck


