Deck 12: Oscillations
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/124
Play
Full screen (f)
Deck 12: Oscillations
1
A particle moving in simple harmonic motion with a period T = 1.5 s passes through the equilibrium point at time t0 = 0 with a velocity of 1.00 m/s to the right. A time t later, the particle is observed to move to the left with a velocity of 0.50 m/s. Note the change in direction of the velocity.) The smallest possible value of the time t is
A) 0.17 s
B) 0.33 s
C) 0.50 s
D) 0.25 s
E) 0.82 s
A) 0.17 s
B) 0.33 s
C) 0.50 s
D) 0.25 s
E) 0.82 s
0.50 s
2
The force constant of a massless spring is 25.0 N/m. A mass of 0.45 kg is oscillating in simple harmonic motion at the end of the spring with an amplitude of 0.32 m. The maximum speed of the mass is
A) 5.7 m/s
B) 56 m/s
C) 7.4 m/s
D) 2.4 m/s
E) 10 m/s
A) 5.7 m/s
B) 56 m/s
C) 7.4 m/s
D) 2.4 m/s
E) 10 m/s
2.4 m/s
3
Graph shows position of an oscillating particle as a function of time. What is the amplitude of the oscillation?
A) 8.0 cm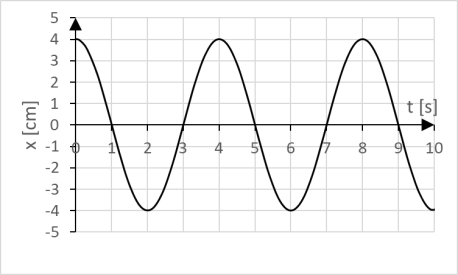
B) 4.0 cm
C) 1.0 s
D) 4.0 s
E) 0.25 Hz
A) 8.0 cm

B) 4.0 cm
C) 1.0 s
D) 4.0 s
E) 0.25 Hz
4.0 cm
4
A particle moving with a simple harmonic motion has its maximum displacement of +18 cm at time t = 0. The frequency of the motion is 10 s-1. At a time t = 0.65 s, the position of the particle is
A) +18 cm
B) zero
C) -13 cm
D) -18 cm
E) +7.3 cm
A) +18 cm
B) zero
C) -13 cm
D) -18 cm
E) +7.3 cm

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
5
Restoring force is such that it always pushes or pulls an object to its maximum displacement.

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
6
The frequency of a simple harmonic motion is 2.6 *10-4 s-1. The oscillation starts t = 0) when the displacement has its maximum positive value of 6.5 * 10-3 cm. The earliest possible time at which the particle can be found at x = -2.6 * 10-3 cm is
A) 7.1 *102 s
B) 1.2 * 103 s
C) 2.7 * 103 s
D) 7.6 * 103 s
E) 4.4 * 103 s
A) 7.1 *102 s
B) 1.2 * 103 s
C) 2.7 * 103 s
D) 7.6 * 103 s
E) 4.4 * 103 s

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
7
The instantaneous speed of a mass undergoing simple harmonic motion on the end of a spring depends on
A) the amplitude of oscillation.
B) the frequency of oscillation.
C) the period of oscillation.
D) the time at which the speed is measured.
E) all of these.
A) the amplitude of oscillation.
B) the frequency of oscillation.
C) the period of oscillation.
D) the time at which the speed is measured.
E) all of these.

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
8
The equation for the period T of a mass m oscillating with simple harmonic motion at the end of a spring with a force constant k is 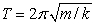 . A mass m that is oscillating on a spring with a force constant of 0.52 N/m has a period of 2.1 s. On a second spring, the same mass has a period of 3.5 s. The force constant of the second spring is
. A mass m that is oscillating on a spring with a force constant of 0.52 N/m has a period of 2.1 s. On a second spring, the same mass has a period of 3.5 s. The force constant of the second spring is
A) 0.43 N/m
B) 0.19 N/m
C) 1.4 N/m
D) 0.31 N/m
E) 0.75 N/m
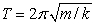 . A mass m that is oscillating on a spring with a force constant of 0.52 N/m has a period of 2.1 s. On a second spring, the same mass has a period of 3.5 s. The force constant of the second spring is
. A mass m that is oscillating on a spring with a force constant of 0.52 N/m has a period of 2.1 s. On a second spring, the same mass has a period of 3.5 s. The force constant of the second spring isA) 0.43 N/m
B) 0.19 N/m
C) 1.4 N/m
D) 0.31 N/m
E) 0.75 N/m

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
9
A spring is cut in half. The ratio of the force constant of one of the halves to the force constant of the original spring is
A)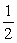
B) 1
C) 2
D) 4
E)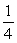
A)
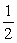
B) 1
C) 2
D) 4
E)
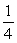

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
10
A particle with a mass of 65 g is undergoing simple harmonic motion. At time t = 0, the particle is at its extreme positive displacement of 18.0 cm. The period of the motion is 0.600 s. At time t = 1.35 s, the velocity of the particle is
A) -1.9 m/s
B) zero
C) 0.84 m/s
D) +1.9 m/s
E) -0.84 m/s
A) -1.9 m/s
B) zero
C) 0.84 m/s
D) +1.9 m/s
E) -0.84 m/s

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
11
A mass m hanging on a spring with a spring constant k executes simple harmonic motion with a period T. If the same mass is hung from a spring with a spring constant of 2k, the period of oscillation
A) increases by a factor of 2.
B) decreases by a factor of 2.
C) increases by a factor of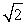 .
.
D) decreases by a factor of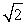 .
.
E) is not affected.
A) increases by a factor of 2.
B) decreases by a factor of 2.
C) increases by a factor of
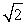 .
.D) decreases by a factor of
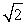 .
.E) is not affected.

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
12
A particle moving with simple harmonic motion has a maximum displacement of +12.0 cm. The particle moves from its maximum positive to its maximum negative displacement in 2.25 s. The motion starts when the displacement is x = +12.0 cm. The time for the particle to move to x = -6.00 cm is
A) 1.70 s
B) 0.750 s
C) 1.50 s
D) 2.20 s
E) 0.983 s
A) 1.70 s
B) 0.750 s
C) 1.50 s
D) 2.20 s
E) 0.983 s

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
13
Newborn baby's heart beats 120 times per minute. What is the frequency of baby's heartbeat?
A) 120 Hz
B) 2.0 Hz
C) 0.50 Hz
D) 1/120 Hz
E) 12.6 Hz
A) 120 Hz
B) 2.0 Hz
C) 0.50 Hz
D) 1/120 Hz
E) 12.6 Hz

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
14
You want a mass that, when hung on the end of a spring, oscillates with a period of 1 s. If the spring has a spring constant of 10 N/m, the mass should be
A) 10 kg
B)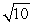 kg
kg
C) 4 210) kg
D) 10/4 2) kg
E) 4 2)/10
A) 10 kg
B)
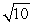 kg
kgC) 4 210) kg
D) 10/4 2) kg
E) 4 2)/10

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
15
Any body moving with simple harmonic motion is being acted on by a force that is
A) constant.
B) proportional to a sine or cosine function of the displacement.
C) proportional to the inverse square of the displacement.
D) directly proportional to the displacement.
E) proportional to the square of the displacement.
A) constant.
B) proportional to a sine or cosine function of the displacement.
C) proportional to the inverse square of the displacement.
D) directly proportional to the displacement.
E) proportional to the square of the displacement.

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
16
If F is the force, x the displacement, and k a particular constant, for simple harmonic motion we must have
A) F = -k/x2
B) F = k/x
C) F = k/x2)1/2
D) F = -kx2
E) F= -kx
A) F = -k/x2
B) F = k/x
C) F = k/x2)1/2
D) F = -kx2
E) F= -kx

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
17
When an object is oscillating in simple harmonic motion in the vertical direction, its maximum speed occurs when the object
A) is at its highest point.
B) is at its lowest point.
C) is at the equilibrium point.
D) has the maximum net force exerted on it.
E) has a position equal to its amplitude.
A) is at its highest point.
B) is at its lowest point.
C) is at the equilibrium point.
D) has the maximum net force exerted on it.
E) has a position equal to its amplitude.

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
18
It takes an oscillating particle 3.0 s to travel from maximum displacement to equilibrium position. What is the period of the oscillation?
A) 3.0 s
B) 6.0 s
C) 9.0 s
D) 12 s
E) It is impossible to determine the period without knowing the actual amplitude.
A) 3.0 s
B) 6.0 s
C) 9.0 s
D) 12 s
E) It is impossible to determine the period without knowing the actual amplitude.

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
19
A mass m hanging on a spring with a spring constant k has simple harmonic motion with a period T. If the mass is doubled to 2m, the period of oscillation
A) increases by a factor of 2.
B) decreases by a factor of 2.
C) increases by a factor of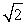 .
.
D) decreases by a factor of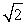 .
.
E) is not affected.
A) increases by a factor of 2.
B) decreases by a factor of 2.
C) increases by a factor of
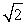 .
.D) decreases by a factor of
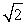 .
.E) is not affected.

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
20
In order for oscillations to occur, the restoring force has to be proportional to an object's displacement from equilibrium.

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
21
A ball moves back and forth in simple harmonic motion along a line 12 cm long. When the ball is 4 cm from the left-hand end of its path, it has an acceleration of 24 cm/s2. When the ball is 1 cm from the right-hand end of its path the magnitude of its acceleration is
A) 15 cm/s2
B) 48 cm/s2
C) 3 cm/s2
D) 60 cm/s2
E) 88 cm/s2
A) 15 cm/s2
B) 48 cm/s2
C) 3 cm/s2
D) 60 cm/s2
E) 88 cm/s2

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
22
A spring vibrates in simple harmonic motion according to the equationx = 15 cos twhere x is in centimeters and t is in seconds. The total number of vibrations this body makes in 10 s is
A) "0.5"
B) "10"
C) " "
D) "15"
E) "5"
A) "0.5"
B) "10"
C) " "
D) "15"
E) "5"

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
23
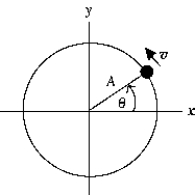 The object in the diagram is in circular motion. Its position at t = 0 was A, 0). Its frequency is f. The y component of its position is given by
The object in the diagram is in circular motion. Its position at t = 0 was A, 0). Its frequency is f. The y component of its position is given byA) y = y0 + v0yt +
 at2
at2B) y = A cos 2 ft
C) y = A sin ft
D) y = A sin 2 ft
E) y = A cos ft

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
24
A body of mass 5.0 kg moves in simple harmonic motion according to the equation x = 0.040 sin30t + /6) where the units are SI. The maximum speed of this body is approximately
A) 0.013 m/s
B) 0.40 m/s
C) 0.60 m/s
D) 1.2 m/s
E) 30 m/s
A) 0.013 m/s
B) 0.40 m/s
C) 0.60 m/s
D) 1.2 m/s
E) 30 m/s

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
25
An object oscillates with simple harmonic motion according to the equation x = 6.0 cos3t + /3) where the units are SI. The speed of the object when it is at the position of x = 3.0 m is
A) 18.0 m/s
B) 56.0 m/s
C) 48.9 m/s
D) 15.8 m/s
E) 5.73 m/s
A) 18.0 m/s
B) 56.0 m/s
C) 48.9 m/s
D) 15.8 m/s
E) 5.73 m/s

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
26
The equation of a body in simple harmonic motion is y = 8.0 cos20t + ) where y is in centimeters and t is in seconds. The frequency of the oscillations is
A) " /10 Hz"
B) " /4 Hz"
C) "10/ Hz"
D) "8 Hz"
E) "20 Hz"
A) " /10 Hz"
B) " /4 Hz"
C) "10/ Hz"
D) "8 Hz"
E) "20 Hz"

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
27
A particle is oscillating with simple harmonic motion. The frequency of the motion is 10 Hz and the amplitude of the motion is 5.0 cm. As the particle passes its central equilibrium position, the acceleration of the particle is
A) 100 cm/s2
B) 1.6 * 105 cm/s2
C) 4 * 106 cm/s2
D) zero
E) 3.2 * 106 cm/s2
A) 100 cm/s2
B) 1.6 * 105 cm/s2
C) 4 * 106 cm/s2
D) zero
E) 3.2 * 106 cm/s2

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
28
A body of mass 5.0 kg moves in simple harmonic motion according to the equation x = 0.040 sin30t + /6) where the units are SI. The period of this motion is
A) "1/30 s"
B) " /15 s"
C) " /6 s"
D) "15/ s"
E) "30 s"
A) "1/30 s"
B) " /15 s"
C) " /6 s"
D) "15/ s"
E) "30 s"

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
29
A particle moving in a circle of radius 15 cm makes 33.3 rev/min. If the particle starts on the positive x axis at time t = 0, what is the x component of the particle's velocity at time t = 1.2 s?
A) 45 cm/s
B) -3.8 cm/s
C) 26 cm/s
D) -45 cm/s
E) 13 cm/s
A) 45 cm/s
B) -3.8 cm/s
C) 26 cm/s
D) -45 cm/s
E) 13 cm/s

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
30
A body of mass M is executing simple harmonic motion with an amplitude of 8.0 cm and a maximum acceleration of 100 cm/s2. When the displacement of this body from the equilibrium position is 6.0 cm, the magnitude of the acceleration is approximately
A) 8.7 cm/s2
B) 66 cm/s2
C) 35 cm/s2
D) 17 cm/s2
E) 1.3 m/s2
A) 8.7 cm/s2
B) 66 cm/s2
C) 35 cm/s2
D) 17 cm/s2
E) 1.3 m/s2

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
31
A body moves with simple harmonic motion according to the equation x = 2/ ) sin4 t + /3), where the units are SI. At t = 2 s, the speed of the body is
A) 1/3 m/s
B) 1/ m/s
C)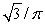 m/s
m/s
D) 4 m/s
E)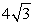 m/s
m/s
A) 1/3 m/s
B) 1/ m/s
C)
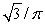 m/s
m/sD) 4 m/s
E)
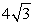 m/s
m/s
Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
32
A body moves in simple harmonic motion with amplitude A and period T. The minimum time for the body to move from a displacement of A to A/2 is
A) T/2
B) T/4
C) T/6
D) T/12
E) T/16
A) T/2
B) T/4
C) T/6
D) T/12
E) T/16

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
33
A 2.00-kg body is attached to a spring of negligible mass and oscillates with a period of 1.00 s. The force constant of the spring is
A) 0.051 N/m
B) 0.500 N/m
C) 2.00 N/m
D) 6.28 N/m
E) 79.0 N/m
A) 0.051 N/m
B) 0.500 N/m
C) 2.00 N/m
D) 6.28 N/m
E) 79.0 N/m

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
34
The acceleration of a particle moving with simple harmonic motion is given by a = -16.0 x, where x is in meters and a is in meters per second squared. The period of the motion is
A) 0.250 s
B) 0.392 s
C) 1.57 s
D) 4.00 s
E) 25.2 s
A) 0.250 s
B) 0.392 s
C) 1.57 s
D) 4.00 s
E) 25.2 s

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
35
In the following equations, a is acceleration, A amplitude, s is position, and m is mass. Which equation could describe simple harmonic motion?
A) a = -kA2
B) a = A2
C) a = -ks-1
D) a = 4 mA2/3
E) a = - 4 mx/3
A) a = -kA2
B) a = A2
C) a = -ks-1
D) a = 4 mA2/3
E) a = - 4 mx/3

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
36
In simple harmonic motion, the displacement x = A cos t and the acceleration a = - 2x. If A = 0.25 m and the period is 0.32 s, the acceleration when t = 0.12 s is
A) zero
B) +39 m/s2
C) -39 m/s2
D) +68 m/s2
E) -68 m/s2
A) zero
B) +39 m/s2
C) -39 m/s2
D) +68 m/s2
E) -68 m/s2

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
37
A body of mass 0.50 kg moves in simple harmonic motion with a period of 1.5 s and an amplitude of 20 mm. Which of the following equations correctly represents this motion?
A) x = 40 cost/1.5) mm
B) x = 40 cos2 t/1.5) mm
C) x = 20 sint/1.5) mm
D) x = 20 sin1.5 t) mm
E) x = 20 sin2 t/1.5) mm
A) x = 40 cost/1.5) mm
B) x = 40 cos2 t/1.5) mm
C) x = 20 sint/1.5) mm
D) x = 20 sin1.5 t) mm
E) x = 20 sin2 t/1.5) mm

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
38
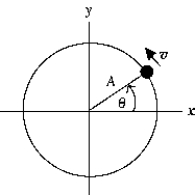 The object in the diagram is in circular motion with frequency f. At t = 0 it was at A, 0). The y component of its acceleration is given by
The object in the diagram is in circular motion with frequency f. At t = 0 it was at A, 0). The y component of its acceleration is given byA) ay = vy - v0y)/t
B) ay = -2 f)2A cos 2 ft
C) ay = -2 )2A sin 2 t
D) ay = -2 f)2A sin 2 ft
E) ay = -2 )2A cos 2 t

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
39
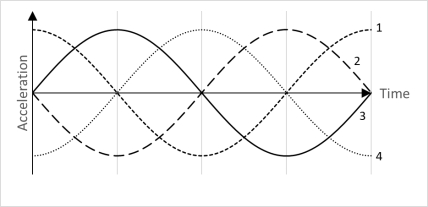
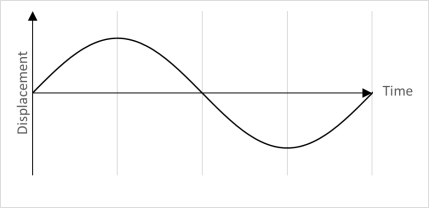 The top graph represents the variation of displacement with time for a particle executing simple harmonic motion. Which curve in the bottom graph represents the variation of acceleration with time for the same particle?
The top graph represents the variation of displacement with time for a particle executing simple harmonic motion. Which curve in the bottom graph represents the variation of acceleration with time for the same particle?A) 1
B) 2
C) 3
D) 4
E) None of these is correct.

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
40
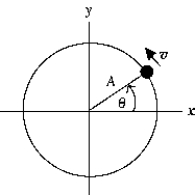 The object in the diagram is in circular motion with frequency f. At t = 0 it was at A, 0). The y component of its velocity is given by
The object in the diagram is in circular motion with frequency f. At t = 0 it was at A, 0). The y component of its velocity is given byA) vy = y - y0)/t
B) vy = 2 f)A cos 2 ft
C) vy = 2 )A sin 2 t
D) vy = 2 f)A sin 2 ft
E) vy = 2 )A cos 2 t

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
41
When the compression of a spring is doubled, the potential energy stored in the spring is
A) the same as before.
B) doubled.
C) tripled.
D) increased by a factor of 8.
E) quadrupled.
A) the same as before.
B) doubled.
C) tripled.
D) increased by a factor of 8.
E) quadrupled.

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
42
An object is moving with simple harmonic motion. When the object is displaced 4 cm from its equilibrium point, its acceleration is 20 cm/s2. Calculate the period T.
A) 0.45 s
B) 0.36 s
C) 2.2 s
D) 2.8 s
E) 5.0 s
A) 0.45 s
B) 0.36 s
C) 2.2 s
D) 2.8 s
E) 5.0 s

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
43
A 2.5-kg object is attached to a spring of force constant k = 4.5 kN/m. The spring is stretched 10 cm from equilibrium and released. What is the kinetic energy of the mass-spring system when the mass is 5.0 cm from its equilibrium position?
A) 5.6 J
B) 11 J
C) 17 J
D) 14 J
E) 42 J
A) 5.6 J
B) 11 J
C) 17 J
D) 14 J
E) 42 J

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
44
A light spring stretches 0.13 m when a 0.35 kg mass is hung from it. The mass is pulled down from this equilibrium position an additional 0.15 m and then released. Determine the maximum speed of the mass.
A) 1.10 m/s
B) 2.75 m/s
C) 11.4 m/s
D) 1.30 m/s
E) 0.02 m/s
A) 1.10 m/s
B) 2.75 m/s
C) 11.4 m/s
D) 1.30 m/s
E) 0.02 m/s

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
45
A particle is moving in SHM. If it has velocities of v1 and v2 when it is at positions x1 and x2 from the equilibrium position respectively, then the square of the frequency of vibration f 2) is given by
A) v12 -v22 ) / 4 2 x12 -x22 ).
B) v12 -v22 ) / 4 2 x22 -x12 ).
C) v12 + v22 ) / 4 2 x22 - x12 ).
D) v12 + v22 ) / 2 2 x22 + x12 ).
E) v12 + v22 ) / x22 -x12 ).
A) v12 -v22 ) / 4 2 x12 -x22 ).
B) v12 -v22 ) / 4 2 x22 -x12 ).
C) v12 + v22 ) / 4 2 x22 - x12 ).
D) v12 + v22 ) / 2 2 x22 + x12 ).
E) v12 + v22 ) / x22 -x12 ).

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
46
A mass attached to a spring has simple harmonic motion with an amplitude of 4.0 cm. When the mass is 2.0 cm from the equilibrium position, what fraction of its total energy is potential energy?
A) one-quarter
B) one-third
C) one-half
D) two-thirds
E) three-quarters
A) one-quarter
B) one-third
C) one-half
D) two-thirds
E) three-quarters

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
47
When the displacement of an object in simple harmonic motion is one-quarter of the amplitude A, the potential energy is what fraction of the total energy?
A)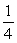
B)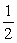
C)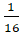
D)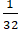
E)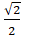
A)
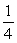
B)
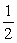
C)
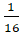
D)
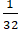
E)
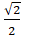

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
48
A light spring stretches 0.13 m when a 0.35-kg mass is hung from it. The mass is pulled down from this equilibrium position an additional 0.12 m and is released. Determine the spring constant k and the amplitude of vibration.
A) 26.4 N/m and 0.25 m
B) 26.4 N/m and 0.12 m
C) 2.70 N/m and 0.12 m
D) 2.70 N/m and 0.25 m
E) 26.4 N/m and 0.13 m
A) 26.4 N/m and 0.25 m
B) 26.4 N/m and 0.12 m
C) 2.70 N/m and 0.12 m
D) 2.70 N/m and 0.25 m
E) 26.4 N/m and 0.13 m

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
49
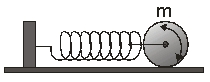 A spring of negligible mass and spring constant k is attached to the wall on one end, and the other end is attached to the center of mass axis of a solid sphere of mass m and radius r. The spring is stretched a distance A from equilibrium and let go. The sphere rolls freely on a smooth surface. What is the linear kinetic energy when the sphere is at the equilibrium position?
A spring of negligible mass and spring constant k is attached to the wall on one end, and the other end is attached to the center of mass axis of a solid sphere of mass m and radius r. The spring is stretched a distance A from equilibrium and let go. The sphere rolls freely on a smooth surface. What is the linear kinetic energy when the sphere is at the equilibrium position?A)

B)

C)
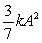
D)
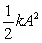
E)


Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
50
If the amplitude of a simple harmonic oscillator is doubled, the total energy is
A) unchanged.
B) one-fourth as large.
C) half as large.
D) doubled.
E) quadrupled.
A) unchanged.
B) one-fourth as large.
C) half as large.
D) doubled.
E) quadrupled.

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
51
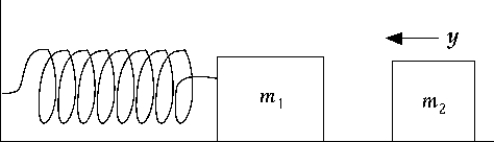 A mass of 0.50 kg is attached to a massless spring with a spring constant k = 600 N/m see figure above). The system rests on a level, friction-free surface and is initially at rest. A second mass of 0.20 kg makes an elastic head-on collision with the mass attached to the spring; thereafter, the oscillating system vibrates with an amplitude of 0.25 m. What was the incident speed of the second mass?
A mass of 0.50 kg is attached to a massless spring with a spring constant k = 600 N/m see figure above). The system rests on a level, friction-free surface and is initially at rest. A second mass of 0.20 kg makes an elastic head-on collision with the mass attached to the spring; thereafter, the oscillating system vibrates with an amplitude of 0.25 m. What was the incident speed of the second mass?A) 15 m/s
B) 8.7 m/s
C) 6.1 m/s
D) 11 m/s
E) 5.3 m/s

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
52
At t = 0 s a mass is at a position x = 0.33A and is moving to the right. The position of the mass is described by equation . The phase angle of this motion, is
A) 0.946 rad.
B) 70.7 rad.
C) 5.34 rad.
D) 0.324 rad.
E) 5.96 rad.
A) 0.946 rad.
B) 70.7 rad.
C) 5.34 rad.
D) 0.324 rad.
E) 5.96 rad.

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
53
The graph below shows velocity of a simple harmonic oscillator as a function of time. What is the amplitude of the oscillation?
A) 13.0 cm
B) 17.3 cm
C) 9.75 cm
D) 1.66 cm
E) 0.211 cm
A) 13.0 cm

B) 17.3 cm
C) 9.75 cm
D) 1.66 cm
E) 0.211 cm

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
54
The energy of a simple harmonic oscillator could be doubled by increasing the amplitude by a factor of
A) 0.7
B) 1.0
C) 1.4
D) 2.0
E) 4.0
A) 0.7
B) 1.0
C) 1.4
D) 2.0
E) 4.0

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
55
A particle is moving in SHM. If it has velocities of 6.0 cm/s and 1.0 cm/s when it is at positions 4.0 cm and 5.0 cm respectively from the equilibrium position the period of oscillation is
A) 3.9 s.
B) 3.2 s.
C) 2.0 s.
D) 4.4 s.
E) 4.1 s.
A) 3.9 s.
B) 3.2 s.
C) 2.0 s.
D) 4.4 s.
E) 4.1 s.

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
56
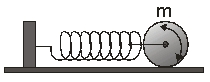 A spring of negligible mass and spring constant k is attached to the wall on one end, and the other end is attached to the center of mass axis of a solid sphere of mass m and radius r. The spring is stretched a distance A from equilibrium and let go. The sphere rolls freely on a smooth surface. What is the rotational kinetic energy when the sphere is at the equilibrium position?
A spring of negligible mass and spring constant k is attached to the wall on one end, and the other end is attached to the center of mass axis of a solid sphere of mass m and radius r. The spring is stretched a distance A from equilibrium and let go. The sphere rolls freely on a smooth surface. What is the rotational kinetic energy when the sphere is at the equilibrium position?A)
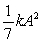
B)
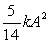
C)
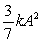
D)
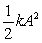
E)

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
57
A 2.50-kg object is attached to a spring of force constant k = 4.50 kN/m. The spring is stretched 10.0 cm from equilibrium and released. What is the maximum kinetic energy of this system?
A) 45.0 J
B) 22.5 J
C) 56.0 J
D) 2.25 * 105 J
E) 4.50 J
A) 45.0 J
B) 22.5 J
C) 56.0 J
D) 2.25 * 105 J
E) 4.50 J

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
58
A simple way to test if a device can withstand high "g-force" is to attach the device to a vibrating platform. Suppose a device has to withstand acceleration up to 5g, and the amplitude of the oscillation is 5.0 cm, the frequency of the vibration should be
A) 5 Hz
B) 981 Hz
C) 31.3 Hz
D) 44.3 Hz
E) 62.6 Hz
A) 5 Hz
B) 981 Hz
C) 31.3 Hz
D) 44.3 Hz
E) 62.6 Hz

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
59
An object is moving with simple harmonic motion. When the object is displaced 5.00 cm from its equilibrium point, its velocity is 15.0 cm/s. Calculate the amplitude of motion if its period of motion is 3.0 s.
A) 13.6 cm
B) 7.1 cm
C) 8.7 cm
D) 5.0 cm
E) 6.55 cm
A) 13.6 cm
B) 7.1 cm
C) 8.7 cm
D) 5.0 cm
E) 6.55 cm

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
60
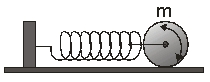 A spring of negligible mass and spring constant k is attached to the wall on one end, and the other end is attached to the center of mass axis of a solid sphere of mass m and radius r. The spring is stretched a distance A from equilibrium and let go. The sphere rolls freely on a smooth surface. The angular frequency of the oscillation is
A spring of negligible mass and spring constant k is attached to the wall on one end, and the other end is attached to the center of mass axis of a solid sphere of mass m and radius r. The spring is stretched a distance A from equilibrium and let go. The sphere rolls freely on a smooth surface. The angular frequency of the oscillation isA)

B)
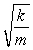
C)
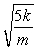
D)
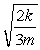
E)
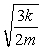

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
61
The displacement of a body moving with simple harmonic motion is given by the equation y = A sin2 t + ). After one-quarter of a period has elapsed since t = 0, which of the following statements is correct?
A) The total energy of the system is equal to zero.
B) The kinetic energy is a maximum.
C) The potential energy is a maximum.
D) The potential and kinetic energy are equal to each other.
E) Both kinetic and potential energies are at their maximum values.
A) The total energy of the system is equal to zero.
B) The kinetic energy is a maximum.
C) The potential energy is a maximum.
D) The potential and kinetic energy are equal to each other.
E) Both kinetic and potential energies are at their maximum values.

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
62
A body moving in simple harmonic motion has maximum acceleration when it has
A) maximum velocity.
B) maximum kinetic energy.
C) minimum potential energy.
D) minimum kinetic energy.
E) zero displacement.
A) maximum velocity.
B) maximum kinetic energy.
C) minimum potential energy.
D) minimum kinetic energy.
E) zero displacement.

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
63
A 10-kg block starts from rest at a vertical height of 1.0 m on a 30o frictionless inclined plane. If the block slides down the incline and then 20 m along a frictionless horizontal surface into a fixed spring with a force constant of 100 N/m, the spring is compressed approximately
A) 1.4 m
B) 2.0 m
C) 0.33 m
D) 0.98 m
E) 2.5 m
A) 1.4 m
B) 2.0 m
C) 0.33 m
D) 0.98 m
E) 2.5 m

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
64
A 0.50-kg mass is suspended from a massless spring that has a force constant of 79 N/m. The mass is displaced 0.1 m down from its equilibrium position and released. If the downward direction is negative, the displacement of the mass as a function of time is given by
A) y = 0.1 cos158t - )
B) y = 0.2 cos158t - )
C) y = 0.1 cos12.6t - )
D) y = 0.2 cos12.6t + )
E) y = 0.1 cos2t + )
A) y = 0.1 cos158t - )
B) y = 0.2 cos158t - )
C) y = 0.1 cos12.6t - )
D) y = 0.2 cos12.6t + )
E) y = 0.1 cos2t + )

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
65
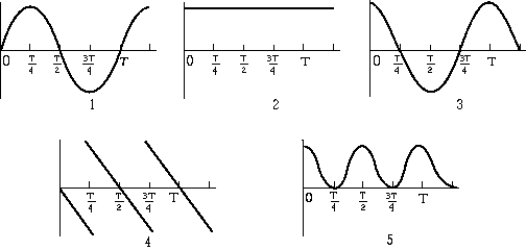 The kinetic energy of a body executing simple harmonic motion is plotted against time expressed in terms of the period T. At T = 0, the displacement is zero. Which of the graphs most closely represents these conditions?
The kinetic energy of a body executing simple harmonic motion is plotted against time expressed in terms of the period T. At T = 0, the displacement is zero. Which of the graphs most closely represents these conditions?A) 1
B) 2
C) 3
D) 4
E) 5

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
66
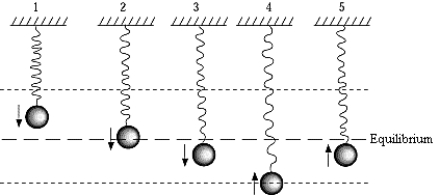 A body on a spring is vibrating in simple harmonic motion about an equilibrium position indicated by the dashed line. The figure above that shows the body with maximum acceleration is
A body on a spring is vibrating in simple harmonic motion about an equilibrium position indicated by the dashed line. The figure above that shows the body with maximum acceleration isA) 1
B) 2
C) 3
D) 4
E) 5

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
67
The displacement in simple harmonic motion is a maximum when the
A) acceleration is zero.
B) velocity is a maximum.
C) velocity is zero.
D) kinetic energy is a maximum.
E) potential energy is a minimum.
A) acceleration is zero.
B) velocity is a maximum.
C) velocity is zero.
D) kinetic energy is a maximum.
E) potential energy is a minimum.

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
68
A body of mass M suspended from a spring oscillates with a period T. If the mass of the spring can be neglected, a body of mass 2M, suspended from the same spring, oscillates with a period of
A) T/2
B)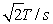
C) T
D)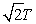
E) 2T
A) T/2
B)
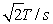
C) T
D)
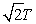
E) 2T

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
69
A 1.0-kg mass oscillates along the x axis with simple harmonic motion. Its position as a function of time is given by x = 2.0 cos t/6 + /3)
Where the units are SI. When t = 2.0 s, the kinetic energy of the mass is
A) " 2/24 J"
B) " 2/72 J"
C) "2 2/9 J"
D) "4 2/9 J"
E) " 2/4 J"
Where the units are SI. When t = 2.0 s, the kinetic energy of the mass is
A) " 2/24 J"
B) " 2/72 J"
C) "2 2/9 J"
D) "4 2/9 J"
E) " 2/4 J"

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
70
A 1.81-kg block slides on a horizontal frictionless table with a speed of 1.22 m/s. It is brought to rest by compressing a spring in its path. If the spring has a force constant of 7.30 N/m, it is compressed
A) 6.89 m.
B) 1.22 m.
C) 1.71 m.
D) 0.369 m.
E) 0.607 m.
A) 6.89 m.
B) 1.22 m.
C) 1.71 m.
D) 0.369 m.
E) 0.607 m.

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
71
Which of the following statements is true for a particle that is moving in simple harmonic motion?
A) The momentum of the particle is constant.
B) The kinetic energy of the particle is constant.
C) The elastic potential energy is constant.
D) The acceleration of the particle is constant.
E) The director of the force exerted on the particle by the spring is opposing the direction of the displacement from equilibrium.
A) The momentum of the particle is constant.
B) The kinetic energy of the particle is constant.
C) The elastic potential energy is constant.
D) The acceleration of the particle is constant.
E) The director of the force exerted on the particle by the spring is opposing the direction of the displacement from equilibrium.

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
72
A system consists of a mass vibrating on the end of a spring. The total mechanical energy of this system
A) varies as a sine or cosine function.
B) is constant only when the mass is at maximum displacement.
C) is a maximum when the mass is at its equilibrium position only.
D) is constant, regardless of the displacement of the mass from the equilibrium position.
E) is always equal to the square of the amplitude.
A) varies as a sine or cosine function.
B) is constant only when the mass is at maximum displacement.
C) is a maximum when the mass is at its equilibrium position only.
D) is constant, regardless of the displacement of the mass from the equilibrium position.
E) is always equal to the square of the amplitude.

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
73
A mass on a spring oscillates with an amplitude of 5.0 cm. What is the position of the mass when the kinetic and potential energies are equal?
A) 1.2 cm
B) 1.5 cm
C) 2.5 cm
D) 3.5 cm
E) 3.8 cm
A) 1.2 cm
B) 1.5 cm
C) 2.5 cm
D) 3.5 cm
E) 3.8 cm

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
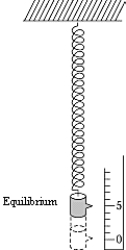
74
 The mass on the end of the spring which stretches linearly) is in equilibrium as shown. It is pulled down so that the pointer is opposite the 11-cm mark and then released. The mass experiences its maximum upward velocity at which of the following positions?
The mass on the end of the spring which stretches linearly) is in equilibrium as shown. It is pulled down so that the pointer is opposite the 11-cm mark and then released. The mass experiences its maximum upward velocity at which of the following positions?A) 3-cm mark
B) 7-cm mark
C) 1-cm mark
D) 11-cm mark
E) 14-cm mark.

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
75
In simple harmonic motion, the magnitude of the acceleration of a body is always directly proportional to its
A) displacement.
B) velocity.
C) mass.
D) potential energy.
E) kinetic energy.
A) displacement.
B) velocity.
C) mass.
D) potential energy.
E) kinetic energy.

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
76
An 8.0-kg block is attached to a spring with a constant of 2.0 N/m. If the spring is stretched 3.0 m from its equilibrium position and released from rest, the maximum velocity attained by the mass is
A) 0.75 m/s
B) 1.5 m/s
C) 3.0 m/s
D) 4.2 m/s
E) 15 m/s
A) 0.75 m/s
B) 1.5 m/s
C) 3.0 m/s
D) 4.2 m/s
E) 15 m/s

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
77
A 0.10-kg mass suspended from a vertical massless spring stretches it by 0.20 m from its equilibrium position. If this same mass is set into vibration on this spring, the frequency is
A) 0.023 Hz
B) 1.1 Hz
C) 2.0 Hz
D) 7.0 Hz
E) 13 Hz
A) 0.023 Hz
B) 1.1 Hz
C) 2.0 Hz
D) 7.0 Hz
E) 13 Hz

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
78
 A mass of 2.00 kg suspended from a spring 100 cm long is pulled down 4.00 cm from its equilibrium position and released. The amplitude of vibration of the resulting simple harmonic motion is
A mass of 2.00 kg suspended from a spring 100 cm long is pulled down 4.00 cm from its equilibrium position and released. The amplitude of vibration of the resulting simple harmonic motion isA) 4.00 cm
B) 2.00 cm
C) 8.00 cm
D) 1.04 cm
E) 1.02 cm

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
79
A 2.0-kg mass oscillates in one dimension with simple harmonic motion on the end of a massless spring on a horizontal frictionless table according to x = 6/ ) cos t + 3 ) where the units are SI. The total mechanical energy of this system is
A) 1.0 J
B) 3.0 J
C) 5.0 J
D) 7.0 J
E) 9.0 J
A) 1.0 J
B) 3.0 J
C) 5.0 J
D) 7.0 J
E) 9.0 J

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck
80
A small block of mass m = 0.130 kg is dropped from height h measured from the top of the spring of spring constant k = 370 N/m, which is originally in equilibrium. When the block hits the mass it compresses it by 2.5 cm. Determine the height from which the spring was dropped.
A) 0.12 m
B) 0.091 m
C) 0.066 m
D) 0.025 m
E) 0.16 m
A) 0.12 m
B) 0.091 m
C) 0.066 m
D) 0.025 m
E) 0.16 m

Unlock Deck
Unlock for access to all 124 flashcards in this deck.
Unlock Deck
k this deck


