Deck 8: Estimation and Confidence Intervals

Question 1

Recently, a university surveyed recent graduates of the English Department for their starting salaries. Four hundred graduates returned the survey. The average salary was $25,000 with a standard deviation of $2,500.
What is the best point estimate of the population mean?

A) $25,000
B) $2,500
C) 400
D) $62.5

Answer 1

A

Question 2

Mileage tests were conducted on a randomly selected sample of 100 newly developed automobile tires. The average tread life was found to be 80,000 kilometres with a standard deviation of 5,600 kilometres. What is the best estimate of the average tread life in miles for the entire population of these tires?

A) 80,000
B) 5,600
C) (80,000/100)
D) (5,600/100)
E) None of the choices are correct

Answer 2

A

Question 3

A random sample of 85 group leaders, supervisors, and similar personnel revealed that on the average a person spent 6.5 years on the job before being promoted. The standard deviation of the population was 1.7 years. Find the 95% confidence interval for the population mean.

A) 6.99 and 7.99
B) 4.15 and 7.15
C) 6.14 and 6.86
D) 6.49 and 7.49
E) None of the choices are correct

Answer 3

C

Question 4

The z-value associated with a 90% level of confidence is:

A) 1.96
B) 1.645
C) 2.33
D) 2.575
E) 1.28

Question 5

A sample standard deviation is the best point estimate of the

A) population range
B) population skewness
C) population mode
D) population standard deviation
E) population variance

Question 6

Recently, a university surveyed recent graduates of the English Department for their starting salaries. One hundred graduates returned the survey. The average salary was $35,000 with a standard deviation of $2,000.
What is the best point estimate of the population mean?

A) $25,000
B) $2,000
C) $500
D) $400
E) $35,000

Question 7

Which of the following would be used as a point estimate for the population mean (µ)?

A) ?
B) x/n
C) $\bar{x}$
D) s
E) None of the choices are correct

Question 8

A sample mean is the best point estimate of the

A) population standard deviation
B) population median
C) population mean
D) the sample standard deviation
E) the population variance

Question 9

Recently, a university surveyed recent graduates of the English Department for their starting salaries. Four hundred graduates returned the survey. The average salary was $25,000. The population standard deviation is known to be $2,500.
What is the 95% confidence interval for the mean salary of all graduates from the English
Department?

A) 22,500, $27,500
B) 24,755, $25,245
C) 24,988, $25,012
D) 24,600, $25,600

Question 10

The z-value associated with a 94% level of confidence is:

A) 1.96
B) 1.645
C) 2.33
D) 2.575
E) 1.88

Question 11

A sample of 50 is selected from a known population of 250 elements. The population standard deviation is 15. What is the standard error of the sample means using the finite population correction factor?

A) 2.89
B) 1.90
C) 2.12
D) 13.44
E) Cannot be determined

Question 12

The z-value associated with an 80% level of confidence is:

A) 1.96
B) 1.645
C) 2.33
D) 2.575
E) 1.28

Question 13

A 95% confidence interval infers that the population mean

A) is between 0 and 100%
B) is within ± 1.96 standard deviations of the sample mean
C) is within ± 1.96 standard errors of the sample mean
D) is within ± 1.645 standard deviations of the sample mean
E) is too large

Question 14

Recently, a university surveyed recent graduates of the English Department for their starting salaries. Four hundred graduates returned the survey. The average salary was $25,000. The population standard deviation is known to be $2,500.
Interpret the results of the 95% confidence interval.

A) The population mean is in the interval
B) The population mean is not in the interval
C) The likelihood that any confidence interval based on a sample of 100 graduates will contain the population mean is 0.95
D) There is a 5% chance that the computed interval does not contain the population mean.
E) Both C and D are correct

Question 15

A sample of 25 is selected from a known population of 100 elements. What is the finite population correction factor?

A) 8.66
B) 75
C) 0.87
D) Cannot be determined

Question 16

The z-value associated with a 96% level of confidence is:

A) 1.96
B) 1.645
C) 2.33
D) 2.05
E) 1.28

Question 17

A confidence interval for a population mean

A) estimates the population range
B) estimates a likely interval for a population mean
C) estimates a likelihood or probability
D) estimates the population standard deviation

Question 18

i. The 95 percent confidence interval states that 95 percent of the sample means of a specified sample size selected from a population will lie within plus and minus 1.96 standard deviations of the hypothesized population mean.
Ii) A distribution of sample means is normally distributed with a mean equal to the population mean and a standard deviation equal to the standard error of the mean.
Iii) A sample mean is the best point estimate of a population mean.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 19

Recently, a university surveyed recent graduates of the English Department for their starting salaries. Four hundred graduates returned the survey. The average salary was $25,000. The population standard deviation is known to be $2,500.
What is the 95% confidence interval for the mean salary of all graduates from the English
Department?

A) 3,496.80, $35,039.20
B) 34,608, $35,392
C) 34,671, $35,329
D) 34,960.80, $3,539.20
E) 34,800, $35,200

Question 20

Recently, a university surveyed recent graduates of the English Department for their starting salaries. Four hundred graduates returned the survey. The average salary was $25,000. The population standard deviation is known to be $2,500.
Interpret the results of the 95% confidence interval.

A) The population mean is in the interval
B) The population mean is not in the interval
C) The likelihood that any confidence interval based on a sample of 400 graduates will contain the population mean is 0.95
D) There is a 5% chance that the computed interval does not contain the population mean.

Question 21

i. The t distribution is positively skewed.
ii. All t distributions have the same mean of zero and a standard deviation of 1.
Iii) The t distribution is more spread out and flatter at the center than is the standard normal distribution. However, as the sample size increases, the t distribution curve approaches the standard normal distribution.

A) (i), (ii) and (iii) are all correct statements
B) (iii) is a correct statement but not (i) or (ii).
C) (i) and, (iii) are correct statements but not (ii).
D) (ii) and, (iii) are correct statements but not (i).
E) All statements are false

Question 22

A survey of 25 grocery stores revealed that the average price of a 4-litre bag of milk was $2.98 with a standard error of $0.10. What is the 95% confidence interval to estimate the true cost of a 4-litre bag of milk?

A) $2.81 to $3.15
B) $2.94 to $3.02
C) $2.77 to $3.19
D) $2.95 to $3.01
E) $2.73 to $3.23

Question 23

A survey of 25 grocery stores revealed that the average price of a 4-litre bag of milk was $2.98 with a standard error of $0.10. If 90% and 95% confidence intervals were developed to estimate the true cost of a 4-litre bag of milk, what similarities would they have?

A) Point estimates
B) t-statistics
C) Standard errors
D) Both "a" and "c"
E) None of the other statements are correct

Question 24

A survey of 144 retail stores revealed that the average price of a DVD was $375 with a standard error of $20. What is the 99% confidence interval to estimate the true cost of the DVD?

A) $323.40 to $426.60
B) $328.40 to $421.60
C) $335.00 to $415.00
D) $335.80 to $414.20
E) None of the choices are correct

Question 25

Dr. Patton is a professor of English. Recently she counted the number of misspelled works in a group of student essays. She noted the distribution of misspelled words per essay followed the normal distribution with a standard deviation of 2.44 words per essay. For her Tuesday class of 50 students, the mean number of misspelled words per essay was 6.05. Construct a 99% confidence interval for the mean number of misspelled words in the population of student essays.

A) 5.374 to 6.726
B) 5.161 to 6.939
C) 5.102 to 6.998
D) 5.482 to 6.618
E) 5.445 to 6.3655

Question 26

How does the t distribution differ from the standard z distribution?

A) Continuous distribution
B) Bell-shaped
C) Family of distributions
D) Symmetrical
E) None of the choices are correct

Question 27

i. The Student t distribution has a greater spread than does the z distribution. As a result, the critical values of t for a given level of significance are larger in magnitude than the corresponding z critical values.
Ii) The test statistic t has n-1 degrees of freedom.
Iii) William S. Gosset, a brewmaster, developed the t test for the Guinness Brewery in Ireland, who published it in 1908 using the pen name "Student."

A) (i), (ii) and (iii) are all correct statements
B) (iii) is a correct statement but not (i) or (ii).
C) (i) and, (iii) are correct statements but not (ii).
D) (ii) and, (iii) are correct statements but not (i).
E) All statements are false

Question 28

i. The t distribution is based on the assumption that the population of interest is normal or nearly normal.
Ii) The t distribution is a discrete distribution.
Iii) There is not one t distribution, but rather a "family" of t distributions.

A) (i), (ii) and (iii) are all correct statements
B) (i) is a correct statement but not (ii) or (iii).
C) (i) and, (iii) are correct statements but not (ii).
D) (ii) and, (iii) are correct statements but not (i).
E) All statements are false

Question 29

i. The test statistic for a problem involving a small sample of fewer than 30 and an unknown population standard deviation is the Student's t distribution.
Ii) The t distribution approaches the Z distribution as the sample size increases.
iii. As the sample size increases, the computed value of t decreases

A) (i), (ii) and (iii) are all correct statements
B) (iii) is a correct statement but not (i) or (ii).
C) (i) and, (iii) are correct statements but not (ii).
D) (ii) and, (iii) are correct statements but not (i).
E) All statements are false

Question 30

What kind of distribution is the t distribution?

A) Continuous
B) Discrete
C) Subjective
D) None of the choices are correct

Question 31

A survey of 25 grocery stores revealed that the average price of a 4-litre bag of milk was $2.98 with a standard error of $0.10. What is the 98% confidence interval to estimate the true cost of a 4-litre bag of milk?

A) $2.73 to $3.23
B) $2.85 to $3.11
C) $2.94 to $3.02
D) $2.95 to $3.01
E) $2.77 to $3.19

Question 32

A survey of 144 retail stores revealed that the average price of a DVD was $375 with a standard error of $20. If 95% and 98% confidence intervals were developed to estimate the true cost of the DVD, what differences would they have?

A) Standard errors
B) Interval widths
C) Z-values would be the same
D) Both "b" and "c"
E) None of the choices are correct

Question 33

i. The t distribution is based on the assumption that the population of interest is normal or nearly normal.
Ii) The t distribution is a continuous distribution.
Iii) There is not one t distribution, but rather a "family" of t distributions.

A) (i), (ii) and (iii) are all correct statements
B) (i) is a correct statement but not (ii) or (iii).
C) (i) and, (iii) are correct statements but not (ii).
D) (ii) and, (iii) are correct statements but not (i).
E) All statements are false

Question 34

Which statement(s) is/are correct about the t distribution?

A) Mean = 0
B) Symmetric
C) Based on degrees of freedom
D) All of the choices are correct are correct
E) None of the choices are correct is correct

Question 35

A survey of 144 retail stores revealed that the average price of a DVD was $375 with a standard error of $20. What is the 95% confidence interval to estimate the true cost of the DVD?

A) $323.40 to $426.60
B) $328.40 to $421.60
C) $335.00 to $415.00
D) $335.80 to $414.20
E) None of the choices are correct

Question 36

A survey of 144 retail stores revealed that the average price of a DVD was $375 with a standard error of $20. If 90% and 95% confidence intervals were developed to estimate the true cost of the DVD, what similarities would they have?

A) Point estimates
B) Z-values would be the same
C) Standard errors
D) Both "a" and "c"
E) None of the choices are correct

Question 37

Dr. Patton is a professor of English. Recently she counted the number of misspelled works in a group of student essays. She noted the distribution of misspelled words per essay followed the normal distribution with a standard deviation of 2.44 words per essay. For her Tuesday class of 50 students, the mean number of misspelled words per essay was 6.05. Construct a 90% confidence interval for the mean number of misspelled words in the population of student essays.

A) 5.374 to 6.726
B) 5.161 to 6.939
C) 5.102 to 6.998
D) 5.482 to 6.618
E) 5.445 to 6.3655

Question 38

Dr. Patton is a professor of English. Recently she counted the number of misspelled works in a group of student essays. She noted the distribution of misspelled words per essay followed the normal distribution with a standard deviation of 2.44 words per essay. For her Tuesday class of 44 students, the mean number of misspelled words per essay was 6.05. Construct a 90% confidence interval for the mean number of misspelled words in the population of student essays.

A) 5.374 to 6.726
B) 5.161 to 6.939
C) 5.102 to 6.998
D) 5.482 to 6.618
E) 5.445 to 6.3655

Question 39

Dr. Patton is a professor of English. Recently she counted the number of misspelled works in a group of student essays. She noted the distribution of misspelled words per essay followed the normal distribution with a standard deviation of 2.44 words per essay. For her Tuesday class of 50 students, the mean number of misspelled words per essay was 6.05. Construct a 95% confidence interval for the mean number of misspelled words in the population of student essays.

A) 5.374 to 6.726
B) 5.161 to 6.939
C) 5.102 to 6.998
D) 5.482 to 6.618
E) 5.445 to 6.3655

Question 40

A sample of 20 is selected from the population. What is the number of degrees of freedom used to determine the appropriate critical t-value?

A) 20
B) 19
C) 21
D) 25
E) None of the choices are correct

Question 41

A student wanted to quickly construct a 95% confidence interval for the average age of students in her statistics class. She randomly selected 9 students. Their average age was 19.1 years with a standard deviation of 1.5 years. What is the 95% confidence interval for the population mean?

A) [0.97, 3.27]
B) [15.64, 22.56]
C) [17.97, 20.23]
D) [17.95, 20.25]
E) [17.42, 20.78]

Question 42

In order to construct a 90% confidence interval for the population mean when the population standard deviation is unknown and the sample size is 18, you should use the t-value indicated as:

A) t0.10,18
B) t0.10,17
C) t0.05,18
D) t0.05,17
E) t0.90,17

Question 43

A student wanted to quickly construct a 95% confidence interval for the average age of students in her statistics class. She randomly selected 9 students. Their average age was 19.1 years with a standard deviation of 1.5 years. What is the best point estimate for the population mean?

A) 2.1 years
B) 1.5 years
C) 19.1 years
D) 9 years
E) 17.6 years

Question 44

A student wanted to quickly construct a 95% confidence interval for the average age of students in her statistics class. She randomly selected 9 students. Their average age was 19.1 years with a standard deviation of 1.5 years. What is the 99% confidence interval for the population mean?

A) [17.42, 20.78]
B) [17.48, 20.72]
C) [14.23, 23.98]
D) [0.44, 3.80]
E) [17.95, 20.25]

Question 45

A sample of 100 students is selected from a known population of 1,000 students to construct a 95% confidence interval for the average SAT score. What correction factor should be used to compute the standard error?

A) 0.949
B) 0.901
C) 1.96
D) 9.01
E) Cannot be determined

Question 46

A manager of a local store wants to estimate the mean amount spent per shopping visit by customers. Summary statistics from a sample taken reveal the following: 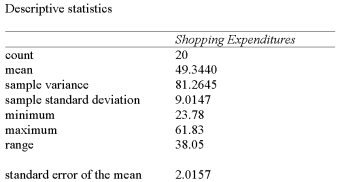If 95% and 98% confidence intervals were developed to estimate the true shopping expenditure, what differences would exist?

A) Standard errors
B) Interval widths
C) t-values
D) Both "b" and "c"
E) None of the choices are correct

Question 47

The t distribution approaches as the sample size increases. As the sample size increases, the computed value of t .

A) Z distribution; decreases
B) Z distribution; increases
C) Z distribution; stays the same
D) 0; decreases
E) 0; increases

Question 48

The t distribution is similar to the z distribution in all BUT ONE of the following characteristics. Which one is it?

A) Continuous
B) Symmetrical
C) Bell-shaped
D) t distribution's mean = 0 and standard deviation = 1
E) None of the choices are correct

Question 49

A statistics professor wishes to estimate the average mark on a term test for a course that has multiple sections and many students. A survey of some of the students registered for the course reveals the following results: The student from the course wonders whether the population mean could have been 75 or 80.

A) Since 80 is within the 95% confidence interval, the population mean is likely to be 80.
B) Since 80 is not within the 95% confidence interval, the population mean is not likely to be 80.
C) Since 75 is within the 95% confidence interval, the population mean is likely to be 75.
D) Since neither 75 nor 80 is within the 95% confidence interval, the population mean is not likely to be 75 or 80.
E) B & C are true

Question 50

In order to construct a 95% confidence interval for the population mean when the population standard deviation is unknown and the sample size is 15, you should use the t-value indicated as:

A) t0.10,15
B) t0.10,14
C) t0.05,15
D) t0.05,14
E) t0.025,14

Question 51

The distribution of Student's t has

A) a mean of zero and a standard deviation of one
B) a mean of one and a standard deviation of one
C) a mean of zero and a standard deviation that depends on the sample size
D) a mean that depends on the sample size and a standard deviation of one

Question 52

Student's t is used when

A) the sample is more than 30 observations
B) the sample size is ≤ 5% of the population
C) the population standard deviation is unknown
D) any time

Question 53

A statistics professor wishes to estimate the average mark on a term test for a course that has multiple sections and many students. A survey of some of the students registered for the course reveals the following results: 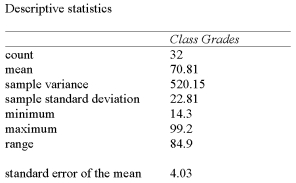If 90% and 95% confidence intervals were developed to estimate the true term test mean, what similarities would exist?

A) Point estimates
B) Z-values
C) Standard errors
D) Both "a" and "c"
E) None of the choices are correct

Question 54

A manager of a local store wants to estimate the mean amount spent per shopping visit by customers. Summary statistics from a sample taken reveal the following: Determine a 95% confidence interval for the mean amount spent.

A) [45.12, 53.56]
B) [40.36, 58.35]
C) [30.54, 68.14]
D) [45.14, 53.54]
E) [29.34, 69.34]

Question 55

A statistics professor wishes to estimate the average mark on a term test for a course that has multiple sections and many students. A survey of some of the students registered for the course reveals the following results: Determine a 98% confidence interval for the term test results.

A) [48.0, 93.6]
B) [66.8, 74.8]
C) [62.9, 78.71]
D) [64.2, 77.3]
E) [61.4, 80.2]

Question 56

A statistics professor wishes to estimate the average mark on a term test for a course that has multiple sections and many students. A survey of some of the students registered for the course reveals the following results: Determine a 95% confidence interval for the term test results.

A) [48.0, 93.6]
B) [66.8, 74.8]
C) [62.9, 78.71]
D) [64.2, 77.3]
E) [61.4, 80.2]

Question 57

A manager of a local store wants to estimate the mean amount spent per shopping visit by customers. Summary statistics from a sample taken reveal the following: 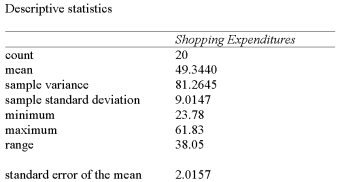The store manager wonders whether the population mean could have been $50 or $60.

A) Since $60 is within the 95% confidence interval, the population mean is likely to be $60.
B) Since $60 is not within the 95% confidence interval, the population mean is not likely to be $60.
C) Since $50 is within the 95% confidence interval, the population mean is likely to be $50.
D) Since $50 is not within the 95% confidence interval, the population mean is not likely to be $50.
E) B & C are true

Question 58

A manager of a local store wants to estimate the mean amount spent per shopping visit by customers. Summary statistics from a sample taken reveal the following: 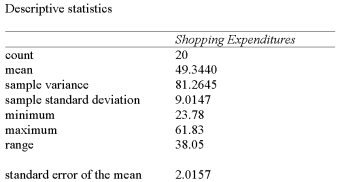If 90% and 95% confidence intervals were developed to estimate the true shopping expenditure, what similarities would exist?

A) Point estimates
B) t-values would be the same
C) Standard errors
D) Both "a" and "c"
E) None of the choices are correct

Question 59

A statistics professor wishes to estimate the average mark on a term test for a course that has multiple sections and many students. A survey of some of the students registered for the course reveals the following results: If 95% and 98% confidence intervals were developed to estimate the true term test mean, what differences would exist?

A) Standard errors
B) Interval widths
C) Z-values
D) Both "b" and "c"
E) None of the choices are correct

Question 60

The distribution of Student's t is

A) symmetrical
B) negatively skewed
C) positively skewed
D) a discrete probability distribution.

Question 61

The following summarizes the amount of snowfall in Ontario over the past number of years. You wonder whether the population mean could have been 2000 or 1900.

A) Since 1900 is within the 95% confidence interval, the population mean is likely to be 1900.
B) Since 1900 is within the 95% confidence interval, the population mean is not likely to be 1900.
C) Since 2000 is within the 95% confidence interval, the population mean is likely to be 2000.
D) Since neither 1900 nor 200 is within the 95% confidence interval, the population mean is not likely to be 1900 or 2000.
E) A & C are true

Question 62

The mean weight of trucks traveling on a particular section of Highway 401 is not known. A provincial highway inspector needs an estimate of the mean. He selects a random sample of 49 trucks passing the weighing station and finds the mean is 15.8 tons, with a standard deviation of the sample of 3.8 tons. What is the 95 percent interval for the population mean?

A) 14.7 and 16.9
B) 13.2 and 17.6
C) 10.0 and 20.0
D) 16.1 and 18.1
E) None of the choices are correct

Question 63

The following summarizes the amount of snowfall in Ontario over the past number of years. Determine a 95% confidence interval for the average annual snowfall.

A) [1,698, 2,046]
B) [1,665, 2,079]
C) [1,213, 2,531]
D) [1,783, 1,961]
E) [0, 3163]

Question 64

The following summarizes the average price of Air Canada stock at the end of 20 randomly selected weeks in 2000. Determine a 90% confidence interval for the average Air Canada stock price in 2000.

A) [16.23, 18.55]
B) [14.90, 19.87]
C) [17.48, 19.40]
D) [16.43, 18.35]
E) Unable to determine from the information given

Question 65

The Sugar Producers Association wants to estimate the mean yearly sugar consumption. A sample of 16 people reveals the mean yearly consumption to be 27 kg with a sample standard deviation of 9 kg. Assume a normal population. For a 95% confidence interval, what is the critical value needed?

A) t = 1.753
B) t = 2.131
C) t = 2.947
D) z = 1.645
E) z = 1.96

Question 66

The Sugar Producers Association wants to estimate the mean yearly sugar consumption. A sample of 25 people reveals the mean yearly consumption to be 27 kg with a sample standard deviation of 9 kg. Assume a normal population. For a 95% confidence interval, what is the critical value needed?

A) t = 2.787
B) t = 2.064
C) t = 2.797
D) z = 1.645
E) z = 1.96

Question 67

7 years. What is the 95% confidence interval for the true population mean?

A) 6.46 and 6.54
B) 3.17 and 9.83
C) 6.14 and 6.86
D) 6.20 and 6.70
E) 6.32 and 6.88

Question 68

The following summarizes the average price of Air Canada stock at the end of 20 randomly selected weeks in 2000.

A) [9467.57, 10 084.68]
B) [9401.76, 10 150.50]
C) [9628.70, 9923.55]
D) [9468.61, 10 083.65]
E) Unable to determine from the information given

Question 69

The following summarizes the average price of Air Canada stock at the end of 20 randomly selected weeks in 2000. Determine a 98% confidence interval for the average TSE 300 stock index in 2000.

A) [9467.57, 10 084.68]
B) [9401.76, 10 150.50]
C) [9628.70, 9923.55]
D) [9468.61, 10 083.65]
E) Unable to determine from the information given

Question 70

The Sugar Producers Association wants to estimate the mean yearly sugar consumption. A sample of 16 people reveals the mean yearly consumption to be 27 kg with a sample standard deviation of 9 kg. Assume a normal population. For a 99% confidence interval, what is the critical value needed?

A) t = 1.753
B) t = 2.131
C) t = 2.947
D) z = 1.645
E) z = 1.96

Question 71

The following summarizes the amount of snowfall in Ontario over the past number of years. Determine a 98% confidence interval for the average annual snowfall.

A) [1698, 2046]
B) [1665, 2079]
C) [1213, 2531]
D) [1783, 1961]
E) [1698, 2079]

Question 72

The Sugar Producers Association wants to estimate the mean yearly sugar consumption. A sample of 25 people reveals the mean yearly consumption to be 27 kg with a sample standard deviation of 9 kg. Assume a normal population. For a 99% confidence interval, what is the critical value needed?

A) t = 1.708
B) t = 1.711
C) t = 2.797
D) z = 1.645
E) z = 1.96

Question 73

The following summarizes the amount of snowfall in Ontario over the past number of years. 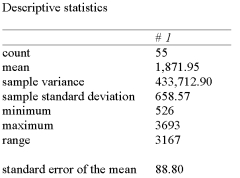If 95% and 98% confidence intervals were developed to estimate the true average annual snowfall, what differences would exist?

A) Standard errors
B) Interval widths
C) Z-values
D) Both "b" and "c"
E) None of the choices are correct

Question 74

The following summarizes the average price of Air Canada stock at the end of 20 randomly selected weeks in 2000. Determine a 95% confidence interval for the average Air Canada stock price in 2000.

A) [16.83, 17.42]
B) [14.90, 19.87]
C) [17.48, 19.40]
D) [16.43, 18.35]
E) [16.23, 18.55]

Question 75

The following summarizes the amount of snowfall in Ontario over the past number of years. 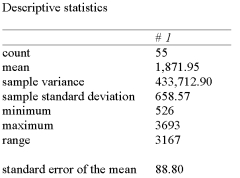If 90% and 95% confidence intervals were developed to estimate the true average annual snowfall, what similarities would they have?

A) Point estimates
B) Z-values
C) Standard errors
D) Both "a" and "c"
E) None of the choices are correct

Question 76

A pharmaceutical company wanted to estimate the population mean of monthly sales for their 250 sales people. Forty sales people were randomly selected. Their mean monthly sales were $10,000 with a standard deviation of $1,000. Construct a 95% confidence interval for the population mean.

A) [9,690.1, 10,309.9]
B) [9,715.5, 10,284.5]
C) [8,040, 11,960]
D) [8,000, 12,000]
E) [9,000, 11,000]

Question 77

The following summarizes the amount of snowfall in Ontario over the past number of years. You wonder whether the population mean could have been 1,600 or 2,100.

A) Since 1,600 is within the 95% confidence interval, the population mean is likely to be 1,600.
B) Since 1,600 is not within the 95% confidence interval, the population mean is not likely to be 1,600.
C) Since 2,100 is within the 95% confidence interval, the population mean is likely to be 2,100.
D) Since neither 1,600 nor 2,100 is within the 95% confidence interval, the population mean is not likely to be 1,600 or 2000.
E) B & C are true

Question 78

The Sugar Producers Association wants to estimate the mean yearly sugar consumption. A sample of 16 people reveals the mean yearly consumption to be 27 kg with a sample standard deviation of 9 kg. Assume a normal population. For a 90% confidence interval, what is the critical value needed?

A) t = 1.753
B) t = 2.131
C) t = 2.947
D) z = 1.645
E) z = 1.96

Question 79

The Sugar Producers Association wants to estimate the mean yearly sugar consumption. A sample of 25 people reveals the mean yearly consumption to be 27 kg with a sample standard deviation of 9 kg. Assume a normal population. For a 90% confidence interval, what is the critical value needed?

A) t = 1.708
B) t = 1.711
C) t = 2.797
D) z = 1.645
E) z = 1.96

Question 80

A statistics professor wishes to estimate the average mark on a term test for a course that has multiple sections and many students. A survey of some of the students registered for the course reveals the following results: 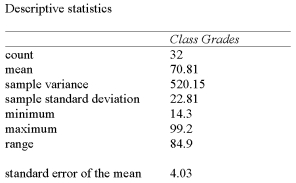The student from the course wonders whether the population mean could have been 60 or 80.

A) Since 80 is within the 95% confidence interval, the population mean is likely to be 80.
B) Since 80 is not within the 95% confidence interval, the population mean is not likely to be 80.
C) Since 60 is within the 95% confidence interval, the population mean is likely to be 60.
D) Since neither 60 nor 80 is within the 95% confidence interval, the population mean is not likely to be 60 or 80.
E) B & C are true