Deck 10: Conic Sections and Analytic Geometry
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/228
Play
Full screen (f)
Deck 10: Conic Sections and Analytic Geometry
1
Find the standard form of the equation of the ellipse satisfying the given conditions.
Foci: ; vertices:
A)
B)
C)
D)
Foci: ; vertices:
A)
B)
C)
D)
A
2
Write Equations of Ellipses in Standard Form
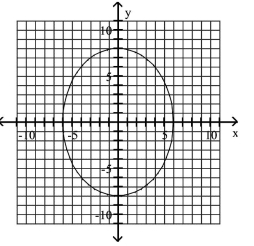
A)
foci at and
B)
foci at and
C)
foci at and
D)
foci at and

A)
foci at and
B)
foci at and
C)
foci at and
D)
foci at and
A
3
Write Equations of Ellipses in Standard Form
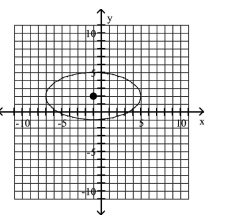
Center at
A)
foci at and
B)
foci at and
C)
foci at and
D)
foci at and

Center at
A)
foci at and
B)
foci at and
C)
foci at and
D)
foci at and
A
4
Write Equations of Ellipses in Standard Form

A)
foci at and
B)
foci at and
C)
foci at and
D)
foci at and

A)
foci at and
B)
foci at and
C)
foci at and
D)
foci at and

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
5
Find the standard form of the equation of the ellipse satisfying the given conditions.
Endpoints of major axis: and ; endpoints of minor axis: and ;
A)
B)
C)
D)
Endpoints of major axis: and ; endpoints of minor axis: and ;
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
6
Find the standard form of the equation of the ellipse satisfying the given conditions.
Foci: ; vertices:
A)
B)
C)
D)
Foci: ; vertices:
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
7
Graph the ellipse and locate the foci.
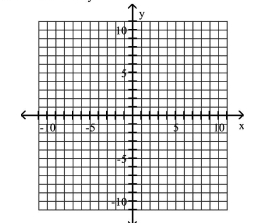
A) foci at and

B) foci at and
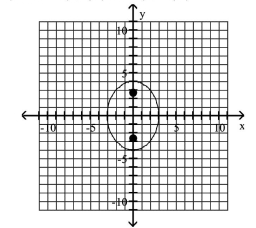
C) foci at and
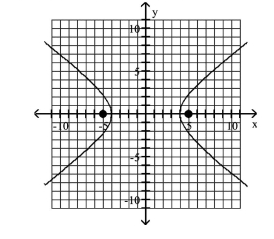
D) foci at and


A) foci at and

B) foci at and

C) foci at and

D) foci at and


Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
8
Graph the ellipse and locate the foci.
Round to the nearest tenth if necessary.

A) foci and
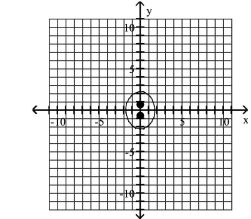
B) foci and
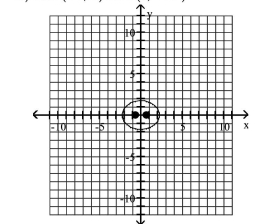
C) foci and
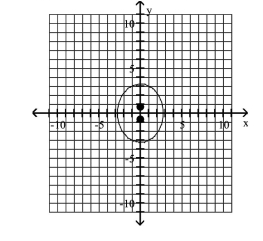
D) foci and

Round to the nearest tenth if necessary.

A) foci and

B) foci and

C) foci and

D) foci and


Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
9
Find the standard form of the equation of the ellipse satisfying the given conditions.
Major axis vertical with length length of minor axis center
A)
B)
C)
D)
Major axis vertical with length length of minor axis center
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
10
Graph the ellipse and locate the foci.
A) foci at and

B) foci at and
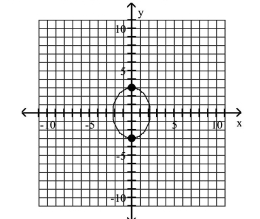
C) foci at and

D) foci at and
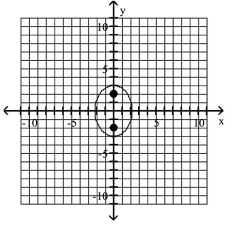

A) foci at and

B) foci at and

C) foci at and

D) foci at and


Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
11
Graph the ellipse and locate the foci.
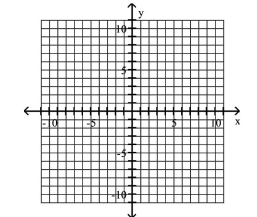
A) foci at and
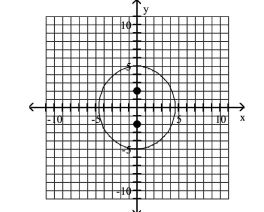
B) foci at and
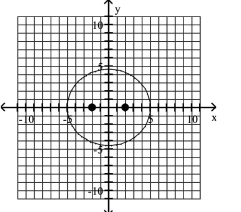
C) foci at and
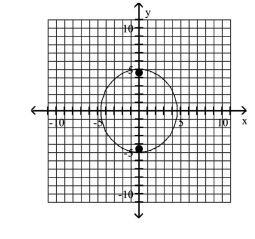
D) foci at and


A) foci at and

B) foci at and

C) foci at and

D) foci at and


Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
12
Find the standard form of the equation of the ellipse satisfying the given conditions.
Foci: -intercepts: and 4
A)
B)
C)
D)
Foci: -intercepts: and 4
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
13
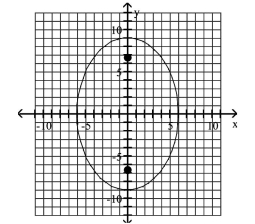
Graph the ellipse and locate the foci.
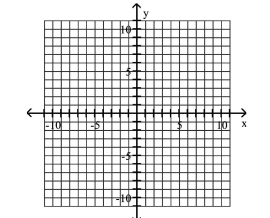
A) foci at and
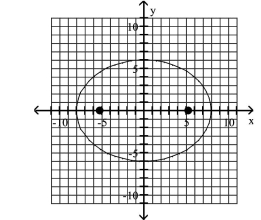
B) foci at and
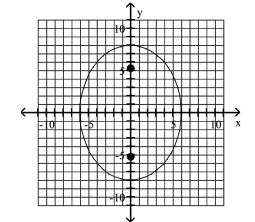
C) foci at and

D) foci at and

A) foci at and

B) foci at and

C) foci at and

D) foci at and


Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
14
Find the standard form of the equation of the ellipse satisfying the given conditions.
Major axis horizontal with length 20 ; length of minor axis center
A)
B)
C)
D)
Major axis horizontal with length 20 ; length of minor axis center
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
15
Find the standard form of the equation of the ellipse satisfying the given conditions.
Endpoints of major axis: and ; endpoints of minor axis: and
A)
B)
C)
D)
Endpoints of major axis: and ; endpoints of minor axis: and
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
16
Graph Ellipses Not Centered at the Origin
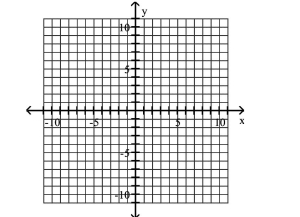
A)
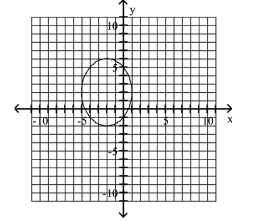
B)
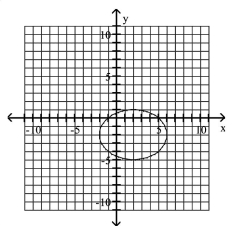
C)
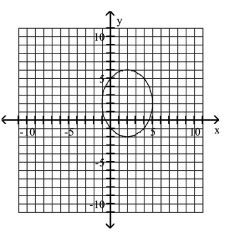
D)
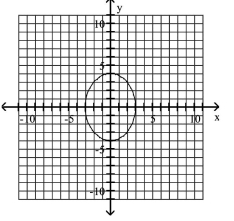

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
17
Graph the ellipse and locate the foci.
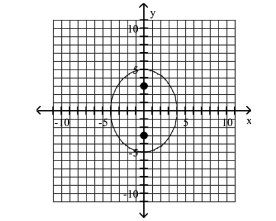
A) foci at and
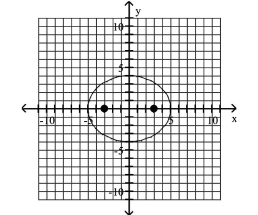
B) foci at and
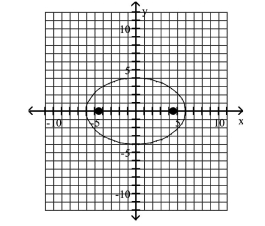
C) foci at and
D) foci at and
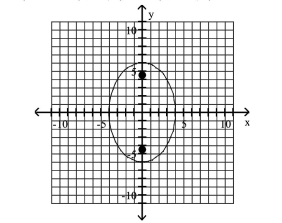

A) foci at and

B) foci at and

C) foci at and

D) foci at and


Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
18
Graph the ellipse and locate the foci.
A) foci at and
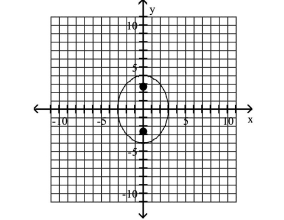
B) foci at and
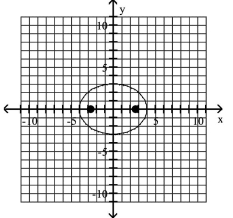
C) foci at and

D) foci at and
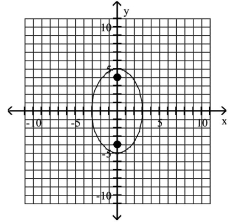

A) foci at and

B) foci at and

C) foci at and

D) foci at and


Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
19
Find the standard form of the equation of the ellipse satisfying the given conditions.
Foci: -intercepts: and 8
A)
B)
C)
D)
Foci: -intercepts: and 8
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
20
Graph Ellipses Not Centered at the Origin
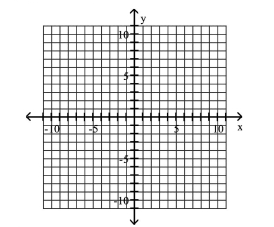
A)
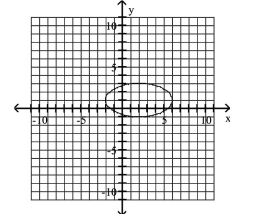
B)

C)
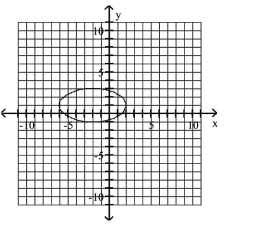
D)
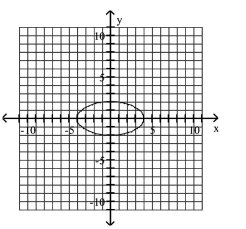

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
21
Convert the equation to the standard form for an ellipse by completing the square on x and y.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
22
The Hyperbola
1 Locate a Hyperbola's Vertices and Foci
A) vertices:
foci:
B) vertices:
foci:
C) vertices:
foci:
D) vertices:
foci:
1 Locate a Hyperbola's Vertices and Foci
A) vertices:
foci:
B) vertices:
foci:
C) vertices:
foci:
D) vertices:
foci:

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
23
The Hyperbola
1 Locate a Hyperbola's Vertices and Foci
A) vertices:
foci:
B) vertices:
foci:
C) vertices:
foci:
D) vertices:
foci:
1 Locate a Hyperbola's Vertices and Foci
A) vertices:
foci:
B) vertices:
foci:
C) vertices:
foci:
D) vertices:
foci:

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
24
Convert the equation to the standard form for an ellipse by completing the square on x and y.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
25
Graph Ellipses Not Centered at the Origin
A) foci at and
B) foci at and
C) foci at and
D) foci at and
A) foci at and
B) foci at and
C) foci at and
D) foci at and

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
26
Additional Concepts
A)
B)
C)
D)

A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
27
Graph Ellipses Not Centered at the Origin
A) foci at and
B) foci at and
C) foci at and
D) foci at and
A) foci at and
B) foci at and
C) foci at and
D) foci at and

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
28
Additional Concepts
A)
B)
C)
D)

A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
29
Graph Ellipses Not Centered at the Origin
A) foci at and
B) foci at and
C) foci at and
D) foci at and
A) foci at and
B) foci at and
C) foci at and
D) foci at and

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
30
The Hyperbola
1 Locate a Hyperbola's Vertices and Foci
A) vertices:
foci:
B) vertices:
foci:
C) vertices:
foci:
D) vertices:
foci:
1 Locate a Hyperbola's Vertices and Foci
A) vertices:
foci:
B) vertices:
foci:
C) vertices:
foci:
D) vertices:
foci:

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
31
Graph Ellipses Not Centered at the Origin
A)
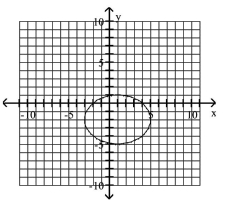
B)
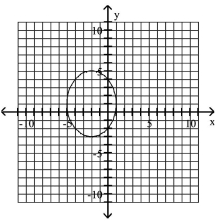
C)
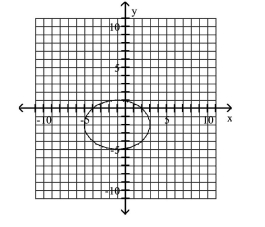
D)


A)

B)

C)

D)


Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
32
Graph the semi-ellipse.
A)
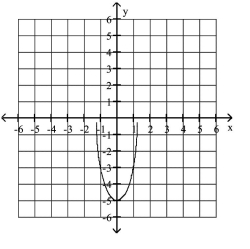
B)
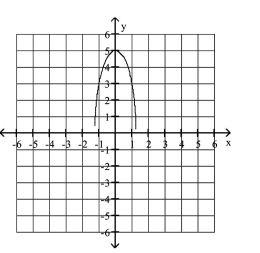 C)
C)

D)
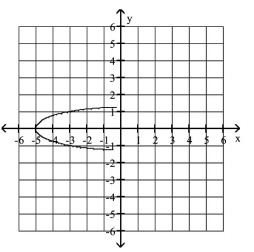

A)

B)
 C)
C)
D)


Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
33
Solve Applied Problems Involving Ellipses
The arch beneath a bridge is semi-elliptical, a one-way roadway passes under the ar
A) Truck 2 can pass under the bridge, but Truck 1 cannot.
B) Both Truck 1 and Truck 2 can pass under the bridge.
C) Neither Truck 1 nor Truck 2 can pass under the bridge.
D) Truck 1 can pass under the bridge, but Truck 2 cannot.
The arch beneath a bridge is semi-elliptical, a one-way roadway passes under the ar
A) Truck 2 can pass under the bridge, but Truck 1 cannot.
B) Both Truck 1 and Truck 2 can pass under the bridge.
C) Neither Truck 1 nor Truck 2 can pass under the bridge.
D) Truck 1 can pass under the bridge, but Truck 2 cannot.

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
34
Graph Ellipses Not Centered at the Origin
A) foci at and
B) foci at and
C) foci at and
D) foci at and
A) foci at and
B) foci at and
C) foci at and
D) foci at and

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
35
The Hyperbola
1 Locate a Hyperbola's Vertices and Foci
A) vertices:
B) vertices:
foci:
foci:
C) vertices:
D) vertices:
foci:
foci:
1 Locate a Hyperbola's Vertices and Foci
A) vertices:
B) vertices:
foci:
foci:
C) vertices:
D) vertices:
foci:
foci:

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
36
The Hyperbola
1 Locate a Hyperbola's Vertices and Foci
A) vertices:
foci:
B) vertices:
foci:
C) vertices:
foci:
D) vertices:
foci:
1 Locate a Hyperbola's Vertices and Foci
A) vertices:
foci:
B) vertices:
foci:
C) vertices:
foci:
D) vertices:
foci:

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
37
Solve Applied Problems Involving Ellipses
The arch beneath a bridge is semi-elliptical, a one-way roadway passes under the ar
A) Truck 1 can pass under the bridge, but Truck 2 cannot.
B) Both Truck 1 and Truck 2 can pass under the bridge.
C) Neither Truck 1 nor Truck 2 can pass under the bridge.
D) Truck 2 can pass under the bridge, but Truck 1 cannot.
The arch beneath a bridge is semi-elliptical, a one-way roadway passes under the ar
A) Truck 1 can pass under the bridge, but Truck 2 cannot.
B) Both Truck 1 and Truck 2 can pass under the bridge.
C) Neither Truck 1 nor Truck 2 can pass under the bridge.
D) Truck 2 can pass under the bridge, but Truck 1 cannot.

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
38
Additional Concepts
A)
B)
C)
D)

A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
39
Solve Applied Problems Involving Ellipses
The arch beneath a bridge is semi-elliptical, a one-way roadway passes under the ar
A) Both Truck 1 and Truck 2 can pass under the bridge.
B) Neither Truck 1 nor Truck 2 can pass under the bridge.
C) Truck 1 can pass under the bridge, but Truck 2 cannot.
D) Truck 2 can pass under the bridge, but Truck 1 cannot.
The arch beneath a bridge is semi-elliptical, a one-way roadway passes under the ar
A) Both Truck 1 and Truck 2 can pass under the bridge.
B) Neither Truck 1 nor Truck 2 can pass under the bridge.
C) Truck 1 can pass under the bridge, but Truck 2 cannot.
D) Truck 2 can pass under the bridge, but Truck 1 cannot.

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
40
Graph Ellipses Not Centered at the Origin
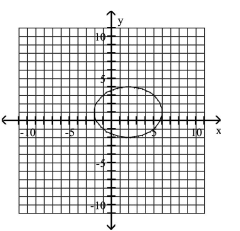
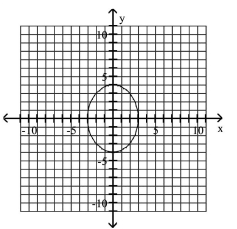
A)

B)
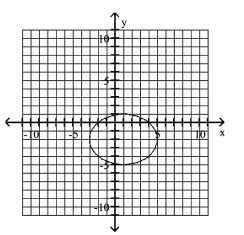
C)
D)
 Find the foci of the ellipse whose equation is given.
Find the foci of the ellipse whose equation is given.

A)

B)

C)

D)
 Find the foci of the ellipse whose equation is given.
Find the foci of the ellipse whose equation is given.
Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
41
Find the standard form of the equation of the hyperbola.
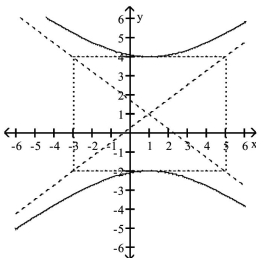
A)
B)
C)
D)

A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
42
Graph Hyperbolas Not Centered at the Origin
A) Center: ; Vertices: and ; Foci: and
B) Center: ; Vertices: and ; Foci: and
C) Center: ; Vertices: and ; Foci: and
D) Center: ; Vertices: and ; Foci: and
A) Center: ; Vertices: and ; Foci: and
B) Center: ; Vertices: and ; Foci: and
C) Center: ; Vertices: and ; Foci: and
D) Center: ; Vertices: and ; Foci: and

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
43
Write Equations of Hyperbolas in Standard Form
Endpoints of transverse axis: ; foci:
A)
B)
C)
D)
Endpoints of transverse axis: ; foci:
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
44
Find the standard form of the equation of the hyperbola.
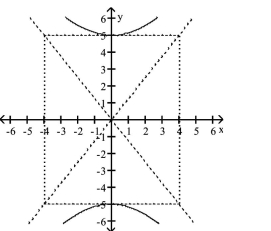
A)
B)
C)
D)

A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
45
Convert the equation to the standard form for a hyperbola by completing the square on x and y.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
46
Write Equations of Hyperbolas in Standard Form
Center: ; Focus: ; Vertex:
A)
B)
C)
D)
Center: ; Focus: ; Vertex:
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
47
Match the equation to the graph.
A)
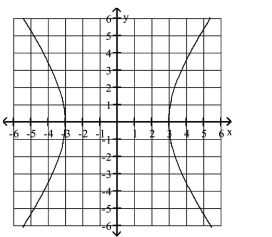
B)
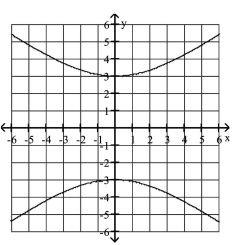
C)
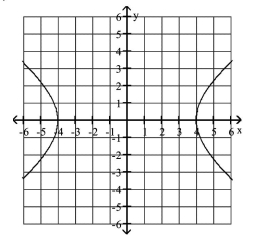
D)
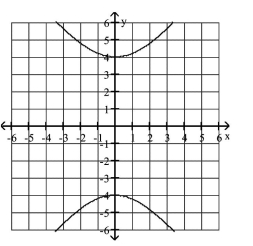
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
48
Graph Hyperbolas Centered at the Origin
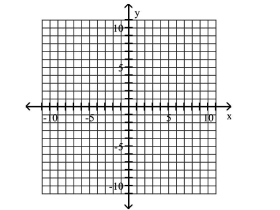
A) Asymptotes:
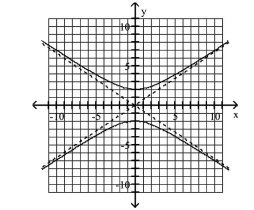
B) Asymptotes:

C) Asymptotes:

D) Asymptotes:


A) Asymptotes:

B) Asymptotes:

C) Asymptotes:

D) Asymptotes:


Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
49
Convert the equation to the standard form for a hyperbola by completing the square on x and y.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
50
Write Equations of Hyperbolas in Standard Form
Foci: ; vertices:
A)
B)
C)
D)
Foci: ; vertices:
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
51
Graph Hyperbolas Centered at the Origin
A) Asymptotes:
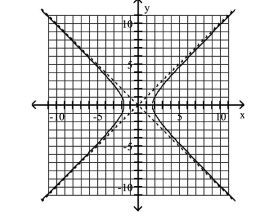
B) Asymptotes:
C) Asymptotes:
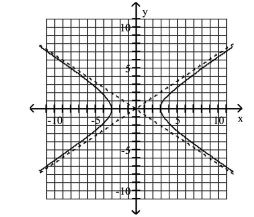
D) Asymptotes:

A) Asymptotes:

B) Asymptotes:
C) Asymptotes:

D) Asymptotes:


Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
52
Write Equations of Hyperbolas in Standard Form
Endpoints of transverse axis: ; asymptote:
A)
B)
C)
D)
Endpoints of transverse axis: ; asymptote:
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
53
Find the standard form of the equation of the hyperbola.
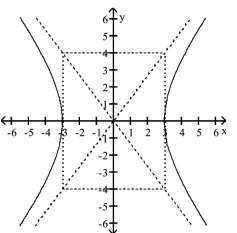
A)
B)
C)
D)

A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
54
Match the equation to the graph.
A)
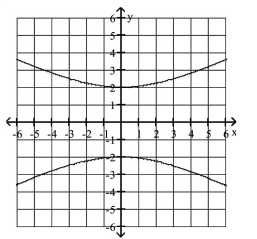
B)
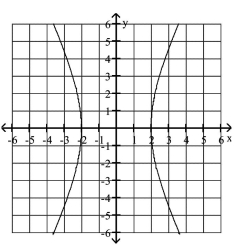
C)
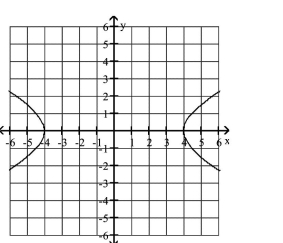
D)

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
55
Convert the equation to the standard form for a hyperbola by completing the square on x and y.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
56
Convert the equation to the standard form for a hyperbola by completing the square on x and y.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
57
Graph Hyperbolas Centered at the Origin
A) Asymptotes:
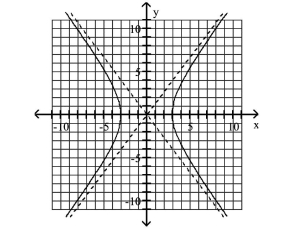
B) Asymptotes:
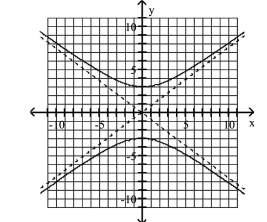
C) Asymptotes:
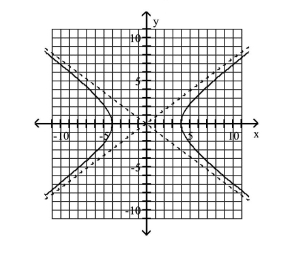
D) Asymptotes:
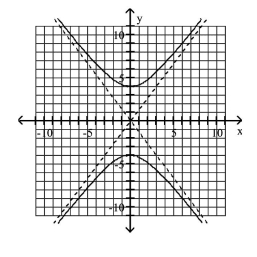

A) Asymptotes:

B) Asymptotes:

C) Asymptotes:

D) Asymptotes:


Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
58
Graph Hyperbolas Centered at the Origin
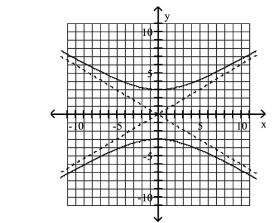
A) Asymptotes:

B) Asymptotes:
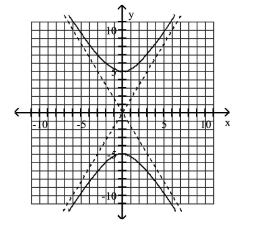
C) Asymptotes:
11ecb96a_d1c1_703f_9fac_351916337d59_TB7044_00
D) Asymptotes:

A) Asymptotes:

B) Asymptotes:

C) Asymptotes:
11ecb96a_d1c1_703f_9fac_351916337d59_TB7044_00
D) Asymptotes:


Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
59
Write Equations of Hyperbolas in Standard Form
Foci: ; vertices:
A)
B)
C)
D)
Foci: ; vertices:
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
60
Graph Hyperbolas Centered at the Origin
A) Asymptotes:
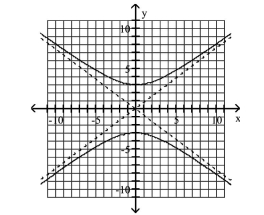
B) Asymptotes:
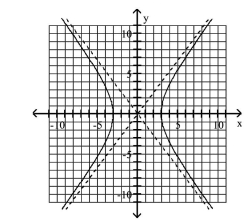
C) Asymptotes:
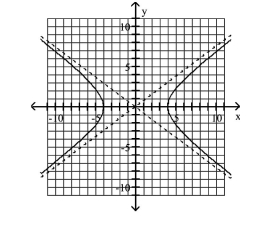
D) Asymptotes:


A) Asymptotes:

B) Asymptotes:

C) Asymptotes:

D) Asymptotes:


Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
61
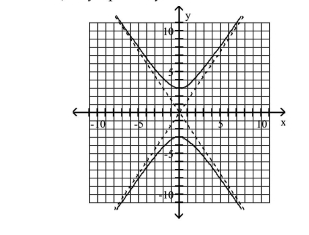
The Parabola
1 Graph Parabolas with Vertices at the Origin
A) focus:
directrix:
B) focus:
directrix:
C) focus:
directrix:
D) focus:
directrix:
1 Graph Parabolas with Vertices at the Origin
A) focus:
directrix:
B) focus:
directrix:
C) focus:
directrix:
D) focus:
directrix:

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
62
The Parabola
1 Graph Parabolas with Vertices at the Origin
A) focus:
directrix:
B) focus:
directrix:
C) focus:
directrix:
D) focus:
directrix:
1 Graph Parabolas with Vertices at the Origin
A) focus:
directrix:
B) focus:
directrix:
C) focus:
directrix:
D) focus:
directrix:

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
63
Use the center, vertices, and asymptotes to graph the hyperbola.
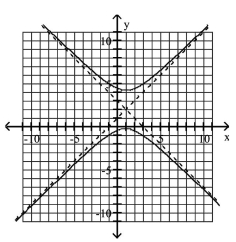
A)

B)
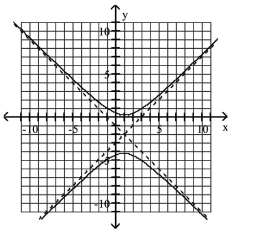
C)
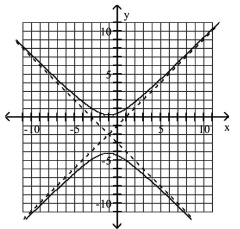
D)

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
64
Use the center, vertices, and asymptotes to graph the hyperbola.
A)
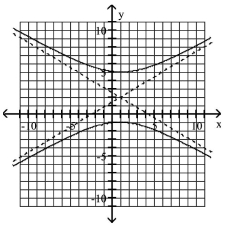
B)
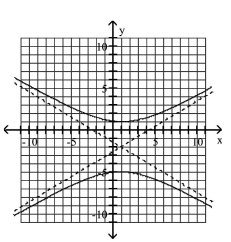
C)
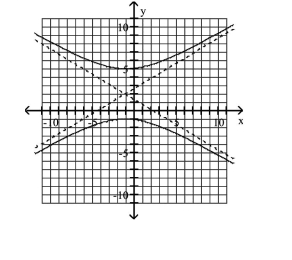
D)
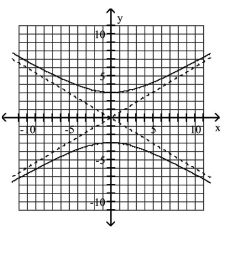

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
65
Additional Concepts
![<strong>Additional Concepts \frac { y ^ { 2 } } { 9 } - \frac { x ^ { 2 } } { 36 } = 1 </strong> A) Domain: ( - \infty , \infty ) Range: ( - \infty , - 3 ] or [ 3 , \infty ) B) Domain: ( - \infty , \infty ) Range: ( - \infty , - 3 ] and [ 3 , \infty ) C) Domain: ( - \infty , - 3 ] or [ 3 , \infty ) Range: ( - \infty , \infty ) D) Domain: ( - \infty , - 3 ] and [ 3 , \infty ) Range: ( - \infty , \infty )](https://storage.examlex.com/TB7044/11ecb96c_a962_9769_9fac_a7cca5a14d51_TB7044_00.jpg)
A) Domain:
Range: or
B) Domain:
Range: and
C) Domain: or
Range:
D) Domain: and
Range:
![<strong>Additional Concepts \frac { y ^ { 2 } } { 9 } - \frac { x ^ { 2 } } { 36 } = 1 </strong> A) Domain: ( - \infty , \infty ) Range: ( - \infty , - 3 ] or [ 3 , \infty ) B) Domain: ( - \infty , \infty ) Range: ( - \infty , - 3 ] and [ 3 , \infty ) C) Domain: ( - \infty , - 3 ] or [ 3 , \infty ) Range: ( - \infty , \infty ) D) Domain: ( - \infty , - 3 ] and [ 3 , \infty ) Range: ( - \infty , \infty )](https://storage.examlex.com/TB7044/11ecb96c_a962_9769_9fac_a7cca5a14d51_TB7044_00.jpg)
A) Domain:
Range: or
B) Domain:
Range: and
C) Domain: or
Range:
D) Domain: and
Range:

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
66
Solve Applied Problems Involving Hyperbolas
Two recording devices are set 3200 feet apart, with the device at point A to the west of the device at point B. At a point on a line between the devices, 400 feet from point B, a small amount of explosive is detonated. The recording devices record the time the sound reaches each one. How far directly north of site B should a second explosion be done so that the measured time difference recorded by the devices is the same as that for the first detonation?
A) feet
B) feet
C) feet
D) feet
Two recording devices are set 3200 feet apart, with the device at point A to the west of the device at point B. At a point on a line between the devices, 400 feet from point B, a small amount of explosive is detonated. The recording devices record the time the sound reaches each one. How far directly north of site B should a second explosion be done so that the measured time difference recorded by the devices is the same as that for the first detonation?
A) feet
B) feet
C) feet
D) feet

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
67
The Parabola
1 Graph Parabolas with Vertices at the Origin
A) focus:
directrix:
B) focus:
directrix:
C) focus:
directrix:
D) focus:
directrix:
1 Graph Parabolas with Vertices at the Origin
A) focus:
directrix:
B) focus:
directrix:
C) focus:
directrix:
D) focus:
directrix:

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
68
Use the center, vertices, and asymptotes to graph the hyperbola.
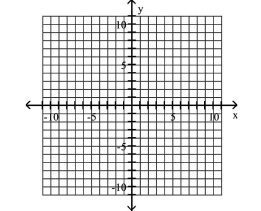
A)
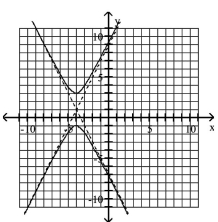
B)
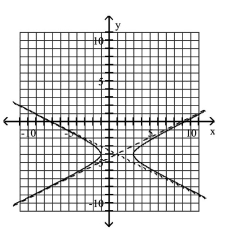
C)
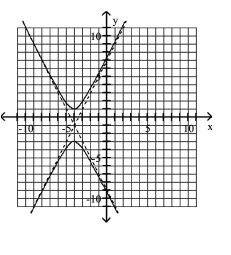
D)


A)

B)

C)

D)


Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
69
Graph Hyperbolas Not Centered at the Origin
A) Center: ; Vertices: and ; Foci: and
B) Center: ; Vertices: and ; Foci: and
C) Center: ; Vertices: and ; Foci: and
D) Center: ; Vertices: and ; Foci: and
A) Center: ; Vertices: and ; Foci: and
B) Center: ; Vertices: and ; Foci: and
C) Center: ; Vertices: and ; Foci: and
D) Center: ; Vertices: and ; Foci: and

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
70
Find the solution set for the system by graphing both of the system's equations in the same rectangular coordinate system and finding points of intersection.
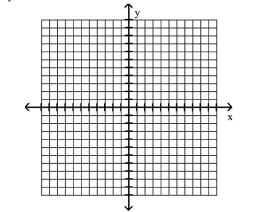
A)
B)
C)
D)

A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
71
The Parabola
1 Graph Parabolas with Vertices at the Origin
A) focus:
directrix:
B) focus:
directrix:
C) focus:
directrix:
D) focus:
directrix:
1 Graph Parabolas with Vertices at the Origin
A) focus:
directrix:
B) focus:
directrix:
C) focus:
directrix:
D) focus:
directrix:

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
72
Solve Applied Problems Involving Hyperbolas
Two LORAN stations are positioned 208 miles apart along a straight shore. A ship records a time difference of seconds between the LORAN signals. (The radio signals travel at 186,000 miles per second.) Where will the ship reach shore if it were to follow the hyperbola corresponding to this time difference? If the ship is 150 miles offshore, what is the position of the ship?
A) 14 miles from the master station,
B) 90 miles from the master station,
C) 14 miles from the master station,
D) 90 miles from the master station,
Two LORAN stations are positioned 208 miles apart along a straight shore. A ship records a time difference of seconds between the LORAN signals. (The radio signals travel at 186,000 miles per second.) Where will the ship reach shore if it were to follow the hyperbola corresponding to this time difference? If the ship is 150 miles offshore, what is the position of the ship?
A) 14 miles from the master station,
B) 90 miles from the master station,
C) 14 miles from the master station,
D) 90 miles from the master station,

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
73
Use the center, vertices, and asymptotes to graph the hyperbola.
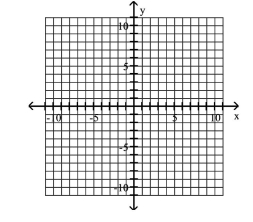
A)

B)
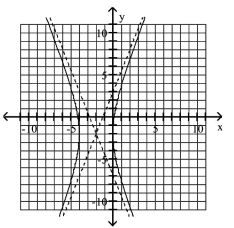
C)
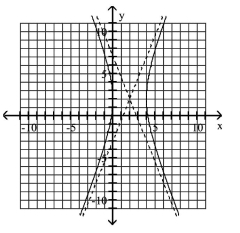
D)

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
74
Find the solution set for the system by graphing both of the system's equations in the same rectangular coordinate system and finding points of intersection.
A)
B)
C)
D)

A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
75
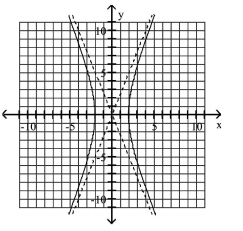
Use the center, vertices, and asymptotes to graph the hyperbola.
A)

B)
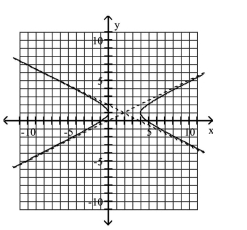
C)

D)
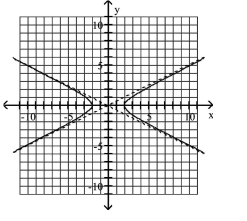

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
76
Graph Hyperbolas Not Centered at the Origin
A) Center: ; Vertices: and ; Foci: and
B) Center: ; Vertices: and ; Foci: and
C) Center: ; Vertices: and ; Foci: and
D) Center: ; Vertices: and ; Foci: and
A) Center: ; Vertices: and ; Foci: and
B) Center: ; Vertices: and ; Foci: and
C) Center: ; Vertices: and ; Foci: and
D) Center: ; Vertices: and ; Foci: and

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
77
Additional Concepts
![<strong>Additional Concepts \frac { x ^ { 2 } } { 9 } - \frac { y ^ { 2 } } { 25 } = 1 </strong> A) Domain: ( - \infty , - 3 ] or [ 3 , \infty ) Range: ( - \infty , \infty ) B) Domain: ( - \infty , \infty ) Range: ( - \infty , - 3 ) or ( 3 , \infty ) C) Domain: ( - \infty , - 3 ] and [ 3 , \infty ) Range: ( - \infty , \infty ) D) Domain: ( - \infty , \infty ) Range: ( - \infty , \infty )](https://storage.examlex.com/TB7044/11ecb96c_7c18_cf97_9fac_a14156e934ab_TB7044_00.jpg)
A) Domain: or
Range:
B) Domain:
Range: or
C) Domain: and
Range:
D) Domain:
Range:
![<strong>Additional Concepts \frac { x ^ { 2 } } { 9 } - \frac { y ^ { 2 } } { 25 } = 1 </strong> A) Domain: ( - \infty , - 3 ] or [ 3 , \infty ) Range: ( - \infty , \infty ) B) Domain: ( - \infty , \infty ) Range: ( - \infty , - 3 ) or ( 3 , \infty ) C) Domain: ( - \infty , - 3 ] and [ 3 , \infty ) Range: ( - \infty , \infty ) D) Domain: ( - \infty , \infty ) Range: ( - \infty , \infty )](https://storage.examlex.com/TB7044/11ecb96c_7c18_cf97_9fac_a14156e934ab_TB7044_00.jpg)
A) Domain: or
Range:
B) Domain:
Range: or
C) Domain: and
Range:
D) Domain:
Range:

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
78
Graph Hyperbolas Not Centered at the Origin
A) Center: ; Vertices: and ; Foci: and
B) Center: ; Vertices: and ; Foci: and
C) Center: ; Vertices: and ; Foci: and
D) Center: ; Vertices: and ; Foci: and
A) Center: ; Vertices: and ; Foci: and
B) Center: ; Vertices: and ; Foci: and
C) Center: ; Vertices: and ; Foci: and
D) Center: ; Vertices: and ; Foci: and

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
79
Additional Concepts
![<strong>Additional Concepts \frac { x ^ { 2 } } { 16 } + \frac { y ^ { 2 } } { 9 } = 1 </strong> A) Domain: [ - 4,4 ] ^ { \downarrow } Range: [ - 3,3 ] B) Domain: [ - 3,3 ] Range: [ - 4,4 ] C) Domain: ( - 4,4 ) Range: ( - 3,3 ) D) Domain: [ - 4,4 ] Range: ( - \infty , \infty )](https://storage.examlex.com/TB7044/11ecb96c_8f75_04a8_9fac_6d47a4527147_TB7044_00.jpg)
A) Domain:
Range:
B) Domain:
Range:
C) Domain:
Range:
D) Domain:
Range:
![<strong>Additional Concepts \frac { x ^ { 2 } } { 16 } + \frac { y ^ { 2 } } { 9 } = 1 </strong> A) Domain: [ - 4,4 ] ^ { \downarrow } Range: [ - 3,3 ] B) Domain: [ - 3,3 ] Range: [ - 4,4 ] C) Domain: ( - 4,4 ) Range: ( - 3,3 ) D) Domain: [ - 4,4 ] Range: ( - \infty , \infty )](https://storage.examlex.com/TB7044/11ecb96c_8f75_04a8_9fac_6d47a4527147_TB7044_00.jpg)
A) Domain:
Range:
B) Domain:
Range:
C) Domain:
Range:
D) Domain:
Range:

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck
80
Solve Applied Problems Involving Hyperbolas
A satellite following the hyperbolic path shown in the picture turns rapidly at and then moves closer and closer to the line as it gets farther from the tracking station at the origin. Find the equation that describes the path of the satellite if the center of the hyperbola is at .
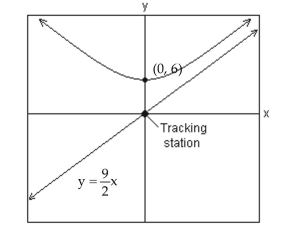
A)
B)
C)
D)
A satellite following the hyperbolic path shown in the picture turns rapidly at and then moves closer and closer to the line as it gets farther from the tracking station at the origin. Find the equation that describes the path of the satellite if the center of the hyperbola is at .

A)
B)
C)
D)

Unlock Deck
Unlock for access to all 228 flashcards in this deck.
Unlock Deck
k this deck


