Deck 8: Systems of Equations
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/118
Play
Full screen (f)
Deck 8: Systems of Equations
1
Solve graphically.
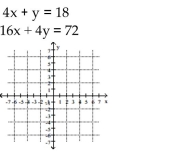
A)Infinite number of solutions
B)(5, -2)
C)No solution
D)(0, 18)

A)Infinite number of solutions
B)(5, -2)
C)No solution
D)(0, 18)
A
2
Solve and graph.
x + y = -14 2x - 2y = -14
A)Inconsistent and independent
B)Consistent and dependent
C)Inconsistent and dependent
D)Consistent and independent
x + y = -14 2x - 2y = -14
A)Inconsistent and independent
B)Consistent and dependent
C)Inconsistent and dependent
D)Consistent and independent
D
3
Classify the system as consistent or inconsistent, and dependent or independent.
x + y = 11 y = 2x + 5
A)(3, 8)
B)(2, 9)
C)(9, 2)
D)(1, 11)
x + y = 11 y = 2x + 5
A)(3, 8)
B)(2, 9)
C)(9, 2)
D)(1, 11)
B
4
Classify the system as consistent or inconsistent, and dependent or independent.
x - 3y = 6 3y + 1 = x
A)Inconsistent and independent
B)Consistent and dependent
C)Inconsistent and dependent
D)Consistent and independent
x - 3y = 6 3y + 1 = x
A)Inconsistent and independent
B)Consistent and dependent
C)Inconsistent and dependent
D)Consistent and independent

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
5
Solve graphically.
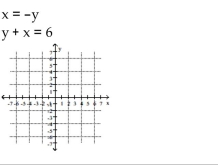
A)No solution
B)(1, 5)
C)Infinite number of solutions
D)(1, 1)

A)No solution
B)(1, 5)
C)Infinite number of solutions
D)(1, 1)

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
6
Solve and graph.
4x - 5y = -4 16x - 20y = -16
A)Inconsistent and independent
B)Consistent and dependent
C)Consistent and independent
D)Inconsistent and dependent
4x - 5y = -4 16x - 20y = -16
A)Inconsistent and independent
B)Consistent and dependent
C)Consistent and independent
D)Inconsistent and dependent

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
7
Solve graphically.
3x + y = -6 2x + 4y = 6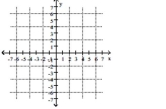
A)(-3, 3)
B)(-1, -3)
C)(-3, -4)
D)(3, 3)
3x + y = -6 2x + 4y = 6

A)(-3, 3)
B)(-1, -3)
C)(-3, -4)
D)(3, 3)

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
8
Solve and graph.
4x + 2y = 8 2x - y = 4
A)Inconsistent and dependent
B)Consistent and independent
C)Consistent and dependent
D)Inconsistent and independent
4x + 2y = 8 2x - y = 4
A)Inconsistent and dependent
B)Consistent and independent
C)Consistent and dependent
D)Inconsistent and independent

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
9
Solve graphically.
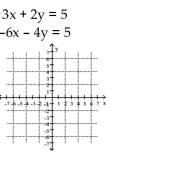
A)(-1.5, -1)
B)(1.5, -1)
C)No solution
D)(1, 1)
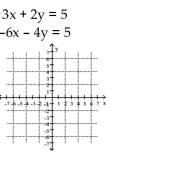
A)(-1.5, -1)
B)(1.5, -1)
C)No solution
D)(1, 1)

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
10
Solve and graph.
x + 4y = 22 2x + 8y = 44
A)Inconsistent and dependent
B)Consistent and independent
C)Consistent and dependent
D)Inconsistent and independent
x + 4y = 22 2x + 8y = 44
A)Inconsistent and dependent
B)Consistent and independent
C)Consistent and dependent
D)Inconsistent and independent

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
11
Solve and graph.
3x = y + 3 6x - 2y = 3
A)Inconsistent and dependent
B)Consistent and dependent
C)Inconsistent and independent
D)Consistent and independent
3x = y + 3 6x - 2y = 3
A)Inconsistent and dependent
B)Consistent and dependent
C)Inconsistent and independent
D)Consistent and independent

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
12
Classify the system as consistent or inconsistent, and dependent or independent.
y = 3x + 4 2x + y = 19
A)(13, -7)
B)(13, 3)
C)(3, 13)
D)(2, 10)
y = 3x + 4 2x + y = 19
A)(13, -7)
B)(13, 3)
C)(3, 13)
D)(2, 10)

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
13
Solve graphically.
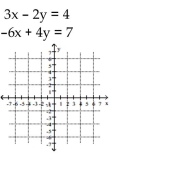
A)(2, 1)
B)(1, 2)
C)No solution
D)Infinite number of solutions

A)(2, 1)
B)(1, 2)
C)No solution
D)Infinite number of solutions

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
14
Solve and graph.

A)Infinitely many solutions
B)No solution
C)(5, 3)
D)(3, 5)

A)Infinitely many solutions
B)No solution
C)(5, 3)
D)(3, 5)

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
15
Solve graphically.
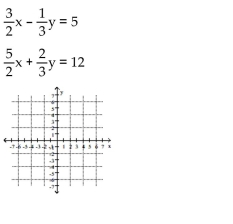
A)(3, 4)
B)(3, 1)
C)(4, 3)
D)(4, -3)
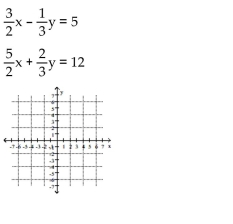
A)(3, 4)
B)(3, 1)
C)(4, 3)
D)(4, -3)

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
16
Solve graphically.
-2x + 4y = 2 3x + 4y = 27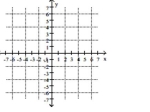
A)No solution
B)(5, 3)
C)(3, 5)
D)(-2, 33)
-2x + 4y = 2 3x + 4y = 27

A)No solution
B)(5, 3)
C)(3, 5)
D)(-2, 33)

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
17
Solve and graph.
x - 6 = y y + 9 = x
A)Inconsistent and dependent
B)Inconsistent and independent
C)Consistent and dependent
D)Consistent and independent
x - 6 = y y + 9 = x
A)Inconsistent and dependent
B)Inconsistent and independent
C)Consistent and dependent
D)Consistent and independent

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
18
Solve and graph.
x + y = 0 x - y = 18
A)Inconsistent and dependent
B)Inconsistent and independent
C)Consistent and independent
D)Consistent and dependent
x + y = 0 x - y = 18
A)Inconsistent and dependent
B)Inconsistent and independent
C)Consistent and independent
D)Consistent and dependent

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
19
Solve and graph.
5x - 25y = 20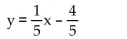
A)Consistent and independent
B)Consistent and dependent
C)Inconsistent and independent
D)Inconsistent and dependent
5x - 25y = 20
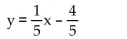
A)Consistent and independent
B)Consistent and dependent
C)Inconsistent and independent
D)Inconsistent and dependent

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
20
Solve and graph.
x + 5y = 12 3x - 4y = -2
A)Consistent and independent
B)Consistent and dependent
C)Inconsistent and dependent
D)Inconsistent and independent
x + 5y = 12 3x - 4y = -2
A)Consistent and independent
B)Consistent and dependent
C)Inconsistent and dependent
D)Inconsistent and independent

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
21
Solve using the substitution method.
x + 2y = -3 8x + 3y = -24
A)(-2, -3)
B)(3, -1)
C)(-3, 0)
D)No solution
x + 2y = -3 8x + 3y = -24
A)(-2, -3)
B)(3, -1)
C)(-3, 0)
D)No solution

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
22
Solve using the substitution method.
x + 7y = 47 8x + 6y = 26
A)(-3, 8)
B)(-2, 7)
C)(2, 8)
D)No solution
x + 7y = 47 8x + 6y = 26
A)(-3, 8)
B)(-2, 7)
C)(2, 8)
D)No solution

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
23
Solve by the substitution method.
- x + 4y = + 2 -3x - 4y = -26
A)(-2, 6)
B)No solution
C)(7, 1)
D)(6, 2)
- x + 4y = + 2 -3x - 4y = -26
A)(-2, 6)
B)No solution
C)(7, 1)
D)(6, 2)

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
24
Solve by the substitution method.
The perimeter of a triangle is 52 cm. The triangle is isosceles now, but if its base were lengthened by 3 cm and each leg were shortened by 2 cm, it would be equilateral. Find the base of the original triangle.
A)17 cm
B)13 cm
C)19 cm
D)14 cm
The perimeter of a triangle is 52 cm. The triangle is isosceles now, but if its base were lengthened by 3 cm and each leg were shortened by 2 cm, it would be equilateral. Find the base of the original triangle.
A)17 cm
B)13 cm
C)19 cm
D)14 cm

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
25
Solve the problem.
The perimeter of a rectangle is 54 m. If the width were doubled and the length were increased by 15 m, the perimeter would be 98 m. What are the length and width of the rectangle?
A)width 8 m, length 13 m
B)width 7 m, length 20 m
C)width 13 m, length 13 m
D)width 20 m, length 7 m
The perimeter of a rectangle is 54 m. If the width were doubled and the length were increased by 15 m, the perimeter would be 98 m. What are the length and width of the rectangle?
A)width 8 m, length 13 m
B)width 7 m, length 20 m
C)width 13 m, length 13 m
D)width 20 m, length 7 m

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
26
Solve the problem.
The sum of two numbers is 39 and their difference is 17. Find the numbers.
A)13 and 30
B)26 and 13
C)28 and 11
D)23 and 16
The sum of two numbers is 39 and their difference is 17. Find the numbers.
A)13 and 30
B)26 and 13
C)28 and 11
D)23 and 16

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
27
Solve using the substitution method.
x + y = -2 x + y = -7
A)No solution
B)(0, -9)
C)(0, 0)
D)(-2, -7)
x + y = -2 x + y = -7
A)No solution
B)(0, -9)
C)(0, 0)
D)(-2, -7)

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
28
Solve by the substitution method.
2 x - y = 9 5x + y = 33
A)(3, 6)
B)(6, 4)
C)(6, 3)
D)No solution
2 x - y = 9 5x + y = 33
A)(3, 6)
B)(6, 4)
C)(6, 3)
D)No solution

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
29
Solve the problem.
The sum of two angles is 211°. One angle is 29° less than twice the other. Find the angles.
A)127° and 84°
B)78° and 127°
C)80° and 131°
D)78° and 133°
The sum of two angles is 211°. One angle is 29° less than twice the other. Find the angles.
A)127° and 84°
B)78° and 127°
C)80° and 131°
D)78° and 133°

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
30
Solve using the substitution method.
x + y = 6 5x + 5y = 30
A)(0, 0)
B)(6, 5)
C)Infinite number of solutions
D)(3, 3)
x + y = 6 5x + 5y = 30
A)(0, 0)
B)(6, 5)
C)Infinite number of solutions
D)(3, 3)

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
31
Solve the problem.
Two angles have a sum of 96°. Their difference is 22°. Find the angles.
A)39° and 61°
B)75° and 21°
C)57° and 39°
D)59° and 37°
Two angles have a sum of 96°. Their difference is 22°. Find the angles.
A)39° and 61°
B)75° and 21°
C)57° and 39°
D)59° and 37°

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
32
Solve using the substitution method.
7x + 6y = 55 -5x - 4y = -39
A)(6, 2)
B)(7, 1)
C)(7, 2)
D)No solution
7x + 6y = 55 -5x - 4y = -39
A)(6, 2)
B)(7, 1)
C)(7, 2)
D)No solution

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
33
Solve using the substitution method.
x - 4y = 20 4x - 3y = 15
A)(1, -6)
B)(5, 0)
C)(0, -5)
D)No solution
x - 4y = 20 4x - 3y = 15
A)(1, -6)
B)(5, 0)
C)(0, -5)
D)No solution

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
34
Solve using the substitution method.
7x - 5y = 20 -3x + 3y = -12
A)(0, -4)
B)(0, -3)
C)(-1, -3)
D)No solution
7x - 5y = 20 -3x + 3y = -12
A)(0, -4)
B)(0, -3)
C)(-1, -3)
D)No solution

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
35
Solve using the substitution method.
6x + 30 = 6y 3x - 4y = -16
A)(-4, 1)
B)(-4, 2)
C)(-5, 2)
D)No solution
6x + 30 = 6y 3x - 4y = -16
A)(-4, 1)
B)(-4, 2)
C)(-5, 2)
D)No solution

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
36
Solve using the substitution method.
x = 4 + -4y x + 4y = 7
A)Infinitely many solutions
B)No solution
C)(- 1, 8)
D)(- 1, - 8)
x = 4 + -4y x + 4y = 7
A)Infinitely many solutions
B)No solution
C)(- 1, 8)
D)(- 1, - 8)

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
37
Solve the problem.
Find two numbers whose sum is 35 and whose difference is 5.
A)31 and 4
B)15 and 20
C)22 and 27
D)13 and 22
Find two numbers whose sum is 35 and whose difference is 5.
A)31 and 4
B)15 and 20
C)22 and 27
D)13 and 22

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
38
Solve the problem.
The perimeter of a rectangle is 44 cm. One side is 10 cm longer than the other side. Find the lengths of the sides.
A)6 cm, 10 cm
B)12 cm, 22 cm
C)9 cm, 19 cm
D)6 cm, 16 cm
The perimeter of a rectangle is 44 cm. One side is 10 cm longer than the other side. Find the lengths of the sides.
A)6 cm, 10 cm
B)12 cm, 22 cm
C)9 cm, 19 cm
D)6 cm, 16 cm

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
39
Solve using the substitution method.
-7x + 8y = 42 2x + 6y = -12
A)(-6, 1)
B)No solution
C)(-6, 0)
D)(-7, 1)
-7x + 8y = 42 2x + 6y = -12
A)(-6, 1)
B)No solution
C)(-6, 0)
D)(-7, 1)

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
40
Solve by the substitution method.
x + y = -13 x - y = -1
A)(-7, -6)
B)(-8, -5)
C)No solution
D)(7, -5)
x + y = -13 x - y = -1
A)(-7, -6)
B)(-8, -5)
C)No solution
D)(7, -5)

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
41
Solve using the elimination method.
x + 8y = 8 6x + 9y = 48
A)(8, 0)
B)(-8, -1)
C)no solution
D)(9, 8)
x + 8y = 8 6x + 9y = 48
A)(8, 0)
B)(-8, -1)
C)no solution
D)(9, 8)

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
42
Solve using the elimination method.



Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
43
Solve using the elimination method.
-4x + 4y = 6 12x - 12y = 18
A)(-24, 24)
B)infinitely many solutions
C)no solution
D)(24, -24)
-4x + 4y = 6 12x - 12y = 18
A)(-24, 24)
B)infinitely many solutions
C)no solution
D)(24, -24)

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
44
Solve the problem using the elimination method.
Two angles are supplementary, and one is 5° more than six times the other. Find the larger angle.
A)155°
B)25°
C)70°
D)110°
Two angles are supplementary, and one is 5° more than six times the other. Find the larger angle.
A)155°
B)25°
C)70°
D)110°

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
45
Solve using the elimination method.
0.8x + 0.3y = 10.7 -0.3x - 0.1y = -3.9
A)(100, 90)
B)(1, 0.9)
C)(10, 9)
D)(9, 10)
0.8x + 0.3y = 10.7 -0.3x - 0.1y = -3.9
A)(100, 90)
B)(1, 0.9)
C)(10, 9)
D)(9, 10)

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
46
Solve using the elimination method.
9x + 7y = 49 -3x - 5y = -35
A)(0, 8)
B)no solution
C)(0, 7)
D)(-1, 8)
9x + 7y = 49 -3x - 5y = -35
A)(0, 8)
B)no solution
C)(0, 7)
D)(-1, 8)

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
47
Solve the problem using the elimination method.
Best Rentals charges a daily fee plus a mileage fee for renting its cars. Barney was charged $81 for 3 days and 300 miles, while Mary was charged $144 for 5 days and 600 miles. What does Best Rental charge per day and
Per mile?
Best Rentals charges a daily fee plus a mileage fee for renting its cars. Barney was charged $81 for 3 days and 300 miles, while Mary was charged $144 for 5 days and 600 miles. What does Best Rental charge per day and
Per mile?


Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
48
Solve the problem using the elimination method.
There were 380 people at a play. The admission price was $2 for adults and $1 for children. The admission receipts were $600. How many adults and how many children attended?
A)150 adults and 230 children
B)80 adults and 300 children
C)160 adults and 220 children
D)220 adults and 160 children
There were 380 people at a play. The admission price was $2 for adults and $1 for children. The admission receipts were $600. How many adults and how many children attended?
A)150 adults and 230 children
B)80 adults and 300 children
C)160 adults and 220 children
D)220 adults and 160 children

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
49
Solve using the elimination method.
-7x + 9y = 28 -3x - 3y = 12
A)no solution
B)(-4, 1)
C)(-5, 1)
D)(-4, 0)
-7x + 9y = 28 -3x - 3y = 12
A)no solution
B)(-4, 1)
C)(-5, 1)
D)(-4, 0)

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
50
Solve using the elimination method.
x + 3y = 13 2x + 3y = 8
A)No solution
B)(5, 5)
C)(-4, -5)
D)(-5, 6)
x + 3y = 13 2x + 3y = 8
A)No solution
B)(5, 5)
C)(-4, -5)
D)(-5, 6)

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
51
Solve using the elimination method.
x - 2y = 8 -7x - 3y = -39
A)(6, -1)
B)no solution
C)(5, 0)
D)(-6, 0)
x - 2y = 8 -7x - 3y = -39
A)(6, -1)
B)no solution
C)(5, 0)
D)(-6, 0)

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
52
Solve the problem using the elimination method.
There were 31,000 people at a ball game in Los Angeles. The day's receipts were $180,000. How many people paid $11 for reserved seats and how many paid $4 for general admission?
A)23,000 paid $11 and 8000 paid $4
B)17,000 paid $11 and 14,000 paid $4
C)8000 paid $11 and 23,000 paid $4
D)14,000 paid $11 and 17,000 paid $4
There were 31,000 people at a ball game in Los Angeles. The day's receipts were $180,000. How many people paid $11 for reserved seats and how many paid $4 for general admission?
A)23,000 paid $11 and 8000 paid $4
B)17,000 paid $11 and 14,000 paid $4
C)8000 paid $11 and 23,000 paid $4
D)14,000 paid $11 and 17,000 paid $4

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
53
Solve using the elimination method.
x + 5y = 25 2x + 6y = 30
A)(-5, 0)
B)no solution
C)(0, 5)
D)(1, 4)
x + 5y = 25 2x + 6y = 30
A)(-5, 0)
B)no solution
C)(0, 5)
D)(1, 4)

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
54
Solve the problem using the elimination method.
In a right triangle, one acute angle is 54° more than twice the other. Find each acute angle.
A)28° and 62°
B)37° and 53°
C)21° and 69°
D)12° and 78°
In a right triangle, one acute angle is 54° more than twice the other. Find each acute angle.
A)28° and 62°
B)37° and 53°
C)21° and 69°
D)12° and 78°

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
55
Solve using the elimination method.



Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
56
Solve the problem using the elimination method.
Two angles are supplementary, and one is 40° more than three times the other. Find the smaller angle.
A)35°
B)145°
C)105°
D)75°
Two angles are supplementary, and one is 40° more than three times the other. Find the smaller angle.
A)35°
B)145°
C)105°
D)75°

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
57
Solve using the elimination method.
2.5x + 0.2y = 10.6 0.5x - 0.4y = 0.8
A)(4.5, 3)
B)(6.5, 3.2)
C)(4, 3)
D)(1.5, 3.2)
2.5x + 0.2y = 10.6 0.5x - 0.4y = 0.8
A)(4.5, 3)
B)(6.5, 3.2)
C)(4, 3)
D)(1.5, 3.2)

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
58
Solve using the elimination method.
0.1x + 0.7y = 1.7 x - 0.3y = -4.9
A)(-4, 3)
B)(3, -4)
C)(-0.4, 0.3)
D)no solution
0.1x + 0.7y = 1.7 x - 0.3y = -4.9
A)(-4, 3)
B)(3, -4)
C)(-0.4, 0.3)
D)no solution

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
59
Solve the problem using the elimination method.
A salesman sold $300 more than the rest of the sales staff. If the sales total for the day was $1050, how much did the rest of the sales staff sell?
A)$375
B)$675
C)$750
D)$525
A salesman sold $300 more than the rest of the sales staff. If the sales total for the day was $1050, how much did the rest of the sales staff sell?
A)$375
B)$675
C)$750
D)$525

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
60
Solve using the elimination method.
-3x - 2y = 4 12x + 8y = -16
A)(-12, -8)
B)infinitely many solutions
C)(-8, -12)
D)no solution
-3x - 2y = 4 12x + 8y = -16
A)(-12, -8)
B)infinitely many solutions
C)(-8, -12)
D)no solution

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
61
Solve the problem.
Walt made an extra $7000 last year from a part-time job. He invested part of the money at 6% and the rest at 8%. He made a total of $480 in interest. How much was invested at 8%?
A)$5000
B)$3500
C)$4000
D)$3000
Walt made an extra $7000 last year from a part-time job. He invested part of the money at 6% and the rest at 8%. He made a total of $480 in interest. How much was invested at 8%?
A)$5000
B)$3500
C)$4000
D)$3000

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
62
Solve the problem.
Ellen wishes to mix candy worth $1.57 per pound with candy worth $3.69 per pound to form 26 pounds of a mixture worth $3.04 per pound. How many pounds of the more expensive candy should she use?
A)8 pounds
B)18 pounds
C)23 pounds
D)10 pounds
Ellen wishes to mix candy worth $1.57 per pound with candy worth $3.69 per pound to form 26 pounds of a mixture worth $3.04 per pound. How many pounds of the more expensive candy should she use?
A)8 pounds
B)18 pounds
C)23 pounds
D)10 pounds

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
63
Solve the problem.
A contractor mixes concrete from bags of pre-mix for small jobs. How many bags with 3% cement should he mix with 8 bags of 11% cement to produce a mix containing 7% cement?
A)21 bags
B)16 bags
C)8 bags
D)10 bags
A contractor mixes concrete from bags of pre-mix for small jobs. How many bags with 3% cement should he mix with 8 bags of 11% cement to produce a mix containing 7% cement?
A)21 bags
B)16 bags
C)8 bags
D)10 bags

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
64
Solve the problem.
Tim and Judy mix two kinds of feed for pedigreed dogs. They wish to make 19 pounds of feed worth $0.76 per pound by mixing one kind worth $0.41 per pound with another worth $0.96 per pound. How many pounds of
The cheaper kind should they use in the mix?
A)17 pounds
B)12 pounds
C)7 pounds
D)9 pounds
Tim and Judy mix two kinds of feed for pedigreed dogs. They wish to make 19 pounds of feed worth $0.76 per pound by mixing one kind worth $0.41 per pound with another worth $0.96 per pound. How many pounds of
The cheaper kind should they use in the mix?
A)17 pounds
B)12 pounds
C)7 pounds
D)9 pounds

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
65
Solve the problem using the elimination method.
A vendor sells hot dogs and bags of potato chips. A customer buys 2 hot dogs and 2 bags of potato chips for $6.50. Another customer buys 5 hot dogs and 3 bags of potato chips for $14.25. Find the cost of each item.
A)$2.25 for a hot dog; $1.00 for a bag of potato chips
B)$2.50 for a hot dog; $1.25 for a bag of potato chips
C)$1.00 for a hot dog; $2.25 for a bag of potato chips
D)$2.25 for a hot dog; $1.25 for a bag of potato chips
A vendor sells hot dogs and bags of potato chips. A customer buys 2 hot dogs and 2 bags of potato chips for $6.50. Another customer buys 5 hot dogs and 3 bags of potato chips for $14.25. Find the cost of each item.
A)$2.25 for a hot dog; $1.00 for a bag of potato chips
B)$2.50 for a hot dog; $1.25 for a bag of potato chips
C)$1.00 for a hot dog; $2.25 for a bag of potato chips
D)$2.25 for a hot dog; $1.25 for a bag of potato chips

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
66
Solve the problem.
Anne and Nancy use a metal alloy that is 17% copper to make jewelry. How many ounces of an alloy that is 11% copper must be mixed with an alloy that is 22% copper to form 55 ounces of the desired alloy?
A)35 ounces
B)27 ounces
C)30 ounces
D)25 ounces
Anne and Nancy use a metal alloy that is 17% copper to make jewelry. How many ounces of an alloy that is 11% copper must be mixed with an alloy that is 22% copper to form 55 ounces of the desired alloy?
A)35 ounces
B)27 ounces
C)30 ounces
D)25 ounces

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
67
Solve the problem using the elimination method.
The sum of two numbers is 8. Three times the larger number plus four times the smaller number is 18. Find the numbers.
A)6 and 2
B)6 and -14
C)14 and -6
D)22 and -14
The sum of two numbers is 8. Three times the larger number plus four times the smaller number is 18. Find the numbers.
A)6 and 2
B)6 and -14
C)14 and -6
D)22 and -14

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
68
Solve the problem.
A sum of money amounting to $4.15 consists of dimes and quarters. If there are 28 coins in all, how many are quarters?
A)21 quarters
B)14 quarters
C)9 quarters
D)19 quarters
A sum of money amounting to $4.15 consists of dimes and quarters. If there are 28 coins in all, how many are quarters?
A)21 quarters
B)14 quarters
C)9 quarters
D)19 quarters

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
69
Solve the problem.
Mrs. Boyd has a desk full of quarters and nickels. If she has a total of 30 coins with a total face value of $4.90, how many of the coins are nickels?
A)22 nickels
B)13 nickels
C)17 nickels
D)15 nickels
Mrs. Boyd has a desk full of quarters and nickels. If she has a total of 30 coins with a total face value of $4.90, how many of the coins are nickels?
A)22 nickels
B)13 nickels
C)17 nickels
D)15 nickels

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
70
Solve the problem.
How many liters of a 10% alcohol solution must be mixed with 90 liters of a 70% solution to get a 60% solution?
A)108 L
B)1.8 L
C)18 L
D)10.8 L
How many liters of a 10% alcohol solution must be mixed with 90 liters of a 70% solution to get a 60% solution?
A)108 L
B)1.8 L
C)18 L
D)10.8 L

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
71
Solve the problem.
There were 520 people at a play. The admission price was $3 for adults and $1 for children. The admission receipts were $1180. How many adults and how many children attended?
A)330 adults and 190 children
B)190 adults and 330 children
C)295 adults and 225 children
D)95 adults and 425 children
There were 520 people at a play. The admission price was $3 for adults and $1 for children. The admission receipts were $1180. How many adults and how many children attended?
A)330 adults and 190 children
B)190 adults and 330 children
C)295 adults and 225 children
D)95 adults and 425 children

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
72
Solve the problem.
A merchant has coffee worth $20 a pound that she wishes to mix with 30 pounds of coffee worth $90 a pound to get a mixture that can be sold for $50 a pound. How many pounds of the $20 coffee should be used?
A)40 lb
B)70 lb
C)35 lb
D)20 lb
A merchant has coffee worth $20 a pound that she wishes to mix with 30 pounds of coffee worth $90 a pound to get a mixture that can be sold for $50 a pound. How many pounds of the $20 coffee should be used?
A)40 lb
B)70 lb
C)35 lb
D)20 lb

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
73
Solve the problem.
A woman made a deposit of $341. If her deposit consisted of 101 bills, some of them one-dollar bills and the rest being five-dollar bills, how many one-dollar bills did she deposit?
A)41 one-dollars
B)36 one-dollars
C)60 one-dollars
D)31 one-dollars
A woman made a deposit of $341. If her deposit consisted of 101 bills, some of them one-dollar bills and the rest being five-dollar bills, how many one-dollar bills did she deposit?
A)41 one-dollars
B)36 one-dollars
C)60 one-dollars
D)31 one-dollars

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
74
Solve the problem using the elimination method.
The sum of two numbers is 33. The larger number minus the smaller number is 9. What are the numbers?
A)21 and 12
B)25 and 8
C)19 and 14
D)14 and 23
The sum of two numbers is 33. The larger number minus the smaller number is 9. What are the numbers?
A)21 and 12
B)25 and 8
C)19 and 14
D)14 and 23

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
75
Solve the problem.
Andy has 17 coins made up of quarters and half dollars, and their total value is $4.75. How many quarters does he have?
A)4 quarters
B)2 quarters
C)15 quarters
D)20 quarters
Andy has 17 coins made up of quarters and half dollars, and their total value is $4.75. How many quarters does he have?
A)4 quarters
B)2 quarters
C)15 quarters
D)20 quarters

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
76
Solve the problem using the elimination method.
The sum of two numbers is 96. The second number is three times as large as the first number. What are the numbers?
A)22 and 74
B)24 and 72
C)21 and 75
D)22 and 66
The sum of two numbers is 96. The second number is three times as large as the first number. What are the numbers?
A)22 and 74
B)24 and 72
C)21 and 75
D)22 and 66

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
77
Solve the problem.
Ron and Kathy are ticket-sellers at their class play, Ron handling student tickets that sell for $2.00 each and Kathy selling adult tickets for $5.50 each. If their total income for 26 tickets was $118.50, how many did Ron sell?
A)9 tickets
B)24 tickets
C)19 tickets
D)7 tickets
Ron and Kathy are ticket-sellers at their class play, Ron handling student tickets that sell for $2.00 each and Kathy selling adult tickets for $5.50 each. If their total income for 26 tickets was $118.50, how many did Ron sell?
A)9 tickets
B)24 tickets
C)19 tickets
D)7 tickets

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
78
Solve the problem using the elimination method.
In a basketball game, Will scored 35 points, consisting only of three-point shots and two-point shots. He made a total of 15 shots. How many shots of each type did he make?
A)two-point shots: 10; three-point shots: 5
B)two-point shots: 11; three-point shots: 4
C)two-point shots: 9; three-point shots: 6
D)two-point shots: 5; three-point shots: 10
In a basketball game, Will scored 35 points, consisting only of three-point shots and two-point shots. He made a total of 15 shots. How many shots of each type did he make?
A)two-point shots: 10; three-point shots: 5
B)two-point shots: 11; three-point shots: 4
C)two-point shots: 9; three-point shots: 6
D)two-point shots: 5; three-point shots: 10

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
79
Solve the problem.
Mardi received an inheritance of $70,000. She invested part at 10% and deposited the remainder in tax-free bonds at 8%. Her total annual income from the investments was $6200. Find the amount invested at 10%.
A)$15,000
B)$30,000
C)$63,800
D)$29,000
Mardi received an inheritance of $70,000. She invested part at 10% and deposited the remainder in tax-free bonds at 8%. Her total annual income from the investments was $6200. Find the amount invested at 10%.
A)$15,000
B)$30,000
C)$63,800
D)$29,000

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck
80
Solve the problem.
In a chemistry class, 9 liters of a 4% silver iodide solution must be mixed with a 10% solution to get a 6% solution. How many liters of the 10% solution are needed?
A)5.5 L
B)4.5 L
C)3.5 L
D)9.0 L
In a chemistry class, 9 liters of a 4% silver iodide solution must be mixed with a 10% solution to get a 6% solution. How many liters of the 10% solution are needed?
A)5.5 L
B)4.5 L
C)3.5 L
D)9.0 L

Unlock Deck
Unlock for access to all 118 flashcards in this deck.
Unlock Deck
k this deck


