Deck 6: Probability in Statistics

Question 1

Would it be "significant" to guess the day of the week of a person's birth.

Answer 1

False

Question 2

Suppose you pay $1.00 to roll a fair die with the understanding that you will get back $3.00 for rolling a 5 or a 2, nothing otherwise. What is your expected value?

A) $1.00
B) $0.00
C) $3.00
D) −$1.00

Answer 2

B

Question 3

The following table is from the Social Security Actuarial Tables. For each age, it gives the probability of death within one year, the number living out of an original 100,000, and the additional life expectancy for a person of that age.
Exact Male Female P(Death Additional P(Death Additional Age.
$\begin{array}{c}\quad\quad\quad\quad\quad\quad\text { Male } \quad\quad\quad\quad\quad\quad\quad\quad\quad\quad\quad\quad\quad\text { Female }\\\begin{array}{|c|c|c|c|c|c|c|}\hline{\text {Exact Age }} & \begin{array}{c}\text { P(Death } \\\text { within one } \\\text { year) }\end{array} & \begin{array}{c}\text { Number of } \\\text { Living }\end{array} & \begin{array}{c}\text { Additional } \\\text { Life } \\\text { Expectancy }\end{array} & \begin{array}{c}\text { P(Death } \\\text { within one } \\\text { year) }\end{array} & \begin{array}{c}\text { Number of } \\\text { Living }\end{array} & \begin{array}{c}\text { Additional } \\\text { Life } \\\text { Expectancy }\end{array} \\\hline 10 & 0.000111 & 99,021 & 65.13 & 0.000105 & 99,217 & 70.22 \\\hline 20 & 0.001287 & 98,451 & 55.46 & 0.000469 & 98,950 & 60.40 \\\hline 30 & 0.001375 & 97,113 & 46.16 & 0.000627 & 98,431 & 50.69 \\\hline 40 & 0.002542 & 95,427 & 36.88 & 0.001498 & 97,513 & 41.11 \\\hline 50 & 0.005696 & 91,853 & 28.09 & 0.003240 & 95,378 & 31.91 \\\hline 60 & 0.012263 & 84,692 & 20.00 & 0.007740 & 90,847 & 23.21 \\\hline 70 & 0.028904 & 70,214 & 12.98 & 0.018938 & 80,583 & 15.45 \\\hline 80 & 0.071687 & 44,272 & 7.43 & 0.049527 & 59,341 & 9.00 \\\hline 90 & 0.188644 & 12,868 & 3.68 & 0.146696 & 24,331 & 4.45 \\\hline\end{array}\end{array}$ To what age may a male of age 70 expect to live on the average?

Answer 3

82.98

Question 4

Joe dealt 20 cards from a standard 52-card deck, and the number of red cards exceeded the number of black cards by 8. He reshuffled the cards and dealt 30 cards. This time, the number of red cards exceeded the number of black cards by 10. Determine which deal is closer to the 50/50 ratio of red/black expected of fairly dealt hands from a fair deck and why.

A) The first series is closer because 1/10 is farther from 1/2 than is 1/8.
B) The series closer to the theoretical 50/50 cannot be determined unless the number of red and black cards for each deal is given.
C) The second series is closer because 20/30 is closer to 1/2 than is 14/20.
D) The first series is closer because the difference between red and black is smaller than the difference in the second series.

Question 5

state whether there is one way for the given observation to occur (outcome) or more than one way for the given observation to occur (in which case it is an event).

-Rolling at least one 6 with two fair dice

A) Outcome
B) Event

Question 6

state whether there is one way for the given observation to occur (outcome) or more than one way for the given observation to occur (in which case it is an event).

-Tossing two tails with three fair coins

A) Outcome
B) Event

Question 7

A study of 600 college students taking Statistics 101 revealed that 54 students received the grade of A. Typically 10% of the class gets an A. The difference between this group of students and the expected value is not significant at the 0.05 level. What does this mean in this case?

A) The probability that the difference occurred due to chance is less than 0.05.
B) The probability of getting an A is 10% and only 9% got an A in this study. The difference is less than 5% so it is not significant.
C) There is not enough information to make any conclusion.
D) The probability that the difference occurred due to chance is more than 0.05.

Question 8

Assume that a study of 500 randomly selected school bus routes showed that 480 arrived on time. Is it "significant" for a school bus to arrive late.

Question 9

Is it "significant" to get an 11 when a pair of dice is rolled.

Question 10

A committee of three people is to be formed. The three people will be selected from a list of five possible committee members. A simple random sample of three people is taken, without replacement, from the group of five people. Using the letters A, B, C, D, E to represent the five people, list the possible samples of size three and use your list to determine the probability that B is included in the sample. (Hint: There are 10 possible samples.)

Question 11

The distribution of B.A. degrees conferred by a local college is listed below, by major. 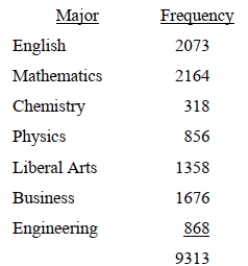What is the probability that a randomly selected degree is not in Business?

Question 12

Based on meteorological records, the probability that it will snow in a certain town on January 1st is 0.413. Find the probability that in a given year it will not snow on January 1st in that town.

Question 13

If you flip a coin three times, the possible outcomes are HHH, HHT, HTH, HTT, THH, THT, TTH, TTT. What is the probability of getting at most one head?

Question 14

Is it "significant" to get a total of 2 when a pair of dice is rolled.

Question 15

A study of students taking Statistics 101 was done. Four hundred students who studied for more than 10 hours averaged a B. Two hundred students who studied for less than 10 hours averaged a C. This difference was significant at the 0.01 level. What does this mean?

A) The probability that the difference was due to chance alone is greater than 0.01.
B) There is less than a 0.01 chance that the first group's grades were better by chance alone.
C) The improvement was due to the fact that more people studied.
D) There is not enough information to make any conclusion.

Question 16

Suppose you have an extremely unfair coin: The probability of a head is 1/3 and the probability of a tail is 2/3. If you toss the coin 72 times, how many heads do you expect to see?

Question 17

A bag contains 4 red marbles, 3 blue marbles, and 7 green marbles. If a marble is randomly selected from the bag, what is the probability that it is blue?

Question 18

From the table in Problem 16, how many 70-year old females on average will be living at age 71?

Question 19

A sample space consists of 46 separate events that are equally likely. What is the probability of each?

Question 20

The data set represents the income levels of the members of a country club. Estimate the probability that a randomly selected member earns at least $98,000.
$$\begin{array} { r r r r r r r r r r } 112,000 & 126,000 & 90,000 & 133,000 & 94,000 & 112,000 & 98,000 & 82,000 & 147,000 & 182,000 \\86,000 & 105,000 & 140,000 & 94,000 & 126,000 & 119,000 & 98,000 & 154,000 & 78,000 & 119,000\end{array}$$

Question 21

If you flip a coin three times, the possible outcomes are HHH, HHT, HTH, HTT, THH, THT, TTH, TTT. What is the probability of getting at least two tails?

Question 22

A study of two types of weed killers was done on two identical weed plots. One weed killer killed 15% more weeds than the other. This difference was significant at the 0.05 level. What Does this mean?

A) The improvement was due to the fact that there were more weeds in one study.
B) The probability that the difference was due to chance alone is greater than 0.05.
C) The probability that one weed killer performed better by chance alone is less than 0.05.
D) There is not enough information to make any conclusion.

Question 23

A class consists of 50 women and 82 men. If a student is randomly selected, what is the probability that the student is a woman?

Question 24

state whether there is one way for the given observation to occur (outcome) or more than one way for the given observation to occur (in which case it is an event).

-Rolling a 4 with a fair die

A) Outcome
B) Event

Question 25

100 employees of a company are asked how they get to work and whether they work full time or part time. The figure below shows the transportation results. If one of the 100 employees is randomly selected, find the probability that the person does not commute by public transportation.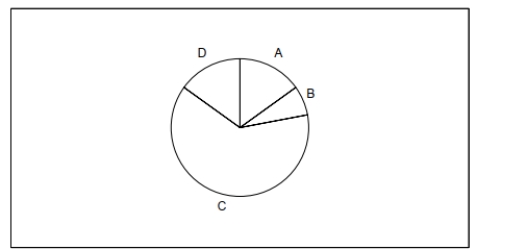

A) Public transportation: 7 full-time, 8 part-time
B) Bicycle: 3 full-time, 4 part-time
C) Drive alone: 29 full-time, 34 part-time
D) Carpool: 9 full-time, 6 part-time

Question 26

state whether there is one way for the given observation to occur (outcome) or more than one way for the given observation to occur (in which case it is an event).

-Drawing three queens from a regular deck of cards

A) Outcome
B) Event

Question 27

state whether there is one way for the given observation to occur (outcome) or more than one way for the given observation to occur (in which case it is an event).

-Tossing one head with a penny followed by one tail with a dime

A) Outcome
B) Event

Question 28

Sammy and Sally each carry a bag containing a banana, a chocolate bar, and a licorice stick. Simultaneously, they take out a single food item and consume it. The possible pairs of food items that Sally and Sammy consumed are as follows.
chocolate bar - chocolate bar
licorice stick - chocolate bar
banana - banana
chocolate bar - licorice stick
licorice stick - licorice stick
chocolate bar - banana
banana - licorice stick
licorice stick - banana
banana - chocolate bar
Find the probability that no chocolate bar was eaten.

Question 29

Of 1308 people who came into a blood bank to give blood, 314 people had high blood pressure. Estimate the probability that the next person who comes in to give blood will have high blood pressure (to 3 decimal places).

Question 30

From the table in Problem 16, how many 60-year old females on average will be living at age 61?

Question 31

In the first series of rolls of a die, the number of odd numbers exceeded the number of even numbers by 5. In the second series of rolls of the same die, the number of odd numbers exceeded the number of even numbers by 11. Determine which series is closer to the 50/50 ratio of odd/even expected of a fairly rolled die.

A) The second series is closer because the difference between odd and even numbers is greater than the difference for the first series.
B) The first series is closer because the difference between odd and even numbers is less than the difference for the second series.
C) Since $1 / 2 > 1 / 5 > 1 / 11$ , the first series is closer.
D) The series closer to the theoretical 50/50 cannot be determined unless the total number of rolls for both series is given.

Question 32

If you flip a coin three times, the possible outcomes are HHH, HHT, HTH, HTT, THH, THT, TTH, TTT. What is the probability that at least two heads occur consecutively?

Question 33

Suppose you buy 1 ticket for $1 out of a lottery of 1000 tickets where the prize for the one winning ticket is to be $500. What is your expected value?

A) $0.00
B) −$0.40
C) −$1.00
D) −$0.50

Question 34

state whether there is one way for the given observation to occur (outcome) or more than one way for the given observation to occur (in which case it is an event).

-Tossing two tails with two fair coins

A) Outcome
B) Event

Question 35

The following table is from the Social Security Actuarial Tables. For each age, it gives the probability of death within one year, the number living out of an original 100,000, and the additional life expectancy for a person of that age.
Exact Male Female P(Death Additional P(Death Additional Age
$\begin{array}{c}\quad\quad\quad\text { Male } \quad\quad\quad\quad\quad\quad\quad\quad\quad\quad\quad\text { Female }\\\begin{array}{|c|c|c|c|c|c|c|}\hline{\text {Exact Age }} & \begin{array}{c}\text { P(Death } \\\text { within one } \\\text { year) }\end{array} & \begin{array}{c}\text { Number of } \\\text { Living }\end{array} & \begin{array}{c}\text { Additional } \\\text { Life } \\\text { Expectancy }\end{array} & \begin{array}{c}\text { P(Death } \\\text { within one } \\\text { year) }\end{array} & \begin{array}{c}\text { Number of } \\\text { Living }\end{array} & \begin{array}{c}\text { Additional } \\\text { Life } \\\text { Expectancy }\end{array} \\\hline 10 & 0.000111 & 99,021 & 65.13 & 0.000105 & 99,217 & 70.22 \\\hline 20 & 0.001287 & 98,451 & 55.46 & 0.000469 & 98,950 & 60.40 \\\hline 30 & 0.001375 & 97,113 & 46.16 & 0.000627 & 98,431 & 50.69 \\\hline 40 & 0.002542 & 95,427 & 36.88 & 0.001498 & 97,513 & 41.11 \\\hline 50 & 0.005696 & 91,853 & 28.09 & 0.003240 & 95,378 & 31.91 \\\hline 60 & 0.012263 & 84,692 & 20.00 & 0.007740 & 90,847 & 23.21 \\\hline 70 & 0.028904 & 70,214 & 12.98 & 0.018938 & 80,583 & 15.45 \\\hline 80 & 0.071687 & 44,272 & 7.43 & 0.049527 & 59,341 & 9.00 \\\hline 90 & 0.188644 & 12,868 & 3.68 & 0.146696 & 24,331 & 4.45 \\\hline\end{array}\end{array}$ To what age may a male of age 50 expect to live on the average?

Question 36

Wisconsin has 72 counties. The names of six of them begin with the letter C. Is it "significant" for a randomly chosen Wisconsin county to be one of those six counties.

Question 37

On a multiple choice test, each question has 6 possible answers. If you make a random guess on the first question, what is the probability that you are correct?

Question 38

If you are told that a mystery person's birthday is in February, would it be "significant" to
guess that person's birth date (not including the year).

Question 39

The probability that Luis will pass his statistics test is 0.94. Find the probability that he will fail his statistics test.

Question 40

Suppose you have an extremely unfair die: The probability of a 6 is 3/8, and the probability of each other number is 1/8. If you toss the die 32 times, how many twos do you expect to see?

Question 41

If you flip a coin three times, the possible outcomes are HHH, HHT, HTH, THH, HTT, THT, TTH, TTT. What is the probability of getting at least one head and at least one tail?

Question 42

The following table is from the Social Security Actuarial Tables. For each age, it gives the probability of death within one year, the number living out of an original 100,000, and the additional life expectancy for a person of that age.
Exact Male Female P(Death Additional P(Death Additional Age
$\begin{array}{c}\quad\quad\quad\text { Male } \quad\quad\quad\quad\quad\quad\quad\quad\quad\quad\quad\text { Female }\\\begin{array}{|c|c|c|c|c|c|c|}\hline{\text {Exact Age }} & \begin{array}{c}\text { P(Death } \\\text { within one } \\\text { year) }\end{array} & \begin{array}{c}\text { Number of } \\\text { Living }\end{array} & \begin{array}{c}\text { Additional } \\\text { Life } \\\text { Expectancy }\end{array} & \begin{array}{c}\text { P(Death } \\\text { within one } \\\text { year) }\end{array} & \begin{array}{c}\text { Number of } \\\text { Living }\end{array} & \begin{array}{c}\text { Additional } \\\text { Life } \\\text { Expectancy }\end{array} \\\hline 10 & 0.000111 & 99,021 & 65.13 & 0.000105 & 99,217 & 70.22 \\\hline 20 & 0.001287 & 98,451 & 55.46 & 0.000469 & 98,950 & 60.40 \\\hline 30 & 0.001375 & 97,113 & 46.16 & 0.000627 & 98,431 & 50.69 \\\hline 40 & 0.002542 & 95,427 & 36.88 & 0.001498 & 97,513 & 41.11 \\\hline 50 & 0.005696 & 91,853 & 28.09 & 0.003240 & 95,378 & 31.91 \\\hline 60 & 0.012263 & 84,692 & 20.00 & 0.007740 & 90,847 & 23.21 \\\hline 70 & 0.028904 & 70,214 & 12.98 & 0.018938 & 80,583 & 15.45 \\\hline 80 & 0.071687 & 44,272 & 7.43 & 0.049527 & 59,341 & 9.00 \\\hline 90 & 0.188644 & 12,868 & 3.68 & 0.146696 & 24,331 & 4.45 \\\hline\end{array}\end{array}$
To what age may a male of age 60 expect to live on the average?

Question 43

From four men and two women, a committee is formed by drawing four names out of a hat.
List all possible drawings and determine the probability that two men and two women are selected. (Hint: Your list should contain 15 possibilities.)

Question 44

The advertising for a cold remedy claimed that no other cold remedy acted faster. In a experiment to compare that remedy with another one, it did act faster on average, but the result was not significant. What does this mean?

A) The difference was so small that it could have happened by chance even if the remedies were equivalent.
B) The difference was so small that it could have happened by chance even if the remedies were not equivalent.
C) The probability of the observed difference occurring by chance if the two remedies are equivalent was less than 0.05.
D) The population mean response times are different, but the samples didn't show it.

Question 45

A bag contains four chips of which one is red, one is blue, one is green, and one is yellow. A chip is selected at random from the bag and then replaced in the bag. A second chip is then selected at random. Make a list of the possible outcomes (for example RB represents the outcome red chip followed by blue chip) and use your list to determine the probability that the two chips selected are the same color. (Hint: There are 16 possible outcomes.)

Question 46

If a sample space has 5000 equally likely possible outcomes, what is the probability of each one?

Question 47

state whether there is one way for the given observation to occur (outcome) or more than one way for the observation to occur (event).

-Five electronic switches are tested at random from a day's production and the first one is found to be defective. Not knowing the status of the other four, is this observation an outcome or an event?

A) Outcome
B) Event

Question 48

Is it significant to be dealt an ace when you are dealt one card from a standard 52-card deck.
(There are four aces in the deck.)

Question 49

From the table in Problem 16, how many 50-year old females on average will be living at age 51?

Question 50

If a person is randomly selected, find the probability that his or her birthday is not in May.
Ignore leap years. There are 365 days in a year. Express your answer as a fraction.

Question 51

In a certain class of students, there are 10 boys from Wilmette, 4 girls from Kenilworth, 9 girls from Wilmette, 5 boys from Glencoe, 5 boys from Kenilworth and 6 girls from Glencoe.
If the teacher calls upon a student to answer a question, what is the probability that the student will be a girl? Express your answer to 3 decimal places.

Question 52

A bag of marbles hold 5 red marbles, 6 green marbles and 14 blue marbles. If one marble is drawn out, what is the probability that it green?

Question 53

Jody checked the temperature 12 times on Monday, and the last digit of the temperature was odd six times more than it was even. On Tuesday, she checked it 18 times and the last digit was odd eight times more than it was even. Determine which series is closer to the 50/50 ratio of odd/even expected of such a series of temperature checks.

A) The Monday series is closer because 1/6 is closer to 1/2 than is 1/8.
B) The Monday series is closer because 6/12 is closer to 0.5 than is 8/18.
C) The Tuesday series is closer because the 13/18 is closer to 0.5 than is 9/12.
D) The series closest to the theoretical 50/50 cannot be determined without knowing the
Number of odds and evens in each series.

Question 54

100 employees of a company are asked how they get to work and whether they work full time or part time. The following figure shows the results for transportation. If one of the 100 employees is randomly selected, find the probability that the person does not ride a bicycle.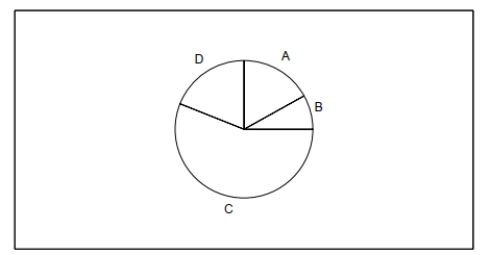

A) Public transportation: 8 full time, 9 part time
B) Bicycle: 3 full time, 5 part time
C) Drive alone: 29 full time, 27 part time
D) Carpool: 9 full time, 10 part time

Question 55

A 28-year-old man pays $125 for a one-year life insurance policy with coverage of $140,000. If the probability that he will live through the year is 0.9994, to the nearest dollar, what is the man's expected value for the insurance policy?

A) $139,916
B) ?$41
C) $84
D) ?$124

Question 56

state whether there is one way for the given observation to occur (outcome) or more than one way for the observation to occur (event).

-A coin is tossed three times and HHT (heads, heads, tails) is observed. Is this result an outcome or an event?

A) Outcome
B) Event

Question 57

In a poll, respondents were asked whether they had ever been in a car accident.
respondents indicated that they had been in a car accident and 370 respondents said that they had not been in a car accident. If one of these respondents is randomly selected, what is the probability of getting someone who has been in a car accident? Round to the nearest thousandth.

Question 58

A die with 12 sides is rolled. What is the probability of rolling a number less than 11? Is this the same as rolling a total less than 11 with two six-sided dice? Explain.

Question 59

A collection of western novels by Zane Grey available from Amazon for a Kindle device contains 26 novels. If one novel is selected at random, would it be considered significant if the novel selected was The Mysterious Rider.

Question 60

Suppose you have an extremely unfair coin: The probability of a head is 1/5, and the probability of a tail is 4/5 . If you toss the coin 40 times, how many heads do you expect to see?

Question 61

In 2003, the U.S. death rate was 1.2 per 100,000 people due to motorcycle accidents.
Motorcycles in the U.S. were involved in fatal crashes at the rate of 35.0 per 100 million miles driven. If the population of the U.S. is 300,000,000, what is the expected number of deaths due to motorcycle accidents?

Question 62

From the table in Problem 16, how many 60-year old females on average will be living at age 61?

Question 63

At a meeting of people interested in genealogy, the following nationalities were represented. $$\begin{array} { | l | c | } \hline \text { Nationality } & \text { Frequency } \\\hline \text { English } & 42 \\\hline \text { Norwegian } & 73 \\\hline \text { Danish } & 37 \\\hline \text { German } & 26 \\\hline \text { French } & 13 \\\hline \text { Italian } & 51 \\\hline & 242 \\\hline\end{array}$$
One person is selected to win a door prize. What is the probability that the person selected is not Danish?

Question 64

The following table is from the Social Security Actuarial Tables. For each age, it gives the probability of death within one year, the number living out of an original 100,000, and the additional life expectancy for a person of that age.
Exact Male Female P(Death Number of Life P(Death Number of Life age
$\begin{array}{c}\quad\quad\quad\text { Male } \quad\quad\quad\quad\quad\quad\quad\quad\quad\quad\quad\text { Female }\\\begin{array}{|c|c|c|c|c|c|c|}\hline{\text {Exact Age }} & \begin{array}{c}\text { P(Death } \\\text { within one } \\\text { year) }\end{array} & \begin{array}{c}\text { Number of } \\\text { Living }\end{array} & \begin{array}{c}\text { Additional } \\\text { Life } \\\text { Expectancy }\end{array} & \begin{array}{c}\text { P(Death } \\\text { within one } \\\text { year) }\end{array} & \begin{array}{c}\text { Number of } \\\text { Living }\end{array} & \begin{array}{c}\text { Additional } \\\text { Life } \\\text { Expectancy }\end{array} \\\hline 10 & 0.000111 & 99,021 & 65.13 & 0.000105 & 99,217 & 70.22 \\\hline 20 & 0.001287 & 98,451 & 55.46 & 0.000469 & 98,950 & 60.40 \\\hline 30 & 0.001375 & 97,113 & 46.16 & 0.000627 & 98,431 & 50.69 \\\hline 40 & 0.002542 & 95,427 & 36.88 & 0.001498 & 97,513 & 41.11 \\\hline 50 & 0.005696 & 91,853 & 28.09 & 0.003240 & 95,378 & 31.91 \\\hline 60 & 0.012263 & 84,692 & 20.00 & 0.007740 & 90,847 & 23.21 \\\hline 70 & 0.028904 & 70,214 & 12.98 & 0.018938 & 80,583 & 15.45 \\\hline 80 & 0.071687 & 44,272 & 7.43 & 0.049527 & 59,341 & 9.00 \\\hline 90 & 0.188644 & 12,868 & 3.68 & 0.146696 & 24,331 & 4.45 \\\hline\end{array}\end{array}$
To what age may a female of age 60 expect to live on the average?

Question 65

In 2012, Michael Fiers' record as a pitcher for the Milwaukee Brewers was 9 wins and 10 losses. In those 19 games he gave up the following numbers of hits:
5, 8, 10, 4, 2, 7, 5, 4, 8, 3, 9, 5, 5, 4, 6, 5, 6, 9, 6
Given that Michael Fiers wins or loses a game, estimate the probability that he gives up fewer than 6 hits.

Question 66

Suppose that you pay $2 to roll the die in Problem 13 with the understanding that you will receive $6 if it comes up a 1 or a 3, but nothing otherwise. What is your expected value?

Question 67

If a quarterback completes 67% of his passes, what is the probability that he will not complete his next pass?

Question 68

Suppose that you have a loaded die for which the probabilities of the numbers 1 through 6 are given below.
$$\begin{array} { | l | l | l | l | l | l | l | } \hline \text { Number } & 1 & 2 & 3 & 4 & 5 & 6 \\\hline \text { Probability } & 1 / 21 & 4 / 21 & 6 / 21 & 2 / 21 & 3 / 21 & 5 / 21 \\\hline\end{array}$$ If you roll this die 630 times, how many times should you expect to see a 1 or a 2?