Deck 22: Gausss Law
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/44
Play
Full screen (f)
Deck 22: Gausss Law
1
An advantage in evaluating surface integrals related to Gauss's law for symmetric charge distributions is
A)the flux is outward.
B)the flux is inward.
C)the electric field is of constant magnitude on certain surfaces.
D)the charge is always on the surface.
E)the electric field is a constant on any surface.
A)the flux is outward.
B)the flux is inward.
C)the electric field is of constant magnitude on certain surfaces.
D)the charge is always on the surface.
E)the electric field is a constant on any surface.
the electric field is of constant magnitude on certain surfaces.
2
Gauss's law may be applied only to charge distributions that are symmetric.
False
3
If the net flux through a closed surface is positive, then the net charge enclosed must be positive.
True
4
Gauss's law can be applied using any surface.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
5
Gaussian surfaces A and B enclose the same positive charge +Q. The area of Gaussian surface A is three times larger than that of Gaussian surface B. The flux of electric field through Gaussian surface A is
A)nine times larger than the flux of electric field through Gaussian surface B.
B)three times larger than the flux of electric field through Gaussian surface B.
C)equal to the flux of electric field through Gaussian surface B.
D)three times smaller than the flux of electric field through Gaussian surface B.
E)unrelated to the flux of electric field through Gaussian surface B.
A)nine times larger than the flux of electric field through Gaussian surface B.
B)three times larger than the flux of electric field through Gaussian surface B.
C)equal to the flux of electric field through Gaussian surface B.
D)three times smaller than the flux of electric field through Gaussian surface B.
E)unrelated to the flux of electric field through Gaussian surface B.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
6
Outside a spherically symmetric charge distribution of net charge Q, Gauss's law can be used to show that the electric field at a given distance
A)must be zero.
B)must be directed outward.
C)acts like it originated in a point charge Q at the center of the distribution.
D)must be directed inward.
E)must be greater than zero.
A)must be zero.
B)must be directed outward.
C)acts like it originated in a point charge Q at the center of the distribution.
D)must be directed inward.
E)must be greater than zero.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
7
Consider a spherical Gaussian surface of radius R centered at the origin. A charge Q is placed inside the sphere. Where should the charge be located to maximize the magnitude of the flux of the electric field through the Gaussian surface?
A)at x = 0, y = 0, z = R/2
B)at the origin
C)at x = R/2, y = 0, z = 0
D)at x = 0, y = R/2, z = 0
E)The flux does not depend on the position of the charge as long as it is inside the sphere
A)at x = 0, y = 0, z = R/2
B)at the origin
C)at x = R/2, y = 0, z = 0
D)at x = 0, y = R/2, z = 0
E)The flux does not depend on the position of the charge as long as it is inside the sphere

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
8
A region of space contains a uniform electric field oriented along the y-axis. A frame of surface area A is placed perpendicular to the y-axis in the xz-plane. The magnitude of the electric flux through this frame is Φ0. A second frame is placed in the same electric field in such a way that the magnitude of the electric flux through it is Φ0/2. How is the plane of second frame oriented with respect to the plane of the first one?
A)at a 90° angle
B)at a 60° angle
C)parallel to the first frame
D)perpendicular to the first frame
E)at a 30° angle
A)at a 90° angle
B)at a 60° angle
C)parallel to the first frame
D)perpendicular to the first frame
E)at a 30° angle

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
9
If the electric flux through a rectangular area is 5.0 N m2/C, and the electric field is then doubled, what is the resulting flux through the area?
A)5.0 N m2/C
B)10 N m2/C
C)2.5 N m2/C
D)1 N m2/C
E)20 N m2/C
A)5.0 N m2/C
B)10 N m2/C
C)2.5 N m2/C
D)1 N m2/C
E)20 N m2/C

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
10
If a closed surface surrounds a dipole, the net flux through the surface is zero.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
11
FIGURE 22-1 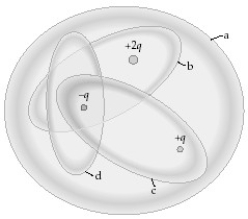
Fig. 22-1 shows four Gaussian surfaces surrounding a distribution of charges. Which Gaussian surfaces have no electric flux through them?
A)a.
B)b.
C)c.
D)b and d.
E)b and c.

Fig. 22-1 shows four Gaussian surfaces surrounding a distribution of charges. Which Gaussian surfaces have no electric flux through them?
A)a.
B)b.
C)c.
D)b and d.
E)b and c.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
12
State Gauss's law.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
13
FIGURE 22-1 
Fig. 22-1 shows four Gaussian surfaces surrounding a distribution of charges. Which Gaussian surfaces have an electric flux of +q/ εO through them?
A)a.
B)b.
C)b and d.
D)b and c.
E)c.

Fig. 22-1 shows four Gaussian surfaces surrounding a distribution of charges. Which Gaussian surfaces have an electric flux of +q/ εO through them?
A)a.
B)b.
C)b and d.
D)b and c.
E)c.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
14
A charge Q is positioned at the center of a sphere of radius R. The flux of the electric field through the sphere is equal to Φ. If the charge Q is now placed at the center of a cube the flux of the electric field through the surface of the cube is equal to
A)Φ/2.
B)Φ.
C)2Φ.
D)0.
E)The value of the flux depends on the dimensions of the cube.
A)Φ/2.
B)Φ.
C)2Φ.
D)0.
E)The value of the flux depends on the dimensions of the cube.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
15
A uniform electric field 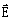 = E0
= E0 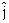 is set-up in a region of space. A frame is placed in that region in such a way that its plane is perpendicular to the y-axis. Which of the following changes would decrease the magnitude of the electric flux through the frame?
is set-up in a region of space. A frame is placed in that region in such a way that its plane is perpendicular to the y-axis. Which of the following changes would decrease the magnitude of the electric flux through the frame?
A)Sliding the frame sideways parallel to the z-axis within the xz-plane
B)moving the frame vertically along the y-axis keeping parallel to the xz-plane
C)rotating the frame in the xz-plane with respect to the y-axis
D)sliding the frame sideways parallel to the x-axis within the xz-plane
E)tilting the frame so that its plane is now in the yz-plane
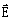 = E0
= E0 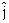 is set-up in a region of space. A frame is placed in that region in such a way that its plane is perpendicular to the y-axis. Which of the following changes would decrease the magnitude of the electric flux through the frame?
is set-up in a region of space. A frame is placed in that region in such a way that its plane is perpendicular to the y-axis. Which of the following changes would decrease the magnitude of the electric flux through the frame?A)Sliding the frame sideways parallel to the z-axis within the xz-plane
B)moving the frame vertically along the y-axis keeping parallel to the xz-plane
C)rotating the frame in the xz-plane with respect to the y-axis
D)sliding the frame sideways parallel to the x-axis within the xz-plane
E)tilting the frame so that its plane is now in the yz-plane

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
16
If a rectangular area is rotated in a uniform electric field from the position where the maximum electric flux goes through it to an orientation where only half the flux goes through it, what has been the angle of rotation?
A)45°
B)26.6°
C)90°
D)30°
E)60°
A)45°
B)26.6°
C)90°
D)30°
E)60°

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
17
If a charge is located at the center of a spherical volume and the electric flux through the surface of the sphere is Φ0, what is the flux through the surface if the radius of the sphere doubles?
A)0.125 Φ0
B)Φ0
C)5 Φ0
D)8 Φ0
E)0.500 Φ0
A)0.125 Φ0
B)Φ0
C)5 Φ0
D)8 Φ0
E)0.500 Φ0

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
18
The electric field in a region of space is oriented along the positive y axis. A circle of radius R is placed in the xz-plane. The flux of the electric field through this circle is Φ. The same electric field passing through a second circle of radius 2R parallel to xz-plane would result in a flux equal to
A)Φ.
B)0.
C)4Φ.
D)2Φ.
E)3Φ.
A)Φ.
B)0.
C)4Φ.
D)2Φ.
E)3Φ.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
19
If the net flux through a closed surface is zero, then there can be no charge or charges within that surface.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
20
A positive charge Q is located at the center of an imaginary Gaussian cube of sides a. The flux of the electric field through the surface of the cube is Φ. A second, negative charge -Q is placed next to Q inside the cube. Which of the following statements will be true in this case?
A)The net electric field on the surface of the cube is equal to zero
B)The electric field on the surface of the cube is perpendicular to the surface
C)The magnitude of the net electric field is constant on the entire surface of the cube
D)The net flux through the surface of the cube is equal to zero
E)The net flux through the surface is equal to 2Φ
A)The net electric field on the surface of the cube is equal to zero
B)The electric field on the surface of the cube is perpendicular to the surface
C)The magnitude of the net electric field is constant on the entire surface of the cube
D)The net flux through the surface of the cube is equal to zero
E)The net flux through the surface is equal to 2Φ

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
21
Two long straight parallel lines of charge, #1 and #2, carry positive charge per unit lengths of λ1 and λ2 respectively. λ1 > λ2. The electric field halfway between the lines, which are separated by a distance a, has magnitude

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
22
A charge q = 2 μC is placed at the origin in a region where there is already a uniform electric field 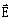 = (100 N/C)
= (100 N/C) 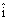 . Calculate the flux of the net electric field through a Gaussian sphere of radius R = 10 cm centered at the origin.
. Calculate the flux of the net electric field through a Gaussian sphere of radius R = 10 cm centered at the origin.
A)5.52 × 105 Nm2/C
B)1.13 × 105 Nm2/C
C)2.26 × 105 Nm2/C
D)0.565 × 105 Nm2/C
E)0
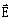 = (100 N/C)
= (100 N/C) 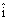 . Calculate the flux of the net electric field through a Gaussian sphere of radius R = 10 cm centered at the origin.
. Calculate the flux of the net electric field through a Gaussian sphere of radius R = 10 cm centered at the origin.A)5.52 × 105 Nm2/C
B)1.13 × 105 Nm2/C
C)2.26 × 105 Nm2/C
D)0.565 × 105 Nm2/C
E)0

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
23
A solid non-conducting sphere of radius R carries a charge Q1 distributed uniformly. The sphere is surrounded by a concentric spherical shell of inner radius Ra and outer radius Rb. The shell carries a total charge Q2 distributed uniformly in its volume. What is the net electric field at a radial distance r such that R < r < Ra?

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
24
Consider two oppositely charged, parallel metal plates. The plates are square with sides L and carry charges Q and -Q. What is the magnitude of the electric field in the region between the plates?
A)E =
B)E =
C)E=0
D)E =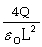
E)E=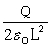
A)E =

B)E =

C)E=0
D)E =
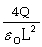
E)E=
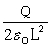

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
25
A long straight line of charge has a uniform positive charge per unit length λ. The line is partially enclosed in a long rectangular box of length L and ends of area A, the line running through the center of each end. The electric flux through the surface of the box is

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
26
If the electric flux through a circular area is 5.0 Nm2/C, what is the electric flux through a circle of double the diameter assuming the orientations of the circles are the same and the electric field is uniform?
A)5.0 Nm2/C
B)20 Nm2/C
C)2.5 Nm2/C
D)1.0 Nm2/C
E)10.0 Nm2/C
A)5.0 Nm2/C
B)20 Nm2/C
C)2.5 Nm2/C
D)1.0 Nm2/C
E)10.0 Nm2/C

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
27
Three parallel flat planes of charge are separated by a distance d between each of the planes. The charge density on each of the planes is σ. The maximum magnitude of the electric field in the vicinity of the planes is

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
28
Consider an electric field 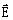 = 2x
= 2x 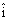 - 3y
- 3y 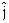 . The coordinates x and y are measured in meters and the electric field is in N/C. What is the magnitude of the flux of this field through a square whose corners are located at (x, y, z) = (0, 2, 0), (2, 2, 0), (0, 2, 2)?
. The coordinates x and y are measured in meters and the electric field is in N/C. What is the magnitude of the flux of this field through a square whose corners are located at (x, y, z) = (0, 2, 0), (2, 2, 0), (0, 2, 2)?
A)6 Nm2/C
B)0
C)24 Nm2/C
D)12 Nm2/C
E)48 Nm2/C
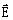 = 2x
= 2x 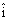 - 3y
- 3y 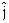 . The coordinates x and y are measured in meters and the electric field is in N/C. What is the magnitude of the flux of this field through a square whose corners are located at (x, y, z) = (0, 2, 0), (2, 2, 0), (0, 2, 2)?
. The coordinates x and y are measured in meters and the electric field is in N/C. What is the magnitude of the flux of this field through a square whose corners are located at (x, y, z) = (0, 2, 0), (2, 2, 0), (0, 2, 2)?A)6 Nm2/C
B)0
C)24 Nm2/C
D)12 Nm2/C
E)48 Nm2/C

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
29
A solid non-conducting sphere of radius R carries a uniform charge density. At a radial distance r1 = R/4 the electric field has a magnitude E0. What is the magnitude of the electric field at a radial distance r2 = 2R?
A)E0/4
B)0
C)E0/2
D)E0
E)2E0
A)E0/4
B)0
C)E0/2
D)E0
E)2E0

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
30
A point charge q = +1 μC is located at the origin. What is the flux of the electric field of this charge through a square whose corners are (x, y, z) = (1, 1, 1), (-1, 1, 1), (-1, 1, -1), and (1, 1, -1)?
A)11.3 × 104 Nm2/C
B)0.5 × 104 Nm2/C
C)0
D)1.0 × 104 Nm2/C
E)1.9 × 104 Nm2/C
A)11.3 × 104 Nm2/C
B)0.5 × 104 Nm2/C
C)0
D)1.0 × 104 Nm2/C
E)1.9 × 104 Nm2/C

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
31
A charge Q is uniformly distributed throughout a nonconducting sphere of radius R. The charge density in the sphere is

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
32
A region of space contains an electric field 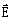 = E1
= E1 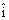 + E2
+ E2 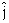 where E1 and E2 are positive constants. A frame whose corners are located at (x, y, z) = (a/2, 0, a/2), (-a/2, 0,-a/2), (a/2, 0,-a/2), and (-a/2, 0, a/2). What is the magnitude of the electric flux through the frame?
where E1 and E2 are positive constants. A frame whose corners are located at (x, y, z) = (a/2, 0, a/2), (-a/2, 0,-a/2), (a/2, 0,-a/2), and (-a/2, 0, a/2). What is the magnitude of the electric flux through the frame?
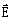 = E1
= E1 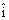 + E2
+ E2 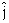 where E1 and E2 are positive constants. A frame whose corners are located at (x, y, z) = (a/2, 0, a/2), (-a/2, 0,-a/2), (a/2, 0,-a/2), and (-a/2, 0, a/2). What is the magnitude of the electric flux through the frame?
where E1 and E2 are positive constants. A frame whose corners are located at (x, y, z) = (a/2, 0, a/2), (-a/2, 0,-a/2), (a/2, 0,-a/2), and (-a/2, 0, a/2). What is the magnitude of the electric flux through the frame?
Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
33
A charge Q is uniformly distributed throughout a nonconducting sphere of radius R.
(a) What is the magnitude of the electric field at a distance R/2 from the center of the sphere?
(b) What is the magnitude of the electric field at a distance 2R from the center of the sphere?
(a) What is the magnitude of the electric field at a distance R/2 from the center of the sphere?
(b) What is the magnitude of the electric field at a distance 2R from the center of the sphere?

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
34
FIGURE 22-2 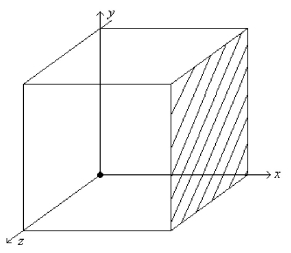
A uniform electric field with a magnitude of 6 × 106 N/C is applied to a cube of edge length 0.1 m as shown in Fig. 22-2. If the direction of the E-field is along the +x-axis, what is the electric flux passing through the shaded face of the cube?
A)0.6 × 104 Nm2/C
B)6 × 104 Nm2/C
C)60 × 104 Nm2/C
D)600 × 104 Nm2/C
E)6000 × 104 Nm2/C

A uniform electric field with a magnitude of 6 × 106 N/C is applied to a cube of edge length 0.1 m as shown in Fig. 22-2. If the direction of the E-field is along the +x-axis, what is the electric flux passing through the shaded face of the cube?
A)0.6 × 104 Nm2/C
B)6 × 104 Nm2/C
C)60 × 104 Nm2/C
D)600 × 104 Nm2/C
E)6000 × 104 Nm2/C

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
35
If a point charge is located at the center of a cylinder and the electric flux leaving one end of the cylinder is 20% of the total flux leaving the cylinder, what portion of the flux leaves the curved surface of the cylinder?
A)20%
B)100%
C)80%
D)40%
E)60%
A)20%
B)100%
C)80%
D)40%
E)60%

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
36
If a point charge is located at the center of a cube and the electric flux through one face of the cube is 5.0 Nm2/C, what is the total flux leaving the cube?
A)20 Nm2/C
B)30 Nm2/C
C)5.0 Nm2/C
D)25 Nm2/C
E)1 Nm2/C
A)20 Nm2/C
B)30 Nm2/C
C)5.0 Nm2/C
D)25 Nm2/C
E)1 Nm2/C

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
37
Three parallel flat planes of charge are separated by a distance d between each of the planes. The charge density on each of the planes is σ. The field in the regions between the planes has magnitude

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
38
Two parallel flat planes of positive charge are separated by a distance d. Plane #1 has charge density σ1 and plane #2 has a charge density σ2. σ1> σ2.
(a) In the region between the planes, the magnitude of the electric field is
(b) In the region outside the planes the magnitude of the electric field is
(a) In the region between the planes, the magnitude of the electric field is
(b) In the region outside the planes the magnitude of the electric field is

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
39
FIGURE 22-2 
An uniform electric field of magnitude E = 100 N/C is oriented along the positive y-axis. What is the magnitude of the flux of this field through a square of surface area A = 2 m2 oriented parallel to the yz-plane?
A)200 Nm2/C
B)100 Nm2/C
C)0
D)400 Nm2/C
E)600 Nm2/C

An uniform electric field of magnitude E = 100 N/C is oriented along the positive y-axis. What is the magnitude of the flux of this field through a square of surface area A = 2 m2 oriented parallel to the yz-plane?
A)200 Nm2/C
B)100 Nm2/C
C)0
D)400 Nm2/C
E)600 Nm2/C

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
40
Two long straight parallel lines of charge, #1 and #2, carry positive charge per unit lengths of λ1 and λ2, respectively. λ1 > λ2. The locus of points where the electric field is zero in this case is
A)along a line between the lines closer to line #2 than line #1.
B)at a point halfway between the lines.
C)along line #1.
D)along a line between the lines closer to line #1 than line #2.
E)cannot be determined
A)along a line between the lines closer to line #2 than line #1.
B)at a point halfway between the lines.
C)along line #1.
D)along a line between the lines closer to line #1 than line #2.
E)cannot be determined

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
41
An infinitely long cylinder of radius R = 2 cm carries a uniform charge density ρ = 18 μC/ m3. Calculate the electric field at distance r = 1 cm from the axis of the cylinder.
A)2.5 × 103 N/C
B)5.1 × 103 N/C
C)0
D)2.0 × 103 N/C
E)10.2 × 103 N/C
A)2.5 × 103 N/C
B)5.1 × 103 N/C
C)0
D)2.0 × 103 N/C
E)10.2 × 103 N/C

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
42
A non-conducting sphere of radius R = 7 cm carries a charge Q = 4 mC distributed uniformly throughout its volume. At what distance, measured from the center of the sphere does the electric field reach a value equal to half its maximum value?
A)3.5 cm only
B)4.9 cm only
C)3.5 cm and 9.9 cm
D)3.5 cm and 4.9 cm
E)9.9 cm only
A)3.5 cm only
B)4.9 cm only
C)3.5 cm and 9.9 cm
D)3.5 cm and 4.9 cm
E)9.9 cm only

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
43
A solid non-conducting sphere of radius R carries a charge Q distributed uniformly throughout its volume. At a radius r (r < R) from the center of the sphere the electric field has a value E. If the same charge Q were distributed uniformly throughout a sphere of radius 2R the magnitude of the electric field at a radius r would be equal to
A)E/8.
B)E/2.
C)2E.
D)8E.
E)E.
A)E/8.
B)E/2.
C)2E.
D)8E.
E)E.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
44
A spherical, non-conducting shell of inner radius r1= 10 cm and outer radius r2= 15 cm carries a total charge Q = 15 μC distributed uniformly throughout its volume. What is the electric field at a distance r= 12 cm from the center of the shell?
A)5.75 × 103 N/C
B)0
C)2.87 × 106 N/C
D)5.75 × 106 N/C
E)2.87 × 103 N/C
A)5.75 × 103 N/C
B)0
C)2.87 × 106 N/C
D)5.75 × 106 N/C
E)2.87 × 103 N/C

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck


