Deck 10: Rotation of a Rigid Object About a Fixed Axis
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/82
Play
Full screen (f)
Deck 10: Rotation of a Rigid Object About a Fixed Axis
1
At t = 0, a wheel rotating about a fixed axis at a constant angular acceleration has an angular velocity of 2.0 rad/s. Two seconds later it has turned through 5.0 complete revolutions. What is the angular acceleration of this wheel?
A) 17 rad/s2
B) 14 rad/s2
C) 20 rad/s2
D) 23 rad/s2
E) 13 rad/s2
A) 17 rad/s2
B) 14 rad/s2
C) 20 rad/s2
D) 23 rad/s2
E) 13 rad/s2
14 rad/s2
2
A wheel (radius = 0.20 m) is mounted on a frictionless, horizontal axis. A light cord wrapped around the wheel supports a 0.50-kg object, as shown in the figure. When released from rest the object falls with a downward acceleration of 5.0 m/s2. What is the moment of inertia of the wheel? 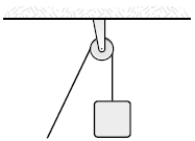
A) 0.023 kg⋅m2
B) 0.027 kg⋅m2
C) 0.016 kg⋅m2
D) 0.019 kg⋅m2
E) 0.032 kg⋅m2

A) 0.023 kg⋅m2
B) 0.027 kg⋅m2
C) 0.016 kg⋅m2
D) 0.019 kg⋅m2
E) 0.032 kg⋅m2
0.019 kg⋅m2
3
A mass (M1 = 5.0 kg) is connected by a light cord to a mass (M2 = 4.0 kg) which slides on a smooth surface, as shown in the figure. The pulley (radius = 0.20 m) rotates about a frictionless axle. The acceleration of M2 is 3.5 m/s2. What is the moment of inertia of the pulley? 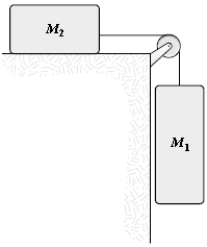
A) 0.29 kg⋅m2
B) 0.42 kg⋅m2
C) 0.20 kg⋅m2
D) 0.62 kg⋅m2
E) 0.60 kg⋅m2

A) 0.29 kg⋅m2
B) 0.42 kg⋅m2
C) 0.20 kg⋅m2
D) 0.62 kg⋅m2
E) 0.60 kg⋅m2
0.20 kg⋅m2
4
A cylinder rotating about its axis with a constant angular acceleration of 1.6 rad/s2 starts from rest at t = 0. At the instant when it has turned through 0.40 radian, what is the magnitude of the total linear acceleration of a point on the rim (radius = 13 cm)?
A) 0.31 m/s2
B) 0.27 m/s2
C) 0.35 m/s2
D) 0.39 m/s2
E) 0.45 m/s2
A) 0.31 m/s2
B) 0.27 m/s2
C) 0.35 m/s2
D) 0.39 m/s2
E) 0.45 m/s2

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
5
A wheel (radius = 0.20 m) starts from rest and rotates with a constant angular acceleration of 2.0 rad/s2. At the instant when the angular velocity is equal to 1.2 rad/s, what is the magnitude of the total linear acceleration of a point on the rim of the wheel?
A) 0.40 m/s2
B) 0.29 m/s2
C) 0.69 m/s2
D) 0.49 m/s2
E) 0.35 m/s2
A) 0.40 m/s2
B) 0.29 m/s2
C) 0.69 m/s2
D) 0.49 m/s2
E) 0.35 m/s2

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
6
A wheel (radius = 0.25 m) is mounted on a frictionless, horizontal axis. The moment of inertia of the wheel about the axis is 0.040 kg⋅m2. A light cord wrapped around the wheel supports a 0.50-kg object as shown in the figure. The object is released from rest. What is the magnitude of the acceleration of the 0.50-kg object? 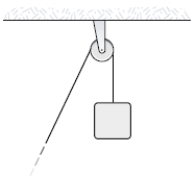
A) 3.0 m/s2
B) 3.4 m/s2
C) 4.3 m/s2
D) 3.8 m/s2
E) 2.7 m/s2

A) 3.0 m/s2
B) 3.4 m/s2
C) 4.3 m/s2
D) 3.8 m/s2
E) 2.7 m/s2

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
7
A wheel rotating about a fixed axis with a constant angular acceleration of 2.0 rad/s2 turns through 2.4 revolutions during a 2.0-s time interval. What is the angular velocity at the end of this time interval?
A) 9.5 rad/s
B) 9.7 rad/s
C) 9.3 rad/s
D) 9.1 rad/s
E) 8.8 rad/s
A) 9.5 rad/s
B) 9.7 rad/s
C) 9.3 rad/s
D) 9.1 rad/s
E) 8.8 rad/s

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
8
A wheel starts from rest and rotates with a constant angular acceleration about a fixed axis. It completes the first revolution 6.0 s after it started. How long after it started will the wheel complete the second revolution?
A) 9.9 s
B) 7.8 s
C) 8.5 s
D) 9.2 s
E) 6.4 s
A) 9.9 s
B) 7.8 s
C) 8.5 s
D) 9.2 s
E) 6.4 s

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
9
A wheel rotates about a fixed axis with a constant angular acceleration of 4.0 rad/s2. The diameter of the wheel is 40 cm. What is the linear speed of a point on the rim of this wheel at an instant when that point has a total linear acceleration with a magnitude of 1.2 m/s2?
A) 39 cm/s
B) 42 cm/s
C) 45 cm/s
D) 35 cm/s
E) 53 cm/s
A) 39 cm/s
B) 42 cm/s
C) 45 cm/s
D) 35 cm/s
E) 53 cm/s

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
10
A disk (radius = 8.0 cm) that rotates about a fixed axis starts from rest and accelerates at a constant rate to an angular velocity of 4.0 rad/s in 2.0 s. What is the magnitude of the total linear acceleration of a point on the rim of the disk at the instant when the angular velocity of the disk is 1.5 rad/s?
A) 24 cm/s2
B) 16 cm/s2
C) 18 cm/s2
D) 34 cm/s2
E) 44 cm/s2
A) 24 cm/s2
B) 16 cm/s2
C) 18 cm/s2
D) 34 cm/s2
E) 44 cm/s2

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
11
At t = 0, a wheel rotating about a fixed axis at a constant angular acceleration of −0.40 rad/s2 has an angular velocity of 1.5 rad/s and an angular position of 2.3 rad. What is the angular position of the wheel at t = 2.0 s?
A) 4.9 rad
B) 4.7 rad
C) 4.5 rad
D) 4.3 rad
E) 4.1 rad
A) 4.9 rad
B) 4.7 rad
C) 4.5 rad
D) 4.3 rad
E) 4.1 rad

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
12
A thin uniform rod (length = 1.2 m, mass = 2.0 kg) is pivoted about a horizontal, frictionless pin through one end of the rod. (The moment of inertia of the rod about this axis is ML2/3.) The rod is released when it makes an angle of 37° with the horizontal. What is the angular acceleration of the rod at the instant it is released?
A) 9.8 rad/s2
B) 7.4 rad/s2
C) 8.4 rad/s2
D) 5.9 rad/s2
E) 6.5 rad/s2
A) 9.8 rad/s2
B) 7.4 rad/s2
C) 8.4 rad/s2
D) 5.9 rad/s2
E) 6.5 rad/s2

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
13
A wheel rotates about a fixed axis with an initial angular velocity of 20 rad/s. During a 5.0-s interval the angular velocity decreases to 10 rad/s. Assume that the angular acceleration is constant during the 5.0-s interval. How many radians does the wheel turn through during the 5.0-s interval?
A) 95 rad
B) 85 rad
C) 65 rad
D) 75 rad
E) 125 rad
A) 95 rad
B) 85 rad
C) 65 rad
D) 75 rad
E) 125 rad

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
14
A horizontal disk with a radius of 10 cm rotates about a vertical axis through its center. The disk starts from rest at t = 0 and has a constant angular acceleration of 2.1 rad/s2. At what value of t will the radial and tangential components of the linear acceleration of a point on the rim of the disk be equal in magnitude?
A) 0.55 s
B) 0.63 s
C) 0.69 s
D) 0.59 s
E) 0.47 s
A) 0.55 s
B) 0.63 s
C) 0.69 s
D) 0.59 s
E) 0.47 s

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
15
A mass m = 4.0 kg is connected, as shown, by a light cord to a mass M = 6.0 kg, which slides on a smooth horizontal surface. The pulley rotates about a frictionless axle and has a radius R = 0.12 m and a moment of inertia I = 0.090 kg⋅m2. The cord does not slip on the pulley. What is the magnitude of the acceleration of m? 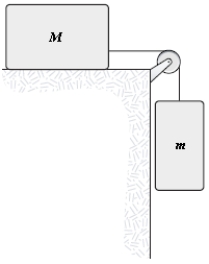
A) 2.4 m/s2
B) 2.8 m/s2
C) 3.2 m/s2
D) 4.2 m/s2
E) 1.7 m/s2

A) 2.4 m/s2
B) 2.8 m/s2
C) 3.2 m/s2
D) 4.2 m/s2
E) 1.7 m/s2

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
16
A wheel rotates about a fixed axis with an initial angular velocity of 20 rad/s. During a 5.0-s interval the angular velocity increases to 40 rad/s. Assume that the angular acceleration was constant during the 5.0-s interval. How many revolutions does the wheel turn through during the 5.0-s interval?
A) 20 rev
B) 24 rev
C) 32 rev
D) 28 rev
E) 39 rev
A) 20 rev
B) 24 rev
C) 32 rev
D) 28 rev
E) 39 rev

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
17
A wheel rotating about a fixed axis has a constant angular acceleration of 4.0 rad/s2. In a 4.0-s interval the wheel turns through an angle of 80 radians. Assuming the wheel started from rest, how long had it been in motion at the start of the 4.0-s interval?
A) 2.5 s
B) 4.0 s
C) 3.5 s
D) 3.0 s
E) 4.5 s
A) 2.5 s
B) 4.0 s
C) 3.5 s
D) 3.0 s
E) 4.5 s

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
18
A wheel rotating about a fixed axis with a constant angular acceleration of 2.0 rad/s2 starts from rest at t = 0. The wheel has a diameter of 20 cm. What is the magnitude of the total linear acceleration of a point on the outer edge of the wheel at t = 0.60 s?
A) 0.25 m/s2
B) 0.50 m/s2
C) 0.14 m/s2
D) 0.34 m/s2
E) 0.20 m/s2
A) 0.25 m/s2
B) 0.50 m/s2
C) 0.14 m/s2
D) 0.34 m/s2
E) 0.20 m/s2

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
19
A wheel rotating about a fixed axis has an angular position given by θ = 3.0 − 2.0t3, where θ is measured in radians and t in seconds. What is the angular acceleration of the wheel at t = 2.0 s?
A) −1.0 rad/s2
B) −24 rad/s2
C) −2.0 rad/s2
D) −4.0 rad/s2
E) −3.5 rad/s2
A) −1.0 rad/s2
B) −24 rad/s2
C) −2.0 rad/s2
D) −4.0 rad/s2
E) −3.5 rad/s2

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
20
The turntable of a record player has an angular velocity of 8.0 rad/s when it is turned off. The turntable comes to rest 2.5 s after being turned off. Through how many radians does the turntable rotate after being turned off? Assume constant angular acceleration.
A) 12 rad
B) 8.0 rad
C) 10 rad
D) 16 rad
E) 6.8 rad
A) 12 rad
B) 8.0 rad
C) 10 rad
D) 16 rad
E) 6.8 rad

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
21
Particles (mass of each = 0.40 kg) are placed at the 60-cm and 100-cm marks of a meter stick of negligible mass. This rigid body is free to rotate about a frictionless pivot at the 0-cm end. The body is released from rest in the horizontal position. What is the magnitude of the initial linear acceleration of the end of the body opposite the pivot?
A) 15 m/s2
B) 9.8 m/s2
C) 5.8 m/s2
D) 12 m/s2
E) 4.7 m/s2
A) 15 m/s2
B) 9.8 m/s2
C) 5.8 m/s2
D) 12 m/s2
E) 4.7 m/s2

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
22
If M = 0.50 kg, L = 1.2 m, and the mass of each connecting rod shown is negligible, what is the moment of inertia about an axis perpendicular to the paper through the center of mass? Treat the mass as particles. 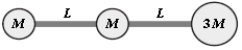
A) 3.7 kg⋅m2
B) 2.8 kg⋅m2
C) 3.2 kg⋅m2
D) 2.3 kg⋅m2
E) 3.9 kg⋅m2

A) 3.7 kg⋅m2
B) 2.8 kg⋅m2
C) 3.2 kg⋅m2
D) 2.3 kg⋅m2
E) 3.9 kg⋅m2

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
23
Particles (mass of each = 0.20 kg) are placed at the 40-cm and 100-cm marks of a meter stick of negligible mass. This rigid body is free to rotate about a frictionless pivot at the 0-cm end. The body is released from rest in the horizontal position. What is the initial angular acceleration of the body?
A) 12 rad/s2
B) 5.9 rad/s2
C) 8.4 rad/s2
D) 5.4 rad/s2
E) 17 rad/s2
A) 12 rad/s2
B) 5.9 rad/s2
C) 8.4 rad/s2
D) 5.4 rad/s2
E) 17 rad/s2

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
24
Three particles, each of which has a mass of 80 g, are positioned at the vertices of an equilateral triangle with sides of length 60 cm. The particles are connected by rods of negligible mass. What is the moment of inertia of this rigid body about an axis that is parallel to one side of the triangle and passes through the respective midpoints of the other two sides?
A) 0.018 kg⋅m2
B) 0.020 kg⋅m2
C) 0.016 kg⋅m2
D) 0.022 kg⋅m2
E) 0.032 kg⋅m2
A) 0.018 kg⋅m2
B) 0.020 kg⋅m2
C) 0.016 kg⋅m2
D) 0.022 kg⋅m2
E) 0.032 kg⋅m2

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
25
A uniform rod (length = 2.0 m) is mounted to rotate freely about a horizontal axis that is perpendicular to the rod and that passes through the rod at a point 0.50 m from one end of the rod. If the rod is released from rest in a horizontal position, what is the angular speed of the rod as it rotates through its lowest position?
A) 3.5 rad/s
B) 3.8 rad/s
C) 4.1 rad/s
D) 2.0 rad/s
E) 5.6 rad/s
A) 3.5 rad/s
B) 3.8 rad/s
C) 4.1 rad/s
D) 2.0 rad/s
E) 5.6 rad/s

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
26
A uniform rod is 3.0 m long. The rod is pivoted about a horizontal, frictionless pin through one end. The rod is released from rest at an angle of 27° above the horizontal. What is the angular speed of the rod as it passes through the horizontal position?
A) 3.0 rad/s
B) 2.8 rad/s
C) 2.1 rad/s
D) 2.5 rad/s
E) 3.4 rad/s
A) 3.0 rad/s
B) 2.8 rad/s
C) 2.1 rad/s
D) 2.5 rad/s
E) 3.4 rad/s

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
27
A wheel (radius = 12 cm) is mounted on a frictionless, horizontal axle that is perpendicular to the wheel and passes through the center of mass of the wheel. A light cord wrapped around the wheel supports a 0.40-kg object. If released from rest with the string taut, the object is observed to fall with a downward acceleration of 3.0 m/s2. What is the moment of inertia (of the wheel) about the given axle?
A) 0.023 kg⋅m2
B) 0.013 kg⋅m2
C) 0.020 kg⋅m2
D) 0.016 kg⋅m2
E) 0.035 kg⋅m2
A) 0.023 kg⋅m2
B) 0.013 kg⋅m2
C) 0.020 kg⋅m2
D) 0.016 kg⋅m2
E) 0.035 kg⋅m2

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
28
Identical particles are placed at the 50-cm and 80-cm marks on a meter stick of negligible mass. This rigid body is then mounted so as to rotate freely about a pivot at the 0-cm mark on the meter stick. If this body is released from rest in a horizontal position, what is the angular speed of the meter stick as it swings through its lowest position?
A) 4.2 rad/s
B) 5.4 rad/s
C) 4.6 rad/s
D) 5.0 rad/s
E) 1.7 rad/s
A) 4.2 rad/s
B) 5.4 rad/s
C) 4.6 rad/s
D) 5.0 rad/s
E) 1.7 rad/s

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
29
A uniform meter stick is pivoted to rotate about a horizontal axis through the 25-cm mark on the stick. The stick is released from rest in a horizontal position. The moment of inertia of a uniform rod about an axis perpendicular to the rod and through the center of mass of the rod is given by (1/12)ML2. Determine the magnitude of the initial angular acceleration of the stick.
A) 17 rad/s2
B) 13 rad/s2
C) 15 rad/s2
D) 19 rad/s2
E) 23 rad/s2
A) 17 rad/s2
B) 13 rad/s2
C) 15 rad/s2
D) 19 rad/s2
E) 23 rad/s2

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
30
A uniform rod of length (L = 2.0 m) and mass (M = 1.5 kg) is pivoted about a horizontal frictionless pin through one end. The rod is released from rest at an angle of 30° below the horizontal. What is the angular speed of the rod when it passes through the vertical position? (The moment of inertia of the rod about the pin is 2.0 kg⋅m2.)
A) 3.5 rad/s
B) 2.7 rad/s
C) 3.1 rad/s
D) 2.3 rad/s
E) 1.6 rad/s
A) 3.5 rad/s
B) 2.7 rad/s
C) 3.1 rad/s
D) 2.3 rad/s
E) 1.6 rad/s

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
31
Four identical particles (mass of each = 0.40 kg) are placed at the vertices of a rectangle (2.5 m × 4.0 m) and held in those positions by four light rods which form the sides of the rectangle. What is the moment of inertia of this rigid body about an axis that passes through the mid-points of the shorter sides and is parallel to the longer sides?
A) 2.2 kg⋅m2
B) 2.8 kg⋅m2
C) 2.5 kg⋅m2
D) 3.1 kg⋅m2
E) 1.6 kg⋅m2
A) 2.2 kg⋅m2
B) 2.8 kg⋅m2
C) 2.5 kg⋅m2
D) 3.1 kg⋅m2
E) 1.6 kg⋅m2

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
32
Four identical particles (mass of each = 0.40 kg) are placed at the vertices of a rectangle (2.0 m × 3.0 m) and held in those positions by four light rods which form the sides of the rectangle. What is the moment of inertia of this rigid body about an axis that passes through the mid-points of the longer sides and is parallel to the shorter sides?
A) 2.7 kg⋅m2
B) 3.6 kg⋅m2
C) 3.1 kg⋅m2
D) 4.1 kg⋅m2
E) 1.6 kg⋅m2
A) 2.7 kg⋅m2
B) 3.6 kg⋅m2
C) 3.1 kg⋅m2
D) 4.1 kg⋅m2
E) 1.6 kg⋅m2

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
33
A uniform rod is 2.0 m long. The rod is pivoted about a horizontal, frictionless pin through one end. The rod is released from rest at an angle of 30° above the horizontal. What is the angular acceleration of the rod at the instant it is released?
A) 4.7 rad/s2
B) 6.9 rad/s2
C) 6.4 rad/s2
D) 5.6 rad/s2
E) 4.2 rad/s2
A) 4.7 rad/s2
B) 6.9 rad/s2
C) 6.4 rad/s2
D) 5.6 rad/s2
E) 4.2 rad/s2

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
34
A uniform rod of mass M = 1.2 kg and length L = 0.80 m, lying on a frictionless horizontal plane, is free to pivot about a vertical axis through one end, as shown. The moment of inertia of the rod about this axis is given by (1/3)ML2. If a force (F = 5.0 N, θ = 40°) acts as shown, what is the resulting angular acceleration about the pivot point? 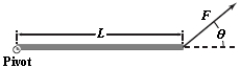
A) 16 rad/s2
B) 12 rad/s2
C) 14 rad/s2
D) 10 rad/s2
E) 33 rad/s2
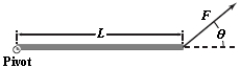
A) 16 rad/s2
B) 12 rad/s2
C) 14 rad/s2
D) 10 rad/s2
E) 33 rad/s2

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
35
A uniform rod (mass = 1.5 kg) is 2.0 m long. The rod is pivoted about a horizontal, frictionless pin through one end. The rod is released from rest in a horizontal position. What is the angular speed of the rod when the rod makes an angle of 30° with the horizontal? (The moment of inertia of the rod about the pin is 2.0 kg⋅m2).
A) 2.2 rad/s
B) 3.6 rad/s
C) 2.7 rad/s
D) 3.1 rad/s
E) 1.8 rad/s
A) 2.2 rad/s
B) 3.6 rad/s
C) 2.7 rad/s
D) 3.1 rad/s
E) 1.8 rad/s

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
36
A uniform rod is 2.0 m long. The rod is pivoted about a horizontal, frictionless pin through one end. The rod is released from rest at the horizontal position. What is the angular acceleration of the rod at the instant the rod makes an angle of 70° with the horizontal?
A) 3.7 rad/s2
B) 1.3 rad/s2
C) 2.5 rad/s2
D) 4.9 rad/s2
E) 1.9 rad/s2
A) 3.7 rad/s2
B) 1.3 rad/s2
C) 2.5 rad/s2
D) 4.9 rad/s2
E) 1.9 rad/s2

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
37
The rigid object shown is rotated about an axis perpendicular to the paper and through point P. The total kinetic energy of the object as it rotates is equal to 1.4 J. If M = 1.3 kg and L = 0.50 m, what is the angular velocity of the object? Neglect the mass of the connecting rods and treat the masses as particles. 
A) 1.3 rad/s
B) 1.5 rad/s
C) 1.7 rad/s
D) 1.2 rad/s
E) 2.1 rad/s

A) 1.3 rad/s
B) 1.5 rad/s
C) 1.7 rad/s
D) 1.2 rad/s
E) 2.1 rad/s

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
38
Two particles (m1 = 0.20 kg, m2 = 0.30 kg) are positioned at the ends of a 2.0-m long rod of negligible mass. What is the moment of inertia of this rigid body about an axis perpendicular to the rod and through the center of mass?
A) 0.48 kg⋅m2
B) 0.50 kg⋅m2
C) 1.2 kg⋅m2
D) 0.80 kg⋅m2
E) 0.70 kg⋅m2
A) 0.48 kg⋅m2
B) 0.50 kg⋅m2
C) 1.2 kg⋅m2
D) 0.80 kg⋅m2
E) 0.70 kg⋅m2

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
39
Four identical particles (mass of each = 0.24 kg) are placed at the vertices of a rectangle (2.0 m × 3.0 m) and held in those positions by four light rods which form the sides of the rectangle. What is the moment of inertia of this rigid body about an axis that passes through the center of mass of the body and is parallel to the shorter sides of the rectangle?
A) 2.4 kg⋅m2
B) 2.2 kg⋅m2
C) 1.9 kg⋅m2
D) 2.7 kg⋅m2
E) 8.6 kg⋅m2
A) 2.4 kg⋅m2
B) 2.2 kg⋅m2
C) 1.9 kg⋅m2
D) 2.7 kg⋅m2
E) 8.6 kg⋅m2

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
40
A uniform rod (mass = 2.0 kg, length = 0.60 m) is free to rotate about a frictionless pivot at one end. The rod is released from rest in the horizontal position. What is the magnitude of the angular acceleration of the rod at the instant it is 60° below the horizontal?
A) 15 rad/s2
B) 12 rad/s2
C) 18 rad/s2
D) 29 rad/s2
E) 23 rad/s2
A) 15 rad/s2
B) 12 rad/s2
C) 18 rad/s2
D) 29 rad/s2
E) 23 rad/s2

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
41
The figure below shows a graph of angular velocity versus time for a woman bicycling around a circular track.  Use this exhibit to answer the following question(s).
Use this exhibit to answer the following question(s).
Refer to Exhibit 10-1. How many revolutions does she complete in the 16 minute period?
A) 8
B) 12
C) 16
D) 20
E) 40
 Use this exhibit to answer the following question(s).
Use this exhibit to answer the following question(s).Refer to Exhibit 10-1. How many revolutions does she complete in the 16 minute period?
A) 8
B) 12
C) 16
D) 20
E) 40

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
42
The angular speed of the minute hand of a clock, in rad/s, is
A)
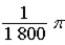 .
.
B)
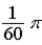 .
.
C)
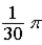 .
.
D) π.
E) 120π.
A)
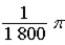 .
.B)
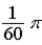 .
.C)
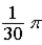 .
.D) π.
E) 120π.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
43
The rigid body shown rotates about an axis through its center of mass and perpendicular to the paper. If M = 2.0 kg and L = 80 cm, what is the kinetic energy of this object when its angular speed about this axis is equal to 5.0 rad/s? Neglect the mass of the connecting rod and treat the masses as particles. 
A) 18 J
B) 15 J
C) 12 J
D) 23 J
E) 26 J

A) 18 J
B) 15 J
C) 12 J
D) 23 J
E) 26 J

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
44
The figure below shows a graph of angular velocity versus time for a woman bicycling around a circular track.  Use this exhibit to answer the following question(s).
Use this exhibit to answer the following question(s).
Refer to Exhibit 10-1. What is her angular displacement (in rad) in the 16 minute period shown in the graph?
A) 0
B) 16π
C) 32π
D) 40π
E) 64π
 Use this exhibit to answer the following question(s).
Use this exhibit to answer the following question(s).Refer to Exhibit 10-1. What is her angular displacement (in rad) in the 16 minute period shown in the graph?
A) 0
B) 16π
C) 32π
D) 40π
E) 64π

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
45
You throw a Frisbee of mass m and radius r so that it is spinning about a horizontal axis perpendicular to the plane of the Frisbee. Ignoring air resistance, the torque exerted about its center of mass by gravity is
A) 0.
B) mgr.
C) 2mgr.
D) a function of the angular velocity.
E) small at first, then increasing as the Frisbee loses the torque given it by your hand.
A) 0.
B) mgr.
C) 2mgr.
D) a function of the angular velocity.
E) small at first, then increasing as the Frisbee loses the torque given it by your hand.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
46
A uniform cylinder of radius R, mass M, and length L rotates freely about a horizontal axis parallel and tangent to the cylinder, as shown below. The moment of inertia of the cylinder about this axis is 
A)
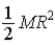 .
.
B)
 .
.
C) MR2.
D)
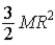 .
.
E)
 .
.

A)
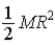 .
.B)
 .
.C) MR2.
D)
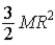 .
.E)
 .
.
Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
47
The figure below shows a graph of angular velocity as a function of time for a car driving around a circular track. Through how many radians does the car travel in the first 10 minutes? 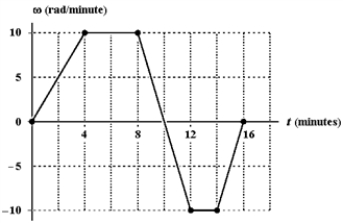
A) 30
B) 50
C) 70
D) 90
E) 100

A) 30
B) 50
C) 70
D) 90
E) 100

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
48
The graphs below show angular velocity as a function of time. In which one is the magnitude of the angular acceleration constantly decreasing?
A)

B)
C)
D)

E)

A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
49
The figure below shows a graph of angular velocity versus time for a woman bicycling around a circular track.  Use this exhibit to answer the following question(s).
Use this exhibit to answer the following question(s).
Refer to Exhibit 10-1. How many revolutions does she complete in the first 12 minutes?
A) 4
B) 8
C) 12
D) 16
E) 32
 Use this exhibit to answer the following question(s).
Use this exhibit to answer the following question(s).Refer to Exhibit 10-1. How many revolutions does she complete in the first 12 minutes?
A) 4
B) 8
C) 12
D) 16
E) 32

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
50
A campus bird spots a member of an opposing football team in an amusement park. The football player is on a ride where he goes around at angular velocity ω at distance R from the center. The bird flies in a horizontal circle above him. Will a dropping the bird releases while flying directly above the person's head hit him?
A) Yes, because it falls straight down.
B) Yes, because it maintains the acceleration of the bird as it falls.
C) No, because it falls straight down and will land behind the person.
D) Yes, because it maintains the angular velocity of the bird as it falls.
E) No, because it maintains the tangential velocity the bird had at the instant it started falling.
A) Yes, because it falls straight down.
B) Yes, because it maintains the acceleration of the bird as it falls.
C) No, because it falls straight down and will land behind the person.
D) Yes, because it maintains the angular velocity of the bird as it falls.
E) No, because it maintains the tangential velocity the bird had at the instant it started falling.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
51
The figure below shows a graph of angular velocity versus time for a woman bicycling around a circular track.  Use this exhibit to answer the following question(s).
Use this exhibit to answer the following question(s).
Refer to Exhibit 10-1. What is her angular displacement (in rad) in the first 12 minutes?
A) 0
B) 2π
C) 4π
D) 16π
E) 32π
 Use this exhibit to answer the following question(s).
Use this exhibit to answer the following question(s).Refer to Exhibit 10-1. What is her angular displacement (in rad) in the first 12 minutes?
A) 0
B) 2π
C) 4π
D) 16π
E) 32π

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
52
Two cylinders made of the same material roll down a plane inclined at an angle θ with the horizontal. Each travels the same distance. The radius of cylinder B is twice the radius of cylinder A. In what order do they reach the bottom?
A) A reaches the bottom first because it has the greater acceleration.
B) A reaches the bottom first because it has a smaller moment of inertia.
C) B reaches the bottom first because is experiences a larger torque.
D) B reaches the bottom first because it travels a larger distance in one rotation.
E) They both reach the bottom at the same time, because each has the same linear acceleration.
A) A reaches the bottom first because it has the greater acceleration.
B) A reaches the bottom first because it has a smaller moment of inertia.
C) B reaches the bottom first because is experiences a larger torque.
D) B reaches the bottom first because it travels a larger distance in one rotation.
E) They both reach the bottom at the same time, because each has the same linear acceleration.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
53
The angular speed of the hour hand of a clock, in rad/s, is
A)
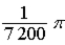 .
.
B)
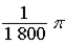 .
.
C)
 .
.
D) 1 800π.
E) 7 200π.
A)
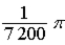 .
.B)
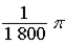 .
.C)
 .
.D) 1 800π.
E) 7 200π.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
54
A uniform sphere of radius R and mass M rotates freely about a horizontal axis that is tangent to an equatorial plane of the sphere, as shown below. The moment of inertia of the sphere about this axis is 
A)
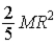 .
.
B)
 .
.
C)
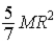 .
.
D)
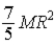 .
.
E)
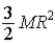 .
.

A)
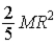 .
.B)
 .
.C)
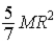 .
.D)
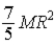 .
.E)
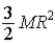 .
.
Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
55
A nonuniform 2.0-kg rod is 2.0 m long. The rod is mounted to rotate freely about a horizontal axis perpendicular to the rod that passes through one end of the rod. The moment of inertia of the rod about this axis is 4.0 kg⋅m2. The center of mass of the rod is 1.2 m from the axis. If the rod is released from rest in the horizontal position, what is its angular speed as it swings through the vertical position?
A) 3.4 rad/s
B) 4.4 rad/s
C) 4.3 rad/s
D) 5.8 rad/s
E) 6.8 rad/s
A) 3.4 rad/s
B) 4.4 rad/s
C) 4.3 rad/s
D) 5.8 rad/s
E) 6.8 rad/s

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
56
Two people are on a ride where the inside cars rotate at constant angular velocity three times the constant angular velocity of the outer cars. If the two cars are in line at t = 0, and moving at 3ω and ω respectively, at what time will they next pass each other?
A) t = 0.
B) t =
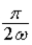 .
.
C) t =
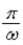 .
.
D) t =
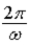 .
.
E) t =
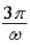 .
.
A) t = 0.
B) t =
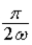 .
.C) t =
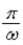 .
.D) t =
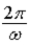 .
.E) t =
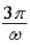 .
.
Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
57
The rigid body shown is rotated about an axis perpendicular to the paper and through the point P. If M = 0.40 kg, a = 30 cm, and b = 50 cm, how much work is required to take the body from rest to an angular speed of 5.0 rad/s? Neglect the mass of the connecting rods and treat the masses as particles. 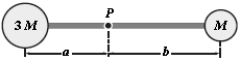
A) 2.9 J
B) 2.6 J
C) 3.1 J
D) 3.4 J
E) 1.6 J

A) 2.9 J
B) 2.6 J
C) 3.1 J
D) 3.4 J
E) 1.6 J

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
58
The figure below shows a graph of angular velocity versus time for a woman bicycling around a circular track.  Use this exhibit to answer the following question(s).
Use this exhibit to answer the following question(s).
Refer to Exhibit 10-1. What is her angular displacement (in rad) in the first 8 minutes?
A) 0
B) π
C) 4π
D) 8π
E) 16π
 Use this exhibit to answer the following question(s).
Use this exhibit to answer the following question(s).Refer to Exhibit 10-1. What is her angular displacement (in rad) in the first 8 minutes?
A) 0
B) π
C) 4π
D) 8π
E) 16π

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
59
A uniform rod (length = 2.4 m) of negligible mass has a 1.0-kg point mass attached to one end and a 2.0-kg point mass attached to the other end. The rod is mounted to rotate freely about a horizontal axis that is perpendicular to the rod and that passes through a point 1.0 m from the 2.0-kg mass. The rod is released from rest when it is horizontal. What is the angular velocity of the rod at the instant the 2.0-kg mass passes through its low point?
A) 1.7 rad/s
B) 2.2 rad/s
C) 2.0 rad/s
D) 1.5 rad/s
E) 3.1 rad/s
A) 1.7 rad/s
B) 2.2 rad/s
C) 2.0 rad/s
D) 1.5 rad/s
E) 3.1 rad/s

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
60
Two forces of magnitude 50 N, as shown in the figure below, act on a cylinder of radius 4 m and mass 6.25 kg. The cylinder, which is initially at rest, sits on a frictionless surface. After 1 second, the velocity and angular velocity of the cylinder in m/s and rad/s are respectively 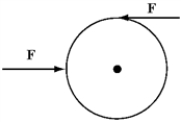
A) v = 0; ω = 0.
B) v = 0; ω = 4.
C) v = 0; ω = 8.
D) v = 8; ω = 8.
E) v = 16; ω = 8.

A) v = 0; ω = 0.
B) v = 0; ω = 4.
C) v = 0; ω = 8.
D) v = 8; ω = 8.
E) v = 16; ω = 8.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
61
The graph below shows a plot of angular acceleration in rad/s2 versus time from t = 0 s to t = 8 s. The change in angular velocity, Δω, during this 8-second period is 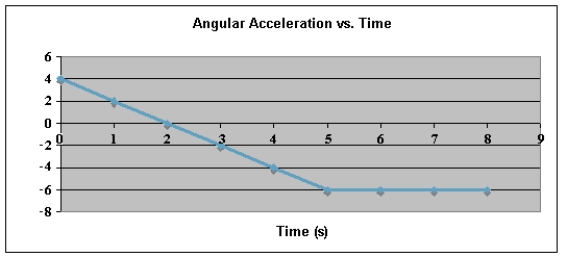
A)
 , CW.
, CW.
B)
 , CCW.
, CCW.
C)
 , CW.
, CW.
D)
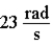 , CCW.
, CCW.
E)
 , CW.
, CW.

A)
 , CW.
, CW.B)
 , CCW.
, CCW.C)
 , CW.
, CW.D)
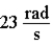 , CCW.
, CCW.E)
 , CW.
, CW.
Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
62
A horizontal force of magnitude 6.5 N is exerted tangentially on a Frisbee of mass 32 grams and radius 14.3 cm. Assuming the Frisbee, a uniform disk, is originally at rest and the force is exerted for 0.08 s, determine the angular velocity of rotation about the central axis when the Frisbee is released.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
63
A solid sphere, a solid cylinder, and a hoop all have the same mass and radius. Each are sent down identical inclined planes starting from rest. Their kinetic energies at the bottom of the incline are Ksphere, Kcylinder, and Khoop. Which of the following is true?
A) Ksphere > Kcylinder
B) Khoop > Ksphere
C) Khoop > Kcylinder
D) Kcylinder > Khoop
E) No answer above is correct.
A) Ksphere > Kcylinder
B) Khoop > Ksphere
C) Khoop > Kcylinder
D) Kcylinder > Khoop
E) No answer above is correct.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
64
The graph below shows a plot of angular acceleration in rad/s2 versus time from t = 0 s to t = 8 s. The angular velocity at t = 0 s is 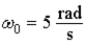 , CCW. The angular velocity, ω, at t = 8 s is
, CCW. The angular velocity, ω, at t = 8 s is 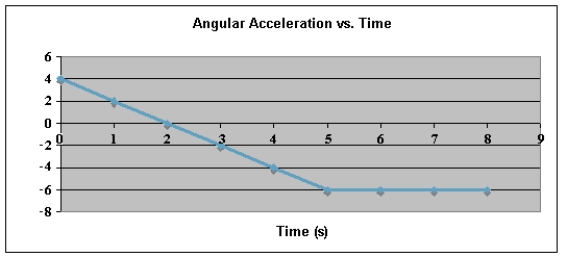
A)
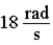 , CW.
, CW.
B)
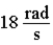 , CCW.
, CCW.
C)
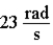 , CW.
, CW.
D)
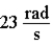 , CCW.
, CCW.
E)
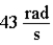 , CW.
, CW.
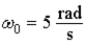 , CCW. The angular velocity, ω, at t = 8 s is
, CCW. The angular velocity, ω, at t = 8 s is 
A)
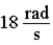 , CW.
, CW.B)
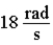 , CCW.
, CCW.C)
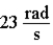 , CW.
, CW.D)
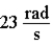 , CCW.
, CCW.E)
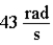 , CW.
, CW.
Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
65
The net work done in accelerating a propeller from rest to an angular velocity of 200 rad/s is 3 000 J. What is the moment of inertia of the propeller?

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
66
A small sphere attached to a light rigid rod rotates about an axis perpendicular to and fixed to the other end of the rod. Relative to the positive direction of the axis of rotation, the angular positions of the sphere are negative, its angular velocity is negative, and its angular acceleration is negative. The sphere is
A) rotating clockwise and slowing down.
B) rotating counterclockwise and slowing down.
C) rotating clockwise and speeding up.
D) rotating counterclockwise and speeding up.
E) first rotating counterclockwise and then clockwise.
A) rotating clockwise and slowing down.
B) rotating counterclockwise and slowing down.
C) rotating clockwise and speeding up.
D) rotating counterclockwise and speeding up.
E) first rotating counterclockwise and then clockwise.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
67
A small sphere attached to a light rigid rod rotates about an axis perpendicular to and fixed to the other end of the rod. Relative to the positive direction of the axis of rotation, the angular positions of the sphere are positive, its angular velocity is positive, and its angular acceleration is negative. The sphere is
A) rotating clockwise and slowing down.
B) rotating counterclockwise and slowing down.
C) rotating clockwise and speeding up.
D) rotating counterclockwise and speeding up.
E) first rotating clockwise and then counterclockwise.
A) rotating clockwise and slowing down.
B) rotating counterclockwise and slowing down.
C) rotating clockwise and speeding up.
D) rotating counterclockwise and speeding up.
E) first rotating clockwise and then counterclockwise.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
68
Exhibit 10-3
The graph below shows a plot of angular velocity in rad/s versus time in s from t = 0 s to t = 7 s.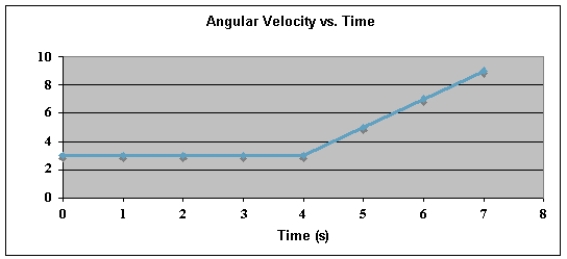 Use this exhibit to answer the following question(s).
Use this exhibit to answer the following question(s).
Refer to Exhibit 10-3. The angular position, θ, at t = 0 s is 3.0 rad, clockwise. The angular position, θ, at t = 7 s is
A) 27 rad, CW.
B) 27 rad, CCW.
C) 33 rad, CW.
D) 33 rad, CCW.
E) 36 rad, CCW.
The graph below shows a plot of angular velocity in rad/s versus time in s from t = 0 s to t = 7 s.
 Use this exhibit to answer the following question(s).
Use this exhibit to answer the following question(s).Refer to Exhibit 10-3. The angular position, θ, at t = 0 s is 3.0 rad, clockwise. The angular position, θ, at t = 7 s is
A) 27 rad, CW.
B) 27 rad, CCW.
C) 33 rad, CW.
D) 33 rad, CCW.
E) 36 rad, CCW.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
69
Exhibit 10-2
The figure below shows a graph of angular velocity versus time for a man bicycling around a circular track.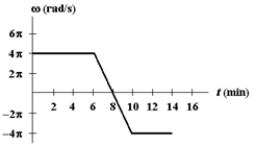 Use this exhibit to answer the following question(s).
Use this exhibit to answer the following question(s).
Refer to Exhibit 10-2. What is his average angular acceleration, in rad/s2, in the first 10 minutes?
A) 0
B)
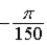
C)
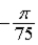
D)
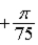
E)
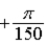
The figure below shows a graph of angular velocity versus time for a man bicycling around a circular track.
 Use this exhibit to answer the following question(s).
Use this exhibit to answer the following question(s).Refer to Exhibit 10-2. What is his average angular acceleration, in rad/s2, in the first 10 minutes?
A) 0
B)
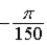
C)
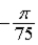
D)
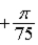
E)
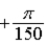

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
70
A small sphere attached to a light rigid rod rotates about an axis perpendicular to and fixed to the other end of the rod. Relative to the positive direction of the axis of rotation, the angular positions of the sphere are negative, its angular velocity is negative, and its angular acceleration is positive. The sphere is
A) rotating clockwise and slowing down.
B) rotating counterclockwise and slowing down.
C) rotating clockwise and speeding up.
D) rotating counterclockwise and speeding up.
E) first rotating counterclockwise and then clockwise.
A) rotating clockwise and slowing down.
B) rotating counterclockwise and slowing down.
C) rotating clockwise and speeding up.
D) rotating counterclockwise and speeding up.
E) first rotating counterclockwise and then clockwise.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
71
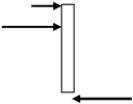
Which of the following diagrams shows the greatest magnitude net torque with a zero net force? All the rods, of length 2r, rotate about an axis that is perpendicular to the rod and fixed in the center of the rod. All the forces are of magnitude F or 2F and all distances from the axis are r or r/2.
A)

B)
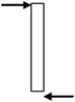
C)
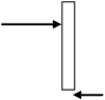
D)

E)
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
72
When a wheel is rolling without slipping, the magnitude of its velocity relative to the ground is greatest at
A) the point in contact with the ground.
B) the point at the center of the wheel.
C) the point at the top of the wheel opposite to the point in contact with the ground.
D) the point farthest forward from the center of mass of the wheel.
E) the point farthest behind the center of mass of the wheel.
A) the point in contact with the ground.
B) the point at the center of the wheel.
C) the point at the top of the wheel opposite to the point in contact with the ground.
D) the point farthest forward from the center of mass of the wheel.
E) the point farthest behind the center of mass of the wheel.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
73
The angular speed of the hour hand of a clock, in rad/min, is
A)
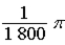 .
.
B)
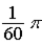 .
.
C)
 .
.
D) π.
E) 120π.
A)
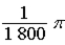 .
.B)
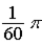 .
.C)
 .
.D) π.
E) 120π.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
74
A solid sphere, a solid cylinder, a spherical shell, and a hoop all have the same mass and radius. Each are rolling on a horizontal surface with the same center of mass speed, and then they roll up identical inclines. Which one goes the greatest distance up its incline?
A) the hoop
B) the solid sphere
C) the spherical shell
D) the cylinder
E) They all go the same distance up their inclines.
A) the hoop
B) the solid sphere
C) the spherical shell
D) the cylinder
E) They all go the same distance up their inclines.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
75
Exhibit 10-2
The figure below shows a graph of angular velocity versus time for a man bicycling around a circular track. Use this exhibit to answer the following question(s).
Use this exhibit to answer the following question(s).
Refer to Exhibit 10-2. What is his average angular acceleration, in rad/s2, in the period from t = 6 min to t = 8 min?
A) 0
B)
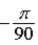
C)
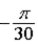
D)
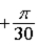
E)

The figure below shows a graph of angular velocity versus time for a man bicycling around a circular track.
 Use this exhibit to answer the following question(s).
Use this exhibit to answer the following question(s).Refer to Exhibit 10-2. What is his average angular acceleration, in rad/s2, in the period from t = 6 min to t = 8 min?
A) 0
B)
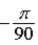
C)
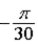
D)
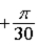
E)


Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
76
A rigid rod of length l rotates about an axis perpendicular to the rod, with one end of the rod fixed to the axis. Which of the following are equal at all points on the rod?
I.The angular position
II.The angular velocity
III.The angular acceleration
IV.The centripetal acceleration
V.The tangential acceleration
A) I and II
B) I, II, and III
C) I, II, III and IV
D) I, II, III, IV and V
E) I, II and IV.
I.The angular position
II.The angular velocity
III.The angular acceleration
IV.The centripetal acceleration
V.The tangential acceleration
A) I and II
B) I, II, and III
C) I, II, III and IV
D) I, II, III, IV and V
E) I, II and IV.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
77
When the sum of the external forces and the sum of the external torques on a body are both zero, we can conclude that
A) the body is moving at constant velocity but is not rotating.
B) the body is rotating at constant angular velocity but has no linear velocity.
C) the body has neither linear nor angular velocity.
D) the body may have constant linear or angular velocity, but not both simultaneously.
E) the body may have constant linear or constant angular velocity, or both simultaneously.
A) the body is moving at constant velocity but is not rotating.
B) the body is rotating at constant angular velocity but has no linear velocity.
C) the body has neither linear nor angular velocity.
D) the body may have constant linear or angular velocity, but not both simultaneously.
E) the body may have constant linear or constant angular velocity, or both simultaneously.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
78
Exhibit 10-3
The graph below shows a plot of angular velocity in rad/s versus time in s from t = 0 s to t = 7 s. Use this exhibit to answer the following question(s).
Use this exhibit to answer the following question(s).
Refer to Exhibit 10-3. The change in angular position, Δθ, during the 7-second period is
A) 21 rad, CW.
B) 21 rad, CCW.
C) 30 rad, CW.
D) 30 rad, CCW.
E) 39 rad, CCW.
The graph below shows a plot of angular velocity in rad/s versus time in s from t = 0 s to t = 7 s.
 Use this exhibit to answer the following question(s).
Use this exhibit to answer the following question(s).Refer to Exhibit 10-3. The change in angular position, Δθ, during the 7-second period is
A) 21 rad, CW.
B) 21 rad, CCW.
C) 30 rad, CW.
D) 30 rad, CCW.
E) 39 rad, CCW.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
79
A celestial object called a pulsar emits its light in short bursts that are synchronized with its rotation. A pulsar in the Crab Nebula is rotating at a rate of 30 revolutions/second. What is the maximum radius of the pulsar, if no part of its surface can move faster than the speed of light (3 × 108 m/s)?

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
80
When the center of a bicycle wheel has linear velocity 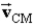 relative to the ground, the velocity relative to the ground of point P' at the top of the wheel is
relative to the ground, the velocity relative to the ground of point P' at the top of the wheel is 
A) 0.
B)
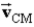 .
.
C)
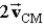 .
.
D)
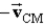 .
.
E)
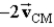 .
.
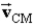 relative to the ground, the velocity relative to the ground of point P' at the top of the wheel is
relative to the ground, the velocity relative to the ground of point P' at the top of the wheel is 
A) 0.
B)
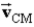 .
.C)
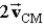 .
.D)
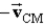 .
.E)
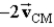 .
.
Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck


