Deck 6: The Normal Probability Distribution
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/196
Play
Full screen (f)
Deck 6: The Normal Probability Distribution
1
In general, the binomial probability P(X  x) is approximated by the area under the normal curve to the right of x - .5.
x) is approximated by the area under the normal curve to the right of x - .5.
 x) is approximated by the area under the normal curve to the right of x - .5.
x) is approximated by the area under the normal curve to the right of x - .5.True
2
The total area under any continuous probability curve is equal to one.
True
3
Adding or subtracting 0.5 from the binomial random variable x's interval endpoints (i.e., the so-called continuity correction) improves the normal approximation to the binomial by correcting for or accounting for the "missing" corners of the probability rectangles corresponding to values of x.
True
4
As a general rule, the normal distribution is used to approximate the sampling distribution of the sample proportion only if:
A) the sample size n is greater than 30
B) the sample size n is greater than 100
C) the population proportion p is close to 0.50
D) the underlying population is normal
E) np and n(1 - p) are both greater than or equal to 5
A) the sample size n is greater than 30
B) the sample size n is greater than 100
C) the population proportion p is close to 0.50
D) the underlying population is normal
E) np and n(1 - p) are both greater than or equal to 5

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
5
Given that X is a binomial random variable, the binomial probability P(X  x) is approximated by the area under a normal curve to the right of:
x) is approximated by the area under a normal curve to the right of:
A) x - 0.5
B) x + 0.5
C) x - 1
D) x + 1
E) x + 0.1
 x) is approximated by the area under a normal curve to the right of:
x) is approximated by the area under a normal curve to the right of:A) x - 0.5
B) x + 0.5
C) x - 1
D) x + 1
E) x + 0.1

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
6
Suppose that the probability p of success on any trail of a binomial distribution equals 0.90. Then for which of the following number of trials, n, would the normal distribution provide a good approximation to the binomial distribution?
A) 25
B) 35
C) 45
D) 55
E) 65
A) 25
B) 35
C) 45
D) 55
E) 65

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
7
Twenty-five psychology instructors have formed a committee to pick next year's textbook, and they have narrowed their decision down to two equally good books, one with a better bibliography and references, and the other with a better format and illustrations. Since the books are considered to be equally good, we will assume the probability an instructor chooses either book is 0.5 and the instructors' decisions are made independently.
Using the binomial distribution, find the probability 15 or more instructors choose the book with the better format and illustrations.
______________
Use the normal approximation to the binomial to find the probability 15 or more instructors choose the book with the better format and illustrations.
______________
Compare the results in parts (a) and (b). Is the normal approximation a good approximation?
______________
Using the binomial distribution, find the probability 15 or more instructors choose the book with the better format and illustrations.
______________
Use the normal approximation to the binomial to find the probability 15 or more instructors choose the book with the better format and illustrations.
______________
Compare the results in parts (a) and (b). Is the normal approximation a good approximation?
______________

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
8
In general, the binomial probability P(X = x) is approximated by the area under a normal curve between x - .5 and x + .5.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
9
Given that X is a binomial random variable, the binomial probability P(X 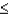 x) is approximated by the area under a normal curve to the left of:
x) is approximated by the area under a normal curve to the left of:
A) x
B) -x
C) x + 0.5
D) x - 0.1
E) -x - 0.1
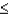 x) is approximated by the area under a normal curve to the left of:
x) is approximated by the area under a normal curve to the left of:A) x
B) -x
C) x + 0.5
D) x - 0.1
E) -x - 0.1

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
10
Consider a binomial random variable x with n = 25 and p = 0.01. Would the normal approximation be appropriate here?
______________
Why or why not?
________________________________________________________
______________
Why or why not?
________________________________________________________

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
11
Past records indicate 60% of the eligible voters in Michigan vote in the primary election.
What is the expected number in a random sample of 200 eligible voters who will vote in the next primary?
______________
What is the approximate probability more than 150 from a sample of 200 will vote in the next primary?
______________
What is the expected number in a random sample of 200 eligible voters who will vote in the next primary?
______________
What is the approximate probability more than 150 from a sample of 200 will vote in the next primary?
______________

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
12
The normal approximation to the binomial distribution works best when the number of trials is large, and when the binomial distribution is symmetrical (like the normal).

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
13
A recent survey of university faculty reported 55% were considering going into another profession. Based on this information, what is the expected number of faculty who would be considering going into another profession if we randomly sampled 200 faculty members?
______________
What is the approximate probability 60 or more faculty from a random sample of 200 would be considering going into another profession?
______________
______________
What is the approximate probability 60 or more faculty from a random sample of 200 would be considering going into another profession?
______________

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
14
All binomial distributions can be approximated very closely by normal distributions.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
15
Even though the normal probability distribution deals with continuous variables, we can use it to approximate the binomial whenever np > 5 and also nq > 5.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
16
Even though the normal probability distribution deals with continuous variables, we can use it to approximate the binomial probability distribution whenever n  30 and also n(q)
30 and also n(q)  30.
30.
 30 and also n(q)
30 and also n(q)  30.
30.
Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
17
Given that X is a binomial random variable, the binomial probability P(X = x) is approximated by the area under a normal curve between:
A) x - 0.5 and 0.0
B) 0.0 and x + 0.5
C) 1 - x and 1 + x
D) x - 0.5 and x + 0.5
E) 0.0 and x + 1
A) x - 0.5 and 0.0
B) 0.0 and x + 0.5
C) 1 - x and 1 + x
D) x - 0.5 and x + 0.5
E) 0.0 and x + 1

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
18
The normal approximation to the binomial is appropriate when the number of trials n is large and the probability of success p is near 0.05.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
19
In general, the binomial probability P(X 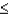 x) is approximated by the area under the normal curve to the left of x + - .5.
x) is approximated by the area under the normal curve to the left of x + - .5.
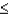 x) is approximated by the area under the normal curve to the left of x + - .5.
x) is approximated by the area under the normal curve to the left of x + - .5.
Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
20
Even though the normal probability distribution deals with continuous variables, under certain circumstances, we can use it to approximate various discrete probability distributions. Such use of the normal probability distribution is permissible:
A) in the case of binomial probabilities, when np > 5 and also nq > 5
B) in the case of Poisson probabilities, when> 100
C) in the case of hypergeometric probabilities, when np > 1 and also n1 > 1
D) in all of these
E) in none of these
A) in the case of binomial probabilities, when np > 5 and also nq > 5
B) in the case of Poisson probabilities, when> 100
C) in the case of hypergeometric probabilities, when np > 1 and also n1 > 1
D) in all of these
E) in none of these

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
21
A normal random variable x is standardized by expressing its value as the number standard deviations  it lies to the right or left of its mean
it lies to the right or left of its mean  .
.
 it lies to the right or left of its mean
it lies to the right or left of its mean  .
.
Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
22
Suppose the records of a motel show that, on the average, 10% of their prospective guests will not claim their reservation. If the motel accepts 218 reservations and there are only 200 rooms in the motel, what is the probability that all guests who arrive to claim a room will receive one?
______________
______________

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
23
Any continuous random variable cannot take on negative values.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
24
A student government representative claims that 55% of the student body of a local university favor a move to Division I in college football. A random sample of 2000 students is selected.
What is the expected number of students that will favor the move to Division I?
______________
What is the approximate probability that less than 1150 students of the 2000 selected will favor the move to Division I?
______________
What is the expected number of students that will favor the move to Division I?
______________
What is the approximate probability that less than 1150 students of the 2000 selected will favor the move to Division I?
______________

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
25
For a continuous random variable x, the probabilities P(0 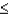 x
x 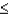 1) and P(0 < x < 1) are always equal.
1) and P(0 < x < 1) are always equal.
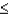 x
x 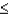 1) and P(0 < x < 1) are always equal.
1) and P(0 < x < 1) are always equal.
Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
26
Let x be a binomial random variable with n = 25 and p = 0.3.
Is the normal approximation appropriate for this binomial random variable?
______________
Find the mean for x.
______________
Find the standard deviation for x.
______________
Use the normal approximation to find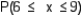 .
.
______________
Use the cumulative binomial probabilities table in Appendix 1 of your book to calculate the exact probability, above.
______________
Compare the results of the last two questions. Was your approximation close, or far, from the exact answer?
______________
Is the normal approximation appropriate for this binomial random variable?
______________
Find the mean for x.
______________
Find the standard deviation for x.
______________
Use the normal approximation to find
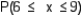 .
.______________
Use the cumulative binomial probabilities table in Appendix 1 of your book to calculate the exact probability, above.
______________
Compare the results of the last two questions. Was your approximation close, or far, from the exact answer?
______________

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
27
The relative frequency associated with a particular class in the population is the fraction of measurements in the population falling in that class.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
28
Historical data collected at First of America Bank in Michigan revealed that 80% of all customers applying for a loan are accepted. Suppose that 50 new loan applications are selected at random.
Find the expected value of the number of loans that will be accepted by the bank.
______________
Find the standard deviation of the number of loans that will be accepted by the bank.
______________
What is the probability that at least 42 loans will be accepted?
______________
What is the probability that the number of loans rejected is between 10 and 15, inclusive?
______________
Find the expected value of the number of loans that will be accepted by the bank.
______________
Find the standard deviation of the number of loans that will be accepted by the bank.
______________
What is the probability that at least 42 loans will be accepted?
______________
What is the probability that the number of loans rejected is between 10 and 15, inclusive?
______________

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
29
The total area under the curve f(x) of any continuous random variable x is equal to one.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
30
In a certain population, 18% of the people have Rh-negative blood. A blood bank serving this population receives 95 blood donors on a particular day.
What is the probability that 10 or fewer are Rh-negative?
______________
What is the probability that 15 to 20 (inclusive) of the donors are Rh-negative?
______________
What is the probability that more than 80 of the donors are Rh-positive?
______________
What is the probability that 10 or fewer are Rh-negative?
______________
What is the probability that 15 to 20 (inclusive) of the donors are Rh-negative?
______________
What is the probability that more than 80 of the donors are Rh-positive?
______________

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
31
Suppose the current median age of U.S. citizens is 30. If a survey of 500 randomly selected U.S. citizens is conducted, what is the probability at most 240 of them will be under 30 years old?
______________
______________

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
32
Do Americans tend to vote for the taller of the two candidates in a presidential election? In 30 of our presidential elections since 1856, 18 of the winners were taller than their opponents. Assume that Americans are not biased by a candidate's height and that the winner is just as likely to be taller or shorter than his opponent. Is the observed number of taller winners in the U.S. presidential elections unusual?
Find the approximate probability of finding 18 or more of the 30 pairs in which the taller candidate wins.
______________
Based on your answer to part (a), can you conclude that Americans consider a candidate's height when casting their ballot?
______________
Find the approximate probability of finding 18 or more of the 30 pairs in which the taller candidate wins.
______________
Based on your answer to part (a), can you conclude that Americans consider a candidate's height when casting their ballot?
______________

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
33
Continuous random variables can assume infinitely many values corresponding to points on a line interval.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
34
Twenty percent of American men over 30 have high blood pressure. To run an experiment, a researcher needs to identify at least 15 men with high blood pressure. If the researcher examines 100 men over 30, what is the approximate probability he or she finds enough men with high blood pressure to run the experiment?
______________
______________

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
35
A firm with many traveling salespersons decides to check the salespersons' travel expenses to see if they are correctly reported. An auditor for the firm selects 200 expense reports at random to audit. What is the probability more than 20% of the sampled reports will be incorrect when, in fact, only 10% of the firm's expense reports are improperly documented?
______________
______________

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
36
For any continuous random variable x, P(x = 5) = 1 is always true.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
37
For a continuous random variable x, P(6 < x < 8) is represented by the area under the probability function over the interval from 6 to 8.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
38
The relative frequency associated with a particular class in the population is the probability of drawing a measurement from that class.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
39
The admissions office of a community college in Indiana is asked to accept deposits from a number of qualified prospective freshmen so that, with probability about 0.95, the size of the freshmen class will be less than or equal to 150. Suppose the applicants constitute a random sample from a population of applicants, 80% of whom would actually enter the freshmen class if accepted.
How many deposits should the admissions counselor accept?
______________
If applicants in the number determined in part (a) are accepted, what is the probability that the freshmen class size will be less than 135?
______________
How many deposits should the admissions counselor accept?
______________
If applicants in the number determined in part (a) are accepted, what is the probability that the freshmen class size will be less than 135?
______________

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
40
The mean, median, and standard deviation of a normally distributed random variable are all at the same position on the horizontal axis since the distribution is symmetric.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
41
Let 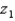 be a z-score that is unknown but identifiable by position and area. If the symmetrical area between -
be a z-score that is unknown but identifiable by position and area. If the symmetrical area between -  and +
and + 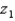 is 0.903, the value of
is 0.903, the value of 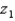 is 1.66.
is 1.66.
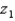 be a z-score that is unknown but identifiable by position and area. If the symmetrical area between -
be a z-score that is unknown but identifiable by position and area. If the symmetrical area between -  and +
and + 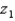 is 0.903, the value of
is 0.903, the value of 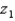 is 1.66.
is 1.66.
Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
42
If x is a normal random variable with mean of 2 and standard deviation of 5, then P(x < 3) = P(x > 7).

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
43
The z-score representing the 10th percentile of the standard normal curve is -1.28.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
44
Given a normal random variable x with mean 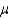 and standard deviation
and standard deviation 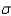 , the mean of the standard normal random variable z associated with x is 1.
, the mean of the standard normal random variable z associated with x is 1.
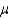 and standard deviation
and standard deviation 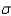 , the mean of the standard normal random variable z associated with x is 1.
, the mean of the standard normal random variable z associated with x is 1.
Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
45
The proportion of the total area under the normal curve that lies within one standard deviation of the mean is approximately 0.75.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
46
Using the standard normal curve, the area between z = -1.50 and z = 1.50 is 0.4332.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
47
Using the standard normal curve, the area between z = 0 and z = 2.2 is 0.4868.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
48
Given a normal random variable x with mean  and standard deviation
and standard deviation 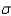 , the standard normal random variable associated with x is
, the standard normal random variable associated with x is 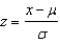 .
.
 and standard deviation
and standard deviation 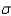 , the standard normal random variable associated with x is
, the standard normal random variable associated with x is 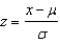 .
.
Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
49
Given that z is a standard normal random variable, a negative value of z indicates that the standard deviation of z is negative.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
50
Let 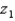 be a z-score that is unknown but identifiable by position and area. If the area to the right of
be a z-score that is unknown but identifiable by position and area. If the area to the right of 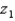 is 0.8849, the value of
is 0.8849, the value of 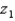 is 1.2.
is 1.2.
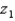 be a z-score that is unknown but identifiable by position and area. If the area to the right of
be a z-score that is unknown but identifiable by position and area. If the area to the right of 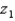 is 0.8849, the value of
is 0.8849, the value of 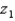 is 1.2.
is 1.2.
Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
51
Given that z is a standard normal random variable, a value of z that is equal to zero indicates that the standard deviation of z is zero also.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
52
Given a normal random variable x with mean 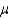 and standard deviation
and standard deviation 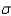 , the variance of the standard normal random variable z associated with x is 0.
, the variance of the standard normal random variable z associated with x is 0.
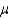 and standard deviation
and standard deviation 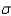 , the variance of the standard normal random variable z associated with x is 0.
, the variance of the standard normal random variable z associated with x is 0.
Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
53
The shape of the normal probability distribution is determined by the population standard deviation 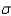 . Small values of
. Small values of 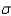 reduce the height of the curve and increase the spread; large values of
reduce the height of the curve and increase the spread; large values of 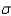 increase the height of the curve and reduce the spread.
increase the height of the curve and reduce the spread.
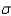 . Small values of
. Small values of 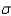 reduce the height of the curve and increase the spread; large values of
reduce the height of the curve and increase the spread; large values of 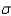 increase the height of the curve and reduce the spread.
increase the height of the curve and reduce the spread.
Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
54
A continuous random variable x is normally distributed with a mean of 1200 and a standard deviation of 150. Given that x = 1410, its corresponding z-score is 1.40.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
55
Given a normal random variable x with mean of 82 and standard deviation of 12, the value of the standard normal random variable z associated with x = 70 is smaller than zero.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
56
Using the standard normal curve, the z-score representing the 95th percentile is 1.645.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
57
The left half of the normal curve is slightly smaller than the right half.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
58
If x is a normal random variable with mean 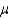 = 4 and standard deviation
= 4 and standard deviation 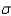 = 2, and Y is a normal random variable with mean
= 2, and Y is a normal random variable with mean 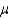 = 10 and standard deviation
= 10 and standard deviation 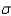 = 5, then P(x < 0) = P(Y < 0).
= 5, then P(x < 0) = P(Y < 0).
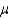 = 4 and standard deviation
= 4 and standard deviation 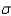 = 2, and Y is a normal random variable with mean
= 2, and Y is a normal random variable with mean 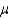 = 10 and standard deviation
= 10 and standard deviation 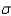 = 5, then P(x < 0) = P(Y < 0).
= 5, then P(x < 0) = P(Y < 0).
Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
59
The normal probability distribution is important because a large number of random variables observed in nature possess a frequency distribution that is approximately a normal probability distribution.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
60
Given a normal random variable x with mean of 70 and standard deviation of 12, the value of the standard normal random variable z associated with x = 82 is larger than zero.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
61
A continuous random variable x is normally distributed with a mean of 100 oz. and a standard deviation of 20 oz. Given that x = 120, its corresponding z-score is -1.0.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
62
The normal random variable's density function is (1) single-peaked above the variable's mean, median, and mode, all of which are equal to one another, (2) perfectly symmetric about this peaked central value and, thus, bell-shaped, and (3) characterized by tails extending indefinitely in both directions from the center, approaching (but never touching) the horizontal axis, which implies a positive probability for finding values of the random variable anywhere between minus infinity and plus infinity.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
63
The mean and variance of any normal distribution are always zero and one, respectively.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
64
For a continuous random variable x, P(x  a) = P(x > a) and P(x
a) = P(x > a) and P(x 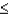 a) = P(x < a).
a) = P(x < a).
 a) = P(x > a) and P(x
a) = P(x > a) and P(x 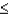 a) = P(x < a).
a) = P(x < a).
Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
65
A standard normal curve is a normal probability density function with a mean of 1 and a standard deviation of 0.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
66
A smooth frequency curve that describes the probability distribution of a continuous random variable is called a standard normal curve.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
67
For any random variable, P(x 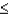 a) = P(x < a) because P(x = a) = 0.
a) = P(x < a) because P(x = a) = 0.
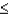 a) = P(x < a) because P(x = a) = 0.
a) = P(x < a) because P(x = a) = 0.
Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
68
One difference between a binomial random variable x and a standard normal random variable z is that with the binomial random variable we cannot determine P(x = a), while for the standard normal variable we can determine P(z = a).

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
69
The z-score representing the 99th percentile of the standard normal curve is 3.0.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
70
The normal probability distribution is right-skewed for large values of the standard deviation 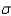 , and is left-skewed for small values of
, and is left-skewed for small values of  .
.
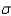 , and is left-skewed for small values of
, and is left-skewed for small values of  .
.
Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
71
For any random variable x, P(x = a) = 0.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
72
The normal probability distribution is one of the most commonly used discrete probability distributions.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
73
Assume that x is a normally distributed random variable with a mean equal to 13.4 and a standard deviation equal to 3.6. Given this information and that P(x > a) = .05, the value of a must be 19.322.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
74
For a continuous random variable, P(x  a) = P(x > a) because P(x = a) = 0.
a) = P(x > a) because P(x = a) = 0.
 a) = P(x > a) because P(x = a) = 0.
a) = P(x > a) because P(x = a) = 0.
Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
75
Continuous random variables can assume values at all points on an interval, with no breaks between possible values.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
76
The possible observations about continuous random variables are infinite in number; characteristics measured in units of money, time, distance, height, volume, length, or weight exemplify the matter.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
77
Examples of continuous probability distributions include the normal probability distribution, the Poisson probability distribution, and the binomial probability distribution.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
78
The total area under the normal probability distribution curve is equal to 1.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
79
If the mean, median, and mode are all equal for a continuous random variable, then the random variable must be normally distributed.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck
80
The time it takes a student to finish a final exam test is known to be normally distributed with a mean equal to 84 minutes with a standard deviation equal to 10 minutes. Given this information, the probability that it will take a randomly selected student between 75 and 90 minutes is approximately .0902.

Unlock Deck
Unlock for access to all 196 flashcards in this deck.
Unlock Deck
k this deck


