Deck 11: Angular Kinematics of Human Movement
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/20
Play
Full screen (f)
Deck 11: Angular Kinematics of Human Movement
1
A 1.2 m golf club is swung in a planar motion by a right-handed golfer with an arm length of 0.76 m. If the initial velocity of the golf ball is 35 m/s, what was the angular velocity of the left shoulder at the point of ball contact? (Assume that the left arm and the club form a straight line and that the initial velocity of the ball is the same as the linear
First, consider the golfer's arm and club as one rigid segment. Add the length of the club and the arm together:
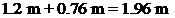 So consider the golf ball as being hit by one 1.96 arm.
So consider the golf ball as being hit by one 1.96 arm.
Next, remember the relationship between angular and linear velocity. The equation for converting angular velocity to linear velocity is v = r?, where v is the linear velocity, r is the radius of rotation for that point, and ? is the angular velocity:
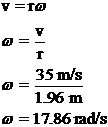 The angular velocity of the shoulder at the moment of contact with the ball will be 17.86 rad/s.
The angular velocity of the shoulder at the moment of contact with the ball will be 17.86 rad/s.
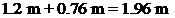 So consider the golf ball as being hit by one 1.96 arm.
So consider the golf ball as being hit by one 1.96 arm.Next, remember the relationship between angular and linear velocity. The equation for converting angular velocity to linear velocity is v = r?, where v is the linear velocity, r is the radius of rotation for that point, and ? is the angular velocity:
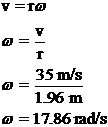 The angular velocity of the shoulder at the moment of contact with the ball will be 17.86 rad/s.
The angular velocity of the shoulder at the moment of contact with the ball will be 17.86 rad/s. 2
The relative angle at the knee changes from 0° to 85° during the knee flexion phase of a squat exercise. If 10 complete squats are performed, what is the total angular distance and the total angular displacement undergone at the knee? (Provide answers in both degrees and radians.) (Answer: ? = 1700°, 29.7 rad; ? = 0)
To find the total angular distance of the knee, first realize that to squat down and then back up, the knee must travel through 85º of flexion to go down, then through another 85º of extension to return to s standing posture. Therefore, the actual angular distance of the squat is 170º. So for ten squats, the knee would go through the full 170º range of motion ten times:
170º × 10 = 1700º
So the angular distance, ?, is equal to 1700º.
To convert this distance into displacement, remember that 2? radians is equal to 360º, or one radian is equal to 57.3º. Thus:
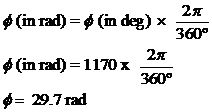 The angular distance is also 29.7 radians.
The angular distance is also 29.7 radians.
Finally, remember that the angular kinematic qualities such as displacement, velocity, and acceleration have the same interrelationships as their linear counterparts. Thus, because angular displacement is equal to angular distance divided by time, the angular displacement of the knee will be zero, because the knee's final position will be the same as it's initial position.
170º × 10 = 1700º
So the angular distance, ?, is equal to 1700º.
To convert this distance into displacement, remember that 2? radians is equal to 360º, or one radian is equal to 57.3º. Thus:
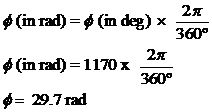 The angular distance is also 29.7 radians.
The angular distance is also 29.7 radians. Finally, remember that the angular kinematic qualities such as displacement, velocity, and acceleration have the same interrelationships as their linear counterparts. Thus, because angular displacement is equal to angular distance divided by time, the angular displacement of the knee will be zero, because the knee's final position will be the same as it's initial position.
3
David is fighting Goliath. If David's 0.75 m sling is accelerated for 1.5 s at 20 rad/s 2 , what will be the initial velocity of the projected stone? (22.5 m/s)
To solve this problem, first convert the given angular acceleration (? = 20 rad/s 2 ) into an angular velocity (?). Remember that the relationships between angular kinematic quantities and linear kinematic quantities are the same, so angular acceleration is just angular velocity over time (t):
 So the angular velocity is 30 rad/s.
So the angular velocity is 30 rad/s.
Next, use the equation relating angular and linear velocity,v = r?, where v is linear velocity, r is the radius of rotation (0.75 m), and ? is the angular velocity, to find what the linear velocity of the stone is after it leaves the sling:
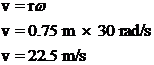 So, after leaving the sling, David's stone will travel toward Goliath with a linear velocity of 22.5 m/s.
So, after leaving the sling, David's stone will travel toward Goliath with a linear velocity of 22.5 m/s.
 So the angular velocity is 30 rad/s.
So the angular velocity is 30 rad/s. Next, use the equation relating angular and linear velocity,v = r?, where v is linear velocity, r is the radius of rotation (0.75 m), and ? is the angular velocity, to find what the linear velocity of the stone is after it leaves the sling:
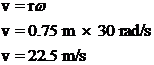 So, after leaving the sling, David's stone will travel toward Goliath with a linear velocity of 22.5 m/s.
So, after leaving the sling, David's stone will travel toward Goliath with a linear velocity of 22.5 m/s. 4
Identify the angular displacement, the angular velocity, and the angular acceleration of the second hand on a clock over the time interval in which it moves from the number 12 to the number 6. Provide answers in both degree- and radian-based units.

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
5
A baseball is struck by a bat 46 cm away from the axis of rotation when the angular velocity of the bat is 70 rad/s. If the ball is hit at a height of 1.2 m at a 45° angle, will the ball clear a 1.2 m fence 110 m away? (Assume that the initial linear velocity of the ball is the same as the linear velocity of the bat at the point at which it is struck.) (No, the ball will fall through a height of 1.2 m at a distance of 105.7 m.)

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
6
How many revolutions are completed by a top spinning with a constant angular velocity of 3 ? rad/s during a 20-second time interval? (Answer: 30 rev)

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
7
A polo player's arm and stick form a 2.5 m rigid segment. If the arm and stick are swung with an angular speed of 1.0 rad/s as the player's horse gallops at 5 m/s, what is the resultant velocity of a motionless ball that is struck head?on? (Assume that ball velocity is the same as the linear velocity of the end of the stick.) (7.5 m/s)

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
8
A kicker's extended leg is swung for 0.4 seconds in a counterclockwise direction while accelerating at 200 deg/s 2. What is the angular velocity of the leg at the instant of contact with the ball? (Answer: 80 deg/s, 1.4 rad/s)t = 0.4 s?? = 200 deg/s 2 ?? = ?
? = ?/t
200 deg/s 2 = ?/0.4 s
? = 80 deg/s
80 deg/s X (1 rad/ 57.3) deg = 1.4 rad/s
? = ?/t
200 deg/s 2 = ?/0.4 s
? = 80 deg/s
80 deg/s X (1 rad/ 57.3) deg = 1.4 rad/s

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
9
Explain how the velocity of the ball in Problem 4 would differ if the stick were swung at a 30° angle to the direction of motion of the horse.

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
10
The angular velocity of a runner's thigh changes from 3 rad/s to 2.7 rad/s during a 0.5 second time period. What has been the average angular acceleration of the thigh? (Answer: -0.6 rad/s 2 , -34.4 deg/s 2 )? 1 = 3 rad/s?? 2 = 2.7 rad/s?t = 0.5 s
? = ? 2 - ? 1 /t
? = (2.7 rad/s - 3 rad/s)/ 0.5 s
? = -0.6 rad/s 2
-0.6 rad/s 2 X 57.3 deg/rad = -34.4 deg/s 2
? = ? 2 - ? 1 /t
? = (2.7 rad/s - 3 rad/s)/ 0.5 s
? = -0.6 rad/s 2
-0.6 rad/s 2 X 57.3 deg/rad = -34.4 deg/s 2

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
11
List three movements for which a relative angle at a particular joint is important and three movements for which the absolute angle of a body segment is important. Explain your choices.

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
12
Identify three movements during which the instantaneous angular velocity at a particular time is the quantity of interest. Explain your choices.

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
13
A majorette in the Rose Bowl Parade tosses a baton into the air with an initial angular velocity of 2.5 rev/s. If the baton undergoes a constant acceleration while airborne of ?0.2 rev/s 2 , and its angular velocity is 0.8 rev/s when the majorette catches it, how many revolutions did it make in the air? (14 rev)
An angular version of one of the equations of constant acceleration may be used:
? 2 2 = ? 1 2 + 2???or?? = (? 2 2 - ? 1 2 ) / 2?
? = ((0.8 rev/s) 2 - (2.5 rev/s) 2 ) / 2(-0.2 rev/s 2 )? = 14 rev
An angular version of one of the equations of constant acceleration may be used:
? 2 2 = ? 1 2 + 2???or?? = (? 2 2 - ? 1 2 ) / 2?
? = ((0.8 rev/s) 2 - (2.5 rev/s) 2 ) / 2(-0.2 rev/s 2 )? = 14 rev

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
14
Fill in the missing corresponding values of angular measure in the table below.
1 rad = 57.3° = ?1 rev = 360° = 2 ? rad
1 rad = 57.3° = ?1 rev = 360° = 2 ? rad

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
15
A cyclist enters a curve of 30 m radius at a speed of 12 m/s. As the brakes are applied, speed is decreased at a constant rate of 0.5 m/s 2. What are the magnitudes of the cyclist's radial and tangential accelerations when his speed is 10 m/s? (a r = 3.33 m/s 2 ,

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
16
Measure and record the following angles for the drawing shown below:
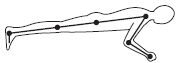
a. The relative angle at the shoulder
b. The relative angle at the elbow
c. The absolute angle of the upper arm
d. The absolute angle of the forearm
Use the right horizontal as your reference for the absolute angles.

a. The relative angle at the shoulder
b. The relative angle at the elbow
c. The absolute angle of the upper arm
d. The absolute angle of the forearm
Use the right horizontal as your reference for the absolute angles.

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
17
A hammer is being accelerated at 15 rad/s 2. Given a radius of rotation of 1.7 m, what are the magnitudes of the radial and tangential components of acceleration when tangential hammer speed is 25 m/s? (a r = 367.6 m/s 2 , a t = 25.5 m/s 2 )

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
18
Calculate the following quantities for the diagram shown below:
a. The angular velocity at the hip over each time interval
b. The angular velocity at the knee over each time interval
Would it provide meaningful information to calculate the average angular velocities at the hip and knee for the movement shown? Provide a rationale for your answer.
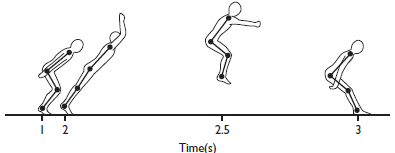
a. The angular velocity at the hip over each time interval
b. The angular velocity at the knee over each time interval
Would it provide meaningful information to calculate the average angular velocities at the hip and knee for the movement shown? Provide a rationale for your answer.


Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
19
An ice skater increases her speed from 10 m/s to 12.5 m/s over a period of 3 s while coming out of a curve of 20 m radius. What are the magnitudes of her radial, tangential, and total accelerations as she leaves the curve. (Remember that a r and a t are the vector components of total acceleration.) (a r = 7.81 m/s 2 , a t = 0.83 m/s 2 , a = 7.85 m/s 2 )

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
20
A tennis racquet swung with an angular velocity of 12 rad/s strikes a motionless ball at a distance of 0.5 m from the axis of rotation. What is the linear velocity of the racquet at the point of contact with the ball?

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck


