Deck 10: Conics and Calculus
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/120
Play
Full screen (f)
Deck 10: Conics and Calculus
1
an equation of the hyperbola with vertices 
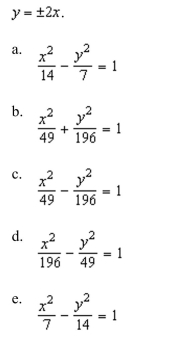

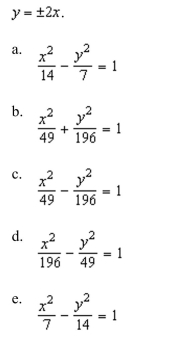
C
2
the center, foci, vertices, and eccentricity of the ellipse. 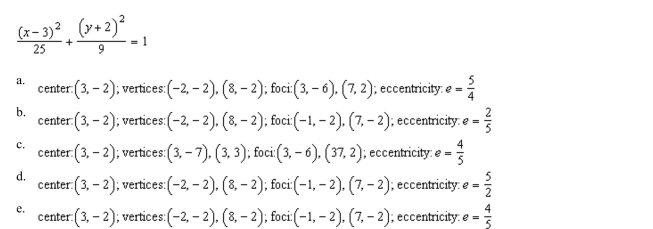
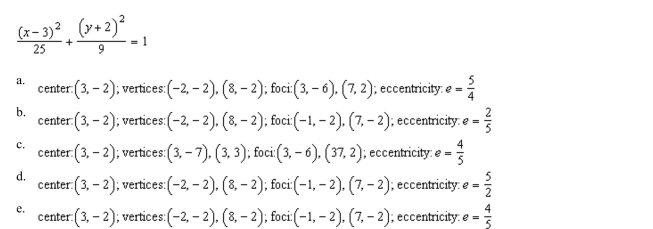
E
3
an equation of the ellipse with vertices 
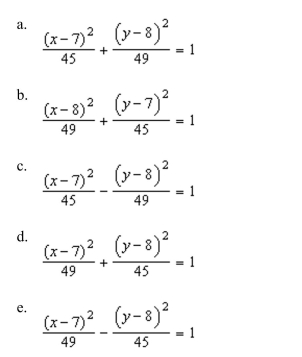

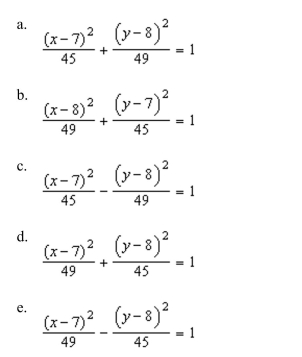
D
4
the focus of the parabola given by 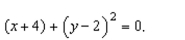

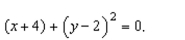


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
5



Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
6
Classify the graph of the equation as a circle, a parabola, an ellipse, or a hyperbola. 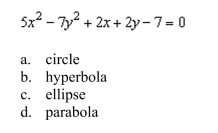
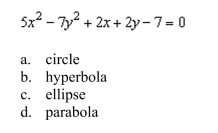

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
7
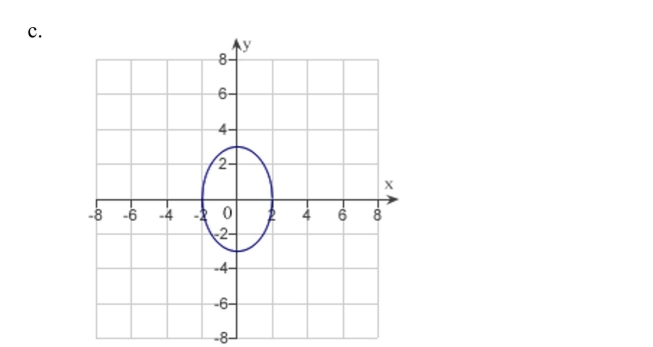
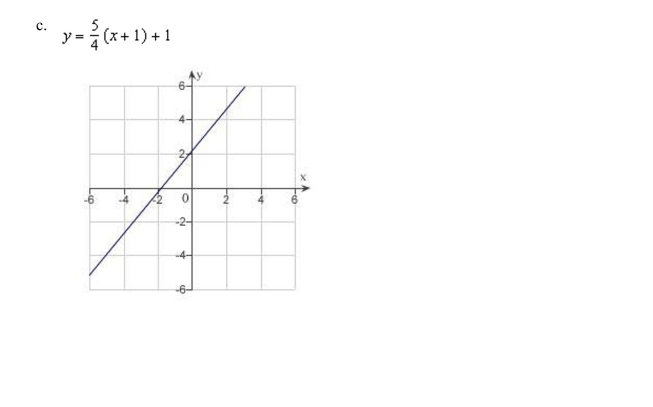
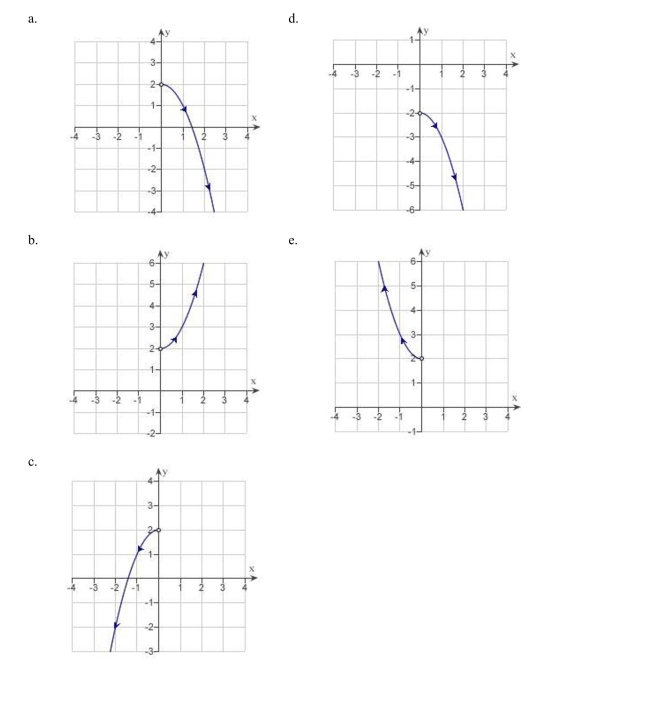
Match the equation with its graph. 



Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
8
Classify the graph of the equation as a circle, a parabola, an ellipse, or a hyperbola. 


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
9
the center of the ellipse given by 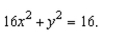

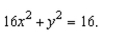


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
10
the vertices of the ellipse given by 


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
11
Match the equation with its graph. 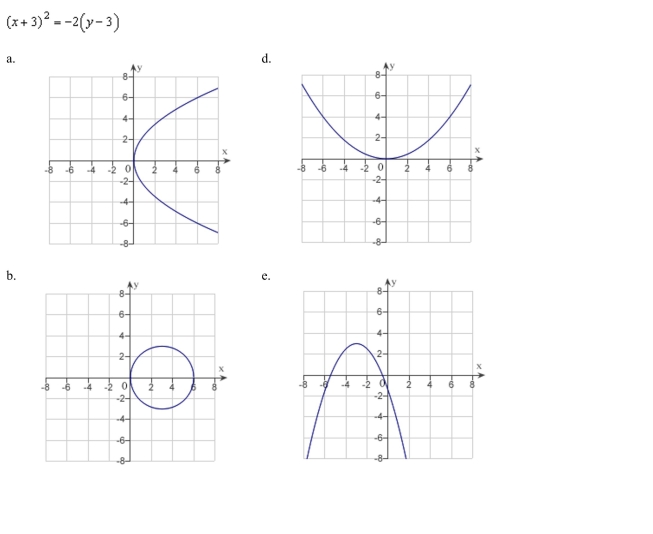
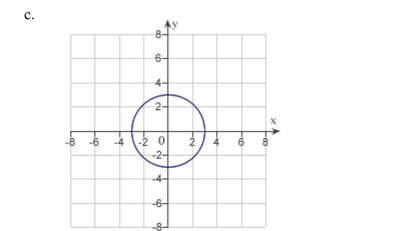



Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
12
the vertex, focus, and directrix of the parabola and sketch its graph. 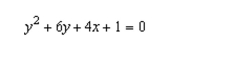

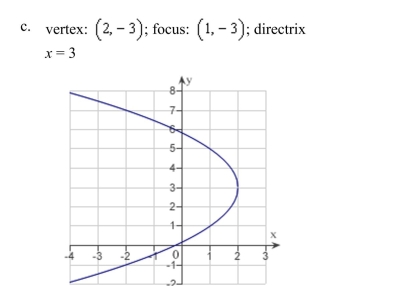
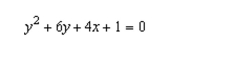



Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
13
Match the equation with its graph. 




Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
14
the foci of the ellipse given by 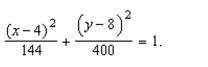

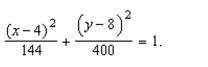


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
15
the directrix of the parabola given by 
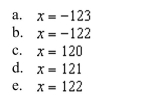

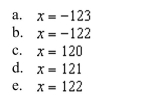

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
16
the vertex of the parabola given by 


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
17


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
18
Match the equation with its graph. 
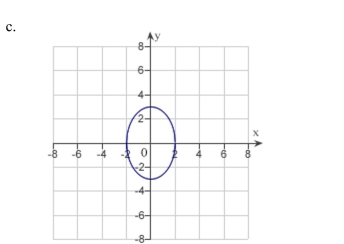
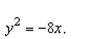


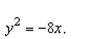

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
19
the eccentricity of the ellipse given by 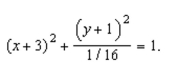
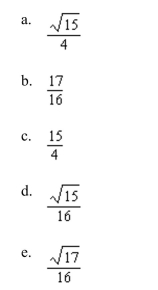
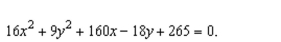
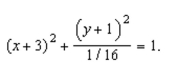
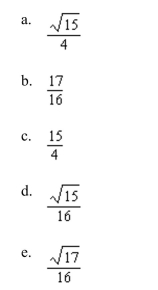
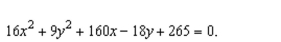

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
20
the center, foci, and vertices of the hyperbola. 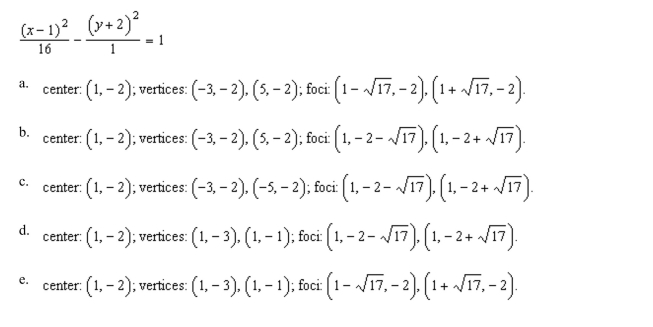
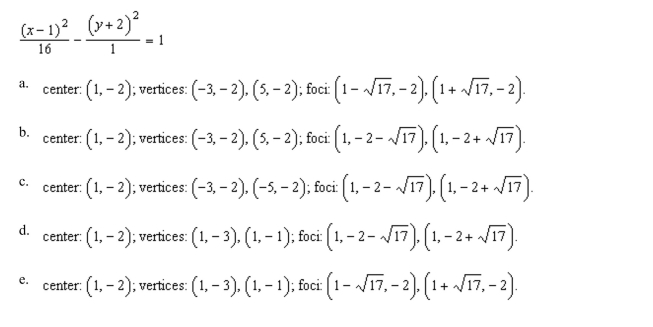

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
21
the result, "the set of parametric equations for the ellipse is 


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
22
the result, "the set of parametric equations for the circle is 


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
23
a set of parametric equations for the rectangular equation 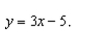
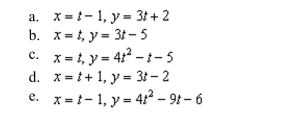
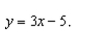
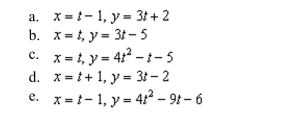

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
24
Sketch the curve represented by the parametric equations 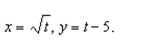
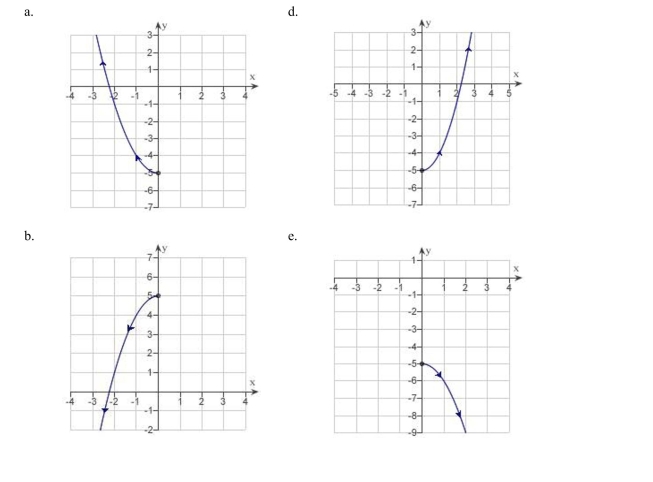

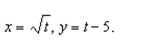



Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
25
Suppose a cable of a suspension bridge is suspended (in the shape of a parabola) between two towers that are 240 meters apart and 40 meters above the roadway as shown in the figure
Given below. The cable touches the roadway midway between the towers. Find the length of the
Parabolic supporting cable. Round your answer to two decimal places.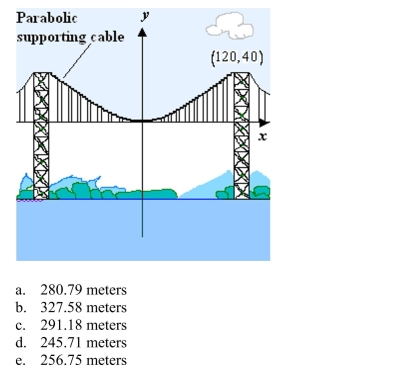
Given below. The cable touches the roadway midway between the towers. Find the length of the
Parabolic supporting cable. Round your answer to two decimal places.


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
26
the result, "the set of parametric equations for the line passing through 




Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
27
Classify the graph of the equation as a circle, a parabola, an ellipse, or a hyperbola. 


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
28
Write the corresponding rectangular equation for the curve represented by the parametric equations 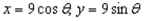 by eliminating the parameter.
by eliminating the parameter. 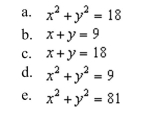
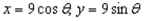 by eliminating the parameter.
by eliminating the parameter. 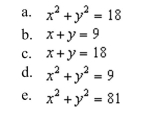

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
29
Write the corresponding rectangular equation for the curve represented by the parametric equation  by eliminating the parameter.
by eliminating the parameter. 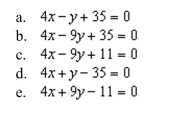
 by eliminating the parameter.
by eliminating the parameter. 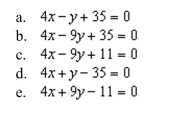

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
30
Suppose a cable of a suspension bridge is suspended (in the shape of a parabola) between two towers that are 240 meters apart and 40 meters above the roadway as shown in the figure
Given below. The cable touches the roadway midway between the towers. Find an equation for the
Parabolic shape of cable.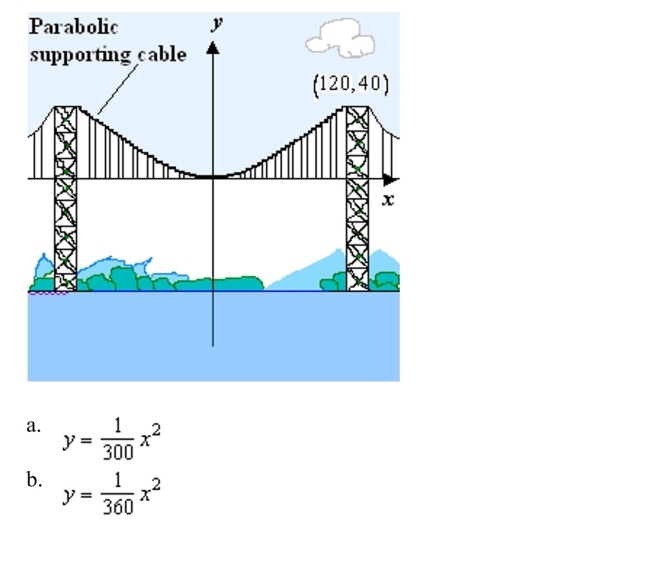
Given below. The cable touches the roadway midway between the towers. Find an equation for the
Parabolic shape of cable.


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
31
Sketch the curve represented by the parametric equations, and write the corresponding rectangular equation by eliminating the parameter. 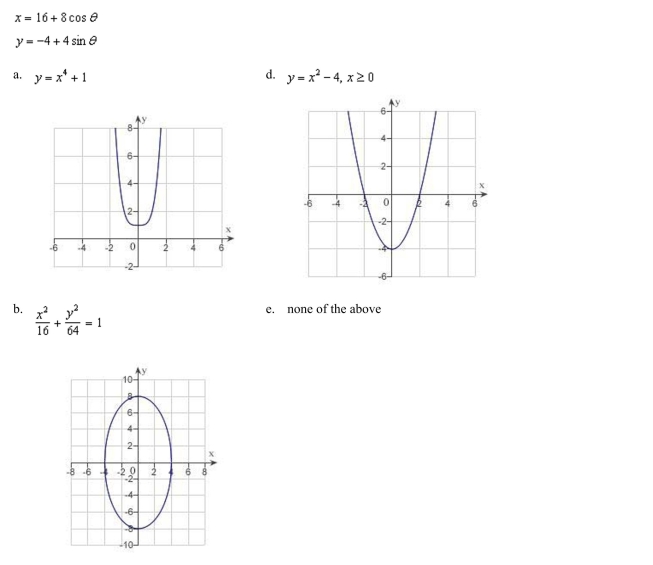



Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
32
Earth moves in an elliptical orbit with the sun at one of the foci. The length of the half of the major axis is 149,598,000 kilometers, and the eccentricity is 0.0167. Find the maximum
Distance (aphelion) of Earth from the sun. Round your answer to nearest kilometer.
A)146,606,040 km
B)2,498,287 km
C)152,589,960 km
D)152,096,287 km
E)147,099,713 km
Distance (aphelion) of Earth from the sun. Round your answer to nearest kilometer.
A)146,606,040 km
B)2,498,287 km
C)152,589,960 km
D)152,096,287 km
E)147,099,713 km

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
33
a set of parametric equations for the rectangular equation 



Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
34
Sketch the curve represented by the parametric equations 



Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
35
a set of parametric equations for the rectangular equation 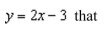
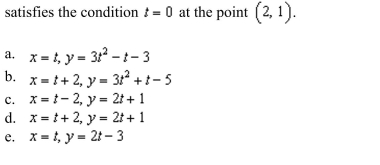
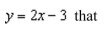
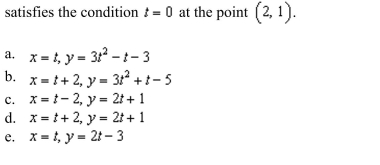

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
36
Write the corresponding rectangular equation for the curve represented by the parametric equations 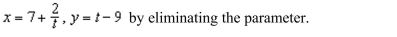
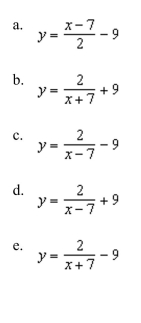
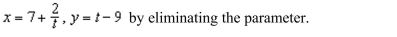
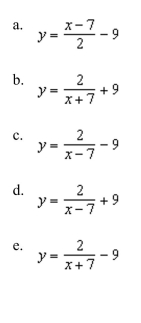

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
37
Uranus moves in an elliptical orbit with the sun at one of the foci. The length of the half of the major axis is 2,876,769,540 kilometers, and the eccentricity is 0.0444. Find the minimum
Distance (perihelion) of Uranus from the sun. Round your answer to nearest kilometer.
A)3,004,498,108 km
B)2,749,040,972 km
C)2,819,234,149 km
D)127,728,568 km
E)2,934,304,931 km
Distance (perihelion) of Uranus from the sun. Round your answer to nearest kilometer.
A)3,004,498,108 km
B)2,749,040,972 km
C)2,819,234,149 km
D)127,728,568 km
E)2,934,304,931 km

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
38
Classify the graph of the equation as a circle, a parabola, an ellipse, or a hyperbola. 


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
39
Suppose a church window is bounded above by a parabola and below by the arc of a circle as shown in figure given below. Find the surface area of the window. Round your answer to
Three decimal places.
Three decimal places.


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
40
Sketch the curve represented by the parametric equations, and write the corresponding rectangular equation by eliminating the parameter. 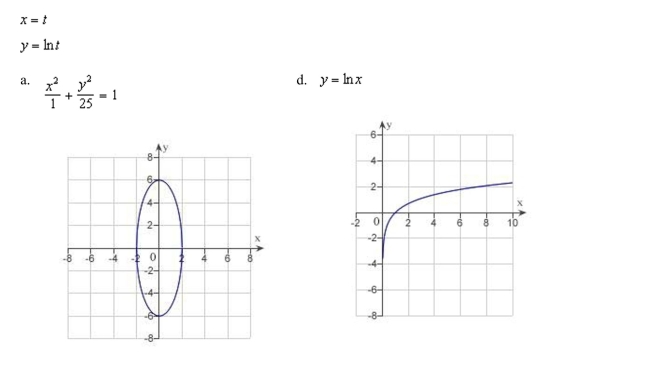




Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
41
the arc length of the curve on the given interval. 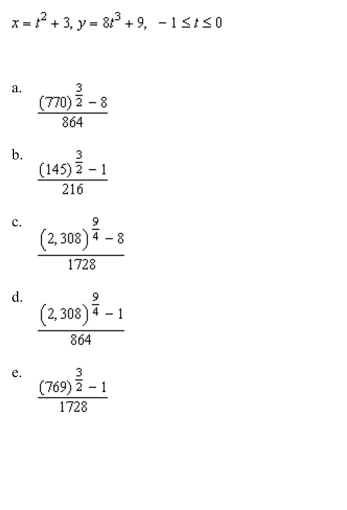
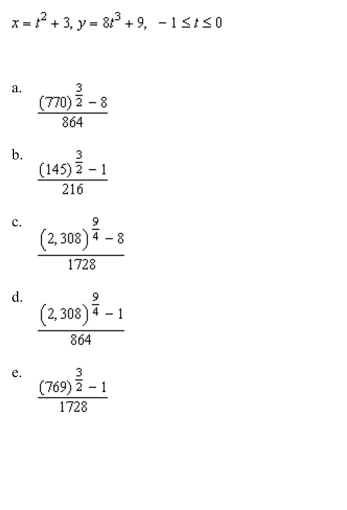

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
42
the arc length of the curve 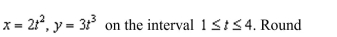 your answer to three decimal places.
your answer to three decimal places.
A)287.453
B)191.635
C)193.606
D)66.480
E)99.721
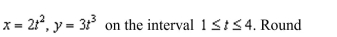 your answer to three decimal places.
your answer to three decimal places.A)287.453
B)191.635
C)193.606
D)66.480
E)99.721

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
43
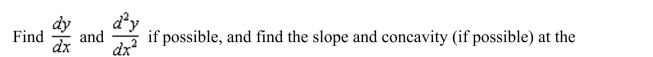
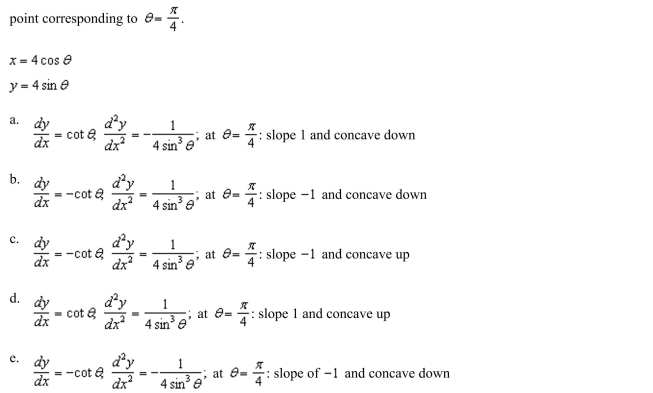

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
44
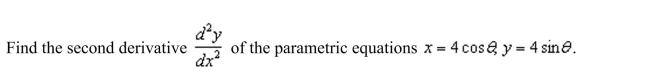


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
45
the arc length of the curve on the given interval. 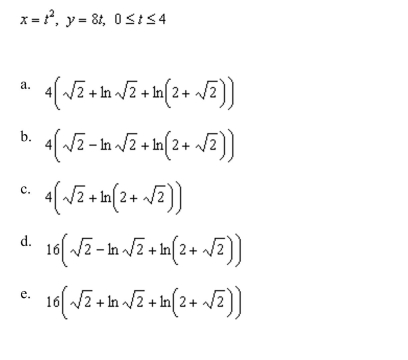
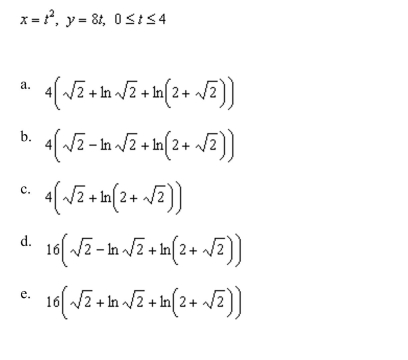

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
46

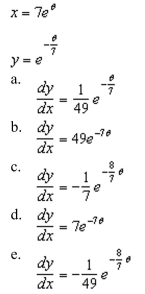

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
47
 Round your answer to two decimal places, if necessary.
Round your answer to two decimal places, if necessary. 

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
48
the arc length of the curve on the given interval. 
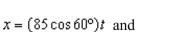

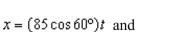

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
49
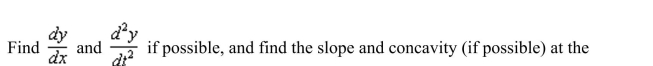 point corresponding to t = 5.
point corresponding to t = 5. 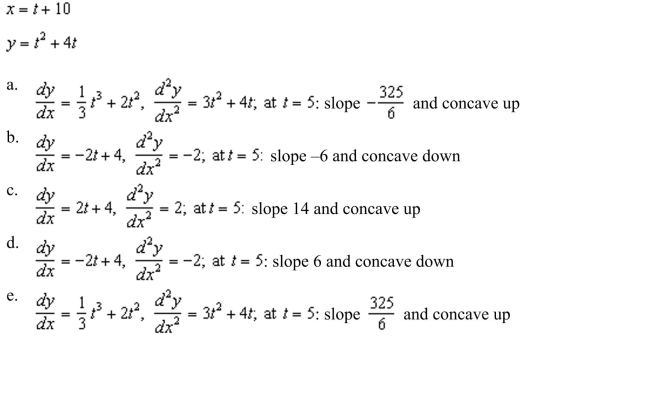

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
50
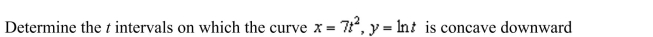 or concave upward.
or concave upward. 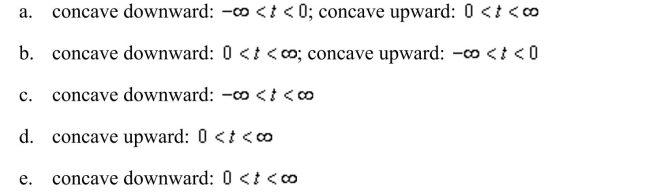

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
51
parametric equations for the path of a projectile launched at a height h feet above 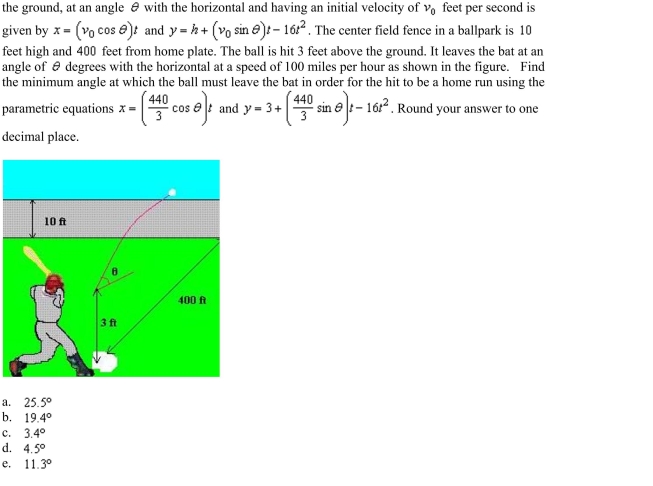


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
52

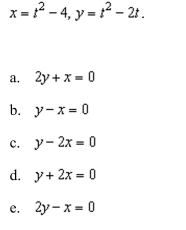

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
53
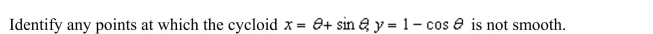
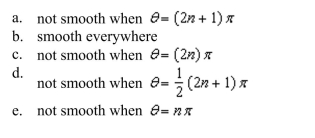

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
54
Determine the t intervals on which the curve  downward or concave upward.
downward or concave upward. 
 downward or concave upward.
downward or concave upward. 

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
55
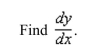
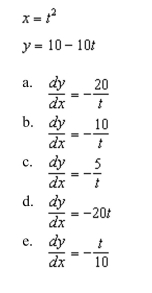


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
56
Identify any points at which the Folium of Descartes  smooth. Round your answer to two decimal places, if necessary.
smooth. Round your answer to two decimal places, if necessary. 
 smooth. Round your answer to two decimal places, if necessary.
smooth. Round your answer to two decimal places, if necessary. 

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
57
parametric equations for the path of a projectile launched at a height h feet above 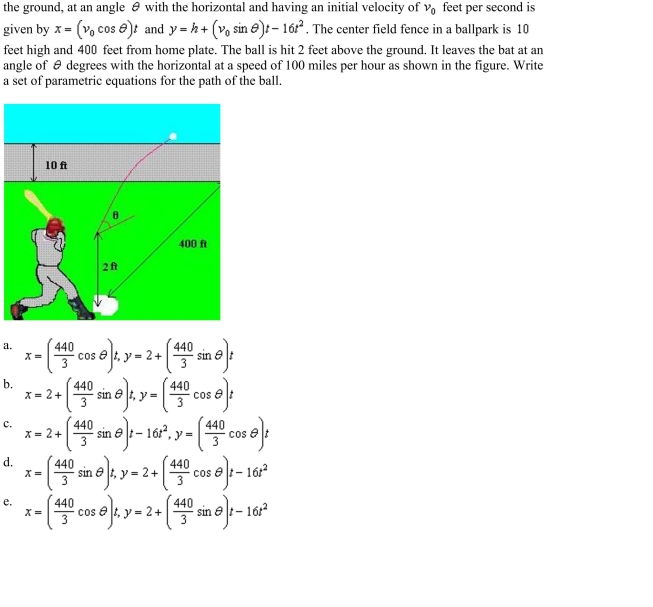


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
58
all points (if any) of horizontal and vertical tangency to the curve 


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
59
all points (if any) of horizontal and vertical tangency to the curve 


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
60
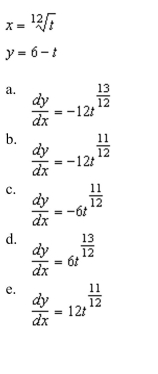

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
61
 Round your answer to two decimal places, if necessary.
Round your answer to two decimal places, if necessary. 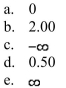

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
62

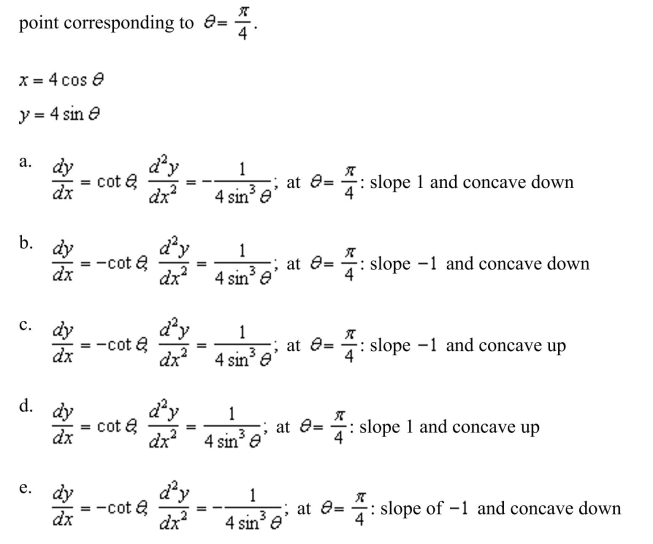

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
63
the arc length of the curve  your answer to three decimal places.
your answer to three decimal places.
A)287.453
B)191.635
C)193.606
D)66.480
E)99.721
 your answer to three decimal places.
your answer to three decimal places.A)287.453
B)191.635
C)193.606
D)66.480
E)99.721

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
64
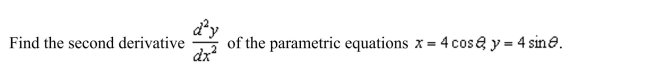
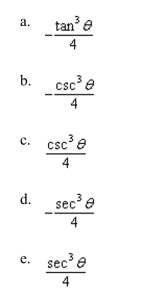

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
65
all points (if any) of horizontal and vertical tangency to the curve 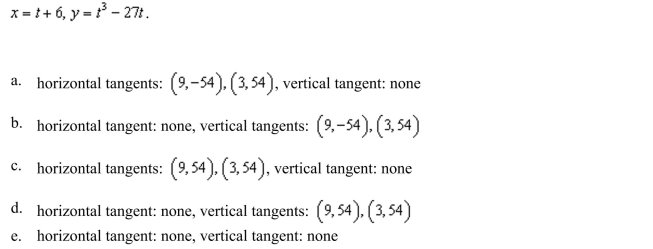


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
66
the area of the surface generated by revolving the curve about the given axis. 


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
67
the area of the surface generated by revolving the curve 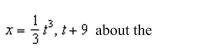

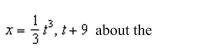


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
68
Determine the t intervals on which the curve  or concave upward.
or concave upward. 
 or concave upward.
or concave upward. 

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
69
the arc length of the curve on the given interval. 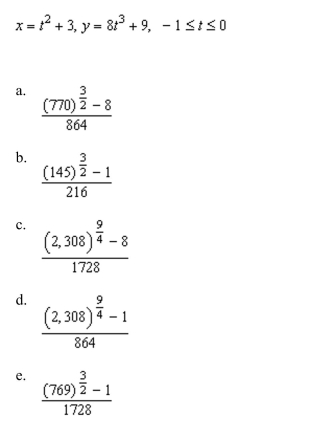
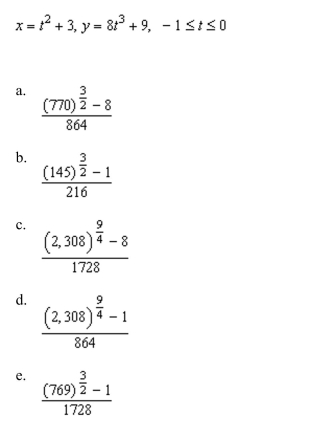

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
70
an equation of the tangent line at a point 
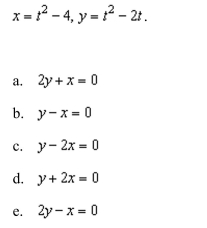

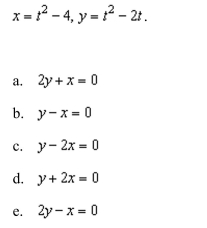

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
71

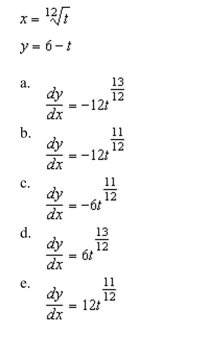

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
72
all points (if any) of horizontal and vertical tangency to the curve 


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
73
path of a projectile is modeled by the parametric equations 


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
74
Determine the t intervals on which the curve 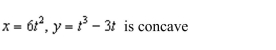 downward or concave upward.
downward or concave upward. 
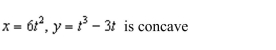 downward or concave upward.
downward or concave upward. 

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
75
the arc length of the curve on the given interval. 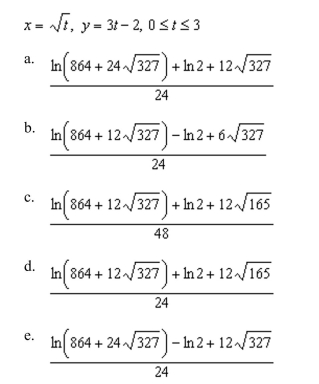


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
76
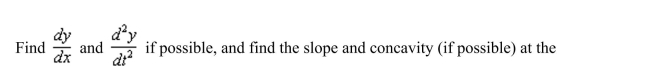 point corresponding to t = 5.
point corresponding to t = 5. 

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
77
the area of the surface generated by revolving the curve about the given axis. 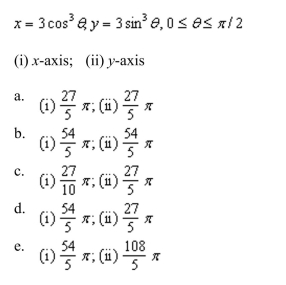

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
78
the arc length of the curve on the given interval. 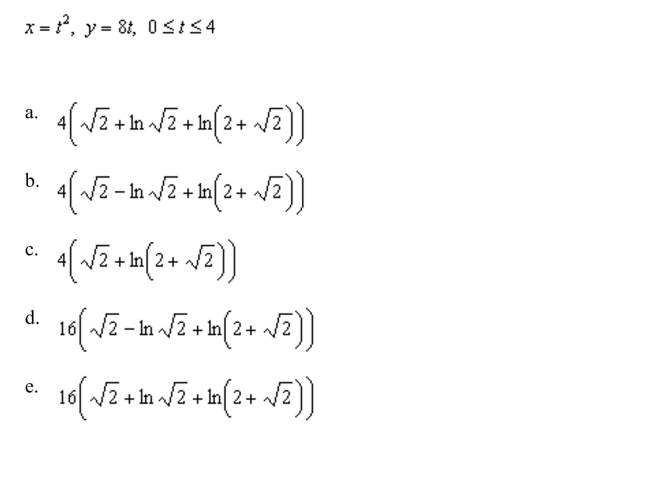
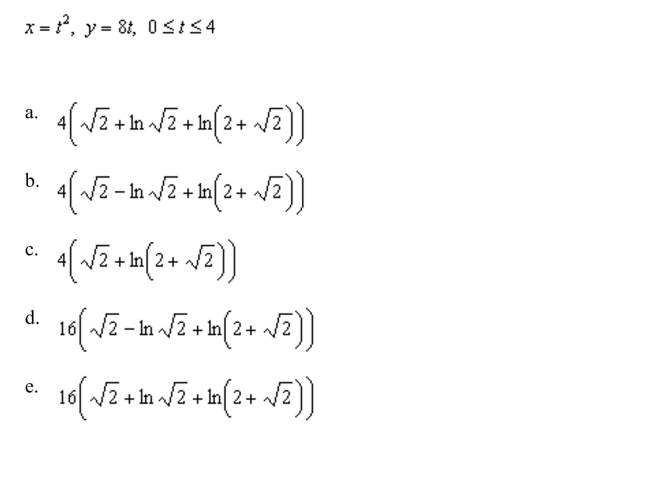

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
79
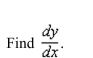
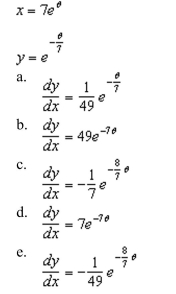

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
80
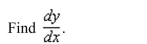
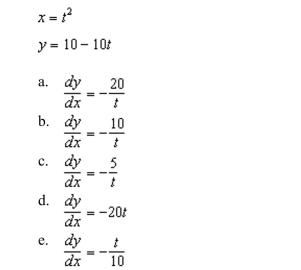

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck


