Deck 2: Sets
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/73
Play
Full screen (f)
Deck 2: Sets
1
Write the set using set-builder notation:
The set of natural numbers greater than 11.
A){12, 13, 14, 15, . . .}
B)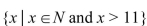
C){11, 12, 13, 14, . . .}
D)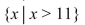
The set of natural numbers greater than 11.
A){12, 13, 14, 15, . . .}
B)
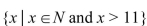
C){11, 12, 13, 14, . . .}
D)
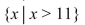
B
2
Write the set using the descriptive method:
{25, 26, 27, . . . , 45}
{25, 26, 27, . . . , 45}
The set of natural numbers from 25 to 45, inclusive.
3
Show that the pair of sets is equivalent by using a one-to-one correspondence.
{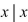 is an odd natural number between 8 and 20} and
is an odd natural number between 8 and 20} and
{x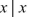 is an even natural number between 13 and 25}
is an even natural number between 13 and 25}
A)
B)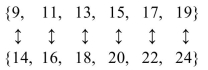
C)
D)
{
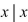 is an odd natural number between 8 and 20} and
is an odd natural number between 8 and 20} and{x
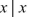 is an even natural number between 13 and 25}
is an even natural number between 13 and 25}A)

B)

C)

D)

B
4
List the elements in the set: {7, 10, 13, . . . , 31}.

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
5
Write the set using roster notation:
The set of even natural numbers less than 10.
A)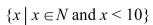
B)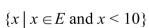
C){2, 4, 6, 8, 10}
D){2, 4, 6, 8}
The set of even natural numbers less than 10.
A)
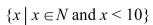
B)
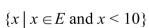
C){2, 4, 6, 8, 10}
D){2, 4, 6, 8}

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
6
Let U = {5, 10, 15, 20, 25, 30, 35, 40}
A = {5, 10, 15, 20}
B = {25, 30, 35, 40}
C = {10, 20, 30, 40}.
Find C'.
A)C' = {5, 15, 25, 35}
B)C' = {5, 10, 15, 20, 25, 30, 35, 40}
C)C' = {10, 20, 30, 40}
D)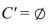
A = {5, 10, 15, 20}
B = {25, 30, 35, 40}
C = {10, 20, 30, 40}.
Find C'.
A)C' = {5, 15, 25, 35}
B)C' = {5, 10, 15, 20, 25, 30, 35, 40}
C)C' = {10, 20, 30, 40}
D)
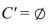

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
7
Which statement is true?
A)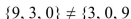 }
}
B)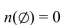
C)
D)All equivalent sets are equal.
A)
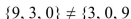 }
}B)
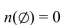
C)

D)All equivalent sets are equal.

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
8
Let U = {8, 16, 24, 32, 40, 48, 56, 64} and A = {24, 32, 40, 56}. 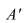
A)
B)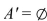
C)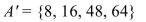
D)
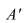
A)

B)
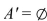
C)
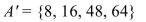
D)


Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
9
State whether the following set is empty. 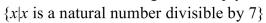
A)Yes
B)No
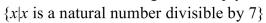
A)Yes
B)No

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
10
Which set is infinite?
A)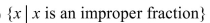
B)
C)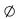
D){3, 5, 7, . . ., 99}
A)
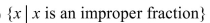
B)

C)
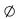
D){3, 5, 7, . . ., 99}

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
11
Write the set using roster notation: 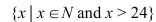
A){25, 26, 27, 28, . . .}
B)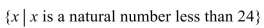
C)
D){24, 25, 26, 27, . . .}
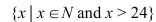
A){25, 26, 27, 28, . . .}
B)
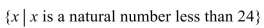
C)

D){24, 25, 26, 27, . . .}

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
12
Which set is finite?
A)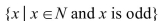
B){2, 4, 6, 8, 10, 12, . . .}
C)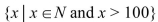
D)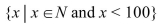
A)
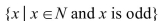
B){2, 4, 6, 8, 10, 12, . . .}
C)
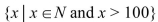
D)
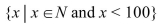

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
13
Write the set using the descriptive method:
{9, 18, 27, 36, 45}
A)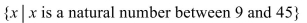
B)The set natural numbers between 9 and 45.
C)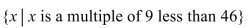
D)The set of the first five positive multiples of 9.
{9, 18, 27, 36, 45}
A)
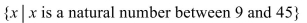
B)The set natural numbers between 9 and 45.
C)
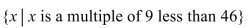
D)The set of the first five positive multiples of 9.

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
14
Classify each pair of sets as equal, equivalent, or neither.
{1, 2, 3, . . ., 10} and {10, 9, 8, . . ., 1}
A)equal and equivalent
B)neither
C)equivalent
{1, 2, 3, . . ., 10} and {10, 9, 8, . . ., 1}
A)equal and equivalent
B)neither
C)equivalent

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
15
State whether the collection is well-defined or not well-defined.
{5, . . . }
A)Well-defined
B)Not well-defined
{5, . . . }
A)Well-defined
B)Not well-defined

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
16
Find all subsets of the set. {2, 4, 9}.

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
17
The graph below displays the median housing prices for all houses sold in Anywhere, US between 2003 and
2008. List the set of years in which the median price was above $150,000.
List the set of years in which the median price was above $150,000.
A)(2005, 2006, 2007, 2008}
B)(2003, 2004}
C)(2005, 2006, 2007}
D)(2003, 2004, 2008}
2008.
 List the set of years in which the median price was above $150,000.
List the set of years in which the median price was above $150,000.A)(2005, 2006, 2007, 2008}
B)(2003, 2004}
C)(2005, 2006, 2007}
D)(2003, 2004, 2008}

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
18
Write the set using set-builder notation:
{1, 3, 5, . . . , 27}
A)
B)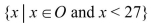
C)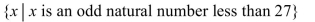
D)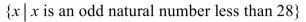
{1, 3, 5, . . . , 27}
A)

B)
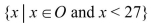
C)
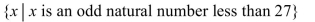
D)
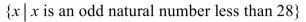

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
19
Classify each pair of sets as equal, equivalent, or neither.
{1, 3, 5} and {2, 4, 6, 8}
A)neither
B)equivalent
C)equal and equivalent
{1, 3, 5} and {2, 4, 6, 8}
A)neither
B)equivalent
C)equal and equivalent

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
20
Classify each pair of sets as equal, equivalent, or neither.
{a, b, c, d, e} and {5, 4, 3, 2, 1}
A)equivalent
B)neither
C)equal and equivalent
{a, b, c, d, e} and {5, 4, 3, 2, 1}
A)equivalent
B)neither
C)equal and equivalent

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
21
Let U = {5, 10, 15, 20, 25, 30, 35, 40} 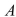 = {5, 10, 15, 20}
= {5, 10, 15, 20} 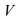 = {25, 30, 35, 40}
= {25, 30, 35, 40} 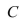 = {10, 20, 30, 40}.
= {10, 20, 30, 40}.
Find .
.
A)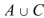 = {5, 10, 15, 20, 30, 40}
= {5, 10, 15, 20, 30, 40}
B)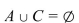
C) = {10, 20}
= {10, 20}
D)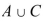 = {5, 15, 30, 40}
= {5, 15, 30, 40}
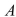 = {5, 10, 15, 20}
= {5, 10, 15, 20}  = {25, 30, 35, 40}
= {25, 30, 35, 40} 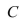 = {10, 20, 30, 40}.
= {10, 20, 30, 40}.Find
 .
.A)
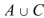 = {5, 10, 15, 20, 30, 40}
= {5, 10, 15, 20, 30, 40}B)
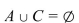
C)
 = {10, 20}
= {10, 20}D)
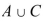 = {5, 15, 30, 40}
= {5, 15, 30, 40}
Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
22
Draw a Venn diagram and shade the sections representing 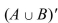 .
.
A)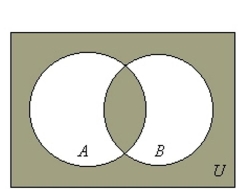
B)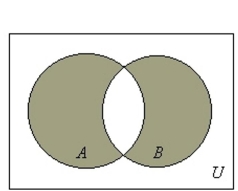
C)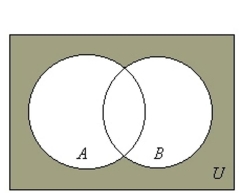
D)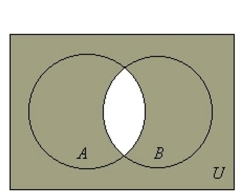
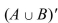 .
.A)

B)

C)

D)


Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
23
Let U = {4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14}
X = {4, 6, 8, 10, 12, 14}
Y = {4, 5, 6, 7, 8, 9}
Z = {5, 7, 8, 9, 12, 13, 14}.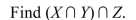
A)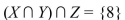
B)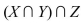 = {4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14}
= {4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14}
C)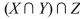 = {4, 5, 6, 7, 8, 9, 10, 12, 13, 14}
= {4, 5, 6, 7, 8, 9, 10, 12, 13, 14}
D)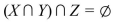
X = {4, 6, 8, 10, 12, 14}
Y = {4, 5, 6, 7, 8, 9}
Z = {5, 7, 8, 9, 12, 13, 14}.
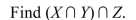
A)
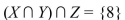
B)
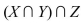 = {4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14}
= {4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14}C)
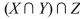 = {4, 5, 6, 7, 8, 9, 10, 12, 13, 14}
= {4, 5, 6, 7, 8, 9, 10, 12, 13, 14}D)
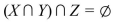

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
24
Find the number of proper subsets the set has. {m, n, p}
A)8
B)3
C)7
D)6
A)8
B)3
C)7
D)6

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
25
True or False? {4} 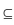 {2, 4, 6, 8, 10}
{2, 4, 6, 8, 10}
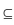 {2, 4, 6, 8, 10}
{2, 4, 6, 8, 10}
Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
26
Find all proper subsets of the set. {c, f, x}
A)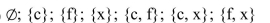
B)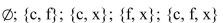
C)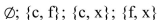
D)
A)
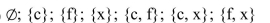
B)
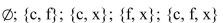
C)
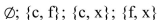
D)


Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
27
Find the number of subsets the set has. {1, 2, 3, 4, 5}
A)16
B)31
C)5
D)32
A)16
B)31
C)5
D)32

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
28
Let  = {n, p, q, r, s, t, u, v}
= {n, p, q, r, s, t, u, v} 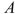 = {n, p, q, r}
= {n, p, q, r} 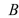 = {n, q, s, u}.
= {n, q, s, u}.
Find B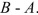 .
.
A)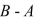 = {s, u}
= {s, u}
B)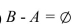
C)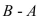 = {n, p, q, r, s, u}
= {n, p, q, r, s, u}
D)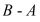 = {s, t, u, v}
= {s, t, u, v}
 = {n, p, q, r, s, t, u, v}
= {n, p, q, r, s, t, u, v} 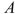 = {n, p, q, r}
= {n, p, q, r} 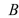 = {n, q, s, u}.
= {n, q, s, u}.Find B
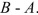 .
.A)
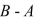 = {s, u}
= {s, u}B)
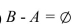
C)
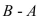 = {n, p, q, r, s, u}
= {n, p, q, r, s, u}D)
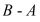 = {s, t, u, v}
= {s, t, u, v}
Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
29
Since the student union is being remodeled, there is a limited choice of foods and drinks a student
Can buy for a snack between classes. Students can choose none, some, or all of these items:
Diet soft drink, cheeseburger, fries. How many different selections can be made?
A)9
B)3
C)7
D)8
Can buy for a snack between classes. Students can choose none, some, or all of these items:
Diet soft drink, cheeseburger, fries. How many different selections can be made?
A)9
B)3
C)7
D)8

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
30
Let 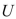 = {5, 10, 15, 20, 25, 30, 35, 40}
= {5, 10, 15, 20, 25, 30, 35, 40}  = {5, 10, 15, 20}
= {5, 10, 15, 20} 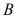 = {25, 30, 35, 40}
= {25, 30, 35, 40} 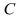 = {10, 20, 30, 40}.
= {10, 20, 30, 40}.
Find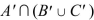 ).
).
A)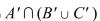 = 5, 10, 15, 20, 25, 30, 35, 40}
= 5, 10, 15, 20, 25, 30, 35, 40}
B)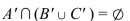
C) = {10, 20, 25, 30, 35, 40}
= {10, 20, 25, 30, 35, 40}
D)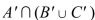 )= {25, 35}
)= {25, 35}
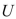 = {5, 10, 15, 20, 25, 30, 35, 40}
= {5, 10, 15, 20, 25, 30, 35, 40}  = {5, 10, 15, 20}
= {5, 10, 15, 20} 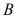 = {25, 30, 35, 40}
= {25, 30, 35, 40} 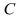 = {10, 20, 30, 40}.
= {10, 20, 30, 40}.Find
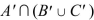 ).
).A)
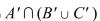 = 5, 10, 15, 20, 25, 30, 35, 40}
= 5, 10, 15, 20, 25, 30, 35, 40}B)
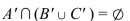
C)
 = {10, 20, 25, 30, 35, 40}
= {10, 20, 25, 30, 35, 40}D)
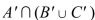 )= {25, 35}
)= {25, 35}
Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
31
Let 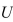 = {5, 10, 15, 20, 25, 30, 35, 40}
= {5, 10, 15, 20, 25, 30, 35, 40}  = {5, 10, 15, 20}
= {5, 10, 15, 20} 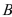 = {25, 30, 35, 40}
= {25, 30, 35, 40} 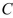 = {10, 20, 30, 40}.
= {10, 20, 30, 40}.
Find A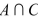 C.
C.
A)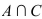 = {5, 15, 30, 40}
= {5, 15, 30, 40}
B)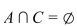
C)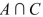 = {10, 20}
= {10, 20}
D)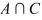 = {5, 10, 15, 20, 30, 40}
= {5, 10, 15, 20, 30, 40}
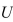 = {5, 10, 15, 20, 25, 30, 35, 40}
= {5, 10, 15, 20, 25, 30, 35, 40}  = {5, 10, 15, 20}
= {5, 10, 15, 20} 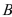 = {25, 30, 35, 40}
= {25, 30, 35, 40} 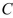 = {10, 20, 30, 40}.
= {10, 20, 30, 40}.Find A
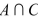 C.
C.A)
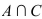 = {5, 15, 30, 40}
= {5, 15, 30, 40}B)
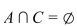
C)
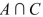 = {10, 20}
= {10, 20}D)
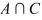 = {5, 10, 15, 20, 30, 40}
= {5, 10, 15, 20, 30, 40}
Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
32
Use the Venn diagram and find  .
. 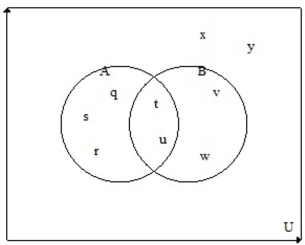
A)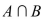 = {q, r, s, v, w}
= {q, r, s, v, w}
B)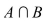 = {q, r, s, v, w, x, y}
= {q, r, s, v, w, x, y}
C)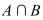 = {q, r, s, t, u, v, w}
= {q, r, s, t, u, v, w}
D)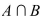 = {t, u}
= {t, u}
 .
. 
A)
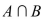 = {q, r, s, v, w}
= {q, r, s, v, w}B)
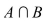 = {q, r, s, v, w, x, y}
= {q, r, s, v, w, x, y}C)
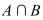 = {q, r, s, t, u, v, w}
= {q, r, s, t, u, v, w}D)
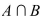 = {t, u}
= {t, u}
Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
33
Let U = {5, 10, 15, 20, 25, 30, 35, 40}  = {5, 10, 15, 20}
= {5, 10, 15, 20} 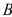 = {25, 30, 35, 40}
= {25, 30, 35, 40} 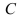 = {10, 20, 30, 40}.
= {10, 20, 30, 40}. 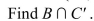
A)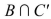 = {5, 15}
= {5, 15}
B)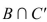 = {30, 40}
= {30, 40}
C)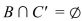
D)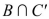 = {25, 35}
= {25, 35}
 = {5, 10, 15, 20}
= {5, 10, 15, 20} 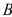 = {25, 30, 35, 40}
= {25, 30, 35, 40} 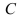 = {10, 20, 30, 40}.
= {10, 20, 30, 40}. 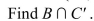
A)
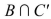 = {5, 15}
= {5, 15}B)
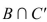 = {30, 40}
= {30, 40}C)
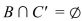
D)
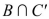 = {25, 35}
= {25, 35}
Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
34
Draw a Venn diagram and shade the sections representing  .
.
A)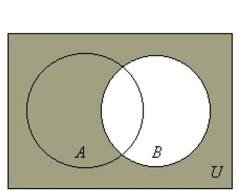
B)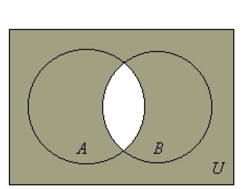
C)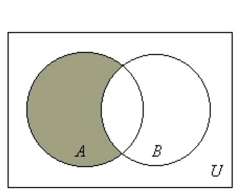
D)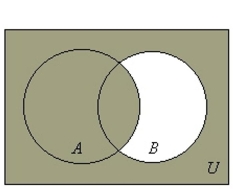
 .
.A)

B)

C)

D)


Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
35
Let 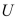 = {5, 10, 15, 20, 25, 30, 35, 40}
= {5, 10, 15, 20, 25, 30, 35, 40} 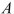 = {5, 10, 15, 20}
= {5, 10, 15, 20} 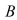 = {25, 30, 35, 40}
= {25, 30, 35, 40} 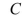 = {10, 20, 30, 40}.
= {10, 20, 30, 40}. 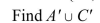
A)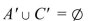
B)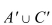 = {25, 35}
= {25, 35}
C) = {5, 10, 15, 20, 25, 30, 35, 40}
= {5, 10, 15, 20, 25, 30, 35, 40}
D)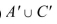 = {5, 15, 25, 30, 35, 40}
= {5, 15, 25, 30, 35, 40}
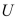 = {5, 10, 15, 20, 25, 30, 35, 40}
= {5, 10, 15, 20, 25, 30, 35, 40} 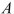 = {5, 10, 15, 20}
= {5, 10, 15, 20} 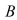 = {25, 30, 35, 40}
= {25, 30, 35, 40} 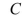 = {10, 20, 30, 40}.
= {10, 20, 30, 40}. 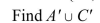
A)
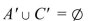
B)
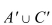 = {25, 35}
= {25, 35}C)
 = {5, 10, 15, 20, 25, 30, 35, 40}
= {5, 10, 15, 20, 25, 30, 35, 40}D)
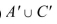 = {5, 15, 25, 30, 35, 40}
= {5, 15, 25, 30, 35, 40}
Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
36
Let U = {5, 10, 15, 20, 25, 30, 35, 40}
A = {5, 10, 15, 20}
B = {25, 30, 35, 40}
C = {10, 20, 30, 40}.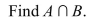
A)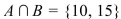
B)
C)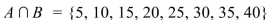
D)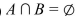
A = {5, 10, 15, 20}
B = {25, 30, 35, 40}
C = {10, 20, 30, 40}.
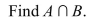
A)
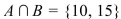
B)

C)
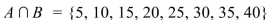
D)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
37
True or False? {4}  {2, 4, 6, 8, 10, . . . }
{2, 4, 6, 8, 10, . . . }
 {2, 4, 6, 8, 10, . . . }
{2, 4, 6, 8, 10, . . . }
Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
38
Draw a Venn diagram and shade the sections representing 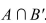 .
.
A)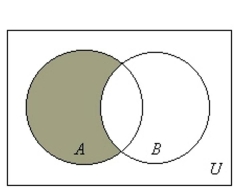
B)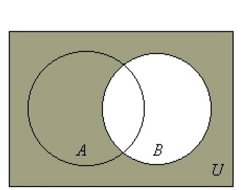
C)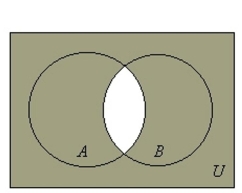
D)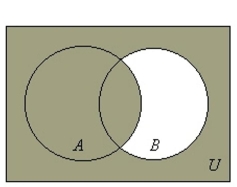
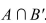 .
.A)

B)

C)

D)


Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
39
Which statement is false?
A)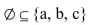
B)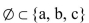
C)
D)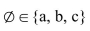
A)
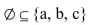
B)
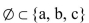
C)

D)
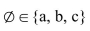

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
40
Let 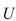 = {1, 2, 3, . . . }
= {1, 2, 3, . . . } 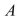 = {4, 8, 12, 16, . . . }
= {4, 8, 12, 16, . . . } 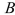 = {12, 24, 36, 48, . . . }.
= {12, 24, 36, 48, . . . }. 
A)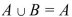
B)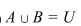
C)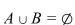
D)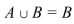
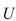 = {1, 2, 3, . . . }
= {1, 2, 3, . . . } 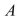 = {4, 8, 12, 16, . . . }
= {4, 8, 12, 16, . . . } 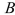 = {12, 24, 36, 48, . . . }.
= {12, 24, 36, 48, . . . }. 
A)
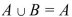
B)
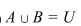
C)
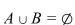
D)
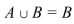

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
41
If 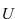 = {Bob, Ann, Mae, Eve, Dan, Kim, Tal, Vic},
= {Bob, Ann, Mae, Eve, Dan, Kim, Tal, Vic}, 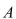 = {Ann, Eve, Dan, Kim}, and
= {Ann, Eve, Dan, Kim}, and 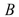 = {Bob, Ann, Mae, Eve} find
= {Bob, Ann, Mae, Eve} find 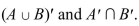
A)Both are {Ann, Eve}
B)
C)Both are {Tal, Vic}
D)Both are {Bob, Ann, Mae, Eve, Dan, Kim}
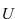 = {Bob, Ann, Mae, Eve, Dan, Kim, Tal, Vic},
= {Bob, Ann, Mae, Eve, Dan, Kim, Tal, Vic}, 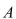 = {Ann, Eve, Dan, Kim}, and
= {Ann, Eve, Dan, Kim}, and 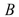 = {Bob, Ann, Mae, Eve} find
= {Bob, Ann, Mae, Eve} find 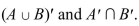
A)Both are {Ann, Eve}
B)

C)Both are {Tal, Vic}
D)Both are {Bob, Ann, Mae, Eve, Dan, Kim}

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
42
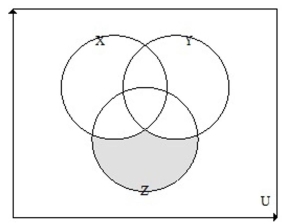
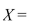 {students running cross country},
{students running cross country}, 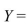 {students swimming}, and
{students swimming}, and  {students running track}.
{students running track}.Draw a Venn diagram of
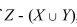 , and write a sentence describing what the set represents.
, and write a sentence describing what the set represents.A)
B)
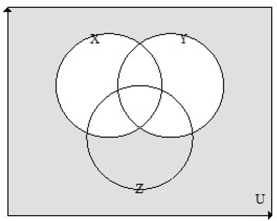
C)
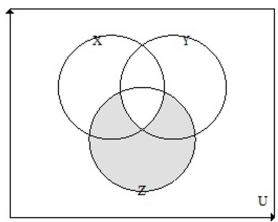
D)
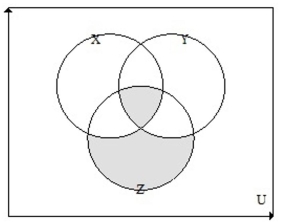

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
43
If  = {Bob, Ann, Mae, Eve, Dan, Pat, Tal, Liz},
= {Bob, Ann, Mae, Eve, Dan, Pat, Tal, Liz}, 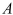 = {Ann, Eve, Dan, Pat}, and
= {Ann, Eve, Dan, Pat}, and
B = {Bob, Ann, Mae, Eve} find
A)Both are {Bob, Mae, Eve, Dan, Pat}
B)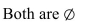
C)Both are {Ann, Eve}
D)Both are {Tal, Liz}
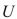 = {Bob, Ann, Mae, Eve, Dan, Pat, Tal, Liz},
= {Bob, Ann, Mae, Eve, Dan, Pat, Tal, Liz}, 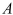 = {Ann, Eve, Dan, Pat}, and
= {Ann, Eve, Dan, Pat}, andB = {Bob, Ann, Mae, Eve} find

A)Both are {Bob, Mae, Eve, Dan, Pat}
B)
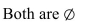
C)Both are {Ann, Eve}
D)Both are {Tal, Liz}

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
44
Determine whether the two sets are equal. 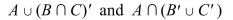
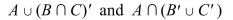

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
45
Determine whether the two sets are equal. 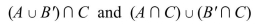
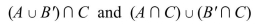

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
46
Let U = { 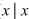 is a natural number
is a natural number 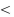 15}
15}  = {
= {  is an odd natural number}
is an odd natural number} 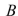 = {
= { 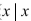 is a prime number}.
is a prime number}.
Find n(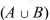 . Note: prime numbers are 2, 3, 5, 7 ,11, 13, 17, 19.
. Note: prime numbers are 2, 3, 5, 7 ,11, 13, 17, 19.
A)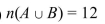
B)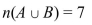
C)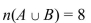
D)
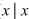 is a natural number
is a natural number 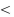 15}
15} 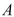 = {
= {  is an odd natural number}
is an odd natural number} 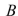 = {
= { 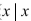 is a prime number}.
is a prime number}.Find n(
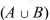 . Note: prime numbers are 2, 3, 5, 7 ,11, 13, 17, 19.
. Note: prime numbers are 2, 3, 5, 7 ,11, 13, 17, 19.A)
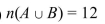
B)
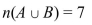
C)
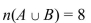
D)


Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
47
Draw a Venn diagram and shade the sections representing 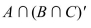
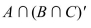

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
48
In a survey of 29 instructors, it was found that 22 liked whiteboards, 11 liked blackboards, and 7
Liked both. How many instructors did not like whiteboards?
A)14
B)7
C)3
D)4
Liked both. How many instructors did not like whiteboards?
A)14
B)7
C)3
D)4

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
49
The table shows the students from Genius High School with the four highest GPAs from 2005 to
2007. Write the region(s)of the Venn diagram that would include Harry. (Note set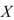 represents
represents
2005 top-ranked students, set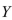 represents 2006 top-ranked students, and set
represents 2006 top-ranked students, and set  represents 2007
represents 2007
Top-ranked students.)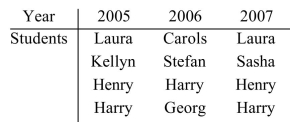
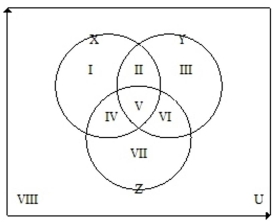
A)Region II
B)Region VI
C)Region IV
D)Region V
2007. Write the region(s)of the Venn diagram that would include Harry. (Note set
 represents
represents2005 top-ranked students, set
 represents 2006 top-ranked students, and set
represents 2006 top-ranked students, and set  represents 2007
represents 2007Top-ranked students.)


A)Region II
B)Region VI
C)Region IV
D)Region V

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
50
One weekend, there were 61 pizzas ordered for the sophomore dorm. That weekend 12 customers
Ordered their pizza with just pepperoni, 18 customers ordered their pizza with just sausage, 13
Ordered theirs with just onions, 6 ordered theirs with pepperoni and sausage, 5 ordered theirs with
Sausage and onions, 4 ordered theirs with pepperoni and onions, and 2 ordered theirs with all three
Items. The remaining pizzas were cheese pizzas with no toppings. How many customers ordered at
Most two toppings on their pizza?
A)58
B)15
C)16
D)59
Ordered their pizza with just pepperoni, 18 customers ordered their pizza with just sausage, 13
Ordered theirs with just onions, 6 ordered theirs with pepperoni and sausage, 5 ordered theirs with
Sausage and onions, 4 ordered theirs with pepperoni and onions, and 2 ordered theirs with all three
Items. The remaining pizzas were cheese pizzas with no toppings. How many customers ordered at
Most two toppings on their pizza?
A)58
B)15
C)16
D)59

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
51
Upon examining the contents of 38 backpacks, it was found that 23 contained a black pen, 27
Contained a blue pen, and 21 contained a pencil, 15 contained both a black pen and a blue pen, 12
Contained both a black pen and a pencil, 18 contained both a blue pen and a pencil, and 10 contained
All three items. How many backpacks contained none of the three writing instruments?
A)2
B)15
C)11
D)3
Contained a blue pen, and 21 contained a pencil, 15 contained both a black pen and a blue pen, 12
Contained both a black pen and a pencil, 18 contained both a blue pen and a pencil, and 10 contained
All three items. How many backpacks contained none of the three writing instruments?
A)2
B)15
C)11
D)3

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
52
Draw a Venn diagram and shade the sections representing 
A)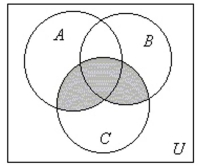
B)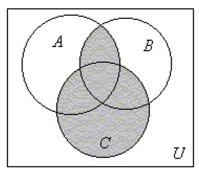
C)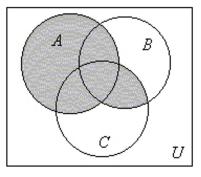
D)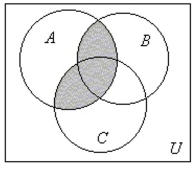

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
53
Upon examining the contents of 38 backpacks, it was found that 23 contained a black pen, 27
Contained a blue pen, and 21 contained a pencil, 15 contained both a black pen and a blue pen, 12
Contained both a black pen and a pencil, 18 contained both a blue pen and a pencil, and 10 contained
All three items. How many backpacks contained exactly one of the three writing instruments?
A)3
B)11
C)2
D)15
Contained a blue pen, and 21 contained a pencil, 15 contained both a black pen and a blue pen, 12
Contained both a black pen and a pencil, 18 contained both a blue pen and a pencil, and 10 contained
All three items. How many backpacks contained exactly one of the three writing instruments?
A)3
B)11
C)2
D)15

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
54
Use the Venn diagram and find 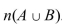 .
. 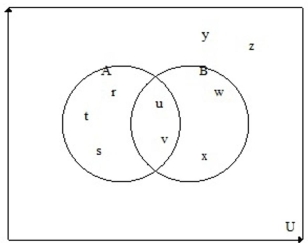
A)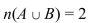
B)
C)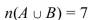
D)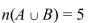
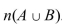 .
. 
A)
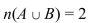
B)

C)
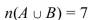
D)
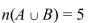

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
55
In a group of 53 children, 22 had a dog, 18 had a cat, and 6 had both a dog and a cat. How many
Children had neither a dog nor a cat as a pet?
A)31
B)37
C)12
D)19
Children had neither a dog nor a cat as a pet?
A)31
B)37
C)12
D)19

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
56
Draw a Venn diagram and shade the sections representing 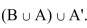
A)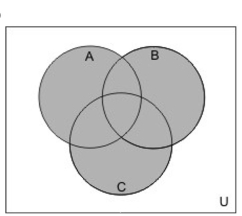
B)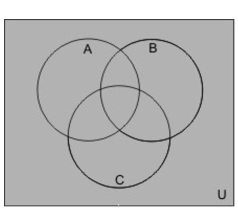
C)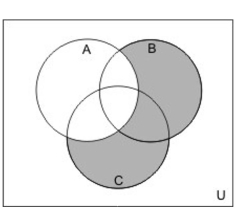
D)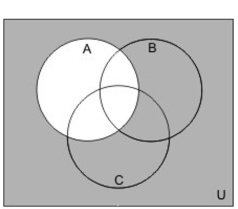
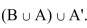
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
57
Determine whether the two sets are equal. 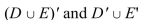
A)not equal
B)equal
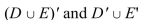
A)not equal
B)equal

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
58
One weekend, there were 98 pizzas ordered for the sophomore dorm. That weekend 19 customers
Ordered their pizza with just pepperoni, 16 customers ordered their pizza with just sausage, 20
Ordered theirs with just onions, 15 ordered theirs with pepperoni and sausage, 9 ordered theirs with
Sausage and onions, 17 ordered theirs with pepperoni and onions, and 2 ordered theirs with all three
Items. How many customers ordered their pizza with pepperoni or sausage (or both)?
A)72
B)35
C)50
D)13
Ordered their pizza with just pepperoni, 16 customers ordered their pizza with just sausage, 20
Ordered theirs with just onions, 15 ordered theirs with pepperoni and sausage, 9 ordered theirs with
Sausage and onions, 17 ordered theirs with pepperoni and onions, and 2 ordered theirs with all three
Items. How many customers ordered their pizza with pepperoni or sausage (or both)?
A)72
B)35
C)50
D)13

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
59
A = {people who drive a compact car} and 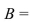 {people who drive a diesel vehicle}. Draw a Venn
{people who drive a diesel vehicle}. Draw a Venn
Diagram of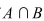 and write a sentence describing what the set represents.
and write a sentence describing what the set represents.
A)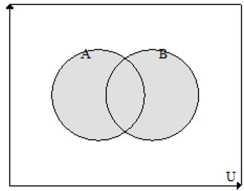
B)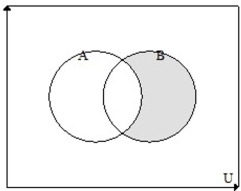
C)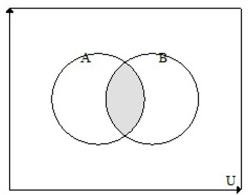
D)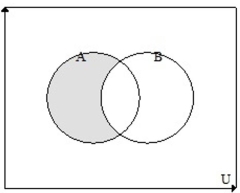
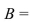 {people who drive a diesel vehicle}. Draw a Venn
{people who drive a diesel vehicle}. Draw a VennDiagram of
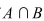 and write a sentence describing what the set represents.
and write a sentence describing what the set represents.A)

B)

C)

D)


Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
60
In a survey of 18 college students, it was found that 11 were taking an English class, 6 were taking a
Math class, and 4 were taking both English and math. How many students were taking a math class
Only?
A)11
B)2
C)7
D)5
Math class, and 4 were taking both English and math. How many students were taking a math class
Only?
A)11
B)2
C)7
D)5

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
61
The general term of the set 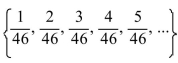 is .
is .
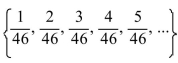 is .
is .
Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
62
Show that the set is an infinite set. 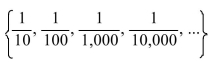
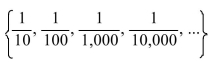

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
63
Find the general term of the set. 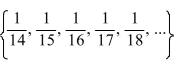
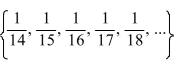

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
64
Find the general term of the set. {9, 12, 15, 18, 21, . . .}
A)
B)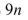
C)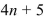
D)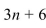
A)

B)
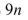
C)
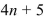
D)
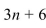

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
65
Which set is finite?
A){2, 4, 6, 8, 10, 12, . . .}
B)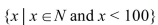
C)
D)
A){2, 4, 6, 8, 10, 12, . . .}
B)
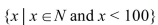
C)

D)


Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
66
Show that the set is an infinite set. {0, 4, 8, 12, 16, . . .}

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
67
Show that the given set is countable. {3, 6, 9, 12, 15, ...}

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
68
Show that the set is an infinite set. 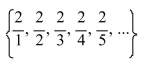
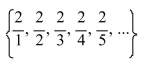

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
69
Show that the set of positive rational numbers with denominators 3 or 5 is a countable set. (Rational
numbers are fractions with integers in the numerator and denominator.)
numbers are fractions with integers in the numerator and denominator.)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
70
Find the general term of the set. {-10, -20, -30, -40, -50, . . .}
A)-11n + 1
B)-9n - 1
C)-10n
D)n - 11
A)-11n + 1
B)-9n - 1
C)-10n
D)n - 11

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
71
Which set is infinite?
A)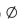
B)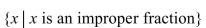
C){3, 5, 7, . . ., 99}
D)
A)
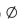
B)
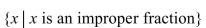
C){3, 5, 7, . . ., 99}
D)


Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
72
Show that the set is an infinite set. {6, 12, 18, 24, 30, . . .}

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
73
Upon examining the contents of 38 backpacks, it was found that 23 contained a black pen, 27
Contained a blue pen, and 21 contained a pencil, 15 contained both a black pen and a blue pen, 12
Contained both a black pen and a pencil, 18 contained both a blue pen and a pencil, and 10 contained
All three items. How many backpacks contained exactly two of the three writing instruments?
A)11
B)2
C)15
D)3
Contained a blue pen, and 21 contained a pencil, 15 contained both a black pen and a blue pen, 12
Contained both a black pen and a pencil, 18 contained both a blue pen and a pencil, and 10 contained
All three items. How many backpacks contained exactly two of the three writing instruments?
A)11
B)2
C)15
D)3

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck


