Deck 6: Systems of Equations
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/73
Play
Full screen (f)
Deck 6: Systems of Equations
1
Use a table to solve the system of equations. If you use a calculator table, record the TbIStart and entered.
A) (4, -2)
B) (3, -2)
C) (5, -2)
D) (3, -3)
E) (3, -1)
A) (4, -2)
B) (3, -2)
C) (5, -2)
D) (3, -3)
E) (3, -1)
(3, -2)
2
Do the graphs of the equations form coincident lines? (It is not necessary to graph them.)
A) yes
B) no
C) insufficient information
A) yes
B) no
C) insufficient information
no
3
Use a table to solve the system of equations. If you use a calculator table, record the TbIStart and entered.
A) (5, -3)
B) (3, 5)
C) (-5, -4)
D) (-4, 3)
E) any (x, y) on line
A) (5, -3)
B) (3, 5)
C) (-5, -4)
D) (-4, 3)
E) any (x, y) on line
any (x, y) on line
4
Do the graphs of the equations form parallel lines? (It is not necessary to graph them.)
A) yes
B) no
C) insufficient information
A) yes
B) no
C) insufficient information

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
5
Do the graphs of the equations form coincident lines? (It is not necessary to graph them.)
A) yes
B) no
C) insufficient information
A) yes
B) no
C) insufficient information

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
6
Do the graphs of the equations form coincident lines? (It is not necessary to graph them.)
A) yes
B) no
C) insufficient information
A) yes
B) no
C) insufficient information

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
7
Do the graphs of the equations form coincident lines? (It is not necessary to graph them.)
A) yes
B) no
C) insufficient information
A) yes
B) no
C) insufficient information

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
8
Write a system of equations to describe the problem situation. It is not necessary to solve the problem. Paolo earns $481 from working 54 total hours at two jobs. He earns $6.55 per hour (x) at the first job and $9.20 per hour (y) at the second job.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
9
Write a system of equations to describe the problem situation. It is not necessary to solve the problem. Martina has 58 nickels and dimes. She has $3.10 altogether. She has x nickels and y dimes.
A) y = 58 - x, 0.05x + 0.10y = 3.10
B) x + y = 58, 0.05y + 0.10x = 3.10
C)
D) x - y = 58, 0.05x + 0.10y = 3.10
E)
A) y = 58 - x, 0.05x + 0.10y = 3.10
B) x + y = 58, 0.05y + 0.10x = 3.10
C)
D) x - y = 58, 0.05x + 0.10y = 3.10
E)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
10
Write a system of equations that will solve the problem. It is not necessary to solve the problem. Ed works on two projects during one month. He works 28 hours longer on one project (y) than on the other (x). He works a total of 186 hours during the month.
A) x + y = 186, x = y + 28
B) x - y = 186, x = y + 28
C) x + y = 186, x = y - 28
D) y - x = 186, y = x - 28
E) x - y = 186, x = y - 28
A) x + y = 186, x = y + 28
B) x - y = 186, x = y + 28
C) x + y = 186, x = y - 28
D) y - x = 186, y = x - 28
E) x - y = 186, x = y - 28

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
11
Write a system of equations that will solve the problem. It is not necessary to solve the problem. Jacques cuts a 14-decimeter submarine sandwich into two pieces. One piece (y) is 2 decimeters longer than the other (x).
A) x + y = 14, x = y + 2
B) x + y = 14, x = y - 2
C) x - y = 14, x = y + 2
D) y - x = 14, y = x - 2
E) y - x = 14, x = y - 2
A) x + y = 14, x = y + 2
B) x + y = 14, x = y - 2
C) x - y = 14, x = y + 2
D) y - x = 14, y = x - 2
E) y - x = 14, x = y - 2

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
12
Use a table to solve the system of equations. If you use a calculator table, record the TbIStart and entered.
A) (4, -1)
B) (2, -2)
C) (3, -2)
D) (3, -3)
E) (4, -2)
A) (4, -1)
B) (2, -2)
C) (3, -2)
D) (3, -3)
E) (4, -2)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
13
Write a system of equations that will solve the problem. It is not necessary to solve the problem. Alexandra works for two clients during one month. She works a total of 180 hours during the month. She works 50 hours more for one client (y) than for the other (x).
A) x + y = 180, x = y + 50
B) x - y = 180, x = y + 50
C) y - x = 180, y = x - 50
D)
E) x + y = 180, x - y = 50
A) x + y = 180, x = y + 50
B) x - y = 180, x = y + 50
C) y - x = 180, y = x - 50
D)
E) x + y = 180, x - y = 50

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
14
Write a system of equations to describe the problem situation. It is not necessary to solve the problem. Nancy has $3.50. She has only dimes (x) and quarters (y). She has 30 coins altogether.
A) x = 30 - y, 0.10x + 0.25y = 3.50
B)
C) y - x = 30, 0.10x + 0.25y = 3.50
D) x + y = 30, 0.05x + 0.25y = 3.50
E)
A) x = 30 - y, 0.10x + 0.25y = 3.50
B)
C) y - x = 30, 0.10x + 0.25y = 3.50
D) x + y = 30, 0.05x + 0.25y = 3.50
E)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
15
Use a table to solve the system of equations. If you use a calculator table, record the TbIStart and entered.
A) (-1, 2)
B) (0, 1)
C) (-1, 0)
D) (-1, 1)
E) (0, 2)
A) (-1, 2)
B) (0, 1)
C) (-1, 0)
D) (-1, 1)
E) (0, 2)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
16
Write a system of equations that will solve the problem. It is not necessary to solve the problem. Renee cuts a 12-meter hose into two lengths. One piece (x) is 2 meters longer than the other (y). What is the length of each piece?
A)
B)
C) x - y = 12, x = y + 2
D) y - x = 12, y = x - 2
E) x + y = 12, x = y - 2
A)
B)
C) x - y = 12, x = y + 2
D) y - x = 12, y = x - 2
E) x + y = 12, x = y - 2

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
17
Do the graphs of the equations form parallel lines? (It is not necessary to graph them.)
A) yes
B) no
C) insufficient information
A) yes
B) no
C) insufficient information

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
18
Do the graphs of the equations form parallel lines? (It is not necessary to graph them.)
A) yes
B) no
C) insufficient information
A) yes
B) no
C) insufficient information

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
19
Write a system of equations to describe the problem situation. It is not necessary to solve the problem. Larry has 26 nickels and quarters. He has $2.20 altogether. He has x nickels and y quarters.
A) x - y = 26, 0.10y + 0.25x = 2.20
B) x + y = 26, 0.10x + 0.25y = 2.20
C) x - y = 26, 0.05x + 0.25y = 2.20
D) x + y = 26, 0.05y + 0.25x = 2.20
E) x = 26 - y, 0.05x + 0.25y = 2.20
A) x - y = 26, 0.10y + 0.25x = 2.20
B) x + y = 26, 0.10x + 0.25y = 2.20
C) x - y = 26, 0.05x + 0.25y = 2.20
D) x + y = 26, 0.05y + 0.25x = 2.20
E) x = 26 - y, 0.05x + 0.25y = 2.20

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
20
Use a table to solve the system of equations. If you use a calculator table, record the TbIStart and entered.
A) (2, 5)
B) (2, 10)
C) (5, 10)
D) (5, 5)
E) no solution
A) (2, 5)
B) (2, 10)
C) (5, 10)
D) (5, 5)
E) no solution

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
21
Decide which variable has a coefficient of 1 or -1, and solve for that variable in terms of the other variable.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
22
Decide which variable has a coefficient of 1 or -1, and solve for that variable in terms of the other variable.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
23
Write a system of equations to describe the problem situation. Use guess and check to solve the problem. Tickets for five students and two adults to a Saturday morning magic show cost $25. Tickets for six students and one adult cost $26.50. Find the price of an adult ticket.
A) Adult ticket is $5.00.
B) Adult ticket is $2.50.
C) Adult ticket is $3.00.
D) Adult ticket is $4.00.
E) Adult ticket is $3.50.
A) Adult ticket is $5.00.
B) Adult ticket is $2.50.
C) Adult ticket is $3.00.
D) Adult ticket is $4.00.
E) Adult ticket is $3.50.

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
24
Write a system of equations to describe the problem situation. Use guess and check to solve the problem. An airplane flies westbound from Denver to San Francisco (950 miles) with the wind in 3.8 hours. On a return flight against the same wind, the travel time is 5 hours. Find the speed of the wind, w.
A) w = 45 mph
B) w = 40 mph
C) w = 35 mph
D) w = 30 mph
E) w = 25 mph
A) w = 45 mph
B) w = 40 mph
C) w = 35 mph
D) w = 30 mph
E) w = 25 mph

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
25
Find the rate of travel for a fishing boat with a speed of 10 miles per hour (mph) moving downstream with a current of 5 mph.
A) 15 mph
B) 10 mph
C) 7.5 mph
D) 5 mph
E) -5 mph
A) 15 mph
B) 10 mph
C) 7.5 mph
D) 5 mph
E) -5 mph

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
26
Write a system of equations to describe the problem situation. Use guess and check to solve the problem. A boat travels 20 miles downstream in an hour. While its passengers are fishing, the tide comes in. The tidewater reaches 20 miles upstream. The trip home on still water take 1 hours. Find the speed of the current, c.
A) c = 1.5 mph
B) c = 2.0 mph
C) c = 2.5 mph
D) c = 3.0 mph
E) c = 4.0 mph
A) c = 1.5 mph
B) c = 2.0 mph
C) c = 2.5 mph
D) c = 3.0 mph
E) c = 4.0 mph

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
27
Write a system of equations to describe the problem situation. Use guess and check to solve the problem. Two of the top-price tickets and five of the lowest-price tickets for a Tim McGraw concert cost $660. Three of the top-price and three of the lowest-price tickets cost $900. Find the cost of the top-price ticket.
A) Top-price ticket costs $270.
B) Top-price ticket costs $105.
C) Top-price ticket costs $230.
D) Top-price ticket costs $280.
E) Top-price ticket costs $250.
A) Top-price ticket costs $270.
B) Top-price ticket costs $105.
C) Top-price ticket costs $230.
D) Top-price ticket costs $280.
E) Top-price ticket costs $250.

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
28
Write a system of equations to describe the problem situation. It is not necessary to solve the problem. Restaurants buy 10-pound and 25-pound bags of carrots. They want five times as many 10-pound bags (x) as 25-pound bags (y). If the company estimates that restaurants will buy 450 pounds of carrots, how many bags of each size should be packaged?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
29
Decide which variable has a coefficient of 1 or -1, and solve for that variable in terms of the other variable.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
30
Write a system of equations to describe the problem situation. Use guess and check to solve the problem. An airplane flies eastbound from Boise to Chicago, covering the 1400 miles with the wind in 4 hours. A return flight against the same wind takes 5 hours. Find the speed of the wind, w.
A) w = 30 mph
B) w = 35 mph
C) w = 40 mph
D) w = 25 mph
E) w = 20 mph
A) w = 30 mph
B) w = 35 mph
C) w = 40 mph
D) w = 25 mph
E) w = 20 mph

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
31
Write a system of equations to describe the problem situation. It is not necessary to solve the problem. The I.R.Rabbit Company sells carrots. It sells six times as many pounds to grocery stores as to restaurants. It has 5,300 pounds of carrots. The company sells x pounds of carrots to grocery stores and y pounds of carrots to restaurants.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
32
Write a system of equations to describe the problem situation. Use guess and check to solve the problem. Two of the top-price tickets and eight of the lowest-price tickets for a Norah Jones concert cost $2,391. Three of the top-price and four of the lowest-price tickets cost $2,946.50. Find the cost of the top-price ticket.
A) Top-price ticket costs $731.50
B) Top-price ticket costs $675.50
C) Top-price ticket costs $835.50
D) Top-price ticket costs $915.50
E) Top-price ticket costs $875.50
A) Top-price ticket costs $731.50
B) Top-price ticket costs $675.50
C) Top-price ticket costs $835.50
D) Top-price ticket costs $915.50
E) Top-price ticket costs $875.50

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
33
Solve 10 = m(4) + b for m.
A)
B)
C)
D) 40 - 4b = m
E) 2.5 - b = m
A)
B)
C)
D) 40 - 4b = m
E) 2.5 - b = m

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
34
Decide which variable has a coefficient of 1 or -1, and solve for that variable in terms of the other variable.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
35
Write a system of equations to describe the problem situation. Use guess and check to solve the problem. On a weekend, two adult movie tickets and three student tickets cost $36.50. Three adult and two student tickets cost $38.50. Find the price of the adult ticket.
A) Adult ticket is $8.50.
B) Adult ticket is $6.50.
C) Adult ticket is $7.50.
D) Adult ticket is $9.50.
E) Adult ticket is $4.50.
A) Adult ticket is $8.50.
B) Adult ticket is $6.50.
C) Adult ticket is $7.50.
D) Adult ticket is $9.50.
E) Adult ticket is $4.50.

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
36
Write a system of equations to describe the problem situation. Use guess and check to solve the problem. A boat travels 12 miles upstream to a fishing spot. The trip takes 1.2 hours. The return trip, downstream, takes 0.8 hour. Find the speed of the current, c.
A) c = 2.0 mph
B) c = 2.5 mph
C) c = 2.8 mph
D) c = 3.0 mph
E) c = 3.5 mph
A) c = 2.0 mph
B) c = 2.5 mph
C) c = 2.8 mph
D) c = 3.0 mph
E) c = 3.5 mph

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
37
Solve D = rt for r.
A)
B) D - r = t
C) D - t = r
D) Dt = r
E)
A)
B) D - r = t
C) D - t = r
D) Dt = r
E)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
38
Solve for x.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
39
Solve by substitution:
A) (-5, -2)
B) (-4, 1)
C) (-6, -5)
D) Dependent
E) No solution
A) (-5, -2)
B) (-4, 1)
C) (-6, -5)
D) Dependent
E) No solution

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
40
Write a system of equations to describe the problem situation. Use guess and check to solve the problem. Three adult and five university student tickets for the alternative music hall cost $86.50. Five adult and eight student tickets cost $141. Find the price of an adult ticket.
A) Adult ticket is $8.50.
B) Adult ticket is $12.
C) Adult ticket is $13.
D) Adult ticket is $9.50.
E) Adult ticket is $15.
A) Adult ticket is $8.50.
B) Adult ticket is $12.
C) Adult ticket is $13.
D) Adult ticket is $9.50.
E) Adult ticket is $15.

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
41
Solve by substitution:
A) (13, 14)
B) (8, 9)
C) (3, 4)
D) Dependent
E) No solution
A) (13, 14)
B) (8, 9)
C) (3, 4)
D) Dependent
E) No solution

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
42
The sum of two numbers is 37. Their difference is 6. Identify two appropriate variables, and use the variables to write equations. Use either the elimination method or substitution method to solve the equations.
A) Twice the larger number is 41.
B) Twice the larger number is 43.
C) Twice the larger number is 42.
D) Twice the smaller number is 30.
E) Twice the smaller number is 29.
A) Twice the larger number is 41.
B) Twice the larger number is 43.
C) Twice the larger number is 42.
D) Twice the smaller number is 30.
E) Twice the smaller number is 29.

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
43
To cut costs, the new manager at Healthy Options wants to mix 4,000 pounds of cat food. The mixture is to have 10% protein. The first ingredient contains 8% protein. The second has 13% protein. How many pounds of the 8% protein ingredient is needed?
A) 1,200 pounds of 8%
B) 2,400 pounds of 8%
C) 1,360 pounds of 8%
D) 1,880 pounds of 8%
E) 1,500 pounds of 8%
A) 1,200 pounds of 8%
B) 2,400 pounds of 8%
C) 1,360 pounds of 8%
D) 1,880 pounds of 8%
E) 1,500 pounds of 8%

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
44
Solve by the elimination method:
A)
B)
C)
D)
E) No solution
A)
B)
C)
D)
E) No solution

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
45
Solve by substitution:
A) (3, -16)
B) (4, -20)
C) (2, -12)
D) Dependent,
E) No solution
A) (3, -16)
B) (4, -20)
C) (2, -12)
D) Dependent,
E) No solution

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
46
Define variables for the measures of angles. Write equations based on the given facts as well as the characteristics of complementary angles and supplementary angles. Solve the given resulting system of equations by elimination.
a) Two angles are complementary. The measure of one angle is larger than that of the other.
b) Two angles are supplementary. The measure of one angle is larger than that of the other.
A) a)
b)
B) a)
b)
C) a)
b)
D) a)
b)
E) a)
b)
a) Two angles are complementary. The measure of one angle is larger than that of the other.
b) Two angles are supplementary. The measure of one angle is larger than that of the other.
A) a)
b)
B) a)
b)
C) a)
b)
D) a)
b)
E) a)
b)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
47
Identify two appropriate variables, and use the variables to write equations. Use either the elimination method or the substitution method to solve the equation The sum of two numbers is . There difference is .
Find the numbers.
A)
B)
C)
D)
E)
Find the numbers.
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
48
Solve by the elimination method:
A) (-5, -4)
B) (3, 0)
C) (-1, -2)
D) Dependent
E) No solution
A) (-5, -4)
B) (3, 0)
C) (-1, -2)
D) Dependent
E) No solution

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
49
A parallelogram has angles A and B as supplementary angles. The measure of angle A is 74 degrees more than angle B. What is the measure of angle A?
A) A = 53 degrees
B) A = 67 degrees
C) A = 82 degrees
D) A = 127 degrees
E) A = 113 degrees
A) A = 53 degrees
B) A = 67 degrees
C) A = 82 degrees
D) A = 127 degrees
E) A = 113 degrees

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
50
Two DVDs and three game disks cost $137.79. Four DVDs and one game disk cost $75.73. What does one of each cost?
A) DVD = $9.09, game disk = $39.87
B) DVD = $11.88, game disk = $40.09
C) DVD = $9.15, game disk = $39.83
D) DVD = $8.94, game disk = $39.97
E) DVD = $9.00, game disk = $39.93
A) DVD = $9.09, game disk = $39.87
B) DVD = $11.88, game disk = $40.09
C) DVD = $9.15, game disk = $39.83
D) DVD = $8.94, game disk = $39.97
E) DVD = $9.00, game disk = $39.93

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
51
Solve by substitution:
A) (3, 0)
B) (4, -4)
C) (2, 4)
D) Dependent
E) No solution
A) (3, 0)
B) (4, -4)
C) (2, 4)
D) Dependent
E) No solution

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
52
The perimeter of the front of a 20-ounce Cheerios box is 44 inches. The height is 4 inches more than the width. What is the width of the box?
A) width = 13 inches
B) width = 10 inches
C) width = 14 inches
D) width = 11 inches
E) width = 9 inches
A) width = 13 inches
B) width = 10 inches
C) width = 14 inches
D) width = 11 inches
E) width = 9 inches

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
53
Solve by the elimination method:
A) (5, 1)
B) (-10, 16)
C) (0, 6)
D) (-5, 11)
E) No solution
A) (5, 1)
B) (-10, 16)
C) (0, 6)
D) (-5, 11)
E) No solution

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
54
Solve by the elimination method:
A) (5, 4)
B) (1, 0)
C) (9, 8)
D) Dependent
E) No solution
A) (5, 4)
B) (1, 0)
C) (9, 8)
D) Dependent
E) No solution

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
55
Yoko has $5.00 in quarters and nickels. She has 24 coins altogether. How many quarters does she have?
A) 19 quarters
B) 5 quarters
C) 20 quarters
D) 4 quarters
E) 21 quarters
A) 19 quarters
B) 5 quarters
C) 20 quarters
D) 4 quarters
E) 21 quarters

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
56
At the bookstore, 2 notebooks and 5 pens cost $14.69. At the same time, 6 notebooks and 3 pens cost $32.67. What is the cost of one of each?
A) notebooks = $4.87, pens = $0.99
B) notebooks = $4.92, pens = $0.97
C) notebooks = $4.97, pens = $0.95
D) notebooks = $4.79, pens = $1.31
E) notebooks = $4.77, pens = $1.03
A) notebooks = $4.87, pens = $0.99
B) notebooks = $4.92, pens = $0.97
C) notebooks = $4.97, pens = $0.95
D) notebooks = $4.79, pens = $1.31
E) notebooks = $4.77, pens = $1.03

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
57
Shrimp is 24.3% protein (by weight), and cooked brown rice is 2.9% protein (by weight). A mixture weighing 1113 grams is made from the shrimp and brown rice. The mixture needs to contain 63 grams of protein. How many grams of shrimp are to be used? Round to the nearest gram.
A) 969 grams of shrimp
B) 144 grams of shrimp
C) 139 grams of shrimp
D) 134 grams of shrimp
A) 969 grams of shrimp
B) 144 grams of shrimp
C) 139 grams of shrimp
D) 134 grams of shrimp

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
58
A right triangle has angles A and B as complementary angles. The measure of angle A is 8 more than the measure of angle B. What is the measure of angle A?
A) A = 94 degrees
B) A = 86 degrees
C) A = 41 degrees
D) A = 53 degrees
E) A = 49 degrees
A) A = 94 degrees
B) A = 86 degrees
C) A = 41 degrees
D) A = 53 degrees
E) A = 49 degrees

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
59
Solve by the elimination method:
A) (0, -2)
B) (-9, -29)
C) (-3, -11)
D) (-6, -20)
E) Dependent. The solutions satisfy the equation
A) (0, -2)
B) (-9, -29)
C) (-3, -11)
D) (-6, -20)
E) Dependent. The solutions satisfy the equation

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
60
Two angles are complementary. The measure of one angle is 60 degrees less than that of the other. Define variables for the measures of the angles. Write equations based on the given facts. Solve the resulting system by elimination to find the measures of the angles.
A) 30 degrees, 60 degrees
B) 60 degrees, 120 degrees
C) 15 degrees, 75 degrees
D) 20 degrees, 80 degrees
E) 150 degrees, 210 degrees
A) 30 degrees, 60 degrees
B) 60 degrees, 120 degrees
C) 15 degrees, 75 degrees
D) 20 degrees, 80 degrees
E) 150 degrees, 210 degrees

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
61
In the graph of y 0, x -1 what is the corner point?
A) (0, 0)
B) (0, -1)
C) (0, 1)
D) (-1, 0)
E) (1, 0)
A) (0, 0)
B) (0, -1)
C) (0, 1)
D) (-1, 0)
E) (1, 0)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
62
In the graph of x 3, y -2, what is the corner point?
A) (-2, -3)
B) (-3, 2)
C) (3, -2)
D) (-3, -2)
E) (-2, 3)
A) (-2, -3)
B) (-3, 2)
C) (3, -2)
D) (-3, -2)
E) (-2, 3)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
63
Nerine combines walking (at 6 km/hr) and running (at 18 km/hr) in her fitness program. Last week she exercised a total of 10 hours and traveled 108 kilometers. How many hours did she walk?
A) 5 hours
B) 6 hours
C) 4 hours
D) 8 hours
E) 2 hours
A) 5 hours
B) 6 hours
C) 4 hours
D) 8 hours
E) 2 hours

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
64
In the graph of y 1, x 0 what is the corner point?
A) (1, 1)
B) (1, 0)
C) (-1, 0)
D) (0, 1)
E) (0, -1)
A) (1, 1)
B) (1, 0)
C) (-1, 0)
D) (0, 1)
E) (0, -1)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
65
Which ordered pairs satisfy the system of inequalities given?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
66
Graph the system of Inequalities and show the solutions set. Label the corner point.
A)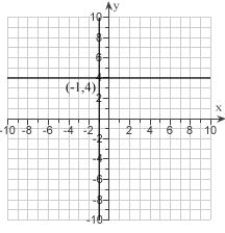
B)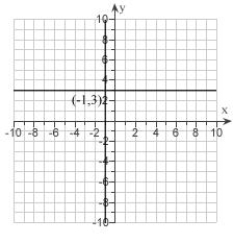
C)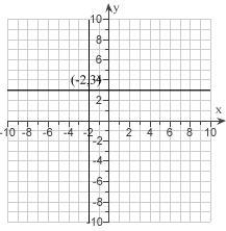
D)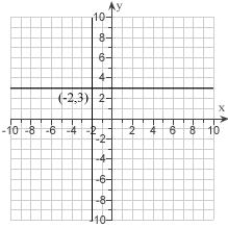
E)
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
67
Which ordered pairs satisfy the system of inequalities given?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
68
In the graph of x < 1, y -2, what is the corner point?
A) (1, 2)
B) (1, -2)
C) (-2, 1)
D) (-2, -1)
E) (0, -2)
A) (1, 2)
B) (1, -2)
C) (-2, 1)
D) (-2, -1)
E) (0, -2)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
69
Graph the system of Inequalities and show the solution set. Label the corner point.
A)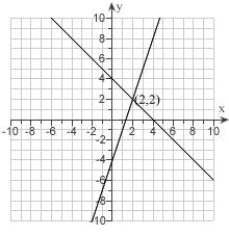
B)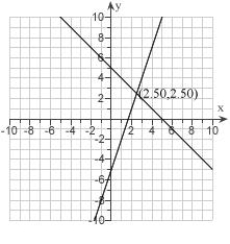
C)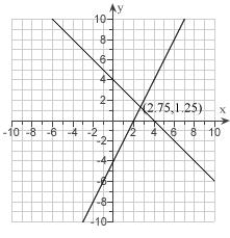
D)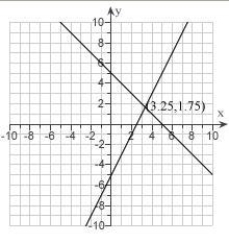
E)
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
70
Graph the system of Inequalities and show the solution set. Label the corner point.
A)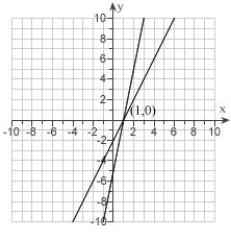
B)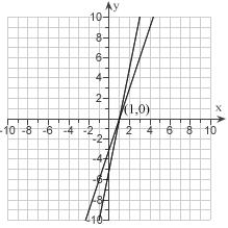
C)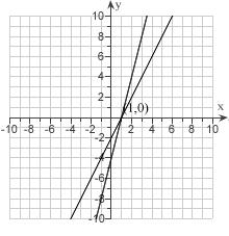
D)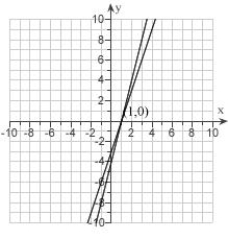
E)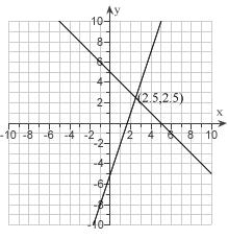
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
71
Which ordered pairs satisfy the system of inequalities given?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
72
Graph the system of inequalities and show the solution set.
A)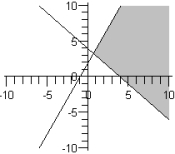
B)
C)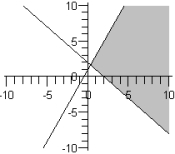
D)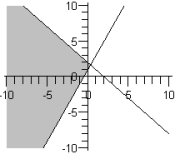
E)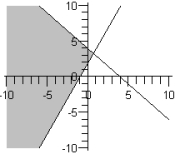
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck
73
Which ordered pairs satisfy the system of inequalities given?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 73 flashcards in this deck.
Unlock Deck
k this deck


