Deck 5: Probability

Question 1

The probability of an event is the _____ of times the event would occur if we repeated a random trial many times.

A) Number
B) Opposite
C) Proportion
D) Sum

Answer 1

C

Question 2

Which of the following statements correctly describes a pair of mutually exclusive events?

A) Pr[A and B] = 0
B) Pr[A or B] = 0
C) Pr[A and B] = 1
D) Pr[A or B] = 1

Answer 2

A

Question 3

Which of the following statements correctly describes a pair of mutually exclusive events?

A) Pr[A and B] = Pr[A] + Pr[B]
B) Pr[A or B] = Pr[A] + Pr[B]
C) Pr[A and B] = Pr[A] × Pr[B]
D) Pr[A or B] = Pr[A] × Pr[B]

Answer 3

B

Question 4

Which of the following statements is correct for a pair of independent events?

A) Pr[A and B] = Pr[A] + Pr[B]
B) Pr[A or B] = Pr[A] + Pr[B]
C) Pr[A and B] = Pr[A] × Pr[B]
D) Pr[A or B] = Pr[A] × Pr[B]

Question 5

If A and B are mutually exclusive events and Pr[A] = 0.2 and Pr[B] = 0.3, then what is Pr[A or B]?

A) 0.06
B) 0.5
C) 0.6
D) Cannot be determined from the information

Question 6

If A and B are independent events and Pr[A] = 0.2 and Pr[B] = 0.3, then what is Pr[A or B]?

A) 0.06
B) 0.5
C) 0.6
D) Cannot be determined from the information

Question 7

If A and B are mutually exclusive events and Pr[A] = 0.2 and Pr[B] = 0.3, then what is Pr[A and B]?

A) 0
B) 0.06
C) 0.5
D) 0.6

Question 8

If A and B are independent events and Pr[A] = 0.2 and Pr[B] = 0.3, then what is Pr[A and B]?

A) 0
B) 0.06
C) 0.5
D) 0.6

Question 9

Consider a collection of 100 birds where 20 of them are penguins, 25 are ostriches, 15 are hawks, and 40 are doves. Penguins and ostriches are unable to fly, whereas hawks and doves can. If a bird is randomly chosen from this population, what is the probability it will be a hawk?

A) 0.15
B) 0.20
C) 0.25
D) 0.40

Question 10

Consider a collection of 100 birds where 20 of them are penguins, 25 are ostriches, 15 are hawks, and 40 are doves. Penguins and ostriches are unable to fly, whereas hawks and doves can. If a bird is randomly chosen from this population, what is the probability it will not be a dove?

A) 0.40
B) 0.60
C) 0.75
D) 0.85

Question 11

Consider a collection of 100 birds where 20 of them are penguins, 25 are ostriches, 15 are hawks, and 40 are doves. Penguins and ostriches are unable to fly, whereas hawks and doves can. If a bird is randomly chosen from this population, what is the probability it will be a penguin or a dove?

A) 0
B) 0.20
C) 0.40
D) 0.60

Question 12

Consider a collection of 100 birds where 20 of them are penguins, 25 are ostriches, 15 are hawks, and 40 are doves. Penguins and ostriches are unable to fly, whereas hawks and doves can. If a bird is randomly chosen from this population, what is the probability it will be either a hawk or unable to fly?

A) 0
B) 0.20
C) 0.40
D) 0.60

Question 13

Consider a collection of 100 birds where 20 of them are penguins, 25 are ostriches, 15 are hawks, and 40 are doves. Penguins and ostriches are unable to fly, whereas hawks and doves can. If a bird is randomly chosen from this population, what is the probability it will be either a penguin or unable to fly?

A) 0.05
B) 0.25
C) 0.45
D) 0.65

Question 14

Consider a collection of 240 snakes where 40 of them are cobras, 60 are rattlesnakes, 60 are boas, and 80 are anacondas. Cobras and rattle snakes are venomous, whereas boas and anacondas are not. If a snake is randomly chosen from this population, what is the probability it will be a boa?

A) 0.167
B) 0.250
C) 0.333
D) 0.600

Question 15

Consider a collection of 240 snakes where 40 of them are cobras, 60 are rattlesnakes, 60 are boas, and 80 are anacondas. Cobras and rattle snakes are venomous, whereas boas and anacondas are not. If a snake is randomly chosen from this population, what is the probability it will not be venomous?

A) 0.167
B) 0.333
C) 0.417
D) 0.583

Question 16

Consider a collection of 240 snakes where 40 of them are cobras, 60 are rattlesnakes, 60 are boas, and 80 are anacondas. Cobras and rattle snakes are venomous, whereas boas and anacondas are not. If a snake is randomly chosen from this population, what is the probability it will be a cobra or a boa?

A) 0.167
B) 0.333
C) 0.417
D) 0.583

Question 17

Consider a collection of 240 snakes where 40 of them are cobras, 60 are rattlesnakes, 60 are boas, and 80 are anacondas. Cobras and rattle snakes are venomous, whereas boas and anacondas are not. If a snake is randomly chosen from this population, what is the probability it will be either a boa or venomous?

A) 0.167
B) 0.417
C) 0.583
D) 0.666

Question 18

Consider a collection of 240 snakes where 40 of them are cobras, 60 are rattlesnakes, 60 are boas, and 80 are anacondas. Cobras and rattle snakes are venomous, whereas boas and anacondas are not. If a snake is randomly chosen from this population, what is the probability it will be either a cobra or venomous?

A) 0.167
B) 0.417
C) 0.583
D) 0.666

Question 19

Consider a study in which trees of two species (elm and oak) were assayed to determine what the probabilities are of being infected with a disease. The data collected are shown in the accompanying table. Which of the mosaic plots below accurately represents the probability values in the data table?
?
Probability that trees will be infected with a disease.


$\begin{array}{lc}\text { Tree species and }\\\text { infection status } & \text { Probability } \\\hline \text { Oak tree, infected } & 0.40 \\\text { Oak tree, not infected } & 0.20 \\\text { Elm tree, infected } & 0.30 \\\text { Elm tree, not infected } & 0.10\end{array}$ ?
?

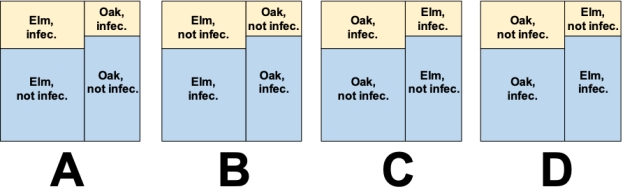A) Plot A
B) Plot B
C) Plot C
D) Plot D

Question 20

Consider a study in which swabs from the skin of a random sample of college students were tested for the presence of drug-resistant Staphylococcus Aureus. The data collected are shown in the accompanying table. Which of the mosaic plots below accurately represents the probability values in the data table?
?
Probability that male and female patients will carry drug-resistant Staphylococcus Aureus on skin.


$\begin{array}{lc}\text { Tree species and }\\\text { infection status } & \text { Probability } \\\hline \text { Male, carriers } & 0.10 \\\text { Male, non-carriers } & 0.35 \\\text { Female, carriers } & 0.20 \\\text { Female, non-carriers } & 0.35\end{array}$ ?

A) Plot A
B) Plot B
C) Plot C
D) Plot D

Question 21

Consider a study in which trees of two species (elm and oak) were assayed to determine what the probabilities are of being infected with a disease. The probabilities that resulted are shown in the probability tree. Which of the columns in the data table below accurately represents the probability values in the probability tree?
​

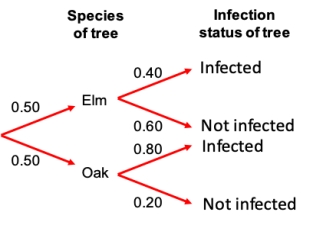
A) Plot A
B) Plot B
C) Plot C
D) Plot D

Question 22

Consider a study in which swabs from the skin of a random sample of college students were tested for the presence of drug-resistant Staphylococcus Aureus. The probabilities that resulted are shown in the probability tree. Which of the columns in the data table below accurately represents the probability values in the probability tree?
​

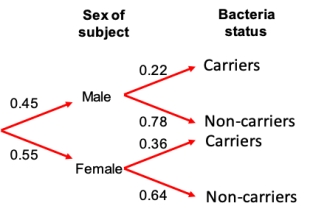
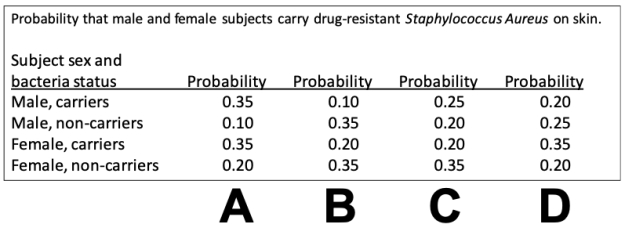
A) Plot A
B) Plot B
C) Plot C
D) Plot D

Question 23

Imagine we are catching fish from a small pond that contains 6 catfish, 10 perch, and 4 walleye. When we catch a fish, we return it to the water so it may be caught again. Individuals of all species are equally likely to be caught, and fish are no more or less likely to be caught a second time than they were the first. What is the probability of catching a catfish followed by a perch in the first two catches?

A) 0.150
B) 0.160
C) 0.480
D) 0.600

Question 24

Imagine we are catching fish from a small pond that contains 6 catfish, 10 perch, and 4 walleye. When we catch a fish, we return it to the water so it may be caught again. Individuals of all species are equally likely to be caught, and fish are no more or less likely to be caught a second time than they were the first. What is the probability of catching a catfish, followed by a perch, followed by a walleye in the first three catches?

A) 0.007
B) 0.015
C) 0.021
D) 0.030

Question 25

Imagine we are catching fish from a small pond that contains 6 catfish, 10 perch, and 4 walleye. When we catch a fish, we keep it in the boat and catch the next fish from the remaining fish in the pond. Individuals of all species are equally likely to be caught, and fish are no more or less likely to be caught a second time than they were the first. What is the probability of catching a catfish followed by a perch in the first two catches?

A) 0.150
B) 0.158
C) 0.526
D) 0.600

Question 26

Imagine we are catching fish from a small pond that contains 6 catfish, 10 perch, and 4 walleye. When we catch a fish, we keep it in the boat and catch the next fish from the remaining fish in the pond. Individuals of all species are equally likely to be caught, and fish are no more or less likely to be caught a second time than they were the first. What is the probability of catching a catfish, followed by a perch, followed by a walleye in the first three catches?

A) 0.007
B) 0.015
C) 0.035
D) 0.030

Question 27

Consider the probabilities of people taking HIV tests. Assume that the true probability of having HIV for all people who take the test is 3%. If HIV tests give a positive result in 99% of the cases in which the person has HIV and give a negative result in 99% of the cases in which the person does not have HIV, what is the probability that a person who gets a positive test result truly has HIV?

A) 0.50
B) 0.75
C) 0.95
D) 0.99

Question 28

Consider the probabilities of people taking HIV tests. Assume that the true probability of having HIV for all people who take the test is 4%. If HIV tests give a positive result in 99% of the cases in which the person has HIV and give a negative result in 99% of the cases in which the person does not have HIV, what is the probability that a person who gets a positive test result does not have HIV?

A) 0.125
B) 0.155
C) 0.165
D) 0.195

Question 29

Full body scans are becoming popular even for people who have no symptoms. At any given time, a person's risk of having lung cancer is approximately 1 in 1000. If a full body scan can detect lung cancer 95% of the time (i.e., gives a positive result when a person has lung cancer) but returns a positive result 1% of the time if a person does not have lung cancer, what is the probability that a person who gets a positive test result truly has lung cancer?

A) 0.012
B) 0.056
C) 0.087
D) 0.115

Question 30

Full body scans are becoming popular even for people who have no symptoms. At any given time, a person's risk of having lung cancer is approximately 1 in 1000. If a full body scan can detect lung cancer 95% of the time (i.e., gives a positive result when a person has lung cancer) but returns a positive result 2% of the time if a person does not have lung cancer, what is the probability that a person who gets a positive test result does not have lung cancer?

A) 0.955
B) 0.975
C) 0.995
D) 0.999

Question 31

Pairs of events that do not influence the probability of occurring for the other are called mutually exclusive.

Question 32

Pairs of events that cannot both occur are called mutually exclusive.

Question 33

Discrete probability distributions are used to model the probabilities of categorical variables.

Question 34

Discrete probability distributions are used to model the probabilities of all numerical variables.

Question 35

Discrete probability distributions are used to model the probabilities of continuous numerical variables.

Question 36

Continuous probability distributions are used to model the probabilities of all numerical variables.

Question 37

The addition rule states that Pr[A and B] = Pr[A] + Pr[B].

Question 38

The general addition rule states that Pr[A or B] = Pr[A] + Pr[B] - Pr[A and B]

Question 39

The general multiplication rule states that Pr[A and B] = Pr[A] × Pr[B].

Question 40

When we sample with replacement, we don't need to worry about conditional probabilities.

Question 41

When we sample without replacement, the probabilities of events may change as we take our sample.

Question 42

Bayes' theorem states that the sum of all mutually exclusive probabilities is one.

Question 43

Describe what a Venn diagram is and how it is used to compute probabilities.

Question 44

Describe a real-world example of something that has a probability of 10%.

Question 45

Describe the difference between discrete and continuous probability distributions. In particular, how are they different in how they compute probabilities for certain outcomes?

Question 46

Describe the difference between sampling with replacement and sampling without replacement with respect to calculating probabilities.

Question 47

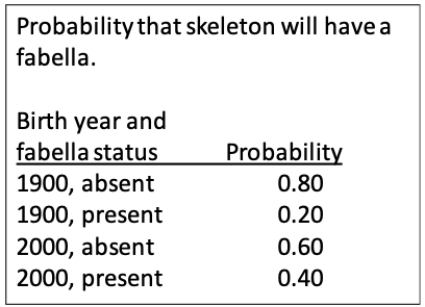
A fabella is a small bone in the knee found in a tendon behind the femur in some species of mammals, including humans. Historically, it has been rare, but recently it has become more common. Probability data for birth year and presence or absence of the bone is presented in the table. Imagine we examined 200 skeletons of individuals born in 1900 and 100 skeletons of individuals born in 2000. Draw a mosaic plot depicting the probabilities of a random individual from our study having or not having a fabella in their skeleton.
​
​

Question 48

A fabella is a small bone in the knee found in a tendon behind the femur in some species of mammals, including humans. Historically, it has been rare, but recently it has become more common. Probability data for birth year and presence or absence of the bone is presented in the table. Imagine we examined 200 skeletons of individuals born in 1900 and 100 skeletons of individuals born in 2000. Draw a probability tree diagram depicting the probabilities of a random individual from our study having or not having a fabella in their skeleton.
​

Question 49

Under what circumstances is drawing a probability tree most useful?

Question 50

When we make a probability tree, we look at outcomes for two different events and draw a diagram from left to right with one event and then the other. Invent a simple example with numbers and clearly show that the order we depict the events in a probability tree does not alter the final probabilities. (Hint: Draw two trees for the same probabilities)