Deck 8: Inferences About Proportions

Question 1

For a random sample of 20 children, 18 attended preschool and 2 did not attend preschool. Test the following hypotheses at the .05 level of significance:

$\begin{array}{l}H_{0}: \pi=75 \\H_{1}: \pi \neq .75\end{array}$

Answer 1

$z=\frac{p-\pi_{0}}{\sqrt{\frac{\pi_{0}\left(1-\pi_{0}\right)}{n}}}=\frac{.90-.75}{\sqrt{\frac{75(1-.75)}{20}}}=\frac{.15}{\sqrt{\frac{.75(.25)}{20}}}=\frac{.15}{.0968}=1.55$
Using the z table, we find critical values of +/-1.96 (split alpha in ½ given a two-tailed test thus 1 - .025 or .975; find P(z) = .975 in the table; this corresponds to z of 1.96). Since z of 1.55 (test statistic value) is less than our critical value (+1.96), we fail to reject the null hypothesis.

Question 2

For a random sample of 25 students, 20 passed the exam and 5 did not pass the exam. Test the following hypotheses at the .01 level of significance:

$\begin{array}{l}H_{0}: \pi=75 \\H_{1}: \pi \neq .75\end{array}$

Answer 2

$z=\frac{p-\pi_{0}}{\sqrt{\frac{\pi_{0}\left(1-\pi_{0}\right)}{n}}}=\frac{.80-.75}{\sqrt{\frac{75(1-.75)}{25}}}=\frac{.05}{\sqrt{\frac{75(.25)}{25}}}=\frac{.05}{.0866}=.577$
Using the z table, we find critical values of +/-2.57 (split alpha in ½ given a two-tailed test thus 1 - .005 or .995; find P(z) = .995 in the table; this corresponds to z of 2.57). Since z of .577 (test statistic value) is less than our critical value (+2.57), we fail to reject the null hypothesis.

Question 3

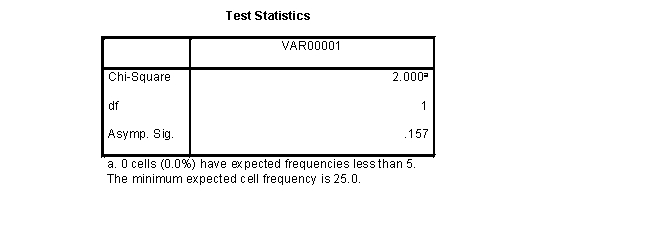
Lilo wants to know if equal proportions of students in her class know how to surf as compared to do not know how to surf. She randomly samples 50 students in her class and asks them if they know how to surf (yes or no). Twenty students indicate "no," that they do not know how to surf while 30 students indicate "yes," they do know how to surf. Using SPSS, conduct the appropriate statistical procedure at alpha of .05. Interpret the output including identifying the specific statistical procedure that has been used and reporting the extent to which the test is statistically significant. Include appropriate evidence (e.g., values from the output).

Answer 3

A chi-square goodness of fit was conducted to determine if equal proportions of students in Lilo's class know how to surf as compared to do not know how to surf. The test was not statistically significant, $\chi$ ² = 2.00, df = 1, p = .157.

Question 4

What is the measurement scale for variables that are used when examining inferences about proportions? Select all that apply.

A) Nominal
B) Ordinal
C) Interval
D) Ratio

Question 5

What are the characteristics of the Chi square distribution? Select all that apply.

A) Chi square values range from negative infinity to positive infinity.
B) The chi square distribution is a family of distributions dependent on degrees of freedom associated with the number of categories in the data.
C) The Chi square calculation yields a negative value.
D) Zero is the maximum value for chi square.

Question 6

What are the characteristics of the Chi square distribution? Select all that apply.

A) Degrees of freedom for the chi square distribution is based on the sample size.
B) The mean of the chi square distribution equals the degrees of freedom.
C) The variance of the chi square distribution equals the product of the sample size and the degrees of freedom.
D) Zero is the minimum value for chi square.