Deck 2: Exponential Notation & Logarithms
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/17
Play
Full screen (f)
Deck 2: Exponential Notation & Logarithms
1
What is the general rule for converting a number greater than 1 into exponential notation?
A) Move the decimal to the left until the number has a value between 1 and 10, and add "× 10ᵇ" where b is the number of places you moved the decimal.
B) Move the decimal to the right until the number has a value between 1 and 10, and add ?"× 10⁻ᵇ" where b is the number of places you moved the decimal.
C) Move the decimal to the left until the number has a value between 1 and 10, and add "× 10⁻ᵇ" where b is the number of places you moved the decimal.
D) Move the decimal to the right until the number has a value between 1 and 10, and add ?"× 10ᵇ" where b is the number of places you moved the decimal.
A) Move the decimal to the left until the number has a value between 1 and 10, and add "× 10ᵇ" where b is the number of places you moved the decimal.
B) Move the decimal to the right until the number has a value between 1 and 10, and add ?"× 10⁻ᵇ" where b is the number of places you moved the decimal.
C) Move the decimal to the left until the number has a value between 1 and 10, and add "× 10⁻ᵇ" where b is the number of places you moved the decimal.
D) Move the decimal to the right until the number has a value between 1 and 10, and add ?"× 10ᵇ" where b is the number of places you moved the decimal.
Move the decimal to the left until the number has a value between 1 and 10, and add "× 10ᵇ" where b is the number of places you moved the decimal.
2
Which of the following equations correctly simplifies the calculation of (5⁵)⁵?
A) 5⁽⁵⁺⁵⁾
B) 5⁽⁵⁻⁵⁾
C) 5⁽⁵×⁵⁾
D) 5⁽⁵÷⁵⁾
A) 5⁽⁵⁺⁵⁾
B) 5⁽⁵⁻⁵⁾
C) 5⁽⁵×⁵⁾
D) 5⁽⁵÷⁵⁾
5⁽⁵×⁵⁾
3
What is the natural logarithm of a number?
A) It is the exponential created from the logarithm of the number taken to the power of e.
B) It is the logarithm of the number to the base e.
C) It is the product of the logarithm of the number multiplied by the logarithm of e.
D) It is the quotient of e divided by the logarithm of the number.
A) It is the exponential created from the logarithm of the number taken to the power of e.
B) It is the logarithm of the number to the base e.
C) It is the product of the logarithm of the number multiplied by the logarithm of e.
D) It is the quotient of e divided by the logarithm of the number.
It is the logarithm of the number to the base e.
4
Which of the following is NOT an example of the usefulness of logarithms?
A) Graphs of logarithmic values make relationships between values more visible.
B) Logarithms simplify the expression of very large and very small numbers.
C) Logarithms can increase the inherent uncertainties of calculated ratios.
D) Logarithms can accelerate calculations.
A) Graphs of logarithmic values make relationships between values more visible.
B) Logarithms simplify the expression of very large and very small numbers.
C) Logarithms can increase the inherent uncertainties of calculated ratios.
D) Logarithms can accelerate calculations.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
5
What is the primary advantage of using exponential notation?
A) Reverse calculations involving exponential terms can be completed because the exponential term simply becomes negative.
B) The exponential term in an exponential number represents the slope of the line generated by the data set.
C) The significand in exponential terms is always reduced to a factor of ten to make calculations faster and easier.
D) Very large and very small numbers become more convenient to write and read in exponential notation.
A) Reverse calculations involving exponential terms can be completed because the exponential term simply becomes negative.
B) The exponential term in an exponential number represents the slope of the line generated by the data set.
C) The significand in exponential terms is always reduced to a factor of ten to make calculations faster and easier.
D) Very large and very small numbers become more convenient to write and read in exponential notation.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
6
What does the term e represent?
A) The algebraic conversion constant used to transform logarithmic values into linear ratios
B) The fundamental amount of change shared by all systems that grow or shrink exponentially and continuously
C) The inherent radius of all naturally occurring cyclical forms that defines the length or duration of the baseline cycle
D) The mathematical constant that is the ratio of the circumference of a circle to its diameter
A) The algebraic conversion constant used to transform logarithmic values into linear ratios
B) The fundamental amount of change shared by all systems that grow or shrink exponentially and continuously
C) The inherent radius of all naturally occurring cyclical forms that defines the length or duration of the baseline cycle
D) The mathematical constant that is the ratio of the circumference of a circle to its diameter

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
7
Which of the following equations can be used to simplify the calculation of log25⁶?
A) log25 + log6
B) log25 - log6
C) 6 × log25
D) 6 ÷ log25
A) log25 + log6
B) log25 - log6
C) 6 × log25
D) 6 ÷ log25

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
8
The ratio of substance X to substance Y in a solution is measured in two separate trials.The concentrations,ratios of X/Y,and log(X/Y)are presented in this table.
What is the log of X/Y from trial 2?
A) 0.301
B) -0.301
C) 3.01
D) -3.01
What is the log of X/Y from trial 2?
A) 0.301
B) -0.301
C) 3.01
D) -3.01

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
9
Calculators able to perform higher order mathematical calculations are widely available.So,why is it still necessary for people working in clinical and laboratory settings to know how to use exponential numbers and logarithms?
A) Errors are easily introduced in the typing of values.
B) There may be instances when calculators are unavailable.
C) To double-check values obtained from calculators
D) All of the above
A) Errors are easily introduced in the typing of values.
B) There may be instances when calculators are unavailable.
C) To double-check values obtained from calculators
D) All of the above

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
10
What value is represented by the exponential number 5.5 × 10⁴?
A) 0.0055
B) 5.5
C) 5500
D) 55,000
A) 0.0055
B) 5.5
C) 5500
D) 55,000

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
11
What is another way of writing "ln 100"?
A) log₍₂.₇₁₈₎100
B) log₍₁₀₀₎2.7182818
C) log₍ln₎100
D) log₍₁₀₎100
A) log₍₂.₇₁₈₎100
B) log₍₁₀₀₎2.7182818
C) log₍ln₎100
D) log₍₁₀₎100

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
12
In the clinical laboratory,what types of expressions are routinely used in calculations from applications such as spectroscopy,first-order processes,bacterial growth,and acidity?
A) Exponents
B) Logarithms
C) Quadratic equations
D) Both A and B are correct.
A) Exponents
B) Logarithms
C) Quadratic equations
D) Both A and B are correct.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
13
The term "common logarithm" applies to all logarithmic functions where
A) the inverse of the logarithm is used.
B) the logarithm is base-10.
C) the logarithm is base-e.
D) the logarithm uses an exponential term.
A) the inverse of the logarithm is used.
B) the logarithm is base-10.
C) the logarithm is base-e.
D) the logarithm uses an exponential term.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
14
The value of e can be determined with which equation?
A)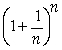
B) (1 + x)ⁿ
C)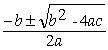
D) (x + a)ⁿ
A)
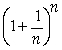
B) (1 + x)ⁿ
C)
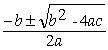
D) (x + a)ⁿ

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
15
Which of the following expressions represents the same information as "x‒ⁿ"?
A)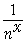
B)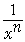
C)
D) logₓn
A)
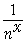
B)
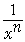
C)

D) logₓn

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
16
What is an alternate way in which the term eˣ can be written?
A)
B) loge
C) exp(x)
D)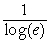
A)

B) loge
C) exp(x)
D)
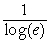

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
17
Which of the following is true about an arithmetic scale?
A) The distance between any two adjacent tick marks is the same as the distance between any other two adjacent tick marks.
B) The tick marks are spaced logarithmically in order to better visualize data encompassing a wide range of values.
C) The tick marks represent the data after conversion to exponential numbers to ensure a straight line is generated.
D) Both B and C are correct.
A) The distance between any two adjacent tick marks is the same as the distance between any other two adjacent tick marks.
B) The tick marks are spaced logarithmically in order to better visualize data encompassing a wide range of values.
C) The tick marks represent the data after conversion to exponential numbers to ensure a straight line is generated.
D) Both B and C are correct.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck


