Deck 12: Appendix A: Basic Algebra Review
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/45
Play
Full screen (f)
Deck 12: Appendix A: Basic Algebra Review
1
Indicate True (T) or False (F), and for each false statement find real number replacements for a, b, and c that will provide a counterexample.
-a - b = b - a
-a - b = b - a
False
2
Indicate True (T) or False (F), and for each false statement find real number replacements for a, b, and c that will provide a counterexample.
-(a - b) - c = a - (b - c)
-(a - b) - c = a - (b - c)
False
3
Indicate True (T) or False (F), and for each false statement find real number replacements for a, b, and c that will provide a counterexample.
-a + b = b + a
-a + b = b + a
True
4
Indicate True (T) or False (F), and for each false statement find real number replacements for a, b, and c that will provide a counterexample.
-(a - b) - c = a - (b - c)
-(a - b) - c = a - (b - c)

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
5
Indicate True (T) or False (F) by each statement.
-Every real number is a natural number.
-Every real number is a natural number.

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
6
Indicate True (T) or False (F) by each statement.
-An irrational number is not a real number.
-An irrational number is not a real number.

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
7
Indicate True (T) or False (F) by each statement.
-Every natural number is an integer.
-Every natural number is an integer.

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
8
Indicate True (T) or False (F) by each statement.
-An integer is a rational number.
-An integer is a rational number.

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
9
Given the sets N, Z, Q, and R, indicate to which set(s) each of the following numbers belong.
(A)
(B) e
(C) -2
(D) 8
(A)

(B) e
(C) -2
(D) 8

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
10
Find the product:
-( 3x + 12y)( 5x + 8y)
A)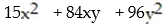
B)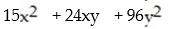
C)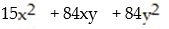
D)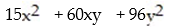
-( 3x + 12y)( 5x + 8y)
A)
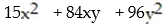
B)
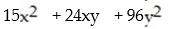
C)
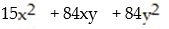
D)
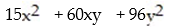

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
11
Find the product:
-
A)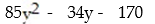
B)
C)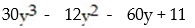
D)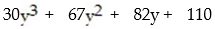
-

A)
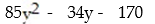
B)

C)
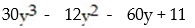
D)
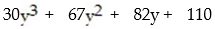

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
12
Find the product:
--3( 10r + 2) + 5( 2r + 3)
A) 7r - 1
B) -36r
C) -20r + 9
D) -20r + 2
--3( 10r + 2) + 5( 2r + 3)
A) 7r - 1
B) -36r
C) -20r + 9
D) -20r + 2

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
13
Factor the GCF from the polynomial:
-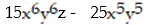
A)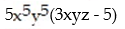
B)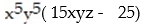
C)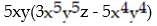
D)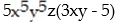
-
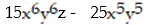
A)
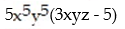
B)
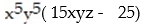
C)
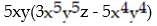
D)
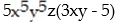

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
14
Factor completely using grouping:
-18y2 + 81y - 45
A) ( 18y - 9)(y + 5)
B) 9(2y + 1)(y - 5)
C) 9(2y - 1)(y + 5)
D) prime
-18y2 + 81y - 45
A) ( 18y - 9)(y + 5)
B) 9(2y + 1)(y - 5)
C) 9(2y - 1)(y + 5)
D) prime

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
15
Factor by grouping: 


Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
16
Factor the expression using integer coefficients: 


Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
17
Which polynomial can be factored using integer coefficients? Find its factored form.
A)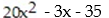
B)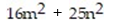
C)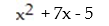
A)
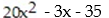
B)
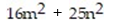
C)
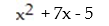

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
18
Factor using integer coefficients: 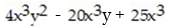
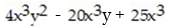

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
19
Simplify the expression:
-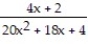
A)
B)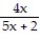
C)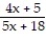
D)
-
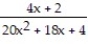
A)

B)
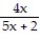
C)
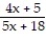
D)


Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
20
Perform the indicated operation and reduce to lowest terms: 


Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
21
Perform the indicated operation and reduce to lowest terms: 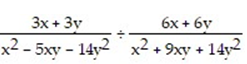
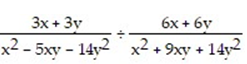

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
22
Combine into a single fraction and simplify: 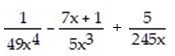
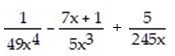

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
23
Combine into a single fraction and simplify: 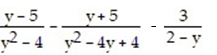
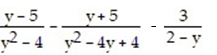

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
24
Express as a simple fraction reduced to lowest terms: 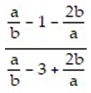
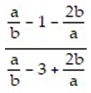

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
25
Simplify. The exponents in the answer should be positive integers:
-
A)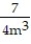
B)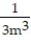
C)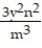
D)
-

A)
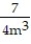
B)
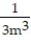
C)
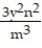
D)


Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
26
Simplify. The exponents in the answer should be positive integers:
-
A) -
B)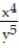
C)
D)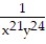
-

A) -

B)
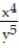
C)

D)
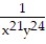

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
27
Simplify and express using positive exponents: 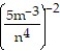
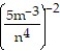

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
28
Simplify and express the answer using positive exponents: 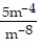
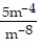

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
29
Write 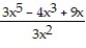 in the form
in the form 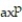 +
+ 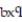 +
+ 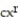 , where a, b, and c are real numbers and p, q and r are integers.
, where a, b, and c are real numbers and p, q and r are integers.
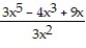 in the form
in the form 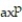 +
+ 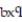 +
+ 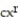 , where a, b, and c are real numbers and p, q and r are integers.
, where a, b, and c are real numbers and p, q and r are integers.
Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
30
Write 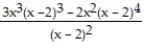 with positive exponents only, and as a single fraction reduced to lowest terms.
with positive exponents only, and as a single fraction reduced to lowest terms.
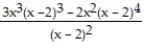 with positive exponents only, and as a single fraction reduced to lowest terms.
with positive exponents only, and as a single fraction reduced to lowest terms.
Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
31
Write 7.214 × 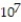 in standard notation.
in standard notation.
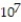 in standard notation.
in standard notation.
Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
32
According to the 2000 U.S. census, the population of the United States on April 1, 2000 was approximately 281,422,000 (Source: www.census.gov). The population of the U.S. in 1900 was approximately 76,212,000. Write both population numbers in scientific notation and use these expressions to calculate the ratio of the population in 2000 to that in 1900. Express the ratio in standard decimal form to four decimal places.

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
33
Rewrite the expression with a positive rational exponent. Simplify, if possible:
-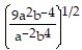
A)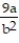
B)
C) 3a2b4
D)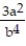
-
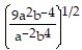
A)
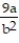
B)

C) 3a2b4
D)
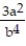

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
34
Simplify and express using positive exponents: 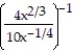
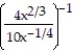

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
35
Change to simplest radical form: 


Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
36
Simplify and express the answer using positive exponents only: 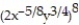
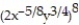

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
37
Change to simplest radical form: 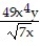
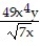

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
38
Write in simplest radical form: 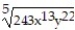

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
39
Change to simplest radical form: 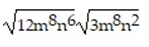

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
40
Rationalize the denominator and simplify. Assume that all variables represent positive real numbers:
-
A)
B)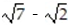
C)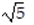
D)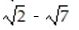
-

A)

B)
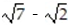
C)
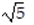
D)
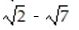

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
41
Use the square root property to solve the equation:
-(x + 3)2 = 12
A)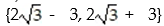
B)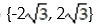
C)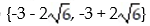
D)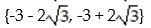
-(x + 3)2 = 12
A)
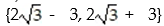
B)
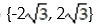
C)
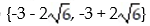
D)
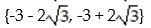

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
42
Solve by factoring: 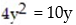
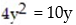

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
43
Use the quadratic formula to solve the equation:
-x2 + 18x + 71 = 0
A)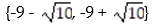
B)
C)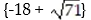
D)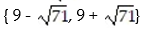
-x2 + 18x + 71 = 0
A)
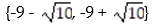
B)

C)
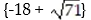
D)
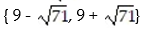

Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
44
Factor, if possible, as the product of two first-degree polynomials with integer coefficients: 


Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck
45
The supply and demand equations for a certain product are 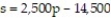 and
and 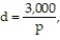 where p is the price in dollars. Find the price where supply equals demand.
where p is the price in dollars. Find the price where supply equals demand.
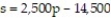 and
and 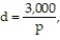 where p is the price in dollars. Find the price where supply equals demand.
where p is the price in dollars. Find the price where supply equals demand.
Unlock Deck
Unlock for access to all 45 flashcards in this deck.
Unlock Deck
k this deck


