Deck 8: Trigonometric Functions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/120
Play
Full screen (f)
Deck 8: Trigonometric Functions
1
The complex number 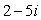 is a root of the quadratic equation
is a root of the quadratic equation 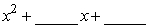 . Note: the quadratic equation has real coefficients.
. Note: the quadratic equation has real coefficients.
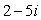 is a root of the quadratic equation
is a root of the quadratic equation 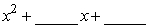 . Note: the quadratic equation has real coefficients.
. Note: the quadratic equation has real coefficients.a) -10
b)50
b)50
2

a)4.62
b)1.91
b)1.91
3

a)2
b)0
b)0
4
In polar form, the complex number -6i is written  , where r = _____ and
, where r = _____ and 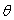 = _____. Round the second answer to 2 decimal places, and give
= _____. Round the second answer to 2 decimal places, and give 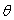 so that
so that 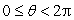
.
 , where r = _____ and
, where r = _____ and 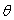 = _____. Round the second answer to 2 decimal places, and give
= _____. Round the second answer to 2 decimal places, and give 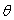 so that
so that 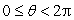
.

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
5
In polar form, the complex number 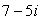 is written
is written 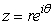 , where r = _____ and
, where r = _____ and 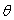 = _____. Round both answers to 2 decimal places, and give
= _____. Round both answers to 2 decimal places, and give 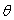 so that
so that 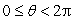 .
.
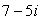 is written
is written 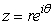 , where r = _____ and
, where r = _____ and 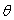 = _____. Round both answers to 2 decimal places, and give
= _____. Round both answers to 2 decimal places, and give 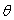 so that
so that 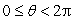 .
.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
6


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
7
Use deMoivre's formula to evaluate 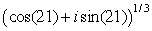 . Choose the root that lies in the first quadrant.
. Choose the root that lies in the first quadrant.
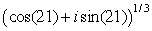 . Choose the root that lies in the first quadrant.
. Choose the root that lies in the first quadrant.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
8
Compute 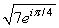 . Choose the root that lies in the first quadrant. Give your answer in Cartesian form, and round to 3 decimal places.
. Choose the root that lies in the first quadrant. Give your answer in Cartesian form, and round to 3 decimal places.
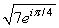 . Choose the root that lies in the first quadrant. Give your answer in Cartesian form, and round to 3 decimal places.
. Choose the root that lies in the first quadrant. Give your answer in Cartesian form, and round to 3 decimal places.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
9
Express 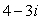 in polar form.
in polar form.
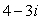 in polar form.
in polar form.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
10
Find the square root of 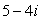 and give your answer in Cartesian form. Choose the root that lies in the fourth quadrant. Round your answer to 3 decimal places.
and give your answer in Cartesian form. Choose the root that lies in the fourth quadrant. Round your answer to 3 decimal places.
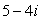 and give your answer in Cartesian form. Choose the root that lies in the fourth quadrant. Round your answer to 3 decimal places.
and give your answer in Cartesian form. Choose the root that lies in the fourth quadrant. Round your answer to 3 decimal places.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
11
Find the square root of 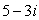 and give your answer in Cartesian form. Choose the root that lies in the second quadrant. Round your answer to 3 decimal places.
and give your answer in Cartesian form. Choose the root that lies in the second quadrant. Round your answer to 3 decimal places.
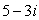 and give your answer in Cartesian form. Choose the root that lies in the second quadrant. Round your answer to 3 decimal places.
and give your answer in Cartesian form. Choose the root that lies in the second quadrant. Round your answer to 3 decimal places.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
12
Compute 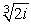 and give your answer in Cartesian form. Choose the root that lies in the first quadrant. Round your answer to 3 decimal places.
and give your answer in Cartesian form. Choose the root that lies in the first quadrant. Round your answer to 3 decimal places.
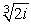 and give your answer in Cartesian form. Choose the root that lies in the first quadrant. Round your answer to 3 decimal places.
and give your answer in Cartesian form. Choose the root that lies in the first quadrant. Round your answer to 3 decimal places.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
13
Find the Cartesian form for all 3 cube roots of 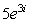 . Round your answers to 3 decimal places.
. Round your answers to 3 decimal places.
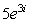 . Round your answers to 3 decimal places.
. Round your answers to 3 decimal places.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
14
The roots of 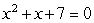 are complex numbers. Find the roots.
are complex numbers. Find the roots.
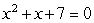 are complex numbers. Find the roots.
are complex numbers. Find the roots.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
15
The roots of  are complex numbers. Find the roots.
are complex numbers. Find the roots.
 are complex numbers. Find the roots.
are complex numbers. Find the roots.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
16
Write 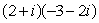 in Cartesian form.
in Cartesian form.
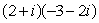 in Cartesian form.
in Cartesian form.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
17
Express 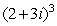 in polar form.
in polar form.
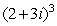 in polar form.
in polar form.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
18
Express 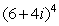 in polar form.
in polar form.
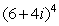 in polar form.
in polar form.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
19
Express 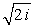 in polar form.
in polar form.
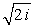 in polar form.
in polar form.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
20
Use de Moivre's formula to evaluate 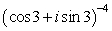 . Round your answer to 3 decimal places.
. Round your answer to 3 decimal places.
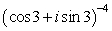 . Round your answer to 3 decimal places.
. Round your answer to 3 decimal places.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
21
What is the Cartesian equation for the circle shown below? 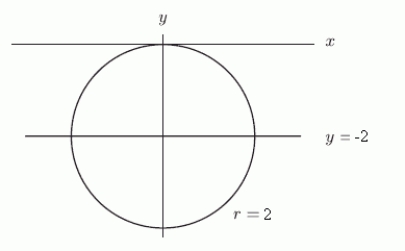
A)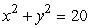
B)
C)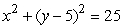
D)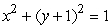

A)
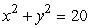
B)

C)
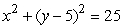
D)
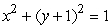

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
22
Do all of the points satisfying  , for
, for 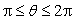 , lie on the circle shown below?
, lie on the circle shown below?
 , for
, for 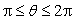 , lie on the circle shown below?
, lie on the circle shown below?

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
23
The origin is at the center of a clock, with the positive x-axis going through 3 and the positive y-axis going through 12. The hour hand is 9 cm long and the minute hand is 11 cm long. The Cartesian coordinates of the minute hand at 11:00 pm are ( _____, _____ ). Round both answers to 2 decimal places.

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
24
The origin is at the center of a clock, with the positive x-axis going through 3 and the positive y-axis going through 12. The hour hand is 9 cm long and the minute hand is 11 cm long. The polar coordinates of the hour hand at 11:15 am are ( _____, _____ ). Round both answers to 2 decimal places.

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
25
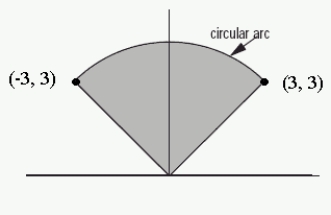
The inequalities 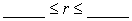 and
and 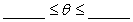 describe the region below. Round the last 2 answers to 3 decimal places, and be sure both are between 0 and
describe the region below. Round the last 2 answers to 3 decimal places, and be sure both are between 0 and 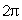 .
.
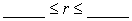 and
and 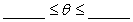 describe the region below. Round the last 2 answers to 3 decimal places, and be sure both are between 0 and
describe the region below. Round the last 2 answers to 3 decimal places, and be sure both are between 0 and 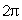 .
.

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
26
The cube root of 4-i which is in the second quadrant is equal to _____ + _____ i. Round both answers to 3 decimal places.

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
27
Simplify 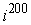
.
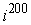
.

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
28
Convert the polar equation 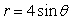 to a Cartesian equation.
to a Cartesian equation.
A)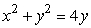
B)
C)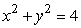
D)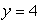
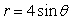 to a Cartesian equation.
to a Cartesian equation.A)
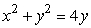
B)

C)
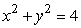
D)
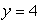

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
29
Convert the equation 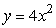 to a polar equation.
to a polar equation.
A)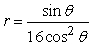
B)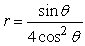
C)
D)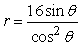
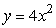 to a polar equation.
to a polar equation.A)
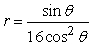
B)
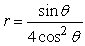
C)

D)
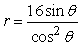

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
30
What are the polar inequalities that describe the cross section of a doughnut centered at the origin with a 5 inch diameter and a 1 inch hole?
A)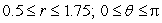
B)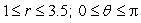
C)
D)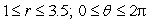
A)
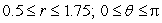
B)
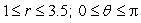
C)

D)
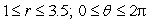

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
31
Describe the graph of the polar equation 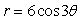
.
A) A 4-petaled rose, each petal of length 3.
B) A 2-petaled rose, each petal of length 3.
C) A 3-petaled rose, each petal of length 2.
D) A 2-petaled rose, each petal of length 2.
E)
None of the above
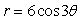
.
A) A 4-petaled rose, each petal of length 3.
B) A 2-petaled rose, each petal of length 3.
C) A 3-petaled rose, each petal of length 2.
D) A 2-petaled rose, each petal of length 2.
E)
None of the above

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
32
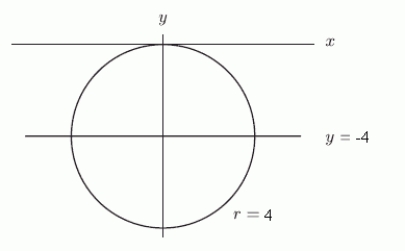
Which of the following are equations for the circle shown below? (The first two are Cartesian equations; the second two are polar equations) 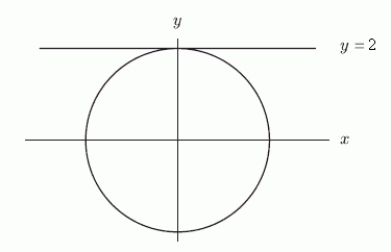
A)
B)
C)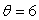
D)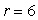

A)

B)

C)
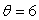
D)
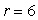

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
33
In which quadrant is a point with the polar coordinate -7 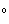 ?
?
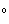 ?
?
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
34
In which quadrant is a point with the polar coordinate -1.9 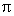 ?
?
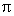 ?
?
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
35
The polar coordinates of the Cartesian point (4, -5) are r = ______, 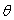 = ______. Round both answers to 2 decimal points, and give
= ______. Round both answers to 2 decimal points, and give  so that
so that 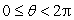 .
.
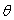 = ______. Round both answers to 2 decimal points, and give
= ______. Round both answers to 2 decimal points, and give  so that
so that 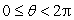 .
.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
36
The Cartesian coordinates of the polar point (1.5, -2.25 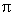 ) are x = ______ and y = ______. Round both answers to 2 decimal places.
) are x = ______ and y = ______. Round both answers to 2 decimal places.
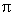 ) are x = ______ and y = ______. Round both answers to 2 decimal places.
) are x = ______ and y = ______. Round both answers to 2 decimal places.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
37
Convert 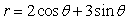 to rectangular coordinates. Classify the graph if possible.
to rectangular coordinates. Classify the graph if possible.
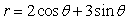 to rectangular coordinates. Classify the graph if possible.
to rectangular coordinates. Classify the graph if possible.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
38
Write the polar equation of a circle of radius 4 centered at the origin.

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
39
What is the equation in polar coordinates of a vertical line through the point
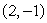
.
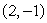
.

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
40
Use inequalities to describe a ring centered at the origin whose outer radius is 2 and inner radius is 9 in polar coordinates.

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
41
At which of the following values of x do the graphs of 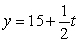 And
And 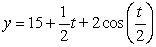 intersect?
intersect?
A)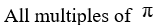
B)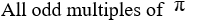
C)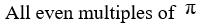
D) None of the above
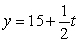 And
And 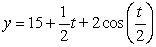 intersect?
intersect?A)
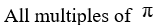
B)
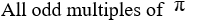
C)
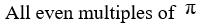
D) None of the above

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
42
Use the inverse sine function to estimate the solution to the equation  for
for 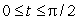 . Round to 2 decimal places.
. Round to 2 decimal places.
 for
for 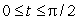 . Round to 2 decimal places.
. Round to 2 decimal places.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
43
Find a solution in the interval  for
for 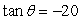 . Round to 3 decimal places.
. Round to 3 decimal places.
 for
for 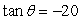 . Round to 3 decimal places.
. Round to 3 decimal places.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
44
Use a graph of 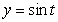 to estimate the solution to the equation
to estimate the solution to the equation 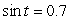 for
for 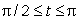 . Round to 2 decimal places.
. Round to 2 decimal places.
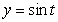 to estimate the solution to the equation
to estimate the solution to the equation 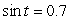 for
for 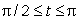 . Round to 2 decimal places.
. Round to 2 decimal places.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
45
Which of the following are x-intercepts of the function 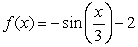 ?
?
A) 0
B)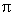
C)
D)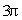
E)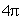
F)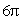
G) None of the above
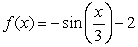 ?
?A) 0
B)
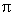
C)

D)
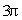
E)
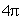
F)
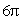
G) None of the above

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
46
Find all solutions to 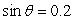 on the interval
on the interval 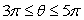 . Give your answers correct to 3 decimal places.
. Give your answers correct to 3 decimal places.
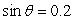 on the interval
on the interval 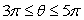 . Give your answers correct to 3 decimal places.
. Give your answers correct to 3 decimal places.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
47
Find all solutions to the equation 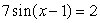 for
for 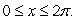 Give your answers to 3 decimal places.
Give your answers to 3 decimal places.
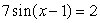 for
for 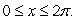 Give your answers to 3 decimal places.
Give your answers to 3 decimal places.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
48
The baseball field's usage (in people per week) is seasonal with the peak in mid-July and the low in mid-January. The usage is 1500 in July and 500 in January. Find a trig function 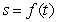 representing the usage at time t months after mid-January.
representing the usage at time t months after mid-January.
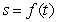 representing the usage at time t months after mid-January.
representing the usage at time t months after mid-January.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
49
Which of the following are defined?
A)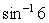
B)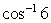
C)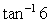
D)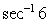
E)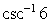
F)
A)
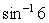
B)
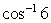
C)
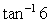
D)
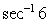
E)
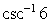
F)


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
50
Find all values of 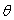 such that
such that 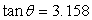 and
and 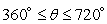 . Give answers correct to 3 decimal places.
. Give answers correct to 3 decimal places.
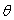 such that
such that 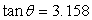 and
and 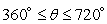 . Give answers correct to 3 decimal places.
. Give answers correct to 3 decimal places.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
51
Find all solutions (if possible) with t in radians: 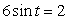 . Give answers correct to 3 decimal places.
. Give answers correct to 3 decimal places.
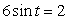 . Give answers correct to 3 decimal places.
. Give answers correct to 3 decimal places.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
52
Find all solutions (if possible) with t in radians: 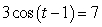 . Give answers correct to 3 decimal places.
. Give answers correct to 3 decimal places.
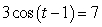 . Give answers correct to 3 decimal places.
. Give answers correct to 3 decimal places.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
53
Find all solutions to 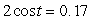 for
for 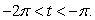 Give answers correct to 3 decimal places.
Give answers correct to 3 decimal places.
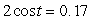 for
for 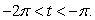 Give answers correct to 3 decimal places.
Give answers correct to 3 decimal places.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
54
Find all solutions to 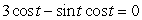 for
for 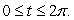 Give answers correct to 3 decimal places.
Give answers correct to 3 decimal places.
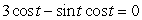 for
for 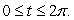 Give answers correct to 3 decimal places.
Give answers correct to 3 decimal places.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
55
How many solutions to 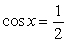 are there for
are there for 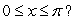
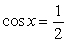 are there for
are there for 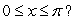

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
56
How many solutions to 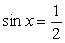 are there for
are there for 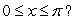
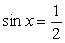 are there for
are there for 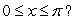

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
57
How many solutions to 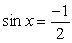 are there for
are there for 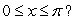
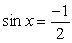 are there for
are there for 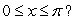

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
58
Solve 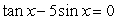 for
for 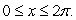
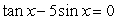 for
for 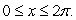

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
59
Solve 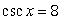 for
for 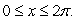
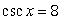 for
for 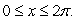

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
60
Solve  for
for 
 for
for 

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
61
What is the amplitude of 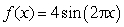 ?
?
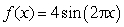 ?
?
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
62
Consider the function 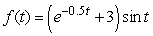 . What happens to the graph of
. What happens to the graph of 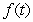 as
as 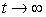 ?
?
A)
B)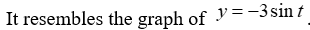
C)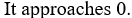
D)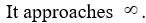
E)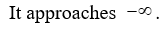
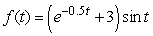 . What happens to the graph of
. What happens to the graph of 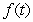 as
as 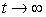 ?
?A)

B)
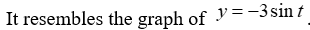
C)
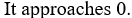
D)
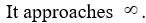
E)
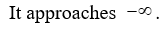

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
63
Constructive and destructive interference are defined in the following figure from an engineering textbook. 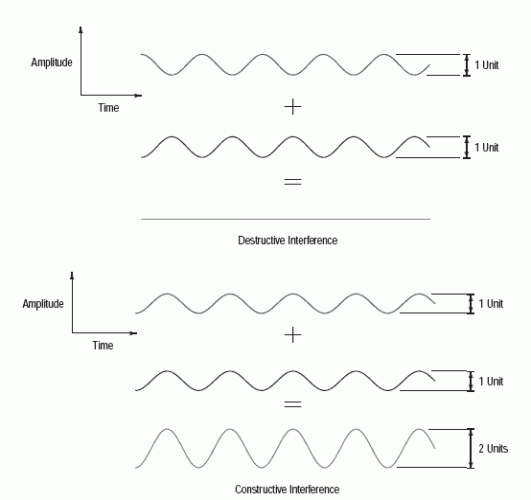 Let
Let 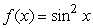 ,
,
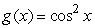 ,
,
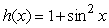 , and
, and  . Do f(x) and h(x) have constructive interference, destructive interference, or neither?
. Do f(x) and h(x) have constructive interference, destructive interference, or neither?
 Let
Let 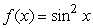 ,
,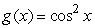 ,
,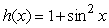 , and
, and  . Do f(x) and h(x) have constructive interference, destructive interference, or neither?
. Do f(x) and h(x) have constructive interference, destructive interference, or neither?
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
64
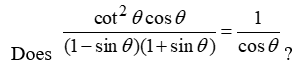

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
65
Use a calculator to graph the function  on the interval
on the interval 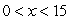 .
.

has a minimum value of _____ at x = _____. Round both answers to 2 decimal places.
 on the interval
on the interval 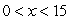 .
.
has a minimum value of _____ at x = _____. Round both answers to 2 decimal places.

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
66
Without a calculator, find the exact value of 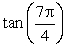 . If it is undefined, enter "undefined".
. If it is undefined, enter "undefined".
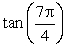 . If it is undefined, enter "undefined".
. If it is undefined, enter "undefined".
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
67
Which of the following quotients is equivalent to 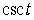 ?
?
A)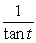
B)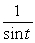
C)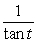
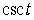 ?
?A)
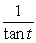
B)
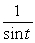
C)
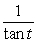

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
68
Without a calculator, find the exact value of  . If it is undefined, enter "undefined".
. If it is undefined, enter "undefined".
 . If it is undefined, enter "undefined".
. If it is undefined, enter "undefined".
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
69
Without a calculator, find the exact value of 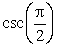 . If it is undefined, enter "undefined".
. If it is undefined, enter "undefined".
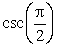 . If it is undefined, enter "undefined".
. If it is undefined, enter "undefined".
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
70
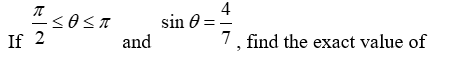
a)

b)
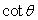

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
71
If 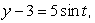 find an expression for
find an expression for 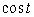 in terms of y.
in terms of y.
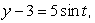 find an expression for
find an expression for 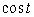 in terms of y.
in terms of y.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
72
Simplify 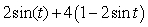 .
.
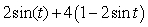 .
.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
73
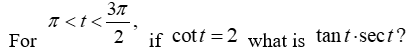

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
74
Simplify 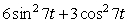 .
.
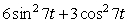 .
.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
75
Using the identity  find an angle
find an angle 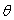 such that
such that 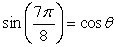 .
.
 find an angle
find an angle 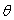 such that
such that 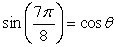 .
.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
76
Let P be a point on the unit circle with coordinates 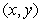 corresponding to an angle of t radians. Write
corresponding to an angle of t radians. Write 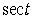
in terms of x and y.
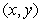 corresponding to an angle of t radians. Write
corresponding to an angle of t radians. Write 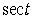
in terms of x and y.

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
77
Let P be a point on the unit circle with coordinates 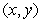 corresponding to an angle of t radians. Write
corresponding to an angle of t radians. Write 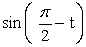 in terms of x and y.
in terms of x and y.
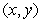 corresponding to an angle of t radians. Write
corresponding to an angle of t radians. Write 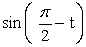 in terms of x and y.
in terms of x and y.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
78
Let P be a point on the unit circle with coordinates  corresponding to an angle of t radians. Write
corresponding to an angle of t radians. Write 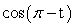 in terms of x and y.
in terms of x and y.
 corresponding to an angle of t radians. Write
corresponding to an angle of t radians. Write 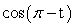 in terms of x and y.
in terms of x and y.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
79
Simplify  (For values for which it is defined).
(For values for which it is defined).
A)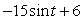
B)
C)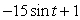
D)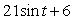
 (For values for which it is defined).
(For values for which it is defined).A)
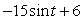
B)

C)
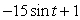
D)
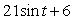

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
80
Simplify  (for values of the variable for which it is defined).
(for values of the variable for which it is defined).
A)
B)
C)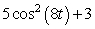
D) None of the above.
1)5
 (for values of the variable for which it is defined).
(for values of the variable for which it is defined).A)

B)

C)
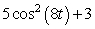
D) None of the above.
1)5

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck


