Deck 3: Basic Game Theory: Games Between Two Players

Question 1

A method of analyzing the strategic interaction that occurs between small numbers of people, firms, organizations, or countries is called

A) clinical observation.
B) microdemographics.
C) forensic economics.
D) game theory.

Answer 1

game theory.

Question 2

Anticipating the decisions others will make in response to your decision while knowing the others are anticipating your response is known as

A) strategic interaction.
B) the wait-and-see game.
C) psychic intuition.
D) best-response analysis.

Answer 2

strategic interaction.

Question 3

The characteristic of games whereby the outcome of the game depends not only on what you do but on what the other players do in response is called

A) strategic interaction.
B) mutual interdependence.
C) optimal choice analysis.
D) decision theory.

Answer 3

mutual interdependence.

Question 4

Game theory is a method of analyzing the strategic interaction that occurs between small numbers of

A) people.
B) firms.
C) countries.
D) All of the above are correct.

Question 5

Which of the following situations is least likely to involve strategic interaction?

A) two countries deciding whether to impose trade restrictions on each other
B) competing convenience stores deciding how much to charge for coffee
C) two customers deciding which toaster to buy from Target
D) a state legislature deciding whether to legalize casino gambling

Question 6

Which of the following situations is least likely to involve mutual interdependence?

A) Canada is thinking about banning all imported beef from the United States.
B) Octavia is thinking about trading in her Toyota Prius for a BMW 220i.
C) Walmart is considering moving up the start-time for its Black Friday sales to 12:01 AM on Thanksgiving day.
D) McDonald's is thinking about lowering all its menu prices by 50 percent.

Question 7

When playing a game, you need to

A) anticipate the moves others might be making.
B) choose a strategy based on the move you anticipate from your rival.
C) both A and B
D) neither A nor B

Question 8

Choosing a pair of shoes that fit your feet most comfortably describes both a decision and a game.

Question 9

When you are playing a game, you must anticipate the move that other players might be making.

Question 10

Of the following situations, explain which are best described as games and which are best described as just decisions:
a. Starbucks is deciding whether to offer a new, 40 oz. option called the Uberventi.
b. Sasha is deciding whether to order a grande latte or venti latte from Starbucks.
c. The people of Catalonia are deciding whether to vote for independence from Spain.
d. MGM Resorts International is preparing to bid to obtain the last available casino license in Macao.
e. The Hollywood Hills Homeowner Association is debating the landscape design for the renovation of the main entrance to their neighborhood.

Question 11

A structured and simplified version of reality that can be used to explain real-world behavior is called

A) an economic model.
B) a rationality assumption.
C) a postulate.
D) a normative alternate reality.

Question 12

A payoff matrix summarizes all of the following except

A) who the players are.
B) the reason each player is playing the game.
C) the actions available to each player.
D) the payoffs available to each player.

Question 13

A Nash equilibrium is an outcome where

A) both players are playing their best strategy, given the strategy chosen by the opponent.
B) both players are playing their best strategy, regardless of the strategy chosen by the opponent.
C) only one player can play his or her best strategy due to the strategy chosen by the opponent.
D) neither player has a best strategy to play.

Question 14

The person often credited with inventing modern game theory is

A) John Nash.
B) Albert Einstein.
C) John von Neumann.
D) Isaac Newton.

Question 15

A strategy which is universally best, regardless of the strategy chosen by others, is called a

A) Nash strategy.
B) dominant strategy.
C) mutually interdependent strategy.
D) zero-sum strategy.

Question 16

Leona and Bernie have committed a crime. If Leona denies committing the crime, Bernie can lower his penalty from 5 years to 2 years by confessing to the crime. If Leona confesses to the crime, Bernie can lower his penalty from 18 years to 7 years by confessing to the crime. Based on this information, we know with certainty that

A) Bernie's dominant strategy is to deny the crime.
B) Bernie has no dominant strategy.
C) Bernie's dominant strategy is to confess to the crime.
D) Bernie's dominant strategy is to confess to the crime only if Leona denies committing the crime.

Question 17

If both players in a game have a dominant strategy, then the game they are playing

A) has no Nash equilibrium.
B) must have one Nash equilibrium.
C) must have two Nash equilibria - one for each player.
D) More information is needed to determine the number of Nash equilibria in the game.

Question 18

Figure 3.1 : Homer and Marge have been accused of committing a crime, and the payoff matrix below represents the payoffs associated with confessing to the crime and denying having committed the crime.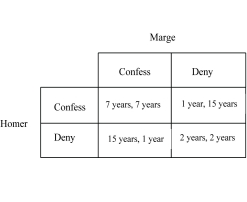

-Refer to Figure 3.1. If Homer confesses to the crime and Marge does not, what is Homer's payout?

A) 1 year
B) 2 years
C) 7 years
D) 15 years

Question 19

Figure 3.1 : Homer and Marge have been accused of committing a crime, and the payoff matrix below represents the payoffs associated with confessing to the crime and denying having committed the crime.

-Refer to Figure 3.1. If Marge confesses to the crime and Homer does not, what is Homer's payout?

A) 1 year
B) 2 years
C) 7 years
D) 15 years

Question 20

Figure 3.1 : Homer and Marge have been accused of committing a crime, and the payoff matrix below represents the payoffs associated with confessing to the crime and denying having committed the crime.

-Refer to Figure 3.1. If Marge and Homer both confess to the crime, what is Marge's payout?

A) 1 year
B) 2 years
C) 7 years
D) 15 years

Question 21

Figure 3.1 : Homer and Marge have been accused of committing a crime, and the payoff matrix below represents the payoffs associated with confessing to the crime and denying having committed the crime.

-Refer to Figure 3.1. If Marge and Homer both deny committing the crime, what is Marge's payout?

A) 1 year
B) 2 years
C) 7 years
D) 15 years

Question 22

Figure 3.1 : Homer and Marge have been accused of committing a crime, and the payoff matrix below represents the payoffs associated with confessing to the crime and denying having committed the crime.

-Refer to Figure 3.1. What is the number of outcomes which represent Nash equilibria in this game?

A) 1
B) 2
C) 3
D) 4

Question 23

Figure 3.1 : Homer and Marge have been accused of committing a crime, and the payoff matrix below represents the payoffs associated with confessing to the crime and denying having committed the crime.

-Refer to Figure 3.1. A Nash Equilibrium occurs where

A) Homer confesses to committing the crime and Marge denies committing the crime.
B) Marge confesses to committing the crime and Homer denies committing the crime.
C) Homer and Marge both confess to committing the crime.
D) Homer and Marge both deny committing the crime.

Question 24

Figure 3.1 : Homer and Marge have been accused of committing a crime, and the payoff matrix below represents the payoffs associated with confessing to the crime and denying having committed the crime.

-Refer to Figure 3.1. Homer's dominant strategy is to ________ committing the crime, and Marge's dominant strategy is to ________ committing the crime.

A) confess to; confess to
B) confess to; deny
C) deny; confess to
D) deny; deny

Question 25

Figure 3.2: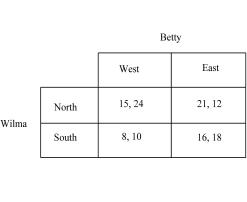

-Refer to Figure 3.2. If Wilma plays North and Betty plays West, what is Wilma's payout?

A) 10
B) 15
C) 21
D) 24

Question 26

Figure 3.2:

-Refer to Figure 3.2. If Wilma plays North and Betty plays East, what is Betty's payout?

A) 12
B) 15
C) 16
D) 21

Question 27

Figure 3.2:

-Refer to Figure 3.2. Betty's dominant strategy is

A) North.
B) West.
C) East.
D) Betty has no dominant strategy.

Question 28

Figure 3.2:

-Refer to Figure 3.2. The Nash equilibrium is where

A) Wilma plays North and Betty plays West.
B) Wilma plays North and Betty plays East.
C) Wilma plays South and Betty plays East.
D) There is no Nash equilibrium in this game.

Question 29

A dominant strategy refers to a strategy where one player knows he will always beat the other player.

Question 30

If only one player in a game has a dominant strategy, there can be no Nash equilibrium.

Question 31

A table that summarizes who the players in a game are, the actions available to each player, and the payoffs available to each player is a ________.

Question 32

In a game, what is meant when a player is said to have a dominant strategy?

Question 33

Figure 3.3 :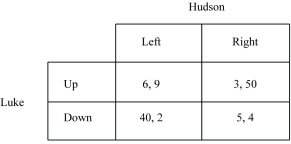
-Refer to Figure 3.3. Based on the information in the above payoff matrix, identify each player's dominant strategy (if there is one) and the location of the Nash equilibrium.

Question 34

A game in which each player has a dominant strategy of defecting, and each ends up worse off than if they had both cooperated is called

A) a pure coordination game.
B) a prisoner's dilemma game.
C) an assurance game.
D) a battle of the sexes game.

Question 35

Which of the following is not a characteristic of a prisoner's dilemma game?

A) Defecting is a dominant strategy for each player.
B) Each player has two strategies: cooperate or defect.
C) There is a single Nash equilibrium.
D) The Nash equilibrium is the best outcome for each individual and for the group.

Question 36

Tobacco companies did not resist the advertising ban instituted in 1970 because they actually increased profits by not being allowed to advertise. This ban forced these companies to abandon their dominant strategy of advertising and wind up at a cooperative outcome of not advertising. Prior to the ban, the tobacco companies were involved in playing

A) a pure coordination game.
B) a prisoner's dilemma game.
C) an assurance game.
D) a battle of the sexes game.

Question 37

The assumption that given the strategy chosen by the other participant, a player will always choose the strategy that brings him or her the best payoff is called

A) strategic interaction.
B) economic self interest.
C) the rationality assumption.
D) the profit-maximizing assumption.

Question 38

A game where it only matters that the players work together to agree on an outcome, not which outcome they agree upon, is called

A) a pure coordination game.
B) a prisoner's dilemma game.
C) an assurance game.
D) a battle of the sexes game.

Question 39

A technique for locating equilibria by marking the best strategy a player can use to counter each of his or her rival's possible moves is called

A) a win-win situation.
B) cooperative coordination.
C) cell-by-cell inspection.
D) best-response analysis.

Question 40

The statement "If Harvey goes to Subway then Candice should go to Subway, and if Harvey goes to Taco Bell, then Candice should go to Taco Bell"is an example of locating equilibria by using the technique known as

A) mutual interdependence.
B) cooperative coordination.
C) cell-by-cell inspection.
D) best-response analysis.

Question 41

All of the following are characteristics of the pure coordination game except

A) Nash equilibria exist at every outcome where players successfully coordinate.
B) each player has a dominant strategy.
C) the payoff for coordinating is higher than the payoff for not coordinating.
D) the two general outcomes are that the players either coordinate with each other or they do not.

Question 42

Figure 3.4 : 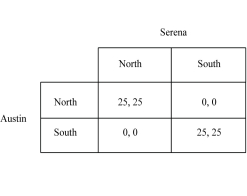Serena and Austin are spending their 5th wedding anniversary on the remote island of Wabo Wabo. One afternoon, Serena decides to go exploring around the island while Austin chooses to go snorkeling. Earlier in the day, they both decided to get together at dusk to watch the sunset, but neglected to agree where to meet. They both know that the two prime spots for watching the sun go down are at the northernmost point of the island and at the southernmost point of the island. They each have time to only go to one end of the island and they cannot communicate with each other because there is no cell service on the island. Neither Serena nor Austin care about at which end of the island they view the sunset. They only care that they are together when they do. The payoffs for each player are shown in the above payoff matrix.

-Refer to Figure 3.4. This is an example of

A) an assurance game.
B) a pure coordination game.
C) a battle of the sexes game.
D) a chicken game.

Question 43

Figure 3.4 : Serena and Austin are spending their 5th wedding anniversary on the remote island of Wabo Wabo. One afternoon, Serena decides to go exploring around the island while Austin chooses to go snorkeling. Earlier in the day, they both decided to get together at dusk to watch the sunset, but neglected to agree where to meet. They both know that the two prime spots for watching the sun go down are at the northernmost point of the island and at the southernmost point of the island. They each have time to only go to one end of the island and they cannot communicate with each other because there is no cell service on the island. Neither Serena nor Austin care about at which end of the island they view the sunset. They only care that they are together when they do. The payoffs for each player are shown in the above payoff matrix.

-Refer to Figure 3.4. Serena's dominant strategy is to play ________ and Austin's dominant strategy is to play ________.

A) North; North
B) South; South
C) North; South
D) Neither Serena nor Austin has a dominant strategy.

Question 44

Figure 3.4 : Serena and Austin are spending their 5th wedding anniversary on the remote island of Wabo Wabo. One afternoon, Serena decides to go exploring around the island while Austin chooses to go snorkeling. Earlier in the day, they both decided to get together at dusk to watch the sunset, but neglected to agree where to meet. They both know that the two prime spots for watching the sun go down are at the northernmost point of the island and at the southernmost point of the island. They each have time to only go to one end of the island and they cannot communicate with each other because there is no cell service on the island. Neither Serena nor Austin care about at which end of the island they view the sunset. They only care that they are together when they do. The payoffs for each player are shown in the above payoff matrix.

-Refer to Figure 3.4. The odds of Serena and Austin meeting randomly to view the sunset are

A) 0%.
B) 25%.
C) 50%.
D) 100%.

Question 45

Figure 3.4 : Serena and Austin are spending their 5th wedding anniversary on the remote island of Wabo Wabo. One afternoon, Serena decides to go exploring around the island while Austin chooses to go snorkeling. Earlier in the day, they both decided to get together at dusk to watch the sunset, but neglected to agree where to meet. They both know that the two prime spots for watching the sun go down are at the northernmost point of the island and at the southernmost point of the island. They each have time to only go to one end of the island and they cannot communicate with each other because there is no cell service on the island. Neither Serena nor Austin care about at which end of the island they view the sunset. They only care that they are together when they do. The payoffs for each player are shown in the above payoff matrix.

-Refer to Figure 3.4. A Nash equilibrium is achieved at which cell(s) in the payoff matrix?

A) only at (North, North)
B) only at (South, South)
C) at both (North, North) and at (South, South)
D) There is no Nash equilibrium.

Question 46

A game in which players want to coordinate on an outcome and in which both agree that coordinating on one particular outcome is preferred to coordinating on the other is called

A) an assurance game.
B) a pure coordination game.
C) a battle of the sexes game.
D) a chicken game.

Question 47

Which of the following is not a characteristic of an assurance game?

A) Neither player has a dominant strategy.
B) Nash equilibria exist at every outcome where the players successfully coordinate.
C) Each of the Nash equilibria offers identical payoffs to any particular player.
D) The payoff for coordinating is higher than the payoff for not coordinating.

Question 48

A game in which both players want to coordinate, but each player prefers coordinating on a different outcome is called

A) an assurance game.
B) a pure coordination game.
C) a battle of the sexes game.
D) a chicken game.

Question 49

In 2009, Samoa changed from everyone driving on the right side of the road to everyone driving on the left side of the road. Although everyone agreed that it was important to drive on the same side of the road, some Samoans were not happy about the change. This created a disagreement about which Nash equilibrium was the best equilibrium, and therefore represented

A) an assurance game.
B) a pure coordination game.
C) a battle of the sexes game.
D) a prisoner's dilemma game.

Question 50

Which of the following is not a characteristic of a battle of the sexes game?

A) Each player has a dominant strategy.
B) Nash equilibria exist at every outcome where the players successfully coordinate.
C) The two general outcomes are where players either coordinate with one another or they do not.
D) The payoff for coordinating is higher than the payoff for not coordinating.

Question 51

All of the following are characteristics of a battle of the sexes game except

A) each player has a tough strategy and a weak strategy.
B) the tough strategy and the weak strategy differ for each player.
C) each player prefers the Nash equilibrium corresponding to his or her tough strategy.
D) each player prefers the same Nash equilibrium.

Question 52

A version of the battle of the sexes game that results in disaster if each player plays his or her tough strategy is called

A) a prisoner's dilemma game.
B) a chicken game.
C) an assurance game.
D) a pure coordination game.

Question 53

All of the following are characteristics of a chicken game except

A) each player has a tough strategy and a weak strategy.
B) coordination occurs when both players either play their tough strategies or both players play their weak strategies.
C) each player prefers the Nash equilibrium corresponding to his or her tough strategy.
D) each player prefers a different Nash equilibrium.

Question 54

Which of the following is not a characteristic of a chicken game?

A) Neither player has a dominant strategy.
B) Nash equilibria exist at every outcome where the players successfully coordinate.
C) The two general outcomes are where players either coordinate with one another or they do not.
D) When both players play their tough strategy, they each receive their best possible payout.

Question 55

Players in all of the following games have no dominant strategy except in

A) a prisoner's dilemma game.
B) a pure coordination game.
C) an assurance game.
D) a battle of the sexes game.

Question 56

There are two equilibria in each of the following two-player games except

A) a prisoner's dilemma game.
B) a pure coordination game.
C) an assurance game.
D) a battle of the sexes game.

Question 57

Of the following types of games, tension between cooperation and individual self-interest tends to be greatest in

A) an assurance game.
B) a pure coordination game.
C) a chicken game.
D) a battle of the sexes game.

Question 58

In a prisoner's dilemma game, the dominant strategy is to defect.

Question 59

In a prisoner's dilemma game, the Nash equilibrium is better for each player than is the cooperative solution.

Question 60

In a pure coordination game, as long as the players coordinate on an outcome, it does not matter what that outcome is.

Question 61

In a pure coordination game, Nash equilibria exist at every outcome where the players successfully coordinate.

Question 62

In an assurance game, each Nash equilibria offer identical payouts to any particular player.

Question 63

In a battle of the sexes game, each player prefers a different Nash equilibrium.

Question 64

In chicken game, Nash equilibria exist at every outcome where the players successfully coordinate.

Question 65

In chicken game, the worst possible outcome occurs when both players play their tough strategy.

Question 66

A game in which each player has a dominant strategy of defecting, and each ends up worse off than if they had both cooperated, is called a ________ game.

Question 67

Figure 3.5: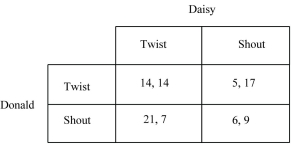
-Refer to Figure 3.5.Answer the following questions about the game represented in the figure:
a. What type of game is represented by the payoff matrix?
b. Does Donald have a dominant strategy, and if so, what is it?
c. Does Donald have a dominant strategy, and if so, what is it?
d. What is the cooperative solution?
e. What is the Nash equilibrium?

Question 68

Figure 3.6: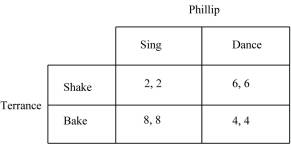
-Refer to Figure 3.6.Answer the following questions about the game represented in the figure:
a. What type of game is represented by the payoff matrix?
b. Does Terrance have a dominant strategy, and if so, what is it?
c. Does Phillip have a dominant strategy, and if so, what is it?
d. At what outcome or outcomes do the players coordinate?
e. Are there any Nash equilibria, and if so, what, are they?

Question 69

Figure 3.7: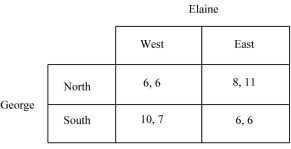
-Refer to Figure 3.7.Answer the following questions about the game represented in the figure:
a. What type of game is represented by the payoff matrix?
b. Does George have a dominant strategy, and if so, what is it?
c. Does Elaine have a dominant strategy, and if so, what is it?
d. Does George have a tough strategy, and if so, what is it?
e. Does Elaine have a tough strategy, and if so, what is it?
f. Are there any Nash equilibria, and if so, what, are they?

Question 70

Of the five game types described in this chapter (prisoner's dilemma, pure coordination, assurance, battle of the sexes, chicken), which have a dominant strategy and which have more than one Nash equilibrium in a two-player game?

Question 71

Describe each of the five game types discussed in this chapter (prisoner's dilemma, pure coordination, assurance, battle of the sexes, chicken) in terms of the amount of tension between cooperation and self-interest of the players.