Deck 11: Further Topics in Algebra
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/48
Play
Full screen (f)
Deck 11: Further Topics in Algebra
1
Write the first four terms for each sequence. State whether the sequence is arithmetic, geometric, or neither.
(a)
(b)
(c) , for
(a)
(b)
(c) , for
(a) ; neither
(b) ; geometric
(c) ; arithmetic
(b) ; geometric
(c) ; arithmetic
2
In each sequence defined, find .
(a) An arithmetic sequence with and .
(b) A geometric sequence with and .
(a) An arithmetic sequence with and .
(b) A geometric sequence with and .
(a) -19
(b)
(b)
3
Find the sum of the first eight terms of the sequence described.
(a) Arithmetic with and .
(b) Geometric with and .
(a) Arithmetic with and .
(b) Geometric with and .
(a) 48
(b) 765
(b) 765
4
Evaluate each sum that exists.
(a)
(b)
(c)
(d)
(a)
(b)
(c)
(d)

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
5
(a) Use the binomial theorem to expand .
(b) Find the fifth term in the expansion of .
(b) Find the fifth term in the expansion of .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
6
Evaluate the following.
(a)
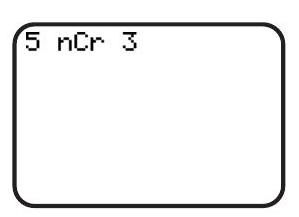
(b)
(c) 11 !
(d)
(a)

(b)
(c) 11 !
(d)

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
7
Use mathematical induction to prove that for all positive integers .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
8
solve each problem involving counting theory.
-A girl opens her tackle box while fishing to find that she has 6 different sizes of hooks, 5 sizes of lead sinkers, and 3 sizes of bobbers. How many different fishing set-ups can she make if she uses one of each?
-A girl opens her tackle box while fishing to find that she has 6 different sizes of hooks, 5 sizes of lead sinkers, and 3 sizes of bobbers. How many different fishing set-ups can she make if she uses one of each?

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
9
solve each problem involving counting theory.
-Suppose that a jeweler wishes to make rings which contain exactly 3 different birthstones in a line. Since there are 12 possible birthstones available, how many such rings are possible?
-Suppose that a jeweler wishes to make rings which contain exactly 3 different birthstones in a line. Since there are 12 possible birthstones available, how many such rings are possible?

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
10
solve each problem involving counting theory.
-A group of 18 third-graders needs to form a 5 -student basketball team. If there are 7 girls and 11 boys in the class and the team is to consist of 2 girls and 3 boys, how many such teams are possible?
-A group of 18 third-graders needs to form a 5 -student basketball team. If there are 7 girls and 11 boys in the class and the team is to consist of 2 girls and 3 boys, how many such teams are possible?

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
11
solve each problem involving counting theory.
-A child's card game consists of a deck of 57 cards, with numbers 1 through 14, in each of four colors (green, red, black, and yellow), and a special card called the Rook card. One card is drawn.
(a) Find the probability of drawing a 2.
(b) Find the probability of drawing a red card lower than 10.
(c) Find the probability of drawing the Rook card or a 14.
(d) What are the odds in favor of drawing a 10 ?
-A child's card game consists of a deck of 57 cards, with numbers 1 through 14, in each of four colors (green, red, black, and yellow), and a special card called the Rook card. One card is drawn.
(a) Find the probability of drawing a 2.
(b) Find the probability of drawing a red card lower than 10.
(c) Find the probability of drawing the Rook card or a 14.
(d) What are the odds in favor of drawing a 10 ?

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
12
solve each problem involving counting theory.
-An experiment consists of rolling a die six times. Find the probability of each event.
(a) Exactly 4 rolls result in a 2.
(b) None of the rolls results in a 3.
-An experiment consists of rolling a die six times. Find the probability of each event.
(a) Exactly 4 rolls result in a 2.
(b) None of the rolls results in a 3.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
13
Write the first four terms for each sequence. State whether the sequence is arithmetic, geometric, or neither.
(a)
(b)
(c) , for
(a)
(b)
(c) , for

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
14
In each sequence defined, find .
(a) An arithmetic sequence with and .
(b) A geometric sequence with and .
(a) An arithmetic sequence with and .
(b) A geometric sequence with and .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
15
Find the sum of the first nine terms of the sequence described.
(a) Arithmetic with and .
(b) Geometric with and .
(a) Arithmetic with and .
(b) Geometric with and .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
16
Evaluate each sum that exists.
(a)
(b)
(c)
(d)
(a)
(b)
(c)
(d)

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
17
(a) Use the binomial theorem to expand .
(b) Find the third term in the expansion of .
(b) Find the third term in the expansion of .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
18
Evaluate the following.
(a)
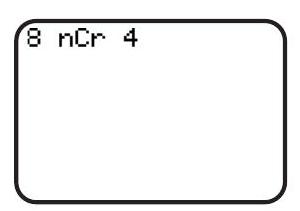
(b)
(c) 10 !
(d)
(a)
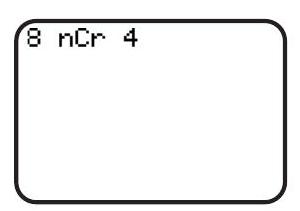
(b)
(c) 10 !
(d)

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
19
Use mathematical induction to prove that for all positive integers .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
20
solve each problem involving counting theory.
-A rental car company offers 3 sizes of cars in 6 different models. How many different cars are there if each car comes with either manual or automatic transmission?
-A rental car company offers 3 sizes of cars in 6 different models. How many different cars are there if each car comes with either manual or automatic transmission?

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
21
solve each problem involving counting theory.
-A child has a box containing 24 different colored markers. The child wants to write his first name in one color, his middle name in a second color, and his last name in a third color. In how many ways can this be done?
-A child has a box containing 24 different colored markers. The child wants to write his first name in one color, his middle name in a second color, and his last name in a third color. In how many ways can this be done?

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
22
solve each problem involving counting theory.
-A cheerleading squad consists of 6 boys and 5 girls. Six cheerleaders are to be selected to form a human pyramid. If the pyramid is made with 3 boys and 3 girls, how many ways can the six cheerleaders be selected?
-A cheerleading squad consists of 6 boys and 5 girls. Six cheerleaders are to be selected to form a human pyramid. If the pyramid is made with 3 boys and 3 girls, how many ways can the six cheerleaders be selected?

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
23
solve each problem involving counting theory.
-Two decks of standard playing cards, including 4 jokers, have a total of 108 cards. One card is drawn.
(a) Find the probability of drawing a queen.
(b) Find the probability of drawing a joker or a two.
(c) Find the probability of drawing a face card (Jack, Queen, King) or a club.
(d) What are the odds in favor of drawing the ace of spades?
-Two decks of standard playing cards, including 4 jokers, have a total of 108 cards. One card is drawn.
(a) Find the probability of drawing a queen.
(b) Find the probability of drawing a joker or a two.
(c) Find the probability of drawing a face card (Jack, Queen, King) or a club.
(d) What are the odds in favor of drawing the ace of spades?

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
24
solve each problem involving counting theory.
-An experiment consists of rolling a die four times. Find the probability of each event.
(a) Exactly 2 rolls result in a 4 .
(b) All four rolls result in a 5 .
-An experiment consists of rolling a die four times. Find the probability of each event.
(a) Exactly 2 rolls result in a 4 .
(b) All four rolls result in a 5 .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
25
Write the first four terms for each sequence. State whether the sequence is arithmetic, geometric, or neither.
(a)
(b)
(c) , for
(a)
(b)
(c) , for

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
26
In each sequence defined, find .
(a) An arithmetic sequence with and .
(b) A geometric sequence with and .
(a) An arithmetic sequence with and .
(b) A geometric sequence with and .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
27
Find the sum of the first seven terms of the sequence described.
(a) Arithmetic with and .
(b) Geometric with and .
(a) Arithmetic with and .
(b) Geometric with and .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
28
Evaluate each sum that exists.
(a)
(b)
(c)
(d)
(a)
(b)
(c)
(d)

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
29
(a) Use the binomial theorem to expand .
(b) Find the sixth term in the expansion of .
(b) Find the sixth term in the expansion of .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
30
Evaluate the following.
(a)
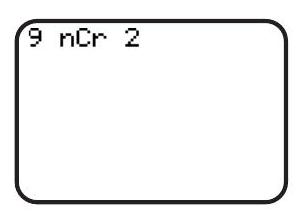
(b)
(c) 8 !
(d)
(a)

(b)
(c) 8 !
(d)

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
31
Use mathematical induction to prove that for all positive integers .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
32
solve each problem involving counting theory.
-Your compact disc collection consists of 9 rock, 6 jazz, and 3 classical discs. How many different ways can you play one rock, one jazz, and one classical recording?
-Your compact disc collection consists of 9 rock, 6 jazz, and 3 classical discs. How many different ways can you play one rock, one jazz, and one classical recording?

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
33
solve each problem involving counting theory.
-Suppose that a jeweler wishes to make rings which contain exactly 4 different birthstones in a line. Since there are 12 possible birthstones available, how many such rings are possible?
-Suppose that a jeweler wishes to make rings which contain exactly 4 different birthstones in a line. Since there are 12 possible birthstones available, how many such rings are possible?

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
34
solve each problem involving counting theory.
-Eighteen college students, 6 men and 12 women, must choose a group of 7 students to ride in a van to a school event. They intend to choose 4 women and 3 men to ride in the van. How many such groups are possible?
-Eighteen college students, 6 men and 12 women, must choose a group of 7 students to ride in a van to a school event. They intend to choose 4 women and 3 men to ride in the van. How many such groups are possible?

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
35
solve each problem involving counting theory.
-Two decks of standard playing cards, including 4 jokers, have a total of 108 cards. One card is drawn.
(a) Find the probability of drawing a black face card (Jack, Queen, King).
(b) Find the probability of drawing a joker or a red 7.
(c) Find the probability of drawing a face card (Jack, Queen, King) or a 3.
(d) What are the odds in favor of drawing the king of hearts?
-Two decks of standard playing cards, including 4 jokers, have a total of 108 cards. One card is drawn.
(a) Find the probability of drawing a black face card (Jack, Queen, King).
(b) Find the probability of drawing a joker or a red 7.
(c) Find the probability of drawing a face card (Jack, Queen, King) or a 3.
(d) What are the odds in favor of drawing the king of hearts?

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
36
solve each problem involving counting theory.
-An experiment consists of rolling a die seven times. Find the probability of each event.
(a) Exactly 2 rolls result in a 6.
(b) None of the rolls results in a 6 .
-An experiment consists of rolling a die seven times. Find the probability of each event.
(a) Exactly 2 rolls result in a 6.
(b) None of the rolls results in a 6 .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
37
Write the first four terms for each sequence. State whether the sequence is arithmetic, geometric, or neither.
(a)
(b)
(c) , for
(a)
(b)
(c) , for

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
38
In each sequence defined, find .
(a) An arithmetic sequence with and .
(b) A geometric sequence with and .
(a) An arithmetic sequence with and .
(b) A geometric sequence with and .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
39
Find the sum of the first eight terms of the sequence described.
(a) Arithmetic with and .
(b) Geometric with and .
(a) Arithmetic with and .
(b) Geometric with and .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
40
Evaluate each sum that exists.
(a)
(b)
(c)
(d)
(a)
(b)
(c)
(d)

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
41
(a) Use the binomial theorem to expand .
(b) Find the fourth term in the expansion of .
(b) Find the fourth term in the expansion of .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
42
Evaluate the following.
(a)

(b)
(c) 9 !
(d)
(a)

(b)
(c) 9 !
(d)

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
43
Use mathematical induction to prove that for all positive integers .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
44
solve each problem involving counting theory.
-A rental car company offers 3 sizes of cars in 5 different colors. How many different cars are there if each car comes with either manual or automatic transmission and either a CD player or satellite radio?
-A rental car company offers 3 sizes of cars in 5 different colors. How many different cars are there if each car comes with either manual or automatic transmission and either a CD player or satellite radio?

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
45
solve each problem involving counting theory.
-A child has a box containing 32 different colored markers. The child wants to write his first name in one color, his middle name in a second color, and his last name in a third color. In how many ways can this be done?
-A child has a box containing 32 different colored markers. The child wants to write his first name in one color, his middle name in a second color, and his last name in a third color. In how many ways can this be done?

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
46
solve each problem involving counting theory.
-A high school soccer team must be made up of 7 seniors and 4 juniors. If 11 seniors and 9 juniors are eligible for the team, how many teams can be formed?
-A high school soccer team must be made up of 7 seniors and 4 juniors. If 11 seniors and 9 juniors are eligible for the team, how many teams can be formed?

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
47
solve each problem involving counting theory.
-A child's card game consists of a deck of 42 cards, with numbers 5 through 14, in each of four colors (green, red, black, and yellow), a red 1, and a special card called the Rook card. One card is drawn.
(a) Find the probability of drawing a 5.
(b) Find the probability of drawing a green card lower than 8 .
(c) Find the probability of drawing the Rook card or a 10
(d) What are the odds in favor of drawing either a green or red 10 ?
-A child's card game consists of a deck of 42 cards, with numbers 5 through 14, in each of four colors (green, red, black, and yellow), a red 1, and a special card called the Rook card. One card is drawn.
(a) Find the probability of drawing a 5.
(b) Find the probability of drawing a green card lower than 8 .
(c) Find the probability of drawing the Rook card or a 10
(d) What are the odds in favor of drawing either a green or red 10 ?

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
48
solve each problem involving counting theory.
-An experiment consists of rolling a die four times. Find the probability of each event.
(a) Exactly 3 rolls result in a 6 .
(b) All four rolls result in a 5.
-An experiment consists of rolling a die four times. Find the probability of each event.
(a) Exactly 3 rolls result in a 6 .
(b) All four rolls result in a 5.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck


