Deck 9: Trigonometric Identities, Models, and Complex Numbers
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/106
Play
Full screen (f)
Deck 9: Trigonometric Identities, Models, and Complex Numbers
1
At which of the following values of do the graphs of and intersect?
A) All multiples of
B) All even multiples of
C) All odd multiples of
D) None of the above
A) All multiples of
B) All even multiples of
C) All odd multiples of
D) None of the above
All odd multiples of
2
Estimate the solution to the equation for . Round to 2 decimal places.
0.78
3
Find a solution in the interval for . Round to 3 decimal places.
4
Use a graph of to estimate the solution to the equation for . Round to 2 decimal places.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
5
Which of the following are -intercepts of the function ?
A) 0
B)
C)
D)
E)
F)
G) None of the above
A) 0
B)
C)
D)
E)
F)
G) None of the above

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
6
Find all solutions to on the interval . Give your answers correct to 3 decimal places.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
7
Find all solutions to the equation for . Give your answers to 3 decimal places.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
8
The baseball field's usage (in people per week) is seasonal with the peak in mid-July and the low in mid-January. The usage is 2,000 in July and 500 in January. Find a trig function representing the usage at time months after mid-January.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
9
Which of the following are defined?
A)
B)
C)
D)
E)
F)
A)
B)
C)
D)
E)
F)

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
10
Find all values of such that and . Give answers correct to 3 decimal places.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
11
Find all solutions (if possible) for in radians: . Give answers correct to 3 decimal places.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
12
Find all solutions (if possible) with in radians: . Give answers correct to 3 decimal places.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
13
Find all solutions to for . Give answers correct to 3 decimal places.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
14
Find all solutions to for . Give answers correct to 3 decimal places.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
15
How many solutions to are there for ?

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
16
How many solutions to are there for ?

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
17
How many solutions to are there for ?

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
18
Solve for .

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
19
Solve for .

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
20
Solve for .

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
21
Graph and use that graph to approximate the solution of on . Give in degrees to 2 decimal places.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
22
Graph and use that graph to approximate the solution of on . Give in degrees to 2 decimal places.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
23
Graph and use that graph to approximate the solution of on . Give in degrees to 2 decimal places.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
24
Does ?

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
25
What is the smallest positive solution to ? Round to 2 decimal places.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
26
What is the smallest positive solution to ? Round to 2 decimal places.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
27
How many solutions does have for ?

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
28
What is the smallest positive solution to ? Round to 2 decimal places.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
29
How many solutions does have for ?

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
30
Does ?

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
31
What is the smallest positive solution to ? Round your answer to 2 decimal places.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
32
How many solutions does have for ?

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
33
Which of the following statements are identities?
A)
B)
C)
D)
E)
F)
A)
B)
C)
D)
E)
F)

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
34
What is for ?

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
35
Write in terms of the tangent function.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
36
Write in terms of the cotangent function.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
37
If and , find , and exactly.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
38
If and , find , and exactly.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
39
Write in terms of the tangent function.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
40
How many solutions does have for ?
A) 4
B) 0
C) 1
D) none of the above.
A) 4
B) 0
C) 1
D) none of the above.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
41
If , in what quadrant is ?
A) I
B) II
C) III
D) IV
A) I
B) II
C) III
D) IV

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
42
If , in what quadrant is ?
A) I
B) II
C) III
D) IV
A) I
B) II
C) III
D) IV

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
43
If , in what quadrant is ?
A) I
B) II
C) III
D) IV
A) I
B) II
C) III
D) IV

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
44
If , in what quadrant is ?
A) I
B) II
C) III
D) IV
A) I
B) II
C) III
D) IV

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
45
Either show the following equation is true, or find a value of for which the equation is false:

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
46
Either show the following equation is true, or find a value of for which the equation is false:

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
47
Either show the following equation is true, or find a value of for which the equation is false:

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
48
Does ?

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
49
If , then can also be written in the form
---------- -------------- ---------- .
---------- -------------- ---------- .

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
50
Does ?

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
51
Does

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
52
Using the sum or difference formulas, .
Round both answers to 4 decimal places.
Round both answers to 4 decimal places.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
53
Using the sum or difference formulas,
. Round all answers to 4 decimal places if necessary.
. Round all answers to 4 decimal places if necessary.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
54
Does ?

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
55
Find the exact value of .

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
56
Find the exact value of .

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
57
Find the smallest value of such that and .

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
58
Find the smallest value of such that and .

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
59
Find the smallest value of such that and .

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
60
Find the smallest value of such that and .

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
61
Calculate exactly.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
62
Does ?

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
63
Show that .

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
64
Show that .

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
65
Does ?

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
66
If , can also be written in the form ?

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
67
Using the sum or difference formulas, --------- -----------). Round both answers to 4 decimal places.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
68
Using the sum or difference formulas,
------------- ------------). Round all answers to 4 decimal places if necessary.
------------- ------------). Round all answers to 4 decimal places if necessary.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
69
Write in the form . Round all numbers to 3 decimal places if necessary.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
70
Write in the form . Round all numbers to 3 decimal places if necessary.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
71
Write in the form . Round all numbers to 3 decimal places if necessary.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
72
Write in the form . Round all numbers to 3 decimal places if necessary.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
73
The following table gives , the percentage of the electorate favoring candidate during the 12 months preceding a presidential election. Time, , is measured in months, and is a year before election day.
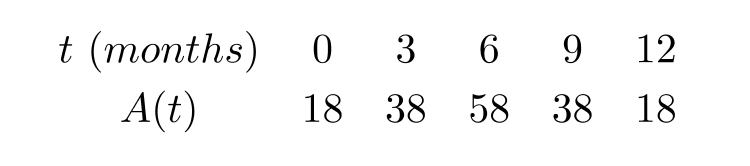
If were approximately trigonometric, its formula could be written . Round the second answer to 3 decimal places.
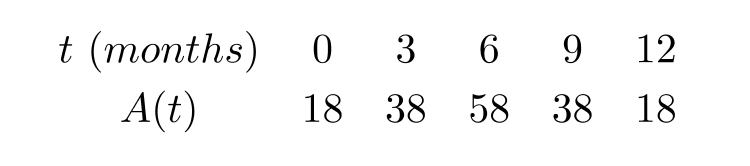
If were approximately trigonometric, its formula could be written . Round the second answer to 3 decimal places.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
74
The following table gives , the percentage of the electorate favoring candidate during the 12 months preceding a presidential election. Time, , is measured in months, and is a year before election day.
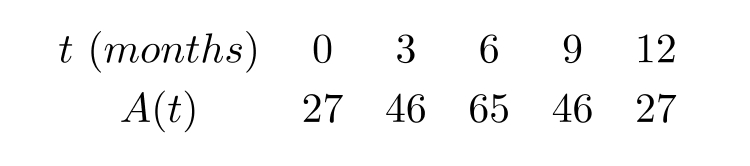
Assume that is approximately trigonometric. A second candidate, candidate , has a percentage of support given by . What is the largest value of , at which the two candidates are tied for electoral support? Round to 2 decimal places.

Assume that is approximately trigonometric. A second candidate, candidate , has a percentage of support given by . What is the largest value of , at which the two candidates are tied for electoral support? Round to 2 decimal places.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
75
The following table gives , the percentage of the electorate favoring candidate during the 12 months preceding a presidential election. Time, , is measured in months, and is a year before election day.
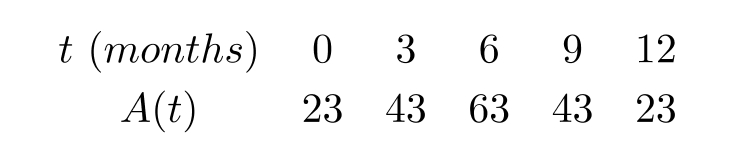
Assume that is approximately trigonometric. A second candidate, candidate , has a percentage of candidate support given by . Let for . What is the meaning of the minimum of ?
A) The minimum percentage lead candidate has over candidate .
B) The minimum percentage lead candidate has over candidate .
C) The minimum combined percentage of the electorate favoring either candidate or candidate .
D) The minimum combined percentage of the electorate favoring neither candidate nor candidate .
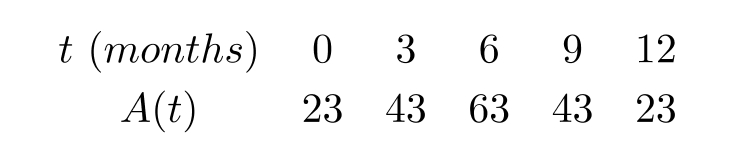
Assume that is approximately trigonometric. A second candidate, candidate , has a percentage of candidate support given by . Let for . What is the meaning of the minimum of ?
A) The minimum percentage lead candidate has over candidate .
B) The minimum percentage lead candidate has over candidate .
C) The minimum combined percentage of the electorate favoring either candidate or candidate .
D) The minimum combined percentage of the electorate favoring neither candidate nor candidate .

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
76
Two weights (weight 1 and weight 2 ) are suspended from the ceiling by springs. At time ( in seconds), the weights are set in motion and begin bobbing up and down. Eventually, however, the oscillation of both weights dies down. The following equations describe the distance of each weight from the ceiling as a function of time:
Which weight is closer to the ceiling at time ?
A) Weight 1
B) Weight 2
Which weight is closer to the ceiling at time ?
A) Weight 1
B) Weight 2

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
77
Two weights (weight 1 and weight 2 ) are suspended from the ceiling by springs. At time ( in seconds), the weights are set in motion and begin bobbing up and down. Eventually, however, the oscillation of both weights dies down. The following equations describe the distance of each weight from the ceiling as a function of time:
Which weight has oscillations which die down the slowest?
A) Weight 1
B) Weight 2
Which weight has oscillations which die down the slowest?
A) Weight 1
B) Weight 2

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
78
Two weights (weight 1 and weight 2 ) are suspended from the ceiling by springs. At time ( in seconds), the weights are set in motion and begin bobbing up and down. Eventually, however, the oscillation of both weights dies down. The following equations describe the distance of each weight from the ceiling as a function of time:
At what time are the two weights furthest apart?
A) At .
B) Between and .
C) At .
D) Between and .
E) At .
At what time are the two weights furthest apart?
A) At .
B) Between and .
C) At .
D) Between and .
E) At .

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
79
Find a formula for a deer population which oscillates over a 6 year period between a low of 1,000 in year and a high of 2,700 in year .

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
80
The deer population in a state park is modelled by where is the number of months since January 1, 2005. Evaluate and interpret the result. Round to the nearest whole number.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck


