Deck 7: Continuous Probability Distributions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/131
Play
Full screen (f)
Deck 7: Continuous Probability Distributions
1
Non-stop Airlines determined that the mean number of passengers per flight is 152 with a standard deviation of ten passengers. Practically all flights have between 142 and 162 passengers.
False
2
Some normal probability distributions are positively skewed.
FAL SE
3
The upper and lower limits of a uniform probability distribution are
A) positive and negative infinity.
B) plus and minus three standard deviations.
C) 0 and 1.
D) the maximum and minimum values of the random variable.
A) positive and negative infinity.
B) plus and minus three standard deviations.
C) 0 and 1.
D) the maximum and minimum values of the random variable.
D
4
The mean of any uniform probability distribution is
A) (b - a)/2
B) (a + b)/2
C)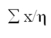
D) n
A) (b - a)/2
B) (a + b)/2
C)
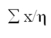
D) n

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
5
The standard normal distribution is a special normal distribution with a mean of 0 and a standard deviation of 1.

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
6
The area under the normal curve within plus and minus one standard deviation of the mean is about 68.26%.

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
7
The shape of any uniform probability distribution is
A) Negatively skewed
B) Positively skewed
C) Rectangular
D) Bell shaped
A) Negatively skewed
B) Positively skewed
C) Rectangular
D) Bell shaped

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
8
The exponential distribution is a probability distribution for a random variable measured as a rate of an event occurring.

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
9
For any uniform probability distribution, the mean and standard deviation can be computed by knowing the maximum and minimum values of the random variable.

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
10
The number of different standard normal distributions is unlimited.

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
11
For the exponential distribution, the mean and standard deviation are equal.

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
12
For a uniform probability distribution, the probability of any event is equal to 1/(b-a).

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
13
The time to fly between New York City and Chicago is uniformly distributed with a minimum of 120 minutes and a maximum of 150 minutes. What is the mean?
A) 120 minutes
B) 150 minutes
C) 135 minutes
D) 270 minutes
A) 120 minutes
B) 150 minutes
C) 135 minutes
D) 270 minutes

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
14
The z-scores for X values greater than the mean are negative.

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
15
The normal distribution can be used to approximate a binomial distribution when n is less than 5.

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
16
In a uniform probability distribution, P(x) is constant between the distribution's minimum and maximum values.

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
17
When referring to the normal probability distribution, there is not just one; there is a "family" of distributions.

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
18
The standard deviation of any uniform probability distribution is
A) (b - a)/2
B) n(1 - )
C)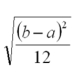
D)
A) (b - a)/2
B) n(1 - )
C)
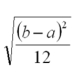
D)

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
19
A "continuity correction factor" is used to compute probabilities when using the normal distribution to approximate the binomial distribution.

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
20
Some normal probability distributions have equal arithmetic means, but their standard deviations may be different.

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
21
What is the area under the normal curve between z = 0.0 and z = 1.79?
A) 0.4633
B) 0.0367
C) 0.9599
D) 0.0401
A) 0.4633
B) 0.0367
C) 0.9599
D) 0.0401

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
22
A new extended-life light bulb has an average service life of 750 hours, with a standard deviation of 50 hours. If the service life of these light bulbs approximates a normal distribution, about what percent of the distribution will be between 600 hours and 900 hours?
A) 95%
B) 68%
C) 34%
D) 99.7%
A) 95%
B) 68%
C) 34%
D) 99.7%

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
23
For the normal distribution, the mean plus and minus 1.96 standard deviations will include about what percent of the observations?
A) 50%
B) 99.7%
C) 95%
D) 68%
A) 50%
B) 99.7%
C) 95%
D) 68%

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
24
Which of the following is true regarding the normal distribution?
A) Mean, median and mode are all equal
B) It has two modes
C) It is asymmetrical
D) The points of the curve meet the X-axis at z = -3 and z = 3
A) Mean, median and mode are all equal
B) It has two modes
C) It is asymmetrical
D) The points of the curve meet the X-axis at z = -3 and z = 3

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
25
An accelerated life test on a large number of type-D alkaline batteries revealed that the mean life for a particular use before they failed is 19.0 hours. The distribution of the lives approximated a normal distribution. The standard deviation of the distribution was 1.2 hours. About 95.44 percent of the batteries failed between what two values?
A) 8.9 and 18.9
B) 12.2 and 14.2
C) 14.1 and 22.1
D) 16.6 and 21.4
A) 8.9 and 18.9
B) 12.2 and 14.2
C) 14.1 and 22.1
D) 16.6 and 21.4

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
26
What is an important similarity between the uniform and normal probability distributions?
A) The mean, median and mode are all equal.
B) The mean and median are equal.
C) They are negatively skewed.
D) About 68% of all observations are within one standard deviation of the mean.
A) The mean, median and mode are all equal.
B) The mean and median are equal.
C) They are negatively skewed.
D) About 68% of all observations are within one standard deviation of the mean.

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
27
A study of a company's practice regarding the payment of invoices revealed that an invoice was paid an average of 20 days after it was received. The standard deviation equaled five days. Assuming that the distribution is normal, what percent of the invoices were paid within 15 days of receipt?
A) 15.87%
B) 37.91%
C) 34.13%
D) 86.74%
A) 15.87%
B) 37.91%
C) 34.13%
D) 86.74%

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
28
The time to fly between New York City and Chicago is uniformly distributed with a minimum of 120 minutes and a maximum of 150 minutes. What is the probability that a flight is more than 140 minutes?
A) 1.00
B) 0.5
C) 0.333 minutes
D) 10 minutes
A) 1.00
B) 0.5
C) 0.333 minutes
D) 10 minutes

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
29
The time to fly between New York City and Chicago is uniformly distributed with a minimum of 120 minutes and a maximum of 150 minutes. What is the probability that a flight is between 125 and 140 minutes?
A) 1.00
B) 0.5
C) 0.333 minutes
D) 10 minutes
A) 1.00
B) 0.5
C) 0.333 minutes
D) 10 minutes

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
30
What is the area under the normal curve between z = -1.0 and z = -2.0?
A) 0.0228
B) 0.3413
C) 0.1359
D) 0.4772
A) 0.0228
B) 0.3413
C) 0.1359
D) 0.4772

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
31
The mean of a normally distributed group of weekly incomes of a large group of executives is $1,000 and the standard deviation is $100. What is the z-score for an income of $1,100?
A) 1.00
B) 2.00
C) 1.683
D) -0.90
A) 1.00
B) 2.00
C) 1.683
D) -0.90

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
32
The mean amount spent by a family of four on food per month is $500 with a standard deviation of $75. Assuming that the food costs are normally distributed, what is the probability that a family spends less than $410 per month?
A) 0.2158
B) 0.8750
C) 0.0362
D) 0.1151
A) 0.2158
B) 0.8750
C) 0.0362
D) 0.1151

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
33
The time to fly between New York City and Chicago is uniformly distributed with a minimum of 120 minutes and a maximum of 150 minutes. What is the standard deviation?
A) 8.66 minutes
B) 75 minutes
C) 135 minutes
D) 270 minutes
A) 8.66 minutes
B) 75 minutes
C) 135 minutes
D) 270 minutes

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
34
For a standard normal distribution, what is the probability that z is greater than 1.75?
A) 0.0401
B) 0.0459
C) 0.4599
D) 0.9599
A) 0.0401
B) 0.0459
C) 0.4599
D) 0.9599

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
35
What is the area under the normal curve between z = 0.0 and z = 2.0?
A) 1.0000
B) 0.7408
C) 0.1359
D) 0.4772
A) 1.0000
B) 0.7408
C) 0.1359
D) 0.4772

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
36
The time to fly between New York City and Chicago is uniformly distributed with a minimum of 120 minutes and a maximum of 150 minutes. What is the probability that a flight is less than 135 minutes?
A) 1.00
B) 0.5
C) 15 minutes
D) 270 minutes
A) 1.00
B) 0.5
C) 15 minutes
D) 270 minutes

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
37
The mean of a normal distribution is 400 pounds. The standard deviation is 10 pounds. What is the area between 415 pounds and the mean of 400 pounds?
A) 0.5000
B) 0.1932
C) 0.4332
D) 0.3413
A) 0.5000
B) 0.1932
C) 0.4332
D) 0.3413

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
38
The mean score of a college entrance test is 500; the standard deviation is 75. The scores are normally distributed. What percent of the students scored below 320?
A) About 50.82%
B) About 34.13%
C) About 7.86%
D) About 0.82%
A) About 50.82%
B) About 34.13%
C) About 7.86%
D) About 0.82%

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
39
Which of the following is a characteristic of the normal probability distribution?
A) Positively-skewed
B) Bell-shaped
C) Asymmetrical
D) Rectangular
A) Positively-skewed
B) Bell-shaped
C) Asymmetrical
D) Rectangular

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
40
What is the percentage of the total area under the normal curve within plus and minus two standard deviations of the mean?
A) 68.26%
B) 99.74%
C) 34.13%
D) 95.44%
A) 68.26%
B) 99.74%
C) 34.13%
D) 95.44%

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
41
An analysis of the grades on the first test in History 101 revealed that they approximate a normal curve with a mean of 75 and a standard deviation of 8. The instructor wants to award the grade of A to the upper 10 percent of the test grades. What is the dividing point between an A and a B grade?
A) 80
B) 85
C) 90
D) 95
A) 80
B) 85
C) 90
D) 95

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
42
The standard normal probability distribution is unique because it has:
A) A mean of 1 and any standard deviation
B) Any mean and a standard deviation of 1
C) A mean of 0 and any standard deviation
D) A mean of 0 and a standard deviation of 1
A) A mean of 1 and any standard deviation
B) Any mean and a standard deviation of 1
C) A mean of 0 and any standard deviation
D) A mean of 0 and a standard deviation of 1

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
43
What is a normal distribution with a mean of 0 and a standard deviation of 1 called?
A) Frequency distribution
B) Z-score
C) Standard normal distribution
D) Binomial probability distribution
A) Frequency distribution
B) Z-score
C) Standard normal distribution
D) Binomial probability distribution

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
44
A national manufacturer of unattached garages discovered that the distribution of the lengths of time it takes two construction workers to erect the Red Barn model is normally distributed with a mean of 32 hours and a standard deviation of 2 hours. What percent of the garages take between 32 and 34 hours to erect?
A) 16.29%
B) 76.71%
C) 3.14%
D) 34.13%
A) 16.29%
B) 76.71%
C) 3.14%
D) 34.13%

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
45
A cola-dispensing machine is set to dispense a mean of 2.02 liters into a container labeled 2 liters. Actual quantities dispensed vary and the amounts are normally distributed with a standard deviation of 0.015 liters. What is the probability a container will have less than 2 liters?
A) 0.0918
B) 0.3413
C) 0.1926
D) 0.8741
A) 0.0918
B) 0.3413
C) 0.1926
D) 0.8741

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
46
Tables of normal distribution probabilities are found in many statistics books. These probabilities are calculated from a normal distribution with
A) a mean of 1 and a standard deviation of 1
B) a mean of 100 and a standard deviation of 15
C) a mean of 0 and a standard deviation of 15
D) a mean of 0 and a standard deviation of 1
A) a mean of 1 and a standard deviation of 1
B) a mean of 100 and a standard deviation of 15
C) a mean of 0 and a standard deviation of 15
D) a mean of 0 and a standard deviation of 1

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
47
Two normal distributions are compared. One has a mean of 10 and a standard deviation of 10. The second normal distribution has a mean of 10 and a standard deviation of 2. Which of the following is true?
A) The locations of the distributions are different
B) The distributions are from two different families of distributions
C) The dispersions of the distributions are different
D) The dispersions of the distributions are the same
A) The locations of the distributions are different
B) The distributions are from two different families of distributions
C) The dispersions of the distributions are different
D) The dispersions of the distributions are the same

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
48
The weekly mean income of a group of executives is $1000 and the standard deviation of this group is $100. The distribution is normal. What percent of the executives have an income of $925 or less?
A) About 15%
B) About 85%
C) About 50%
D) About 23%
A) About 15%
B) About 85%
C) About 50%
D) About 23%

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
49
The employees of Cartwright Manufacturing are awarded efficiency ratings. The distribution of the ratings approximates a normal distribution. The mean is 400, the standard deviation 50. What is the area under the normal curve between 400 and 482?
A) 0.5000
B) 0.4495
C) 0.3413
D) 0.4750
A) 0.5000
B) 0.4495
C) 0.3413
D) 0.4750

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
50
Which of the following is true in a normal distribution?
A) The mean equals zero.
B) Mode and the 3rd quartile are equal.
C) Mean divides the distribution into two equal parts.
D) The area under the curve is 0.68.
A) The mean equals zero.
B) Mode and the 3rd quartile are equal.
C) Mean divides the distribution into two equal parts.
D) The area under the curve is 0.68.

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
51
The seasonal output of a new experimental strain of pepper plants was carefully weighed. The mean weight per plant is 15.0 pounds, and the standard deviation of the normally distributed weights is 1.75 pounds. Of the 200 plants in the experiment, how many produced peppers weighing between 13 and 16 pounds?
A) 100
B) 118
C) 197
D) 53
A) 100
B) 118
C) 197
D) 53

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
52
The total area of a normal probability distribution is
A) between -3.0 and 3.0.
B) 1.00.
C) dependent on a value of 'z'.
D) approximated by the binomial distribution.
A) between -3.0 and 3.0.
B) 1.00.
C) dependent on a value of 'z'.
D) approximated by the binomial distribution.

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
53
The annual commissions per salesperson employed by a manufacturer of light machinery averaged $40,000 with a standard deviation of $5,000. What percent of the sales persons earn between $32,000 and $42,000?
A) 60.06%
B) 39.94%
C) 34.13%
D) 81.66%
A) 60.06%
B) 39.94%
C) 34.13%
D) 81.66%

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
54
In an illustration of a normal probability distribution, a shaded area represents
A) a permutation
B) a combination
C) a probability
D) a standard deviation
A) a permutation
B) a combination
C) a probability
D) a standard deviation

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
55
A random variable from an experiment where outcomes are normally distributed
A) can have any value between - and + .
B) can have only a few discrete values.
C) can have a mean of 0 and a standard deviation of 1.
D) can have no values.
A) can have any value between - and + .
B) can have only a few discrete values.
C) can have a mean of 0 and a standard deviation of 1.
D) can have no values.

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
56
The weight of cans of fruit is normally distributed with a mean of 1,000 grams and a standard deviation of 50 grams. What percent of the cans weigh 860 grams or less?
A) 0.0100
B) 0.8400
C) 0.0026
D) 0.0001
A) 0.0100
B) 0.8400
C) 0.0026
D) 0.0001

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
57
The distribution of the annual incomes of a group of middle management employees approximated a normal distribution with a mean of $37,200 and a standard deviation of $800. About 68 percent of the incomes lie between what two incomes?
A) $30,000 and $40,000
B) $36,400 and $38,000
C) $34,800 and $39,600
D) $35,600 and $38,800
A) $30,000 and $40,000
B) $36,400 and $38,000
C) $34,800 and $39,600
D) $35,600 and $38,800

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
58
The mean of a normal probability distribution is 500 and the standard deviation is 10. About 95 percent of the observations lie between what two values?
A) 475 and 525
B) 480 and 520
C) 400 and 600
D) 350 and 650
A) 475 and 525
B) 480 and 520
C) 400 and 600
D) 350 and 650

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
59
Ball-Bearings, Inc. produces ball bearings automatically on a Kronar BBX machine. For one of the ball bearings, the mean diameter is set at 20.00 mm (millimeters). The standard deviation of the production over a long period of time was computed to be 0.150 mm. What percent of the ball bearings will have diameters 20.27 mm or more?
A) 41.00%
B) 12.62%
C) 3.59%
D) 85.00%
A) 41.00%
B) 12.62%
C) 3.59%
D) 85.00%

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
60
A large manufacturing firm tests job applicants who recently graduated from college. The test scores are normally distributed with a mean of 500 and a standard deviation of 50. Management is considering placing a new hire in an upper level management position if the person scores in the upper 6 percent of the distribution. What is the lowest score a college graduate must earn to qualify for a responsible position?
A) 50
B) 625
C) 460
D) 578
A) 50
B) 625
C) 460
D) 578

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
61
How is the standard deviation of a uniform distribution computed? _________

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
62
The mean amount of gasoline and services charged by Key Refining Company credit customers is $70 per month. The distribution of amounts spent is approximately normal with a standard deviation of $10. What is the probability of selecting a credit card customer at random and finding the customer charged between $70 and $83?
A) 0.1962
B) 0.4032
C) 0.3413
D) 0.4750
A) 0.1962
B) 0.4032
C) 0.3413
D) 0.4750

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
63
A binomial distribution has 50 trials (n = 50) with a probability of success of 0.50 ( =0.50). To use the normal distribution to approximate the binomial, what are the mean and standard deviation?
A) µ = 25 and = 3.5355
B) µ =25 and = 12.5
C) µ =25 and = 4.33
D) µ =50 and = 100
A) µ = 25 and = 3.5355
B) µ =25 and = 12.5
C) µ =25 and = 4.33
D) µ =50 and = 100

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
64
The average score of 100 students taking a statistics final was 70 with a standard deviation of 7. Assuming a normal distribution, what is the probability that a student scored 90 or higher?
A) 0.4979
B) 0.0021
C) 0.9979
D) 2.86
A) 0.4979
B) 0.0021
C) 0.9979
D) 2.86

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
65
If an average of 12 customers is served per hour, what is the probability that the next customer will arrive in 6 minutes or less?
A) 0.61
B) 0.30
C) 0.70
D) 0.39
A) 0.61
B) 0.30
C) 0.70
D) 0.39

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
66
Suppose a tire manufacturer wants to set a mileage guarantee on its new XB 70 tire. Tests revealed that the tire's mileage is normally distributed with a mean of 47,900 miles and a standard deviation of 2,050 miles. The manufacturer wants to set the guaranteed mileage so that no more than 5 percent of the tires will have to be replaced. What guaranteed mileage should the manufacturer announce?
A) 44,528
B) 32,960
C) 49,621
D) 40,922
A) 44,528
B) 32,960
C) 49,621
D) 40,922

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
67
The shape of the uniform distribution is ____________.

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
68
Management is considering adopting a bonus system to increase production. One suggestion is to pay a bonus on the highest 5 percent of production based on past experience. Past records indicate that, on the average, 4,000 units of a small assembly are produced during a week. The distribution of the weekly production is approximately normally distributed with a standard deviation of 60 units. If the bonus is paid on the upper 5 percent of production, the bonus will be paid on how many units or more?
A) 6255
B) 5120
C) 3196
D) 4099
A) 6255
B) 5120
C) 3196
D) 4099

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
69
The average score of 100 students taking a statistics final was 70 with a standard deviation of 7. Assuming a normal distribution, what is the probability that a student scored greater than 65?
A) 0.2611
B) 0.2389
C) 0.7611
D) -0.714
A) 0.2611
B) 0.2389
C) 0.7611
D) -0.714

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
70
A binomial distribution has 100 trials (n = 100) with a probability of success of 0.25 ( =0.25). We would like to find the probability of 34 or more successes using the normal distribution to approximate the binomial. Applying the continuity correction factor, what z-score should be used?
A) 2.079
B) 2.194
C) 1.963
D) 0.25
A) 2.079
B) 2.194
C) 1.963
D) 0.25

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
71
A binomial distribution has 100 trials (n = 100) with a probability of success of 0.25 ( =0.25). To apply the normal distribution to approximate the binomial, what are the mean and standard deviation?
A) µ = 100 and = 0.25
B) µ =25 and = 100
C) µ =25 and = 4.33
D) µ =100 and = 2500
A) µ = 100 and = 0.25
B) µ =25 and = 100
C) µ =25 and = 4.33
D) µ =100 and = 2500

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
72
The average score of 100 students taking a statistics final was 70 with a standard deviation of 7. Assuming a normal distribution, what test score separates the top 5% of the students from the lower 95% of students?
A) 81.48
B) 90.00
C) 83.72
D) 78.96
A) 81.48
B) 90.00
C) 83.72
D) 78.96

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
73
If an average of 60 customers are served per hour, then one customer arrives every
A) 1 minute
B) 60 minutes
C) 12 minutes
D) 10 minutes
A) 1 minute
B) 60 minutes
C) 12 minutes
D) 10 minutes

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
74
How is the expected value of a uniform distribution computed? __________

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
75
The average score of 100 students taking a statistics final was 70 with a standard deviation of 7. Assuming a normal distribution, what test score separates the top 25% of the students from the lower 75% of students?
A) 70.00
B) 74.69
C) 65.31
D) 75.25
A) 70.00
B) 74.69
C) 65.31
D) 75.25

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
76
If an average of 12 customers are served per hour, then one customer arrives every
A) 12 minutes
B) 60 minutes
C) 5 minutes
D) 10 minutes
A) 12 minutes
B) 60 minutes
C) 5 minutes
D) 10 minutes

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
77
A binomial distribution has 50 trials (n = 50) with a probability of success of 0.50 ( =0.50). We would like to find the probability of 34 or more successes using the normal distribution to approximate the binomial. Applying the continuity correction factor, what z-score should be used?
A) 2.404
B) 2.546
C) 2.687
D) 0.50
A) 2.404
B) 2.546
C) 2.687
D) 0.50

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
78
If an average of 12 customers is served per hour, what is the probability that the next customer will arrive in 3 minutes or less?
A) 0.55
B) 0.30
C) 0.45
D) 0.39
A) 0.55
B) 0.30
C) 0.45
D) 0.39

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
79
A binomial distribution has 100 trials (n = 100) with a probability of success of 0.25 ( =0.25). We would like to find the probability of 75 or more successes using the normal distribution to approximate the binomial. Applying the continuity correction factor, what value would be used to calculate a z-score?
A) 74.5
B) 75
C) 75.5
D) 25
A) 74.5
B) 75
C) 75.5
D) 25

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck
80
If an average of 12 customers is served per hour, what is the probability that the next customer will arrive in 3 minutes or more?
A) 0.55
B) 0.30
C) 0.45
D) 0.39
A) 0.55
B) 0.30
C) 0.45
D) 0.39

Unlock Deck
Unlock for access to all 131 flashcards in this deck.
Unlock Deck
k this deck


