Deck 3: Applications of the Derivative
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/122
Play
Full screen (f)
Deck 3: Applications of the Derivative
1
Find the x-values of all relative maxima of the given function.
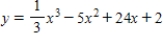
A) 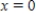
B) 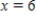
C) 
D) 
E) no relative maxima
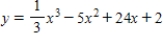
A)
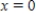
B)
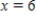
C)

D)

E) no relative maxima


2
For the function 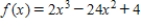 (a)Find the critical numbers of f (if any);
(a)Find the critical numbers of f (if any);
(b)Find the open intervals where the function is increasing or decreasing; and
(c)Apply the First Derivative Test to identify all relative extrema.
A) (a)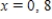
(b) increasing: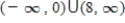 ; decreasing:
; decreasing: 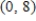
(c) relative max: ; relative min:
; relative min: 
B) (a)
(b) decreasing: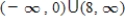 ; increasing:
; increasing: 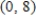
(c) relative min: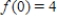 ; relative max:
; relative max: 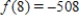
C) (a)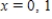
(b) increasing: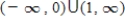 ; decreasing:
; decreasing: 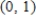
(c) relative max: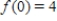 ; relative min:
; relative min: 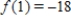
D) (a)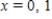
(b) decreasing: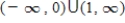 ; increasing:
; increasing: 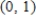
(c) relative min: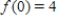 ; relative max:
; relative max: 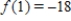
E) (a)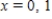
(b)increasing: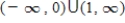 ; decreasing:
; decreasing: 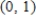
(c)relative max: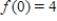 ; relative min: no relative min.
; relative min: no relative min.
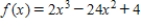 (a)Find the critical numbers of f (if any);
(a)Find the critical numbers of f (if any);(b)Find the open intervals where the function is increasing or decreasing; and
(c)Apply the First Derivative Test to identify all relative extrema.
A) (a)
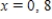
(b) increasing:
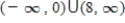 ; decreasing:
; decreasing: 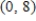
(c) relative max:
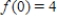 ; relative min:
; relative min: 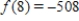
B) (a)

(b) decreasing:
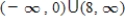 ; increasing:
; increasing: 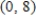
(c) relative min:
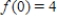 ; relative max:
; relative max: 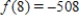
C) (a)
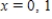
(b) increasing:
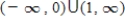 ; decreasing:
; decreasing: 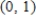
(c) relative max:
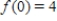 ; relative min:
; relative min: 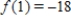
D) (a)
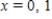
(b) decreasing:
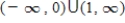 ; increasing:
; increasing: 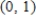
(c) relative min:
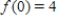 ; relative max:
; relative max: 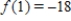
E) (a)
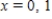
(b)increasing:
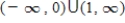 ; decreasing:
; decreasing: 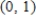
(c)relative max:
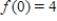 ; relative min: no relative min.
; relative min: no relative min.
(a) 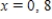
(b) increasing: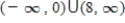 ; decreasing:
; decreasing: 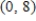
(c) relative max: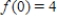 ; relative min:
; relative min: 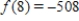
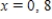
(b) increasing:
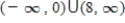 ; decreasing:
; decreasing: 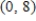
(c) relative max:
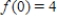 ; relative min:
; relative min: 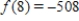
3
Both a function and its derivative are given.Use them to find all critical numbers.
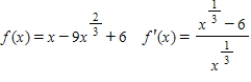
A) 
B) 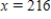
C) 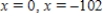
D) 
E) 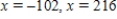
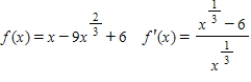
A)

B)
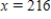
C)
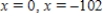
D)

E)
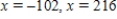


4
Find the open intervals on which the function 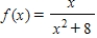 is increasing or decreasing.
is increasing or decreasing.
A) The function is increasing on the interval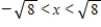 , and decreasing on the intervals
, and decreasing on the intervals 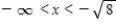 and
and 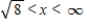
B) The function is increasing on the interval , and decreasing on the intervals
, and decreasing on the intervals  and
and 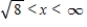
C) The function is increasing on the interval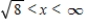 , and decreasing on the intervals
, and decreasing on the intervals 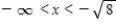 and
and 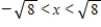
D) The function is decreasing on the interval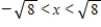 , and increasing on the intervals
, and increasing on the intervals 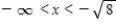 and
and 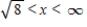
E) The function is decreasing on the interval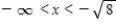 ,and increasing on the intervals
,and increasing on the intervals 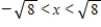 and
and 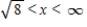
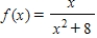 is increasing or decreasing.
is increasing or decreasing. A) The function is increasing on the interval
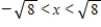 , and decreasing on the intervals
, and decreasing on the intervals 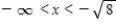 and
and 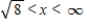
B) The function is increasing on the interval
 , and decreasing on the intervals
, and decreasing on the intervals  and
and 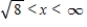
C) The function is increasing on the interval
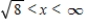 , and decreasing on the intervals
, and decreasing on the intervals 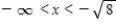 and
and 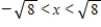
D) The function is decreasing on the interval
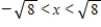 , and increasing on the intervals
, and increasing on the intervals 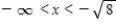 and
and 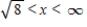
E) The function is decreasing on the interval
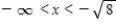 ,and increasing on the intervals
,and increasing on the intervals 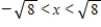 and
and 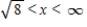

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
5
Identify the open intervals where the function 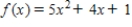 is increasing or decreasing.
is increasing or decreasing.
A) decreasing: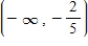 ; increasing:
; increasing: 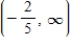
B) increasing: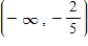 ; decreasing:
; decreasing: 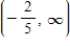
C) increasing on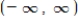
D) decreasing on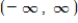
E) none of the above
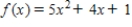 is increasing or decreasing.
is increasing or decreasing. A) decreasing:
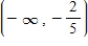 ; increasing:
; increasing: 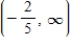
B) increasing:
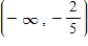 ; decreasing:
; decreasing: 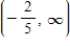
C) increasing on
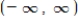
D) decreasing on
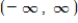
E) none of the above

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
6
Suppose the number y of medical degrees conferred in the United States can be modeled by 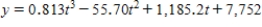 ,for
,for 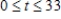 ,where t is the time in years,with
,where t is the time in years,with 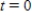 corresponding to 1972.Use the test for increasing and decreasing functions to estimate the years during which the number of medical degrees is increasing and the years during which it is decreasing.
corresponding to 1972.Use the test for increasing and decreasing functions to estimate the years during which the number of medical degrees is increasing and the years during which it is decreasing.
A) The number of medical degrees is increasing from 1972 to 1989 and 1997 to 2005, and decreasing during 1989 to 1997.
B) The number of medical degrees is increasing from 1972 to 1988 and 1996 to 2005, and decreasing during 1988 to 1996.
C) The number of medical degrees is increasing from 1972 to 1989 and 2001 to 2005, and decreasing during 1989 to 2001.
D) The number of medical degrees is increasing from 1972 to 1990 and 1996 to 2005, and decreasing during 1990 to 2001.
E) The number of medical degrees is increasing from 1972 to 1989 and 1995 to 2005,and decreasing during 1989 to 1995.
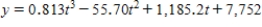 ,for
,for 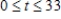 ,where t is the time in years,with
,where t is the time in years,with 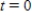 corresponding to 1972.Use the test for increasing and decreasing functions to estimate the years during which the number of medical degrees is increasing and the years during which it is decreasing.
corresponding to 1972.Use the test for increasing and decreasing functions to estimate the years during which the number of medical degrees is increasing and the years during which it is decreasing. A) The number of medical degrees is increasing from 1972 to 1989 and 1997 to 2005, and decreasing during 1989 to 1997.
B) The number of medical degrees is increasing from 1972 to 1988 and 1996 to 2005, and decreasing during 1988 to 1996.
C) The number of medical degrees is increasing from 1972 to 1989 and 2001 to 2005, and decreasing during 1989 to 2001.
D) The number of medical degrees is increasing from 1972 to 1990 and 1996 to 2005, and decreasing during 1990 to 2001.
E) The number of medical degrees is increasing from 1972 to 1989 and 1995 to 2005,and decreasing during 1989 to 1995.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
7
Locate the absolute extrema of the function 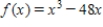 on the closed interval
on the closed interval 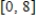
A) absolute max: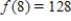 ; absolute min:
; absolute min: 
B) absolute max: ; absolute min:
; absolute min: 
C) absolute max: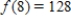 ; absolute min: no absolute min
; absolute min: no absolute min
D) no absolute max; absolute min: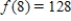
E) no absolute max or min
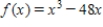 on the closed interval
on the closed interval 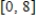
A) absolute max:
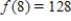 ; absolute min:
; absolute min: 
B) absolute max:
 ; absolute min:
; absolute min: 
C) absolute max:
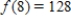 ; absolute min: no absolute min
; absolute min: no absolute minD) no absolute max; absolute min:
E) no absolute max or min

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
8
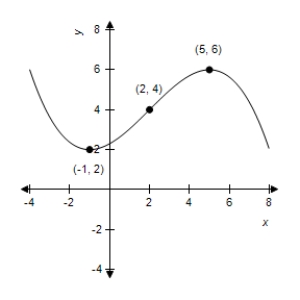
Use the graph of  to identify at which of the indicated points the derivative
to identify at which of the indicated points the derivative 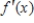 changes from negative to positive.
changes from negative to positive. 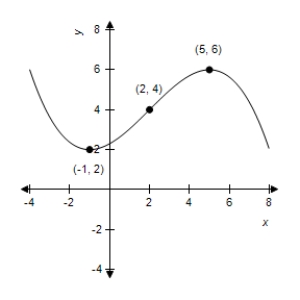
A) (2,4), (5,6)
B) (-1,2)
C) (-1,2), (2,4)
D) (2,4)
E) (-1,2),(5,6)
 to identify at which of the indicated points the derivative
to identify at which of the indicated points the derivative 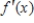 changes from negative to positive.
changes from negative to positive. 
A) (2,4), (5,6)
B) (-1,2)
C) (-1,2), (2,4)
D) (2,4)
E) (-1,2),(5,6)

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
9
Locate the absolute extrema of the function 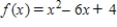 on the closed interval
on the closed interval 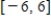
A) no absolute max; absolute min: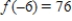
B) absolute max: ; absolute min:
; absolute min: 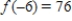
C) absolute max: ; no absolute min
; no absolute min
D) absolute max: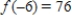 ;
; 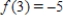
E) no absolute max or min
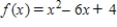 on the closed interval
on the closed interval 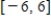
A) no absolute max; absolute min:
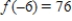
B) absolute max:
 ; absolute min:
; absolute min: 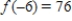
C) absolute max:
 ; no absolute min
; no absolute minD) absolute max:
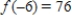 ;
; 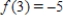
E) no absolute max or min

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
10
For the given function,find all critical numbers.

A) 
B) 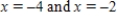
C) 
D) 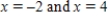
E) 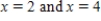

A)

B)
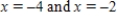
C)

D)
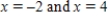
E)
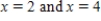

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
11
Identify the open intervals where the function 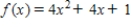 is increasing or decreasing.
is increasing or decreasing.
A) decreasing: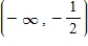 ; increasing:
; increasing: 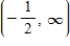
B) increasing: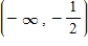 ; decreasing:
; decreasing: 
C) increasing on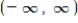
D) decreasing on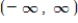
E) none of the above
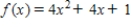 is increasing or decreasing.
is increasing or decreasing. A) decreasing:
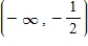 ; increasing:
; increasing: 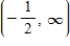
B) increasing:
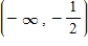 ; decreasing:
; decreasing: 
C) increasing on
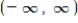
D) decreasing on
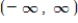
E) none of the above

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
12
Find all relative minima of the given function.
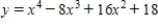
A) 
B) 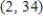
C) 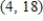
D) 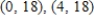
E) no relative maxima
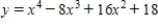
A)

B)
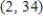
C)
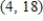
D)
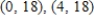
E) no relative maxima

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
13
Identify the open intervals where the function 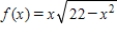 is increasing or decreasing.
is increasing or decreasing.
A) decreasing: ; increasing:
; increasing: 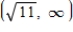
B) increasing: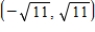 ; decreasing:
; decreasing: 
C) increasing: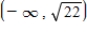 ; decreasing:
; decreasing: 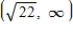
D) increasing: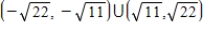 ; decreasing:
; decreasing: 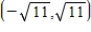
E) decreasing for all x
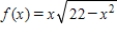 is increasing or decreasing.
is increasing or decreasing. A) decreasing:
 ; increasing:
; increasing: 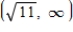
B) increasing:
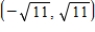 ; decreasing:
; decreasing: 
C) increasing:
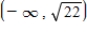 ; decreasing:
; decreasing: 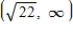
D) increasing:
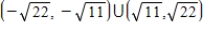 ; decreasing:
; decreasing: 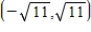
E) decreasing for all x

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
14
Find the open intervals on which the function 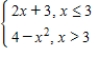 is increasing or decreasing.
is increasing or decreasing.
A) The function is increasing on the interval and decreasing on the interval
and decreasing on the interval 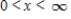
B) The function is increasing on the interval and decreasing on the interval
and decreasing on the interval 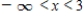
C) The function is increasing on the interval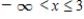 and decreasing on the interval
and decreasing on the interval 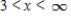
D) The function is increasing on the interval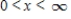 and decreasing on the interval
and decreasing on the interval 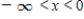
E) The function is increasing on the interval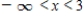 and decreasing on the interval
and decreasing on the interval 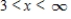
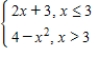 is increasing or decreasing.
is increasing or decreasing. A) The function is increasing on the interval
 and decreasing on the interval
and decreasing on the interval 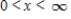
B) The function is increasing on the interval
 and decreasing on the interval
and decreasing on the interval 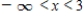
C) The function is increasing on the interval
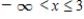 and decreasing on the interval
and decreasing on the interval 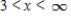
D) The function is increasing on the interval
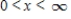 and decreasing on the interval
and decreasing on the interval 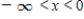
E) The function is increasing on the interval
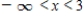 and decreasing on the interval
and decreasing on the interval 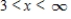

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
15
Find any critical numbers of the function 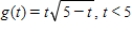 .
.
A) 0
B) 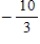
C) 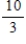
D) both A and B
E) both A and C
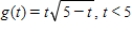 .
. A) 0
B)
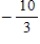
C)
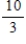
D) both A and B
E) both A and C

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
16
Use the graph of  to identify at which of the indicated points the derivative
to identify at which of the indicated points the derivative  changes from positive to negative.
changes from positive to negative.
A) (5, 6)
B) (-1, 2), (2, 4)
C) (2, 4), (5, 6)
D) (2, 4)
E) (-1,2)
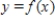 to identify at which of the indicated points the derivative
to identify at which of the indicated points the derivative  changes from positive to negative.
changes from positive to negative.

A) (5, 6)
B) (-1, 2), (2, 4)
C) (2, 4), (5, 6)
D) (2, 4)
E) (-1,2)

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
17
For the given function,find the relative minima.
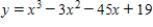
A) 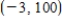
B) 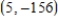
C) 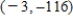
D) 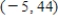
E) no relative minima
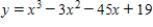
A)
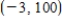
B)
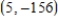
C)
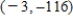
D)
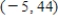
E) no relative minima

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
18
Find all relative maxima of the given function.
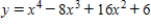
A) 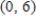
B) 
C) 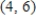
D) 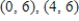
E) no relative maxima
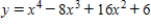
A)
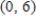
B)

C)
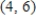
D)
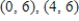
E) no relative maxima

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
19
A fast-food restaurant determines the cost model, 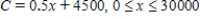 and revenue model,
and revenue model, 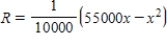 for
for 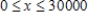 where x is the number of hamburgers sold.Determine the intervals on which the profit function is increasing and on which it is decreasing.
where x is the number of hamburgers sold.Determine the intervals on which the profit function is increasing and on which it is decreasing.
A) The profit function is increasing on the interval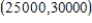 and decreasing on the interval
and decreasing on the interval 
B) The profit function is increasing on the interval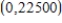 and decreasing on the interval
and decreasing on the interval 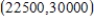
C) The profit function is increasing on the interval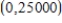 and decreasing on the interval
and decreasing on the interval 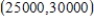
D) The profit function is increasing on the interval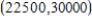 and decreasing on the interval
and decreasing on the interval 
E) The profit function is increasing on the interval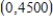 and decreasing on the interval
and decreasing on the interval 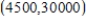
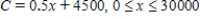 and revenue model,
and revenue model, 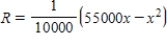 for
for 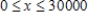 where x is the number of hamburgers sold.Determine the intervals on which the profit function is increasing and on which it is decreasing.
where x is the number of hamburgers sold.Determine the intervals on which the profit function is increasing and on which it is decreasing.
A) The profit function is increasing on the interval
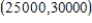 and decreasing on the interval
and decreasing on the interval 
B) The profit function is increasing on the interval
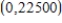 and decreasing on the interval
and decreasing on the interval 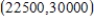
C) The profit function is increasing on the interval
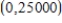 and decreasing on the interval
and decreasing on the interval 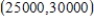
D) The profit function is increasing on the interval
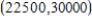 and decreasing on the interval
and decreasing on the interval 
E) The profit function is increasing on the interval
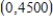 and decreasing on the interval
and decreasing on the interval 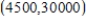

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
20
For the given function,find the critical numbers.

A) 
B) 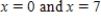
C) 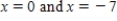
D) 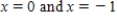
E) 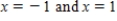

A)

B)
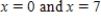
C)
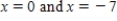
D)
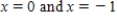
E)
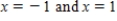

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
21
Sketch a graph of a function f having the following characteristics.
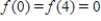
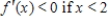
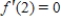
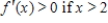
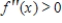
A) 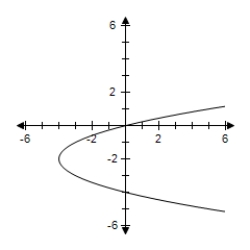
B) 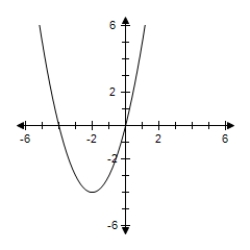
C)
D) 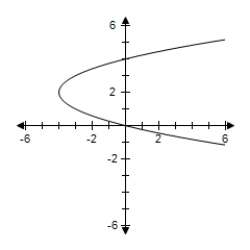
E) 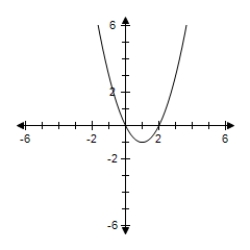
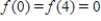
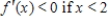
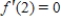
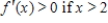
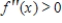
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
22
Determine the open intervals on which the graph of 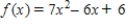 is concave downward or concave upward.
is concave downward or concave upward.
A) concave upward on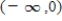 ; concave downward on
; concave downward on 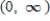
B) concave downward on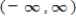
C) concave upward on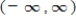
D) concave downward on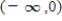 ; concave upward
; concave upward 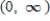
E) concave upward on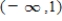 ; concave downward on
; concave downward on 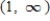
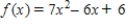 is concave downward or concave upward.
is concave downward or concave upward.A) concave upward on
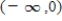 ; concave downward on
; concave downward on 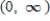
B) concave downward on
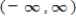
C) concave upward on
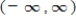
D) concave downward on
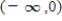 ; concave upward
; concave upward 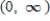
E) concave upward on
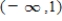 ; concave downward on
; concave downward on 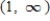

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
23
Medication.The number of milligrams x of a medication in the bloodstream t hours after a dose is taken can be modeled by 
 Find the t-value at which x is maximum.Round your answer to two decimal places.
Find the t-value at which x is maximum.Round your answer to two decimal places.
A) 0 hours
B) 2.83 hours
C) 707.11 hours
D) 4.83 hours
E) 6.91 hours

 Find the t-value at which x is maximum.Round your answer to two decimal places.
Find the t-value at which x is maximum.Round your answer to two decimal places. A) 0 hours
B) 2.83 hours
C) 707.11 hours
D) 4.83 hours
E) 6.91 hours

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
24
Find all relative extrema of the function  Use the Second Derivative Test where applicable.
Use the Second Derivative Test where applicable.
A) relative max: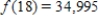 ; no relative min
; no relative min
B) relative max: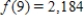 ; no relative min
; no relative min
C) no relative max or min
D) relative min: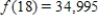 ; no relative max
; no relative max
E) relative min: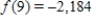 ; no relative max
; no relative max
 Use the Second Derivative Test where applicable.
Use the Second Derivative Test where applicable.
A) relative max:
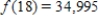 ; no relative min
; no relative minB) relative max:
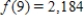 ; no relative min
; no relative minC) no relative max or min
D) relative min:
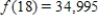 ; no relative max
; no relative maxE) relative min:
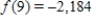 ; no relative max
; no relative max
Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
25
Suppose the resident population P(in millions)of the United States can be modeled by  ,
,  ,where
,where 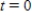 corresponds to 1800.Analytically find the minimum and maximum populations in the U.S.for
corresponds to 1800.Analytically find the minimum and maximum populations in the U.S.for 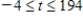
A) The population is minimum at and maximum at
and maximum at 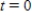
B) The population is minimum at and maximum at
and maximum at 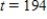
C) The population is minimum at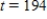 and maximum at
and maximum at 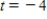
D) The population is minimum at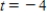 and maximum at
and maximum at 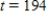
E) The population is minimum at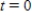 and maximum at
and maximum at 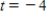
 ,
,  ,where
,where 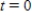 corresponds to 1800.Analytically find the minimum and maximum populations in the U.S.for
corresponds to 1800.Analytically find the minimum and maximum populations in the U.S.for 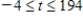
A) The population is minimum at
 and maximum at
and maximum at 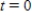
B) The population is minimum at
 and maximum at
and maximum at 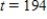
C) The population is minimum at
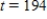 and maximum at
and maximum at 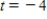
D) The population is minimum at
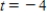 and maximum at
and maximum at 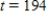
E) The population is minimum at
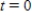 and maximum at
and maximum at 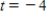

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
26
Find all relative extrema of the function  Use the Second-Derivative Test when applicable.
Use the Second-Derivative Test when applicable.
A) The relative minimum is and the relative maximum is
and the relative maximum is 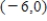
B) The relative maximum is
C) The relative minimum is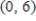
D) The relative maximum is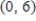 and the relative minima are
and the relative minima are  and
and 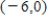
E) The relative minimum is and the relative maximum is
and the relative maximum is 
 Use the Second-Derivative Test when applicable.
Use the Second-Derivative Test when applicable. A) The relative minimum is
 and the relative maximum is
and the relative maximum is 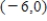
B) The relative maximum is

C) The relative minimum is
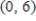
D) The relative maximum is
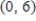 and the relative minima are
and the relative minima are  and
and 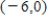
E) The relative minimum is
 and the relative maximum is
and the relative maximum is 

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
27
State the signs of 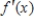 and
and 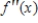 on the interval (0,2).
on the interval (0,2).

A) f ' < 0
f '' = 0
B) f ' < 0
f '' > 0
C) f ' > 0
f '' < 0
D) f ' > 0
f '' > 0
E) f ' < 0
f '' < 0
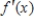 and
and 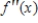 on the interval (0,2).
on the interval (0,2).

A) f ' < 0
f '' = 0
B) f ' < 0
f '' > 0
C) f ' > 0
f '' < 0
D) f ' > 0
f '' > 0
E) f ' < 0
f '' < 0

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
28
The graph of f is shown.Graph f,f ' and f '' on the same set of coordinate axes.
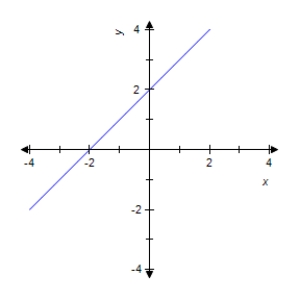
A) 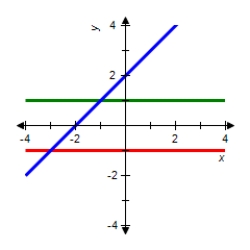
B) 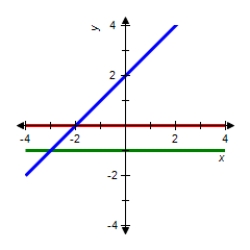
C) 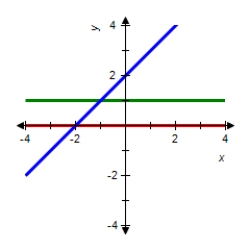
D) 
E) none of the above

A)

B)

C)

D)

E) none of the above

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
29
A function and its graph are given.Use the second derivative to locate all x-values of points of inflection on the graph of  Check these results against the graph shown.
Check these results against the graph shown.

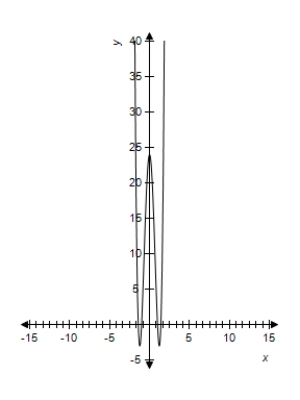
A) 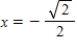
B) 
C) 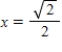
D) 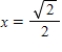 ,
, 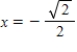
E)  ,
, 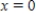 ,
, 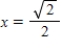
 Check these results against the graph shown.
Check these results against the graph shown.


A)
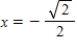
B)

C)
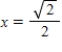
D)
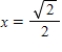 ,
, 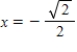
E)
 ,
, 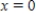 ,
, 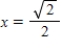

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
30
Find the points of inflection and discuss the concavity of the function.
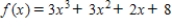
A) inflection point at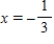 ; concave downward on
; concave downward on 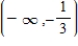 ; concave upward on
; concave upward on 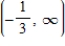
B) inflection point at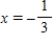 ; concave upward on
; concave upward on 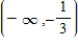 ; concave downward on
; concave downward on 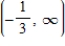
C) inflection point at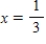 ; concave downward on
; concave downward on  ; concave upward on
; concave upward on 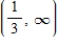
D) inflection point at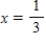 ; concave upward on
; concave upward on 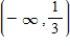 ; concave downward on
; concave downward on 
E) none of the above
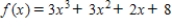
A) inflection point at
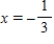 ; concave downward on
; concave downward on 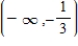 ; concave upward on
; concave upward on 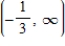
B) inflection point at
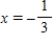 ; concave upward on
; concave upward on 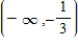 ; concave downward on
; concave downward on 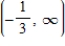
C) inflection point at
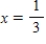 ; concave downward on
; concave downward on  ; concave upward on
; concave upward on 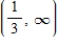
D) inflection point at
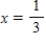 ; concave upward on
; concave upward on 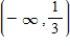 ; concave downward on
; concave downward on 
E) none of the above

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
31
Find the absolute extrema of the function 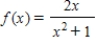 on the interval
on the interval 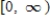
A) The maximum of the function is 1 and the minimum of the function is 0..
B) The maximum of the function is 0 and the minimum of the function is -1..
C) The maximum of the function is -1 and the minimum of the function is 0.
D) The maximum of the function is 1 and the minimum of the function is 0.
E) The maximum of the function is 0 and the minimum of the function is 1.
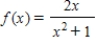 on the interval
on the interval 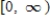
A) The maximum of the function is 1 and the minimum of the function is 0..
B) The maximum of the function is 0 and the minimum of the function is -1..
C) The maximum of the function is -1 and the minimum of the function is 0.
D) The maximum of the function is 1 and the minimum of the function is 0.
E) The maximum of the function is 0 and the minimum of the function is 1.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
32
Find all relative extrema of the function 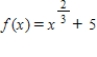 Use the Second Derivative Test where applicable.
Use the Second Derivative Test where applicable.
A) relative max:
B) relative min: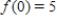
C) no relative max or min
D) both A and B
E) none of the above
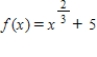 Use the Second Derivative Test where applicable.
Use the Second Derivative Test where applicable. A) relative max:

B) relative min:
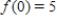
C) no relative max or min
D) both A and B
E) none of the above

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
33
Find the x-value at which the given function has a point of inflection.
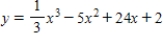
A) 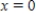
B) 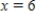
C) 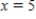
D) 
E) no point of inflection
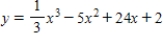
A)
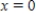
B)
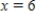
C)
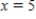
D)

E) no point of inflection

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
34
Find all relative extrema of the function  Use the Second-Derivative Test when applicable.
Use the Second-Derivative Test when applicable.
A) The relative maximum is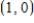
B) The relative minimum is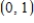
C) The relative maximum is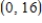
D) The relative minimum is
E) The relative maximum is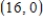
 Use the Second-Derivative Test when applicable.
Use the Second-Derivative Test when applicable.A) The relative maximum is
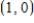
B) The relative minimum is
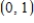
C) The relative maximum is
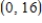
D) The relative minimum is

E) The relative maximum is
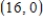

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
35
Graph a function on the interval 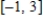 having the following characteristics. Absolute maximum at
having the following characteristics. Absolute maximum at 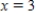 Absolute minimum at
Absolute minimum at 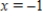 Relative minimum at
Relative minimum at  Relative maximum at
Relative maximum at 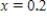
A) 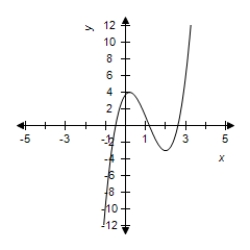
B) 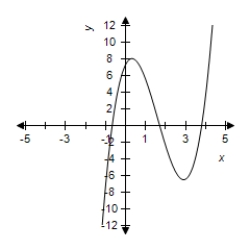
C) 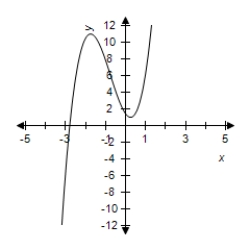
D) 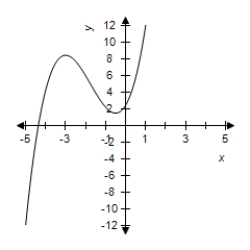
E) 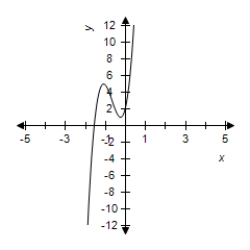
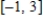 having the following characteristics. Absolute maximum at
having the following characteristics. Absolute maximum at 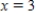 Absolute minimum at
Absolute minimum at 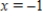 Relative minimum at
Relative minimum at  Relative maximum at
Relative maximum at 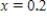
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
36
Find the absolute extrema of the function 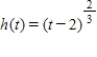 on the closed interval
on the closed interval 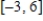 Round your answer to two decimal places.
Round your answer to two decimal places.
A) The maximum of the function is 1 and the minimum of the function is 0.
B) The maximum of the function is 2.92 and the minimum of the function is 1.
C) The maximum of the function is 2.92 and the minimum of the function is 0.
D) The maximum of the function is1 and the minimum of the function is 2.52.
E) The maximum of the function is 0 and the minimum of the function is 2.52.
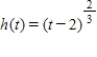 on the closed interval
on the closed interval 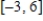 Round your answer to two decimal places.
Round your answer to two decimal places. A) The maximum of the function is 1 and the minimum of the function is 0.
B) The maximum of the function is 2.92 and the minimum of the function is 1.
C) The maximum of the function is 2.92 and the minimum of the function is 0.
D) The maximum of the function is1 and the minimum of the function is 2.52.
E) The maximum of the function is 0 and the minimum of the function is 2.52.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
37
Medication.The number of milligrams x of a medication in the bloodstream t hours after a dose is taken can be modeled by 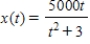
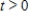 Find the maximum value of x.Round your answer to two decimal places.
Find the maximum value of x.Round your answer to two decimal places.
A) 1.73 hours.
B) 1443.37 hours
C) 3.73 hours
D) 4.22 hours
E) 1.73 hours
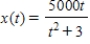
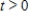 Find the maximum value of x.Round your answer to two decimal places.
Find the maximum value of x.Round your answer to two decimal places. A) 1.73 hours.
B) 1443.37 hours
C) 3.73 hours
D) 4.22 hours
E) 1.73 hours

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
38
Approximate the critical numbers of the function shown in the graph and determine whether the function has a relative maximum,a relative minimum,an absolute maximum,an absolute minimum,or none of these at each critical number on the interval shown. 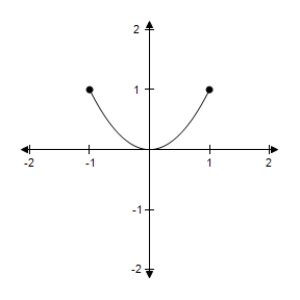
A) The critical number 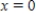 yields an absolute maximum and the critical number
yields an absolute maximum and the critical number 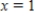 yields an absolute minimum.
yields an absolute minimum.
B) Both the critical numbers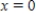 &
& 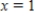 yield an absolute maximum.
yield an absolute maximum.
C) The critical number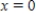 yields an absolute minimum and the critical number
yields an absolute minimum and the critical number 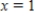 yields an absolute maximum.
yields an absolute maximum.
D) Both the critical numbers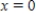 and
and 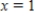 yield an absolute minimum.
yield an absolute minimum.
E) The critical number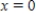 yields a relative minimum and the critical number
yields a relative minimum and the critical number 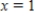 yields a relative maximum.
yields a relative maximum.

A) The critical number
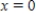 yields an absolute maximum and the critical number
yields an absolute maximum and the critical number 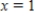 yields an absolute minimum.
yields an absolute minimum.B) Both the critical numbers
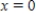 &
& 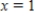 yield an absolute maximum.
yield an absolute maximum.C) The critical number
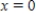 yields an absolute minimum and the critical number
yields an absolute minimum and the critical number 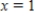 yields an absolute maximum.
yields an absolute maximum.D) Both the critical numbers
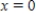 and
and 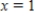 yield an absolute minimum.
yield an absolute minimum.E) The critical number
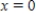 yields a relative minimum and the critical number
yields a relative minimum and the critical number 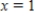 yields a relative maximum.
yields a relative maximum.
Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
39
Determine the open intervals on which the graph of 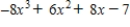 is concave downward or concave upward.
is concave downward or concave upward.
A) concave downward on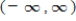
B) concave downward on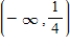 ; concave upward on
; concave upward on 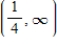
C) concave upward on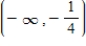 ; concave downward on
; concave downward on 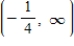
D) concave downward on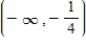 ; concave upward on
; concave upward on 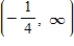
E) concave upward on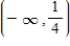 ; concave downward on
; concave downward on 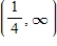
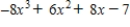 is concave downward or concave upward.
is concave downward or concave upward. A) concave downward on
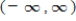
B) concave downward on
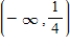 ; concave upward on
; concave upward on 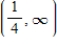
C) concave upward on
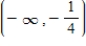 ; concave downward on
; concave downward on 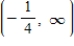
D) concave downward on
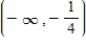 ; concave upward on
; concave upward on 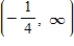
E) concave upward on
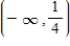 ; concave downward on
; concave downward on 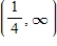

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
40
Find the x-value at which the absolute minimum of f(x)occurs on the interval [a,b].
![<strong>Find the x-value at which the absolute minimum of f(x)occurs on the interval [a,b]. </strong> A) B) C) D) E) ](https://storage.examlex.com/TB1301/11ea8970_6a28_c2d2_861b_11e8b722a524_TB1301_11.jpg)
A) ![<strong>Find the x-value at which the absolute minimum of f(x)occurs on the interval [a,b]. </strong> A) B) C) D) E) ](https://storage.examlex.com/TB1301/11ea8970_6a28_e9e3_861b_41b2fa8d7b94_TB1301_11.jpg)
B) ![<strong>Find the x-value at which the absolute minimum of f(x)occurs on the interval [a,b]. </strong> A) B) C) D) E) ](https://storage.examlex.com/TB1301/11ea8970_6a28_e9e4_861b_4faf7d96be92_TB1301_11.jpg)
C) ![<strong>Find the x-value at which the absolute minimum of f(x)occurs on the interval [a,b]. </strong> A) B) C) D) E) ](https://storage.examlex.com/TB1301/11ea8970_6a28_e9e5_861b_8bd7ea811539_TB1301_11.jpg)
D) ![<strong>Find the x-value at which the absolute minimum of f(x)occurs on the interval [a,b]. </strong> A) B) C) D) E) ](https://storage.examlex.com/TB1301/11ea8970_6a28_e9e6_861b_ffd11e05b186_TB1301_11.jpg)
E) ![<strong>Find the x-value at which the absolute minimum of f(x)occurs on the interval [a,b]. </strong> A) B) C) D) E) ](https://storage.examlex.com/TB1301/11ea8970_6a29_10f7_861b_e91db55c8201_TB1301_11.jpg)
![<strong>Find the x-value at which the absolute minimum of f(x)occurs on the interval [a,b]. </strong> A) B) C) D) E) ](https://storage.examlex.com/TB1301/11ea8970_6a28_c2d2_861b_11e8b722a524_TB1301_11.jpg)
A)
![<strong>Find the x-value at which the absolute minimum of f(x)occurs on the interval [a,b]. </strong> A) B) C) D) E) ](https://storage.examlex.com/TB1301/11ea8970_6a28_e9e3_861b_41b2fa8d7b94_TB1301_11.jpg)
B)
![<strong>Find the x-value at which the absolute minimum of f(x)occurs on the interval [a,b]. </strong> A) B) C) D) E) ](https://storage.examlex.com/TB1301/11ea8970_6a28_e9e4_861b_4faf7d96be92_TB1301_11.jpg)
C)
![<strong>Find the x-value at which the absolute minimum of f(x)occurs on the interval [a,b]. </strong> A) B) C) D) E) ](https://storage.examlex.com/TB1301/11ea8970_6a28_e9e5_861b_8bd7ea811539_TB1301_11.jpg)
D)
![<strong>Find the x-value at which the absolute minimum of f(x)occurs on the interval [a,b]. </strong> A) B) C) D) E) ](https://storage.examlex.com/TB1301/11ea8970_6a28_e9e6_861b_ffd11e05b186_TB1301_11.jpg)
E)
![<strong>Find the x-value at which the absolute minimum of f(x)occurs on the interval [a,b]. </strong> A) B) C) D) E) ](https://storage.examlex.com/TB1301/11ea8970_6a29_10f7_861b_e91db55c8201_TB1301_11.jpg)

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
41
If the total cost function for a product is 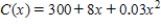 dollars.Find the minimum average cost.
dollars.Find the minimum average cost.
A) $14.25 per unit
B) $18 per unit
C) $14.36 per unit
D) $14 per unit
E) $13 per unit
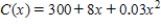 dollars.Find the minimum average cost.
dollars.Find the minimum average cost. A) $14.25 per unit
B) $18 per unit
C) $14.36 per unit
D) $14 per unit
E) $13 per unit

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
42
A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: ![<strong>A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: where k > 0 is the proportionality constant.] </strong> A) inches and inches B) inches and inches C) inches and inches D) inches and inches E) inches and inches](https://storage.examlex.com/TB1301/11ea8970_6a31_26ff_861b_8d445c2fc44e_TB1301_11.jpg) where k > 0 is the proportionality constant.]
where k > 0 is the proportionality constant.] ![<strong>A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: where k > 0 is the proportionality constant.] </strong> A) inches and inches B) inches and inches C) inches and inches D) inches and inches E) inches and inches](https://storage.examlex.com/TB1301/11ea8970_6a31_2700_861b_4f11f8d2b4c7_TB1301_00.jpg)
A)![<strong>A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: where k > 0 is the proportionality constant.] </strong> A) inches and inches B) inches and inches C) inches and inches D) inches and inches E) inches and inches](https://storage.examlex.com/TB1301/11ea8970_6a31_2701_861b_91398ba9b0c2_TB1301_11.jpg) inches and
inches and ![<strong>A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: where k > 0 is the proportionality constant.] </strong> A) inches and inches B) inches and inches C) inches and inches D) inches and inches E) inches and inches](https://storage.examlex.com/TB1301/11ea8970_6a31_2702_861b_e974f7349586_TB1301_11.jpg) inches
inches
B)![<strong>A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: where k > 0 is the proportionality constant.] </strong> A) inches and inches B) inches and inches C) inches and inches D) inches and inches E) inches and inches](https://storage.examlex.com/TB1301/11ea8970_6a31_4e13_861b_1b3c3d464f1a_TB1301_11.jpg) inches and
inches and ![<strong>A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: where k > 0 is the proportionality constant.] </strong> A) inches and inches B) inches and inches C) inches and inches D) inches and inches E) inches and inches](https://storage.examlex.com/TB1301/11ea8970_6a31_4e14_861b_2f49d292c854_TB1301_11.jpg) inches
inches
C)![<strong>A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: where k > 0 is the proportionality constant.] </strong> A) inches and inches B) inches and inches C) inches and inches D) inches and inches E) inches and inches](https://storage.examlex.com/TB1301/11ea8970_6a31_4e15_861b_7d139805eca6_TB1301_11.jpg) inches and
inches and ![<strong>A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: where k > 0 is the proportionality constant.] </strong> A) inches and inches B) inches and inches C) inches and inches D) inches and inches E) inches and inches](https://storage.examlex.com/TB1301/11ea8970_6a31_4e16_861b_f1d7dda4b0a6_TB1301_11.jpg) inches
inches
D)![<strong>A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: where k > 0 is the proportionality constant.] </strong> A) inches and inches B) inches and inches C) inches and inches D) inches and inches E) inches and inches](https://storage.examlex.com/TB1301/11ea8970_6a31_4e17_861b_c3ffa7e8e9ac_TB1301_11.jpg) inches and
inches and ![<strong>A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: where k > 0 is the proportionality constant.] </strong> A) inches and inches B) inches and inches C) inches and inches D) inches and inches E) inches and inches](https://storage.examlex.com/TB1301/11ea8970_6a31_7528_861b_15645d055f4b_TB1301_11.jpg) inches
inches
E)![<strong>A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: where k > 0 is the proportionality constant.] </strong> A) inches and inches B) inches and inches C) inches and inches D) inches and inches E) inches and inches](https://storage.examlex.com/TB1301/11ea8970_6a31_7529_861b_a31a1ac4439b_TB1301_11.jpg) inches and
inches and ![<strong>A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: where k > 0 is the proportionality constant.] </strong> A) inches and inches B) inches and inches C) inches and inches D) inches and inches E) inches and inches](https://storage.examlex.com/TB1301/11ea8970_6a31_752a_861b_71c9fec96556_TB1301_11.jpg) inches
inches
![<strong>A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: where k > 0 is the proportionality constant.] </strong> A) inches and inches B) inches and inches C) inches and inches D) inches and inches E) inches and inches](https://storage.examlex.com/TB1301/11ea8970_6a31_26ff_861b_8d445c2fc44e_TB1301_11.jpg) where k > 0 is the proportionality constant.]
where k > 0 is the proportionality constant.] ![<strong>A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: where k > 0 is the proportionality constant.] </strong> A) inches and inches B) inches and inches C) inches and inches D) inches and inches E) inches and inches](https://storage.examlex.com/TB1301/11ea8970_6a31_2700_861b_4f11f8d2b4c7_TB1301_00.jpg)
A)
![<strong>A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: where k > 0 is the proportionality constant.] </strong> A) inches and inches B) inches and inches C) inches and inches D) inches and inches E) inches and inches](https://storage.examlex.com/TB1301/11ea8970_6a31_2701_861b_91398ba9b0c2_TB1301_11.jpg) inches and
inches and ![<strong>A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: where k > 0 is the proportionality constant.] </strong> A) inches and inches B) inches and inches C) inches and inches D) inches and inches E) inches and inches](https://storage.examlex.com/TB1301/11ea8970_6a31_2702_861b_e974f7349586_TB1301_11.jpg) inches
inchesB)
![<strong>A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: where k > 0 is the proportionality constant.] </strong> A) inches and inches B) inches and inches C) inches and inches D) inches and inches E) inches and inches](https://storage.examlex.com/TB1301/11ea8970_6a31_4e13_861b_1b3c3d464f1a_TB1301_11.jpg) inches and
inches and ![<strong>A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: where k > 0 is the proportionality constant.] </strong> A) inches and inches B) inches and inches C) inches and inches D) inches and inches E) inches and inches](https://storage.examlex.com/TB1301/11ea8970_6a31_4e14_861b_2f49d292c854_TB1301_11.jpg) inches
inchesC)
![<strong>A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: where k > 0 is the proportionality constant.] </strong> A) inches and inches B) inches and inches C) inches and inches D) inches and inches E) inches and inches](https://storage.examlex.com/TB1301/11ea8970_6a31_4e15_861b_7d139805eca6_TB1301_11.jpg) inches and
inches and ![<strong>A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: where k > 0 is the proportionality constant.] </strong> A) inches and inches B) inches and inches C) inches and inches D) inches and inches E) inches and inches](https://storage.examlex.com/TB1301/11ea8970_6a31_4e16_861b_f1d7dda4b0a6_TB1301_11.jpg) inches
inchesD)
![<strong>A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: where k > 0 is the proportionality constant.] </strong> A) inches and inches B) inches and inches C) inches and inches D) inches and inches E) inches and inches](https://storage.examlex.com/TB1301/11ea8970_6a31_4e17_861b_c3ffa7e8e9ac_TB1301_11.jpg) inches and
inches and ![<strong>A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: where k > 0 is the proportionality constant.] </strong> A) inches and inches B) inches and inches C) inches and inches D) inches and inches E) inches and inches](https://storage.examlex.com/TB1301/11ea8970_6a31_7528_861b_15645d055f4b_TB1301_11.jpg) inches
inchesE)
![<strong>A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: where k > 0 is the proportionality constant.] </strong> A) inches and inches B) inches and inches C) inches and inches D) inches and inches E) inches and inches](https://storage.examlex.com/TB1301/11ea8970_6a31_7529_861b_a31a1ac4439b_TB1301_11.jpg) inches and
inches and ![<strong>A wooden beam has a rectangular cross section of height h and width w (see figure).The strength S of the beam is directly proportional to the width and the square of the height.What are the dimensions of the strongest beam that can be cut from a round log of diameter d = 26 inches? Round your answers to two decimal places.[Hint: where k > 0 is the proportionality constant.] </strong> A) inches and inches B) inches and inches C) inches and inches D) inches and inches E) inches and inches](https://storage.examlex.com/TB1301/11ea8970_6a31_752a_861b_71c9fec96556_TB1301_11.jpg) inches
inches
Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
43
A rectangular page is to contain 144 square inches of print.The margins on each side are 1 inch.Find the dimensions of the page such that the least amount of paper is used.
A) 14, 14
B) 12, 12
C) 10, 10
D) 13, 13
E) 11,11
A) 14, 14
B) 12, 12
C) 10, 10
D) 13, 13
E) 11,11

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
44
Average costs.Suppose the average costs of a mining operation depend on the number of machines used,and average costs,in dollars,are given by 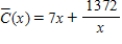 ,
, 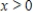 ,where x is the number of machines used.How many machines give minimum average costs?
,where x is the number of machines used.How many machines give minimum average costs?
A) Using 14 machines gives the minimum average costs.
B) Using zero machines gives the minimum average costs.
C) Using 24 machines gives the minimum average costs.
D) Using 28 machines gives the minimum average costs.
E) Using 33 machines gives the minimum average costs.
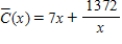 ,
, 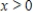 ,where x is the number of machines used.How many machines give minimum average costs?
,where x is the number of machines used.How many machines give minimum average costs? A) Using 14 machines gives the minimum average costs.
B) Using zero machines gives the minimum average costs.
C) Using 24 machines gives the minimum average costs.
D) Using 28 machines gives the minimum average costs.
E) Using 33 machines gives the minimum average costs.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
45
If the total revenue function for a blender is 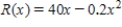 ,find the maximum revenue.
,find the maximum revenue.
A) $100
B) $2,500
C) $40
D) $250
E) $2,000
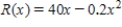 ,find the maximum revenue.
,find the maximum revenue. A) $100
B) $2,500
C) $40
D) $250
E) $2,000

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
46
Determine the dimensions of a rectangular solid (with a square base)with maximum volume if its surface area is 441 square meters.
A) square base side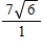 ; height
; height 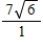
B) square base side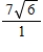 ; height
; height 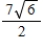
C) square base side ; height
; height 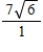
D) square base side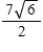 ; height
; height 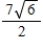
E) square base side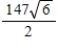 ; height
; height 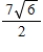
A) square base side
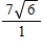 ; height
; height 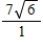
B) square base side
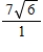 ; height
; height 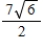
C) square base side
 ; height
; height 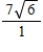
D) square base side
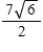 ; height
; height 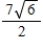
E) square base side
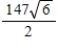 ; height
; height 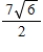

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
47
Minimum cost.From a tract of land,a developer plans to fence a rectangular region and then divide it into two identical rectangular lots by putting a fence down the middle.Suppose that the fence for the outside boundary costs $7 per foot and the fence for the middle costs $4 per foot.If each lot contains 5000 square feet,find the dimensions of each lot that yield the minimum cost for the fence.
A) Dimensions are 51.59 ft for the side parallel to the divider and 96.92 ft for the other side.
B) Dimensions are 96.92 ft for the side parallel to the divider and 51.59 ft for the other side.
C) Dimensions are 70.71 ft for the side parallel to the divider and 70.71 ft for the other side.
D) Dimensions are 88.19 ft for the side parallel to the divider and 56.7 ft for the other side.
E) Dimensions are 56.7 ft for the side parallel to the divider and 88.19 ft for the other side.
A) Dimensions are 51.59 ft for the side parallel to the divider and 96.92 ft for the other side.
B) Dimensions are 96.92 ft for the side parallel to the divider and 51.59 ft for the other side.
C) Dimensions are 70.71 ft for the side parallel to the divider and 70.71 ft for the other side.
D) Dimensions are 88.19 ft for the side parallel to the divider and 56.7 ft for the other side.
E) Dimensions are 56.7 ft for the side parallel to the divider and 88.19 ft for the other side.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
48
A firm has total revenue given by 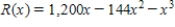 for x units of a product.Find the maximum revenue from sales of that product.
for x units of a product.Find the maximum revenue from sales of that product.
A) $2400
B) $2432
C) $404
D) $3300
E) $307
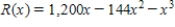 for x units of a product.Find the maximum revenue from sales of that product.
for x units of a product.Find the maximum revenue from sales of that product. A) $2400
B) $2432
C) $404
D) $3300
E) $307

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
49
Volume.A rectangular box with a square base is to be formed from a square piece of metal with 54-inch sides.If a square piece with side x is cut from each corner of the metal and the sides are folded up to form an open box,the volume of the box is 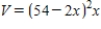 What value of x will maximize the volume of the box?
What value of x will maximize the volume of the box? 
A) 27
B) 2
C) 9
D) 14
E) 1
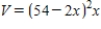 What value of x will maximize the volume of the box?
What value of x will maximize the volume of the box? 
A) 27
B) 2
C) 9
D) 14
E) 1

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
50
Find the point on the graph of  that is closest to the point (8,0.5).Round your answer to two decimal places.
that is closest to the point (8,0.5).Round your answer to two decimal places.
A) (1.59, 2.53)
B) (2, 4)
C) (2.52, 6.35)
D) (1.39, 1.93)
E) (1.26,1.59)
 that is closest to the point (8,0.5).Round your answer to two decimal places.
that is closest to the point (8,0.5).Round your answer to two decimal places. A) (1.59, 2.53)
B) (2, 4)
C) (2.52, 6.35)
D) (1.39, 1.93)
E) (1.26,1.59)

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
51
If the total revenue function for a blender is  ,determine how many units x must be sold to provide the maximum total revenue in dollars.
,determine how many units x must be sold to provide the maximum total revenue in dollars.
A) 625
B) 15,000
C) 25
D) 300
E) 50
 ,determine how many units x must be sold to provide the maximum total revenue in dollars.
,determine how many units x must be sold to provide the maximum total revenue in dollars. A) 625
B) 15,000
C) 25
D) 300
E) 50

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
52
A Norman window is constructed by adjoining a semicircle to the top of an ordinary rectangular window (see figure).Find the dimensions of a Norman window of maximum area if the total perimeter is 22 feet.Round yours answers to two decimal places. 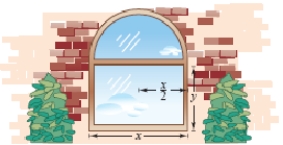
A) 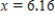 feet and
feet and 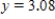 feet
feet
B) 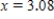 feet and
feet and 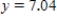 feet
feet
C) 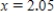 feet and
feet and 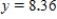 feet
feet
D) 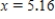 feet and
feet and 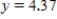 feet
feet
E) 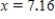 feet and
feet and 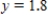 feet
feet

A)
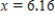 feet and
feet and 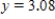 feet
feetB)
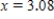 feet and
feet and 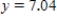 feet
feetC)
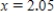 feet and
feet and 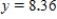 feet
feetD)
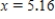 feet and
feet and 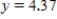 feet
feetE)
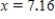 feet and
feet and 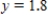 feet
feet
Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
53
Production.Suppose that the total number of units produced by a worker in t hours of an 8-hour shift can be modeled by the production function  Find the number of hours before the rate of production is maximized.That is,find the point of diminishing returns.
Find the number of hours before the rate of production is maximized.That is,find the point of diminishing returns.
A) 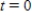
B) 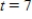
C) 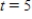
D) 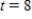
E) 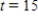
 Find the number of hours before the rate of production is maximized.That is,find the point of diminishing returns.
Find the number of hours before the rate of production is maximized.That is,find the point of diminishing returns. A)
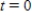
B)
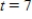
C)
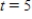
D)
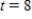
E)
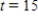

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
54
Find the length and width of a rectangle that has perimeter 56 meters and a maximum area.
A) 7, 21
B) 1, 27
C) 14, 14
D) 15, 13
E) 18,10
A) 7, 21
B) 1, 27
C) 14, 14
D) 15, 13
E) 18,10

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
55
You are in a boat 2 miles from the nearest point on the coast.You are to go to point Q located 3 miles down the coast and 1 mile inland (see figure).You can row at a rate of 1 miles per hour and you can walk at a rate of 2 miles per hour.Toward what point on the coast should you row in order to reach point Q in the least time? 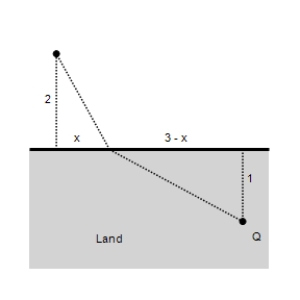
A) 3 miles
B) 8 miles
C) 2 miles
D) 1 mile
E) 5 miles

A) 3 miles
B) 8 miles
C) 2 miles
D) 1 mile
E) 5 miles

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
56
The profit P (in thousands of dollars)for a company spending an amount s (in thousands of dollars)on advertising is 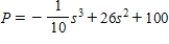 The point of diminishing returns is the point at which the rate of growth of the profit function begins to decline.Find the point of diminishing returns.Round your answer to the nearest thousand dollars.
The point of diminishing returns is the point at which the rate of growth of the profit function begins to decline.Find the point of diminishing returns.Round your answer to the nearest thousand dollars.
A) 43 thousand dollars
B) 130 thousand dollars
C) 104 thousand dollars
D) 173 thousand dollars
E) 87 thousand dollars
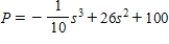 The point of diminishing returns is the point at which the rate of growth of the profit function begins to decline.Find the point of diminishing returns.Round your answer to the nearest thousand dollars.
The point of diminishing returns is the point at which the rate of growth of the profit function begins to decline.Find the point of diminishing returns.Round your answer to the nearest thousand dollars. A) 43 thousand dollars
B) 130 thousand dollars
C) 104 thousand dollars
D) 173 thousand dollars
E) 87 thousand dollars

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
57
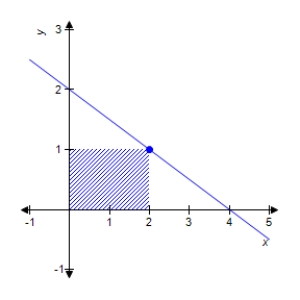
Find the dimensions of the rectangle of maximum area bounded by the x-axis and y-axis and the graph of 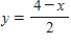
A) length 1.5; width 1.25
B) length 2; width 1
C) length 0.5; width 1.75
D) length 1; width 1.5
E) none of the above
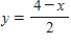

A) length 1.5; width 1.25
B) length 2; width 1
C) length 0.5; width 1.75
D) length 1; width 1.5
E) none of the above

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
58
The number of people who donated to a certain organization between 1975 and 1992 can be modeled by the equation  donors,where t is the number of years after 1975.Find the inflection point(s)from
donors,where t is the number of years after 1975.Find the inflection point(s)from  through
through  ,if any exist.
,if any exist.
A) There are no inflection points from
B) There is one inflection point at
C) There are inflection points at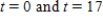
D) There is one inflection point at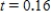
E) There are inflection points at ,
,  ,
, 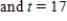
 donors,where t is the number of years after 1975.Find the inflection point(s)from
donors,where t is the number of years after 1975.Find the inflection point(s)from 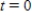 through
through 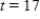 ,if any exist.
,if any exist. A) There are no inflection points from
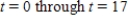
B) There is one inflection point at

C) There are inflection points at
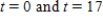
D) There is one inflection point at
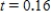
E) There are inflection points at
 ,
,  ,
, 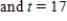

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
59
A rancher has 440 feet of fencing to enclose two adjacent rectangular corrals (see figure).What dimensions should be used so that the enclosed area will be a maximum?
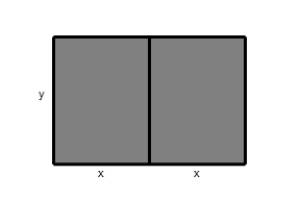
A) 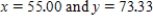
B) 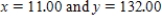
C) 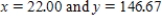
D) 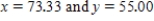
E) 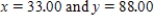

A)
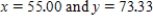
B)
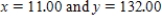
C)
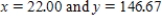
D)
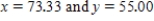
E)
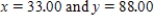

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
60
If the total cost function for a product is 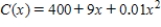 dollars,determine how many units x should be produced to minimize the average cost per unit?
dollars,determine how many units x should be produced to minimize the average cost per unit?
A) 450 units
B) 400 units
C) 141 units
D) 200 units
E) 97 units
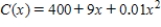 dollars,determine how many units x should be produced to minimize the average cost per unit?
dollars,determine how many units x should be produced to minimize the average cost per unit? A) 450 units
B) 400 units
C) 141 units
D) 200 units
E) 97 units

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
61
Find any vertical asymptotes for the given function.
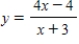
A) 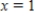
B) 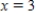
C) 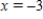
D) 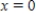
E) no vertical asymptotes
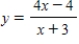
A)
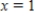
B)
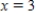
C)
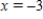
D)
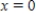
E) no vertical asymptotes

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
62
p is in dollars and q is the number of units.Find the elasticity of the demand function 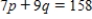 at the price
at the price 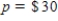
A) -4.04
B) 1.00
C) 4.04
D) -0.78
E) 0.78
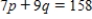 at the price
at the price 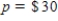
A) -4.04
B) 1.00
C) 4.04
D) -0.78
E) 0.78

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
63
A power station is on one side of a river that is 0.5 mile wide,and a factory is 6 miles downstream on the other side of the river.It costs $14 per foot to run overland power lines and $20 per foot to run underwater power lines.Estimate the value of x that minimizes the cost.The value of  is the length of the overland piece of power line.
is the length of the overland piece of power line.
A) 2.56
B) 5.51
C) 3
D) 5.49
E) 0.87
 is the length of the overland piece of power line.
is the length of the overland piece of power line. A) 2.56
B) 5.51
C) 3
D) 5.49
E) 0.87

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
64
A travel agency will plan a tour for groups of size 53 or larger.If the group contains exactly 53 people,the price is $800 per person.However,each person's price is reduced by $10 for each additional person above the 53.If the travel agency incurs a price of $150 per person for the tour,what size group will give the agency the maximum profit?
A) 6
B) 91
C) 59
D) 70
E) 32
A) 6
B) 91
C) 59
D) 70
E) 32

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
65
Find any vertical asymptotes for the given function.
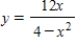
A) 
B)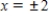
C) 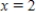
D) 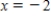
E) no vertical asymptotes
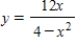
A)

B)
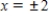
C)
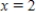
D)
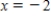
E) no vertical asymptotes

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
66
Average costs.Suppose the average costs of a mining operation depend on the number of machines used,and average costs,in dollars,are given by 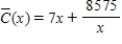 ,
, 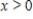 ,where x is the number of machines used.What is the minimum average cost?
,where x is the number of machines used.What is the minimum average cost?
A) $0
B) $35
C) $490
D) $245
E) $8582
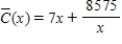 ,
, 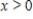 ,where x is the number of machines used.What is the minimum average cost?
,where x is the number of machines used.What is the minimum average cost? A) $0
B) $35
C) $490
D) $245
E) $8582

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
67
A function and its graph are given.Use the graph to find the horizontal asymptotes,if they exist,where 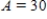 Confirm your results analytically.
Confirm your results analytically.


A) 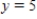
B) 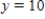
C) 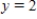
D) 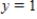
E) no horizontal asymptotes
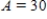 Confirm your results analytically.
Confirm your results analytically.


A)
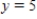
B)
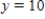
C)
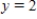
D)
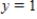
E) no horizontal asymptotes

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
68
Find the speed v,in miles per hour,that will minimize costs on a 125-mile delivery trip.The cost per hour for fuel is 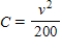 dollars,and the driver is paid
dollars,and the driver is paid 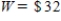 dollars per hour.(Assume there are no costs other than wages and fuel.)
dollars per hour.(Assume there are no costs other than wages and fuel.)
A) 75 mph
B) 125 mph
C) 200 mph
D) 80 mph
E) 40 mph
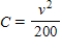 dollars,and the driver is paid
dollars,and the driver is paid 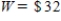 dollars per hour.(Assume there are no costs other than wages and fuel.)
dollars per hour.(Assume there are no costs other than wages and fuel.) A) 75 mph
B) 125 mph
C) 200 mph
D) 80 mph
E) 40 mph

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
69
Analytically determine the location(s)of any horizontal asymptote(s).

A) 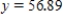
B) 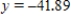
C) 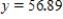 ,
, 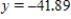
D) 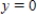
E) no horizontal asymptotes

A)
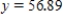
B)
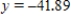
C)
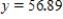 ,
, 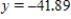
D)
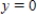
E) no horizontal asymptotes

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
70
Find any horizontal asymptotes for the given function.

A) 
B) 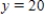
C) 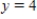
D) 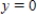
E) no horizontal asymptotes

A)

B)
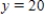
C)
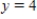
D)
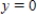
E) no horizontal asymptotes

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
71
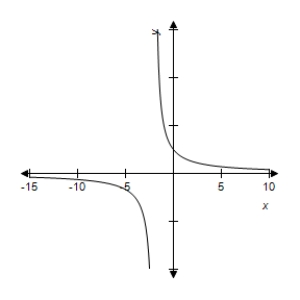
A function and its graph are given.Use the graph to find the vertical asymptotes,if they exist.Confirm your results analytically. 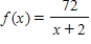
A) 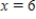
B) 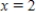
C) 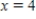
D) 
E) no vertical asymptotes
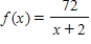

A)
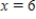
B)
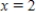
C)
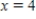
D)

E) no vertical asymptotes

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
72
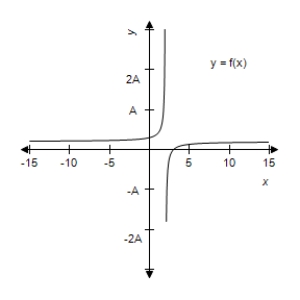
This problem contains a function and its graph,where 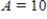 Use the graph to determine,as well as you can,the vertical asymptote.Check your conclusion by using the function to determine the vertical asymptote analytically.
Use the graph to determine,as well as you can,the vertical asymptote.Check your conclusion by using the function to determine the vertical asymptote analytically.
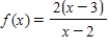
A) 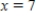
B) 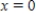
C) 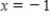
D) 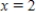
E) no vertical asymptote
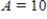 Use the graph to determine,as well as you can,the vertical asymptote.Check your conclusion by using the function to determine the vertical asymptote analytically.
Use the graph to determine,as well as you can,the vertical asymptote.Check your conclusion by using the function to determine the vertical asymptote analytically.
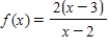

A)
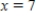
B)
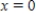
C)
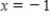
D)
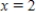
E) no vertical asymptote

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
73
Suppose the sales S (in billions of dollars per year)for Proctor & Gamble for the years 1997 through 2002 can be modeled by 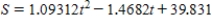 ,
,  where t represents the year.During which year were the sales increasing at the lowest rate?
where t represents the year.During which year were the sales increasing at the lowest rate?
A) Sales are increasing at the lowest rate in the year 2002.
B) Sales are increasing at the lowest rate in the year 1997.
C) Sales are increasing at the lowest rate in the year 1998.
D) Sales are increasing at the lowest rate in the year 2000.
E) Sales are increasing at the lowest rate in the year 1999.
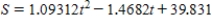 ,
,  where t represents the year.During which year were the sales increasing at the lowest rate?
where t represents the year.During which year were the sales increasing at the lowest rate? A) Sales are increasing at the lowest rate in the year 2002.
B) Sales are increasing at the lowest rate in the year 1997.
C) Sales are increasing at the lowest rate in the year 1998.
D) Sales are increasing at the lowest rate in the year 2000.
E) Sales are increasing at the lowest rate in the year 1999.

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
74
Analytically determine the location(s)of any vertical asymptote(s).
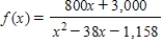
A) 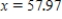
B) 
C) 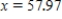 ,
, 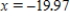
D) 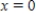
E) no vertical asymptotes
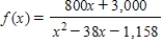
A)
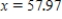
B)

C)
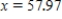 ,
, 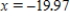
D)
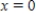
E) no vertical asymptotes

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
75
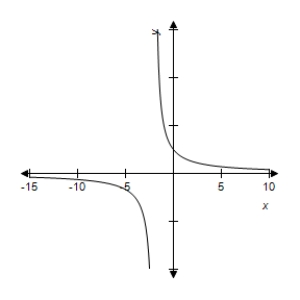
A function and its graph are given.Use the graph to find the horizontal asymptotes,if they exist.Confirm your results analytically. 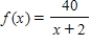
A) 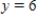
B) 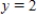
C) 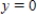
D) 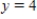
E) no horizontal asymptotes
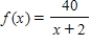

A)
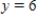
B)
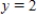
C)
D)
E) no horizontal asymptotes

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
76
Find any horizontal asymptotes for the given function. 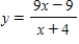
A)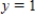
B)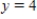
C)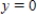
D)
E) no horizontal asymptotes
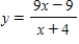
A)
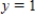
B)
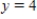
C)
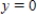
D)

E) no horizontal asymptotes

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
77
A firm can produce 100 units per week.If its total cost function is 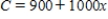 dollars,and its total revenue function is
dollars,and its total revenue function is  dollars,find the maximum profit.
dollars,find the maximum profit.
A) $3,679
B) $1,600
C) $7,031
D) $1,265
E) $1,941
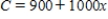 dollars,and its total revenue function is
dollars,and its total revenue function is  dollars,find the maximum profit.
dollars,find the maximum profit. A) $3,679
B) $1,600
C) $7,031
D) $1,265
E) $1,941

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
78
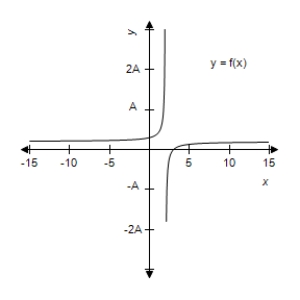
This problem contains a function and its graph,where 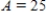 Use the graph to determine,as well as you can,the horizontal asymptote.Check your conclusion by using the function to determine the horizontal asymptote analytically.
Use the graph to determine,as well as you can,the horizontal asymptote.Check your conclusion by using the function to determine the horizontal asymptote analytically.

A) 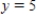
B) 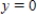
C) 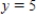
D) 
E) 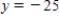
 Use the graph to determine,as well as you can,the horizontal asymptote.Check your conclusion by using the function to determine the horizontal asymptote analytically.
Use the graph to determine,as well as you can,the horizontal asymptote.Check your conclusion by using the function to determine the horizontal asymptote analytically.


A)
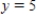
B)
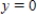
C)
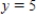
D)

E)
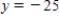

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
79
A function and its graph are given.Use the graph to find the vertical asymptotes,if they exist,where 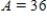 Confirm your results analytically.
Confirm your results analytically.

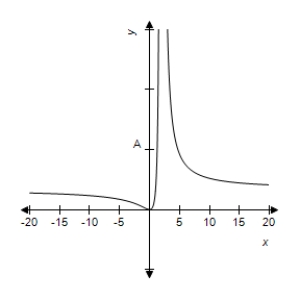
A) 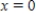
B) 
C) 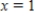
D) 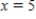
E) no vertical asymptotes
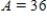 Confirm your results analytically.
Confirm your results analytically.


A)
B)

C)
D)
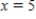
E) no vertical asymptotes

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck
80
A firm can produce 100 units per week.If its total cost function is 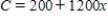 dollars,and its total revenue function is
dollars,and its total revenue function is 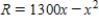 dollars,how many units x should it produce to maximize its profit?
dollars,how many units x should it produce to maximize its profit?
A) 1250 units
B) 650 units
C) 97 units
D) 50 units
E) 100 units
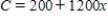 dollars,and its total revenue function is
dollars,and its total revenue function is 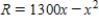 dollars,how many units x should it produce to maximize its profit?
dollars,how many units x should it produce to maximize its profit? A) 1250 units
B) 650 units
C) 97 units
D) 50 units
E) 100 units

Unlock Deck
Unlock for access to all 122 flashcards in this deck.
Unlock Deck
k this deck


