Deck 7: Extension B: Differential Equations
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/13
Play
Full screen (f)
Deck 7: Extension B: Differential Equations
1
Find the volume of a cap of a sphere with radius r = 100 and height h = 39 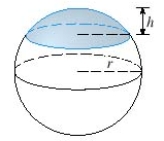

7857 π
2
Find the volume of a pyramid with height  and base an equilateral triangle with side a =
and base an equilateral triangle with side a = 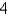

A)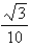
B)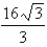
C)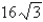
D)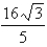
E)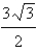
 and base an equilateral triangle with side a =
and base an equilateral triangle with side a = 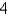

A)
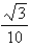
B)
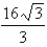
C)
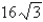
D)
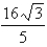
E)
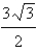
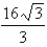
3
Find the volume of the solid obtained by rotating the region bounded by the given curves about the specified line. 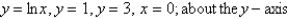
A)
B)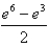
C)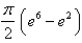
D)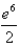
E) None
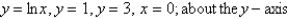
A)

B)
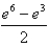
C)
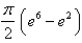
D)
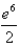
E) None
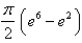
4
Find the volume of the solid obtained by rotating the region bounded by 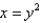 and
and 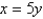 about the y-axis.
about the y-axis.
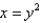 and
and 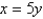 about the y-axis.
about the y-axis.
Unlock Deck
Unlock for access to all 13 flashcards in this deck.
Unlock Deck
k this deck
5
Find the volume of the solid obtained by rotating the region bounded by 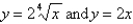 about the line
about the line 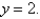
A)
B)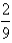
C)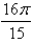
D)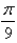
E)
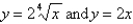 about the line
about the line 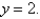
A)

B)
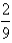
C)
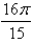
D)
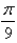
E)


Unlock Deck
Unlock for access to all 13 flashcards in this deck.
Unlock Deck
k this deck
6
Find the volume of the frustum of a pyramid with square base of side 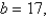 square top of side
square top of side 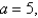 and height
and height 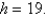
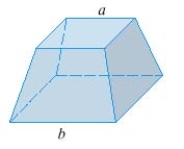
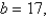 square top of side
square top of side 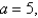 and height
and height 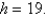


Unlock Deck
Unlock for access to all 13 flashcards in this deck.
Unlock Deck
k this deck
7
Use a computer algebra system to find the exact volume of the solid obtained by rotating the region bounded by the given curves about the specified line. 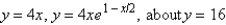
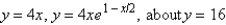

Unlock Deck
Unlock for access to all 13 flashcards in this deck.
Unlock Deck
k this deck
8
The volume of a solid torus (the donut-shaped solid shown in the figure)with r =  and R =
and R = 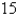 is
is 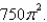
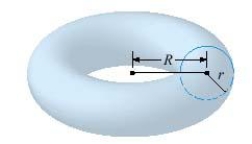
 and R =
and R = 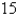 is
is 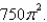


Unlock Deck
Unlock for access to all 13 flashcards in this deck.
Unlock Deck
k this deck
9
The base of S is the parabolic region 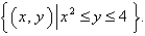 Cross-sections perpendicular to the y axis are squares. Find the volume of S.
Cross-sections perpendicular to the y axis are squares. Find the volume of S.
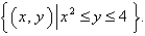 Cross-sections perpendicular to the y axis are squares. Find the volume of S.
Cross-sections perpendicular to the y axis are squares. Find the volume of S.
Unlock Deck
Unlock for access to all 13 flashcards in this deck.
Unlock Deck
k this deck
10
Find the volume common to two spheres,each with radius r = 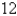 if the center of each sphere lies on the surface of the other sphere.
if the center of each sphere lies on the surface of the other sphere.
 if the center of each sphere lies on the surface of the other sphere.
if the center of each sphere lies on the surface of the other sphere.
Unlock Deck
Unlock for access to all 13 flashcards in this deck.
Unlock Deck
k this deck
11
Find the volume of the solid obtained by rotating the region bounded by 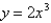 and
and 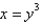 about the line
about the line 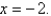
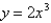 and
and 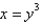 about the line
about the line 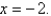

Unlock Deck
Unlock for access to all 13 flashcards in this deck.
Unlock Deck
k this deck
12
Find the volume of the solid obtained by rotating the region bounded by 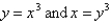 about the x-axis.
about the x-axis.
A)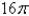
B)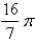
C)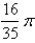
D)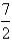
E)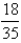
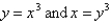 about the x-axis.
about the x-axis.A)
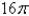
B)
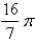
C)
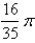
D)
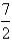
E)
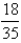

Unlock Deck
Unlock for access to all 13 flashcards in this deck.
Unlock Deck
k this deck
13
The base of S is a circular region with boundary curve 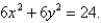 Cross-sections perpendicular to the x axis are isosceles right triangles with hypotenuse in the base. Find the volume of S.
Cross-sections perpendicular to the x axis are isosceles right triangles with hypotenuse in the base. Find the volume of S.
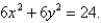 Cross-sections perpendicular to the x axis are isosceles right triangles with hypotenuse in the base. Find the volume of S.
Cross-sections perpendicular to the x axis are isosceles right triangles with hypotenuse in the base. Find the volume of S.
Unlock Deck
Unlock for access to all 13 flashcards in this deck.
Unlock Deck
k this deck


