Deck 13: Roots of Polynomial Equations
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/25
Play
Full screen (f)
Deck 13: Roots of Polynomial Equations
1
Express 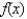 in the form
in the form 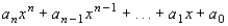 . Find a quadratic function that has a maximum value of 2 and that has - 2 and 4 as zeros.
. Find a quadratic function that has a maximum value of 2 and that has - 2 and 4 as zeros.
A)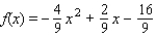
B)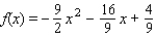
C)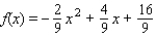
D)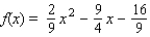
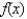 in the form
in the form 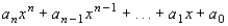 . Find a quadratic function that has a maximum value of 2 and that has - 2 and 4 as zeros.
. Find a quadratic function that has a maximum value of 2 and that has - 2 and 4 as zeros.A)
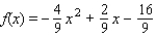
B)
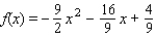
C)
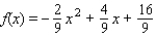
D)
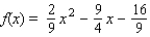
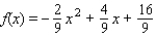
2
Determine the constants (denoted with capital letters) so that the equation is an identity. 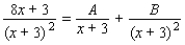
A)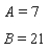
B)
C)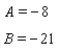
D)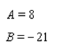
E)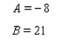
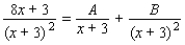
A)
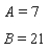
B)

C)
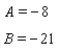
D)
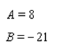
E)
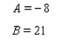
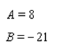
3
Find a quadratic equation with rational coefficients, one of whose roots is the given number. Write your answer so that the coefficient of  is 1.
is 1. 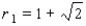
A)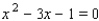
B)
C)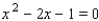
D)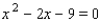
E)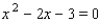
 is 1.
is 1. 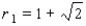
A)
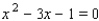
B)

C)
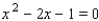
D)
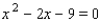
E)
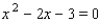
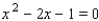
4
List the distinct roots of the following equation. For both repeated and single roots, specify their multiplicity. Enter 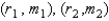 , ... where
, ... where 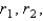 etc. are the roots of the polynomial and
etc. are the roots of the polynomial and 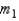 is the multiplicity of
is the multiplicity of 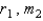 is the multiplicity of
is the multiplicity of 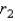 etc.
etc. 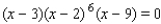
A) (3, 1), (2, 4), (9, 1)
B) (3, 1), (2, 1), (9, 1)
C) (3, 1), (2, 5), (9, 1)
D) (3, 1), (2, 6), (9, 1)
E) (3, 4), (2, 4), (9, 4)
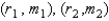 , ... where
, ... where 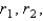 etc. are the roots of the polynomial and
etc. are the roots of the polynomial and 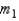 is the multiplicity of
is the multiplicity of 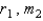 is the multiplicity of
is the multiplicity of 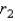 etc.
etc. 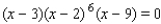
A) (3, 1), (2, 4), (9, 1)
B) (3, 1), (2, 1), (9, 1)
C) (3, 1), (2, 5), (9, 1)
D) (3, 1), (2, 6), (9, 1)
E) (3, 4), (2, 4), (9, 4)

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
5
Use long division to find the quotient and the remainder. 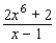
A)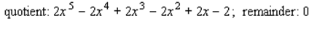
B)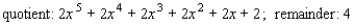
C)
D)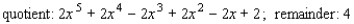
E)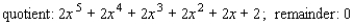
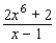
A)
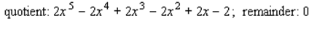
B)
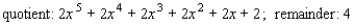
C)

D)
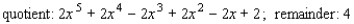
E)
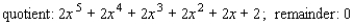

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
6
Find the rational roots and solve the equation. 4x 3 + x 2 - 20x - 5 = 0
A)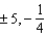
B)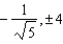
C)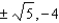
D)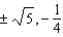
E)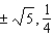
A)
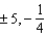
B)
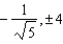
C)
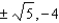
D)
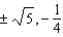
E)
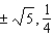

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
7
Use synthetic division to find the quotient and the remainder. 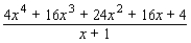
A)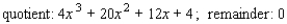
B)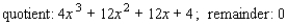
C)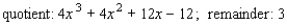
D)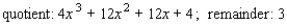
E)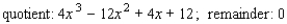
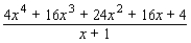
A)
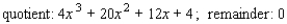
B)
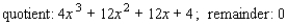
C)
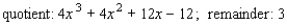
D)
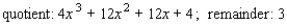
E)
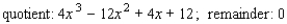

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
8
Determine the constants (denoted by capital letters) so that the equation is an identity. 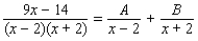
A)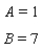
B)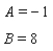
C)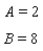
D)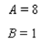
E)
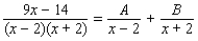
A)
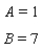
B)
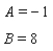
C)
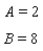
D)
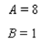
E)


Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
9
Use Descartes's rule of signs to obtain information regarding the roots of the equation. 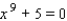
A) 1 negative root, 8 complex roots
B) 9 complex roots
C) 1 negative root, 9 complex roots
D) 1 positive root, 1 negative real root, 7 complex roots
E) 1 positive root, 8 complex roots
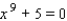
A) 1 negative root, 8 complex roots
B) 9 complex roots
C) 1 negative root, 9 complex roots
D) 1 positive root, 1 negative real root, 7 complex roots
E) 1 positive root, 8 complex roots

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
10
Find the rational roots and solve the equation. 3x 3 - 19x 2 + 21x - 5 = 0
A)
B)
C)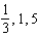
D)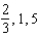
E)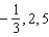
A)

B)

C)
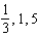
D)
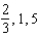
E)
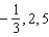

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
11
Use synthetic division to find the quotient and the remainder. 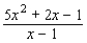
A)
B)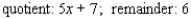
C)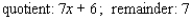
D)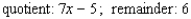
E)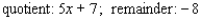
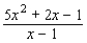
A)

B)
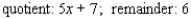
C)
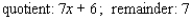
D)
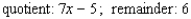
E)
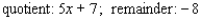

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
12
Use a graph to determine whether the equation has at least one real root. 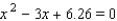
A) three real roots
B) two real roots
C) no real roots
D) one real root
E) It is not possible to make this determination by graphing the equation.
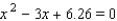
A) three real roots
B) two real roots
C) no real roots
D) one real root
E) It is not possible to make this determination by graphing the equation.

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
13
Use long division to find the quotient and the remainder. 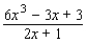
A)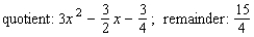
B)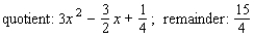
C)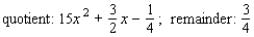
D)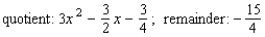
E)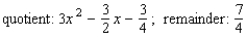
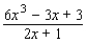
A)
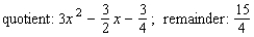
B)
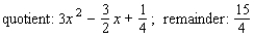
C)
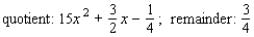
D)
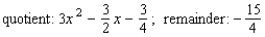
E)
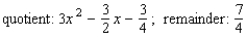

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
14
Find the rational roots and solve the equation. 4x 3 - 10x 2 - 25x + 4 = 0
A)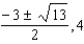
B)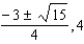
C)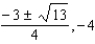
D)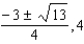
E)
A)
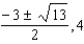
B)
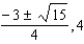
C)
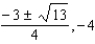
D)
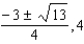
E)


Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
15
Determine which of the following real numbers is a root of the equation. 
A)
B)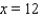
C)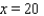
D)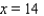
E)

A)

B)
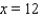
C)
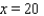
D)
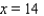
E)


Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
16
Determine which of the following real numbers is a root of the equation. 
A)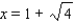
B)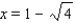
C)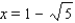
D)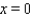
E)

A)
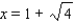
B)
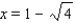
C)
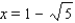
D)
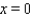
E)


Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
17
One root of the equation is given. Determine the remaining roots. 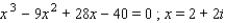
A)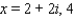
B)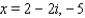
C)
D)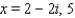
E)
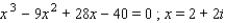
A)
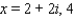
B)
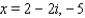
C)

D)
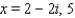
E)


Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
18
The equation has exactly one positive root. Locate the root between successive hundredths. x 3 - 3x 2 + 3x - 12 = 0
A) between 3.11 and 3.12
B) between 4.31 and 4.32
C) between 2.22 and 2.23
D) between 3.22 and 3.23
E) between 2.02 and 2.03
A) between 3.11 and 3.12
B) between 4.31 and 4.32
C) between 2.22 and 2.23
D) between 3.22 and 3.23
E) between 2.02 and 2.03

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
19
Use long division to find the quotient and the remainder. 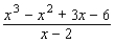
A)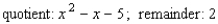
B)
C)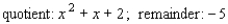
D)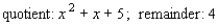
E)
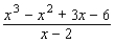
A)
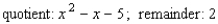
B)

C)
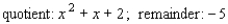
D)
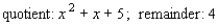
E)


Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
20
Determine upper and lower bounds for the real roots of the equation. 5x 4 - 10x - 12 = 0
A) 2 is an upper bound, - 1 is a lower bound
B) 8 is an upper bound, - 1 is a lower bound
C) 1 is an upper bound, - 2 is a lower bound
D) 2 is an upper bound, - 4 is a lower bound
E) 8 is an upper bound, - 4 is a lower bound
A) 2 is an upper bound, - 1 is a lower bound
B) 8 is an upper bound, - 1 is a lower bound
C) 1 is an upper bound, - 2 is a lower bound
D) 2 is an upper bound, - 4 is a lower bound
E) 8 is an upper bound, - 4 is a lower bound

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
21
Determine the constants (denoted by capital letters) so that the equation is an identity. 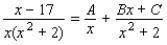
A)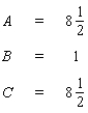
B)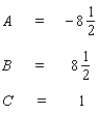
C)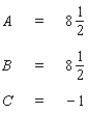
D)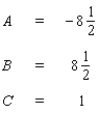
E)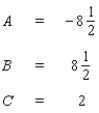
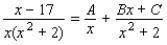
A)
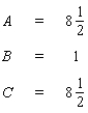
B)
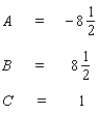
C)
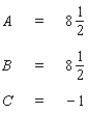
D)
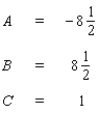
E)
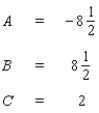

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
22
Factor the denominator of the given rational expression, and then find the partial fraction decomposition. 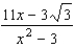
A)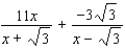
B)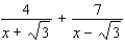
C)
D)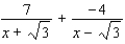
E)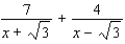
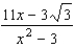
A)
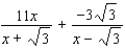
B)
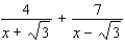
C)

D)
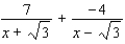
E)
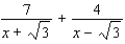

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
23
Factor the denominator of the given rational expression, and then find the partial fraction decomposition. 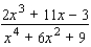
A)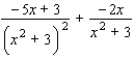
B)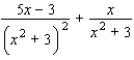
C)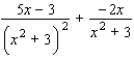
D)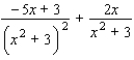
E)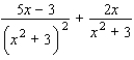
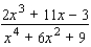
A)
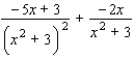
B)
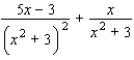
C)
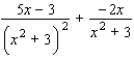
D)
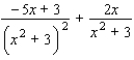
E)
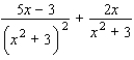

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
24
Determine which of the following polynomials is not reducible.
A)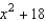
B)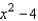
C)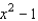
D)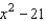
E)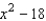
A)
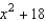
B)
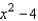
C)
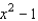
D)
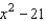
E)
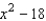

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
25
Factor the denominator of the given rational expression, and then find the partial fraction decomposition. 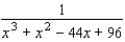
A)
B)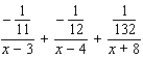
C)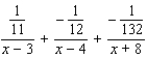
D)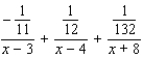
E)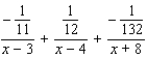
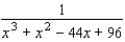
A)

B)
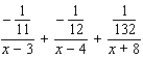
C)
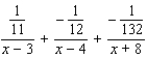
D)
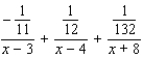
E)
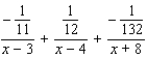

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck


