Deck 9: Sequences, Series, and Probability
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/25
Play
Full screen (f)
Deck 9: Sequences, Series, and Probability
1
Find the specified term of the arithmetic sequence that has the two given terms. 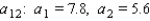
A)
B)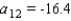
C)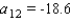
D)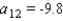
E)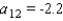
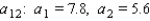
A)

B)
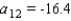
C)
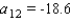
D)
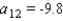
E)
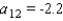
B
2
Express the sum in terms of n. 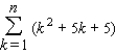 (Hint: Use the theorem on sums to write the sum as
(Hint: Use the theorem on sums to write the sum as 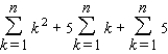 . Next, use the following formulas
. Next, use the following formulas 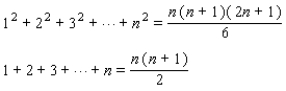 and the theorem on the sum of a constant.)
and the theorem on the sum of a constant.)
A)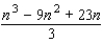
B)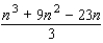
C)
D)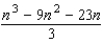
E)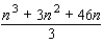
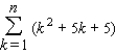 (Hint: Use the theorem on sums to write the sum as
(Hint: Use the theorem on sums to write the sum as 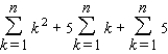 . Next, use the following formulas
. Next, use the following formulas 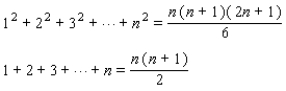 and the theorem on the sum of a constant.)
and the theorem on the sum of a constant.)A)
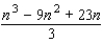
B)
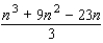
C)

D)
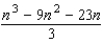
E)
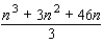
C
3
Find the total length of the red-line curve in the figure if the width of the maze formed by the curve is 48 inches and all halls in the maze have width 3 inches. 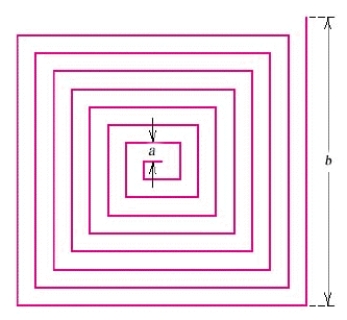
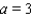 in. and
in. and 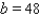 in.
in.
A) 825 inches
B) 816 inches
C) 810 inches
D) 822 inches
E) 918 inches

 in. and
in. and 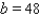 in.
in.A) 825 inches
B) 816 inches
C) 810 inches
D) 822 inches
E) 918 inches
B
4
Bode's sequence, defined by 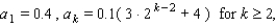 can be used to approximate distances of planets from the sun. These distances are measured in astronomical units, with 1 AU = 93,000,000 mi. For example, the third term corresponds to earth and the fifth term to the minor planet Ceres. Find the 5th term of the sequence.
can be used to approximate distances of planets from the sun. These distances are measured in astronomical units, with 1 AU = 93,000,000 mi. For example, the third term corresponds to earth and the fifth term to the minor planet Ceres. Find the 5th term of the sequence.
A)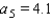
B)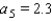
C)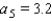
D)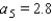
E)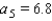
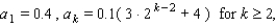 can be used to approximate distances of planets from the sun. These distances are measured in astronomical units, with 1 AU = 93,000,000 mi. For example, the third term corresponds to earth and the fifth term to the minor planet Ceres. Find the 5th term of the sequence.
can be used to approximate distances of planets from the sun. These distances are measured in astronomical units, with 1 AU = 93,000,000 mi. For example, the third term corresponds to earth and the fifth term to the minor planet Ceres. Find the 5th term of the sequence.A)
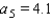
B)
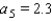
C)
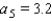
D)
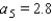
E)
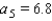

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
5
Without expanding completely, find the middle term in the expansion of the expression. 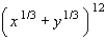
A)
B)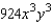
C)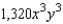
D)
E)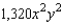
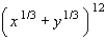
A)

B)
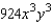
C)
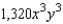
D)

E)
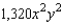

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
6
Find the smallest positive integer j for which the statement is true. 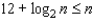
A) 15
B) 16
C) 12
D) 19
E) 256
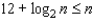
A) 15
B) 16
C) 12
D) 19
E) 256

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
7
In how many different ways can a test consisting of five true-or-false questions be completed?
A) 10
B) 25
C) 7
D) 32
E) 64
A) 10
B) 25
C) 7
D) 32
E) 64

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
8
A pile of logs has 38 logs in the bottom layer, 37 in the second layer, 36 in the third, and so on. The top layer contains 28 logs. Find the total number of logs in the pile.
A) 363 logs
B) 361 logs
C) 726 logs
D) 359 logs
E) 365 logs
A) 363 logs
B) 361 logs
C) 726 logs
D) 359 logs
E) 365 logs

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
9
Find the sum. 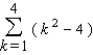
A)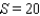
B)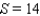
C)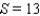
D)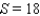
E)
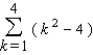
A)
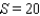
B)
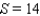
C)
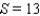
D)
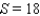
E)


Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
10
If ten basketball teams are in a tournament, find the number of different ways that first, second, and third place can be decided, assuming ties are not allowed.
A) 720
B) 90
C) 6
D) 7,920
E) 5,040
A) 720
B) 90
C) 6
D) 7,920
E) 5,040

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
11
Find the fourth term of the recursively defined infinite sequence. 
A)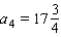
B)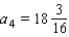
C)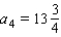
D)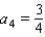
E)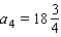

A)
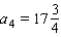
B)
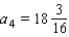
C)
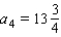
D)
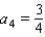
E)
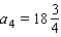

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
12
A bicycle rider coasts downhill, traveling 7 feet the first second. In each succeeding second, the rider travels 5 feet farther than in the preceding second. If the rider reaches the bottom of the hill in 10 seconds, find the total distance traveled.
A) 275 feet
B) 300 feet
C) 290 feet
D) 295 feet
E) 310 feet
A) 275 feet
B) 300 feet
C) 290 feet
D) 295 feet
E) 310 feet

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
13
A girl has three skirts, ten blouses and five sweaters. How many different skirt-blouse-sweater combinations can she wear?
A) 160
B) 18
C) 120
D) 146
E) 150
A) 160
B) 18
C) 120
D) 146
E) 150

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
14
Use the principle of mathematical induction to find the equivalent expression for every positive integer n. 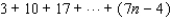
A)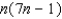
B)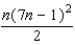
C)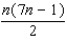
D)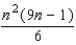
E)
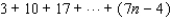
A)
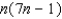
B)
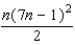
C)
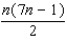
D)
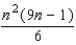
E)


Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
15
Find the nth term and the fifth term of the geometric sequence. 
A)
B)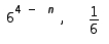
C)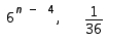
D)
E)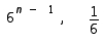

A)

B)
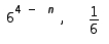
C)
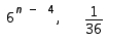
D)

E)
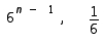

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
16
Use the principle of mathematical induction to find the equivalent expression for every positive integer n. 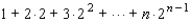
A)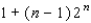
B)
C)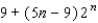
D)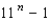
E)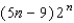
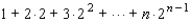
A)
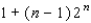
B)

C)
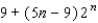
D)
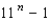
E)
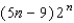

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
17
A contest will have five cash prizes totaling $13,000, and there will be a $500 difference between successive prizes. Find the first prize.
A) $4,100
B) $3,100
C) $3,600
D) $5,100
E) $6,100
A) $4,100
B) $3,100
C) $3,600
D) $5,100
E) $6,100

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
18
Find the number of terms in the arithmetic sequence with the given conditions. 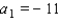 ,
, 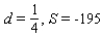
A)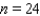
B)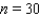
C)
D)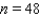
E)
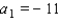 ,
, 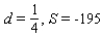
A)
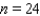
B)
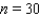
C)

D)
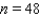
E)


Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
19
Evaluate the expression. 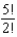
A) 24
B) 360
C) 120
D) 60
E) 121
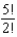
A) 24
B) 360
C) 120
D) 60
E) 121

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
20
Find the number. 
A)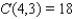
B)
C)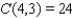
D)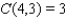
E)

A)
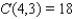
B)

C)
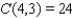
D)
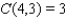
E)


Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
21
A committee of 3 men and 2 women is to be chosen from a group of 11 men and 9 women. Determine the number of different ways of selecting the committee.
A) 5,940
B) 3
C) 35,640
D) 165
A) 5,940
B) 3
C) 35,640
D) 165

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
22
To win a state lottery game, a player must correctly select six numbers from the numbers 1 through 49. Find the total number of selections possible if a player selects only even numbers.
A) 96,909,120
B) 60,410,085,120
C) 134,596
D) 6,991,908
E) 144
A) 96,909,120
B) 60,410,085,120
C) 134,596
D) 6,991,908
E) 144

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
23
During 1990, smoking caused 418,890 deaths in the United States. Of these deaths, cardiovascular disease accounted for 179,820, cancer for 151,322, and respiratory diseases such as emphysema for 84,475. Find the probability that a smoking-related death was the result of either cancer or cardiovascular disease.
A) 0.813
B) 0.209
C) 0.791
D) 0.803
E) 0.786
A) 0.813
B) 0.209
C) 0.791
D) 0.803
E) 0.786

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
24
A committee is going to select 26 students from a pool of 800 to receive scholarships. How may ways could the students be selected if each scholarship is worth the same amount?
A)
B)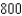
C)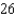
D)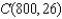
E)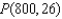
A)

B)
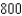
C)
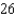
D)
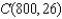
E)
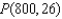

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck
25
A man is 52 years old and a woman is 34 years old. The probability that the man will be alive in 10 years is 0.78, whereas the probability that the woman will be alive 10 years from now is 0.88. Assume that their life expectancies are unrelated. a) Find the probability that they will both be alive 10 years from now.
B) Find the probability that neither one will be alive 10 years from now.
C) Find the probability that at least one of the two will be alive 10 years from now.
A)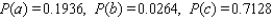
B)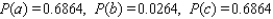
C)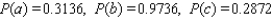
D)
E)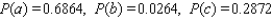
B) Find the probability that neither one will be alive 10 years from now.
C) Find the probability that at least one of the two will be alive 10 years from now.
A)
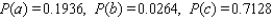
B)
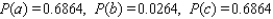
C)
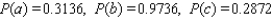
D)

E)
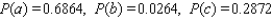

Unlock Deck
Unlock for access to all 25 flashcards in this deck.
Unlock Deck
k this deck


