Deck 5: Applications of Integration
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/120
Play
Full screen (f)
Deck 5: Applications of Integration
1
Find the integral. 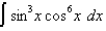
A)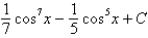
B)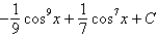
C)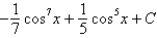
D)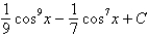
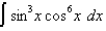
A)
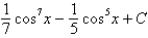
B)
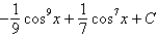
C)
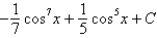
D)
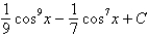
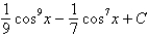
2
Find the integral. 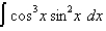
A)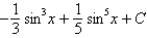
B)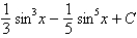
C)
D)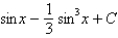
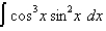
A)
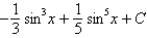
B)
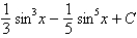
C)

D)
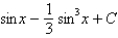
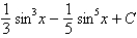
3
Find the integral. 
A)
B)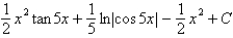
C)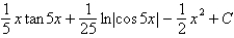
D)

A)

B)
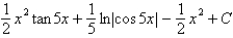
C)
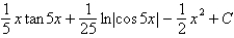
D)

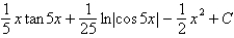
4
Find the integral using the indicated substitution.  ,
, 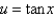
A)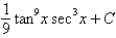
B)
C)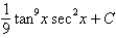
D)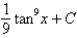
 ,
, 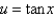
A)
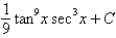
B)

C)
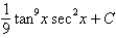
D)
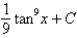

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
5
Find the average value of the function 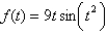 on the interval
on the interval 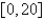 . Round your answer to 3 decimal places.
. Round your answer to 3 decimal places.
A)0.45
B)9.342
C)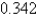
D)0.432
E)18
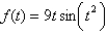 on the interval
on the interval 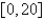 . Round your answer to 3 decimal places.
. Round your answer to 3 decimal places.A)0.45
B)9.342
C)
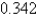
D)0.432
E)18

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
6
Evaluate the integral. 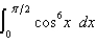
A)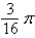
B)
C)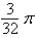
D)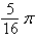
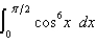
A)
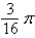
B)

C)
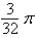
D)
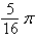

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
7
Find the integral using the indicated substitution. 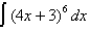 ,
, 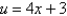
A)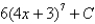
B)
C)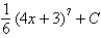
D)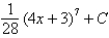
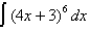 ,
, 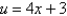
A)
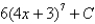
B)

C)
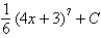
D)
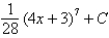

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
8
Find the integral using an appropriate trigonometric substitution. 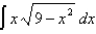
A)
B)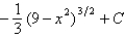
C)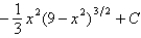
D)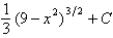
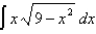
A)

B)
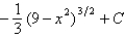
C)
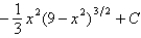
D)
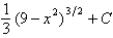

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
9
Find the integral. 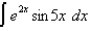
A)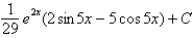
B)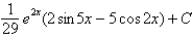
C)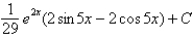
D)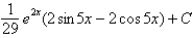
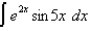
A)
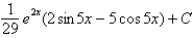
B)
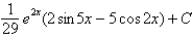
C)
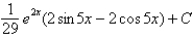
D)
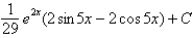

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
10
Find the integral. 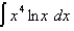
A)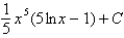
B)
C)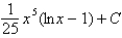
D)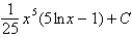
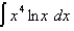
A)
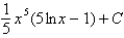
B)

C)
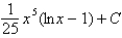
D)
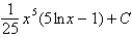

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
11
The temperature of a metal rod, 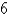 m long, is
m long, is 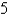 x (in degree Celsius) at a distance x meters from one end of the rod. What is the average temperature of the rod?
x (in degree Celsius) at a distance x meters from one end of the rod. What is the average temperature of the rod?
A)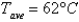
B)
C)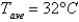
D)
E)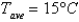
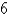 m long, is
m long, is 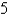 x (in degree Celsius) at a distance x meters from one end of the rod. What is the average temperature of the rod?
x (in degree Celsius) at a distance x meters from one end of the rod. What is the average temperature of the rod?A)
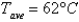
B)

C)
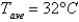
D)

E)
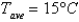

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
12
Find the integral. 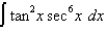
A)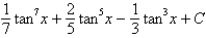
B)
C)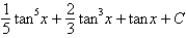
D)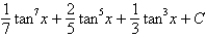
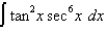
A)
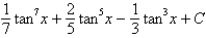
B)

C)
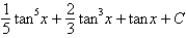
D)
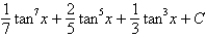

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
13
Find the indefinite integral 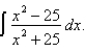
A)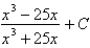
B)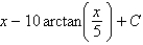
C)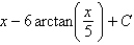
D)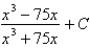
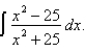
A)
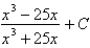
B)
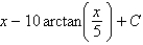
C)
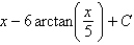
D)
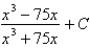

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
14
Find the indefinite integral. 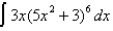
A)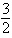
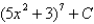
B)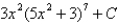
C)
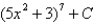
D)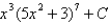
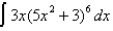
A)
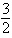
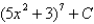
B)
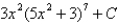
C)

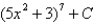
D)
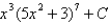

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
15
A steel girder weighing 200 lb is hoisted from ground level to the roof of a 40-ft building using a chain that weighs 5 lb/running foot. Find the work done.
A)4,000 ft-lb
B)8,100 ft-lb
C)12,000 ft-lb
D)8,000 ft-lb
A)4,000 ft-lb
B)8,100 ft-lb
C)12,000 ft-lb
D)8,000 ft-lb

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
16
Find the integral. 
A)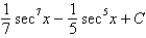
B)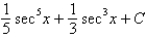
C)
D)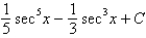

A)
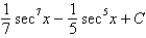
B)
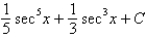
C)

D)
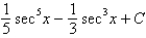

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
17
Find the indefinite integral 
A)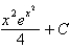
B)
C)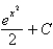
D)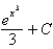

A)
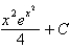
B)

C)
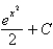
D)
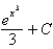

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
18
Find the integral using an appropriate trigonometric substitution. 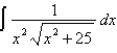
A)
B)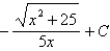
C)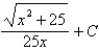
D)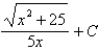
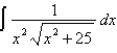
A)

B)
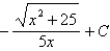
C)
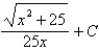
D)
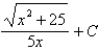

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
19
Find the indefinite integral. 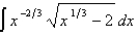
A)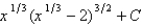
B)
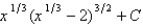
C)
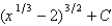
D)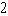

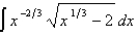
A)
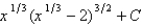
B)
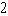
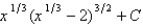
C)

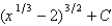
D)
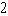


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
20
Find the indefinite integral. 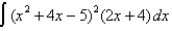
A)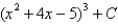
B)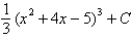
C)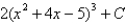
D)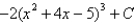
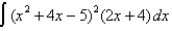
A)
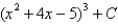
B)
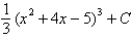
C)
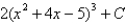
D)
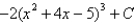

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
21
Find the number(s) a such that the average value of the function 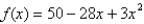 on the interval
on the interval 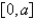 is equal to 10.
is equal to 10.
A)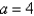
B)
C)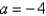
D)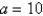
E)
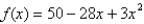 on the interval
on the interval 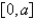 is equal to 10.
is equal to 10. A)
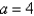
B)

C)
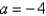
D)
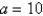
E)


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
22
Find the average value of the function 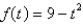 on the interval
on the interval  .
.
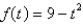 on the interval
on the interval  .
.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
23
Find the integral. 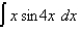
A)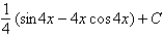
B)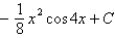
C)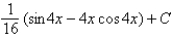
D)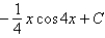
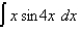
A)
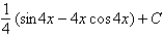
B)
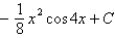
C)
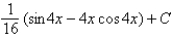
D)
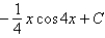

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
24
A tank is full of water. Find the work required to pump the water out of the outlet. 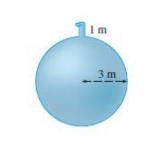
A)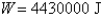
B)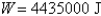
C)
D)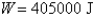
E)None of these

A)
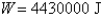
B)
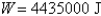
C)

D)
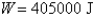
E)None of these

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
25
If 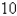 J of work are needed to stretch a spring from
J of work are needed to stretch a spring from 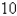 cm to
cm to 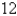 cm and another
cm and another 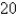 J are needed to stretch it from
J are needed to stretch it from 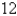 cm to
cm to  cm, what is the natural length of the spring? Round the answer to nearest integer.
cm, what is the natural length of the spring? Round the answer to nearest integer.
A)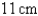
B)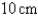
C)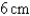
D)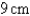
E)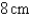
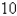 J of work are needed to stretch a spring from
J of work are needed to stretch a spring from 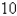 cm to
cm to 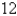 cm and another
cm and another 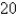 J are needed to stretch it from
J are needed to stretch it from 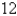 cm to
cm to  cm, what is the natural length of the spring? Round the answer to nearest integer.
cm, what is the natural length of the spring? Round the answer to nearest integer.A)
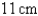
B)
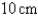
C)
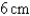
D)
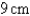
E)
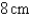

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
26
Find the average value of the function 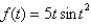 on the interval
on the interval 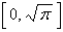 .
.
A)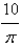
B)
C)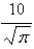
D)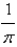
E)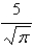
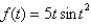 on the interval
on the interval 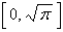 .
.A)
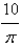
B)

C)
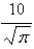
D)
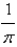
E)
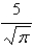

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
27
Evaluate the integral using an appropriate trigonometric substitution. 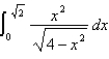
A)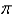
B)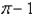
C)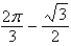
D)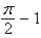
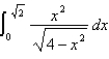
A)
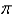
B)
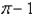
C)
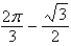
D)
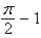

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
28
Find the work done in pushing a car a distance of 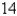 m while exerting a constant force of
m while exerting a constant force of  N.
N.
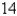 m while exerting a constant force of
m while exerting a constant force of  N.
N.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
29
The velocity v of blood that flows in a blood vessel with radius  and length l at a distance
and length l at a distance 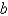 from the central axis is
from the central axis is 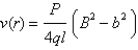 where P is the pressure difference between the ends of the vessel and q is the viscosity of the blood. Find the average velocity (with respect to r) over the interval
where P is the pressure difference between the ends of the vessel and q is the viscosity of the blood. Find the average velocity (with respect to r) over the interval 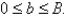
 and length l at a distance
and length l at a distance 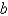 from the central axis is
from the central axis is 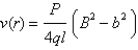 where P is the pressure difference between the ends of the vessel and q is the viscosity of the blood. Find the average velocity (with respect to r) over the interval
where P is the pressure difference between the ends of the vessel and q is the viscosity of the blood. Find the average velocity (with respect to r) over the interval 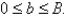

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
30
A heavy rope, 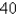 ft long, weighs
ft long, weighs 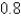 lb/ft and hangs over the edge of a building 110 ft high. How much work is done in pulling the rope to the top of the building?
lb/ft and hangs over the edge of a building 110 ft high. How much work is done in pulling the rope to the top of the building?
A)
B)
C)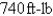
D)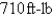
E)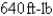
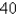 ft long, weighs
ft long, weighs 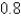 lb/ft and hangs over the edge of a building 110 ft high. How much work is done in pulling the rope to the top of the building?
lb/ft and hangs over the edge of a building 110 ft high. How much work is done in pulling the rope to the top of the building?A)

B)

C)
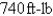
D)
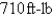
E)
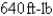

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
31
Find the integral using an appropriate trigonometric substitution. 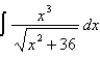
A)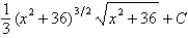
B)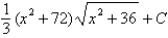
C)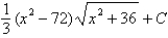
D)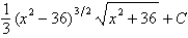
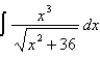
A)
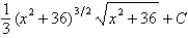
B)
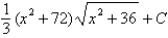
C)
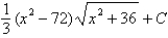
D)
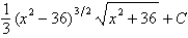

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
32
A force of 30 N is required to maintain a spring stretched from its natural length of 12 cm to a length of 15 cm. How much work is done in stretching the spring from  cm to
cm to 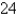 cm?
cm?
A)
B)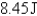
C)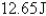
D)
E)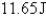
 cm to
cm to 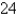 cm?
cm?A)

B)
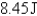
C)
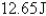
D)

E)
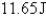

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
33
Find the average value of the function 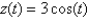 on the interval
on the interval  .
.
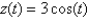 on the interval
on the interval  .
.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
34
The linear density of a 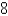 m long rod is
m long rod is 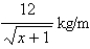 where x is measured in meters from one end of the rod. Find the average density of the rod.
where x is measured in meters from one end of the rod. Find the average density of the rod.
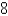 m long rod is
m long rod is 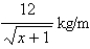 where x is measured in meters from one end of the rod. Find the average density of the rod.
where x is measured in meters from one end of the rod. Find the average density of the rod.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
35
Find the integral. 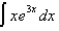
A)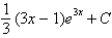
B)
C)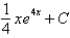
D)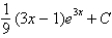
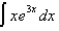
A)
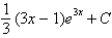
B)

C)
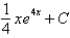
D)
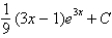

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
36
Find the integral using an appropriate trigonometric substitution. 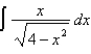
A)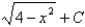
B)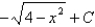
C)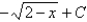
D)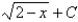
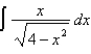
A)
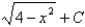
B)
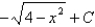
C)
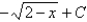
D)
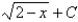

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
37
Find the indefinite integral. 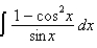
A)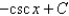
B)
C)
D)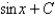
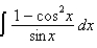
A)
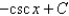
B)

C)

D)
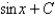

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
38
Find the indefinite integral. 
A)
B)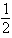
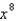

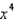 + 8x + C
+ 8x + C
C)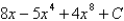
D)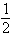
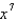
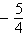
 + 8x + C
+ 8x + C

A)

B)
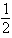
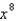

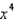 + 8x + C
+ 8x + CC)
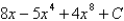
D)
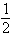
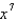
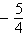
 + 8x + C
+ 8x + C
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
39
Find the indefinite integral. 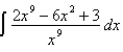
A)2x +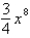

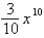 + C
+ C
B)2x +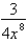

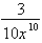 + C
+ C
C)2x +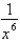

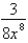 + C
+ C
D)2x +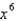

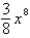 + C
+ C
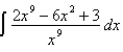
A)2x +
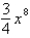

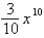 + C
+ CB)2x +
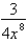

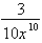 + C
+ CC)2x +
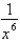

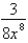 + C
+ CD)2x +
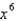

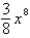 + C
+ C
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
40
In a certain city the temperature  hours after 7 A.M. was modeled by the function
hours after 7 A.M. was modeled by the function 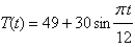 Find the average temperature to three decimal places during the period from 7 A.M. to 7 P.M.
Find the average temperature to three decimal places during the period from 7 A.M. to 7 P.M.
 hours after 7 A.M. was modeled by the function
hours after 7 A.M. was modeled by the function 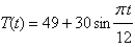 Find the average temperature to three decimal places during the period from 7 A.M. to 7 P.M.
Find the average temperature to three decimal places during the period from 7 A.M. to 7 P.M.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
41
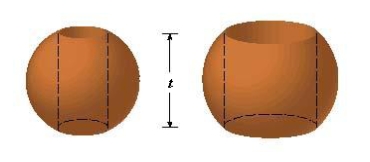
Suppose you make napkin rings by drilling holes with different diameters through two wooden balls (which also have different diameters). You discover that both napkin rings have the same height h as shown in the figure. Use cylindrical shells to compute the volume of a napkin ring created by drilling a hole with radius d through the center of a sphere of radius D and express the answer in terms of 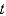 .
. 
A)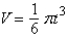
B)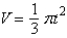
C)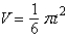
D)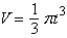
E)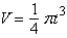
 .
. 
A)
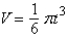
B)
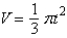
C)
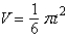
D)
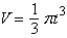
E)
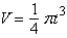

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
42
Find the derivative of the function 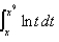
A)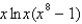
B)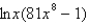
C)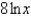
D)
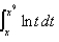
A)
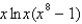
B)
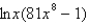
C)
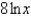
D)


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
43
A tank has the shape of an inverted right circular cone with a base radius of 4 m and a height of 9 m. If the tank is filled to a height of 3 m, find the work required (to the nearest joule) to empty the tank by pumping the water over the top of the tank. (The mass of water is 1000 kg/m3 and the force of gravity is 9.8 m/sec2.)

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
44
In a steam engine the pressure and volume of steam satisfy the equation  , where k is a constant. (This is true for adiabatic expansion, that is, expansion in which there is no heat transfer between the cylinder and its surroundings.) Calculate the work done by the engine (in ft-lb) during a cycle when the steam starts at a pressure of
, where k is a constant. (This is true for adiabatic expansion, that is, expansion in which there is no heat transfer between the cylinder and its surroundings.) Calculate the work done by the engine (in ft-lb) during a cycle when the steam starts at a pressure of  and a volume of
and a volume of  and expands to a volume of
and expands to a volume of 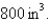 Use the fact that the work done by the gas when the volume expands from
Use the fact that the work done by the gas when the volume expands from 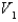 to volume
to volume 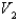 is
is 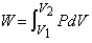 .
.
 , where k is a constant. (This is true for adiabatic expansion, that is, expansion in which there is no heat transfer between the cylinder and its surroundings.) Calculate the work done by the engine (in ft-lb) during a cycle when the steam starts at a pressure of
, where k is a constant. (This is true for adiabatic expansion, that is, expansion in which there is no heat transfer between the cylinder and its surroundings.) Calculate the work done by the engine (in ft-lb) during a cycle when the steam starts at a pressure of  and a volume of
and a volume of  and expands to a volume of
and expands to a volume of 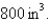 Use the fact that the work done by the gas when the volume expands from
Use the fact that the work done by the gas when the volume expands from 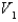 to volume
to volume 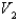 is
is 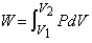 .
.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
45
An aquarium 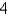 m long,
m long, 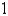 m wide, and 1 m deep is full of water. Find the work (in J) needed to pump half of the water out of the aquarium. (Use the facts that the density of water is
m wide, and 1 m deep is full of water. Find the work (in J) needed to pump half of the water out of the aquarium. (Use the facts that the density of water is  and
and 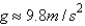 )
)
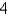 m long,
m long, 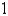 m wide, and 1 m deep is full of water. Find the work (in J) needed to pump half of the water out of the aquarium. (Use the facts that the density of water is
m wide, and 1 m deep is full of water. Find the work (in J) needed to pump half of the water out of the aquarium. (Use the facts that the density of water is  and
and 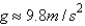 )
)
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
46
Use cylindrical shells to find the volume of the solid. A sphere of radius 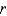 .
.
A)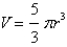
B)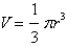
C)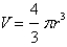
D)
E)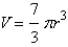
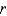 .
.A)
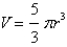
B)
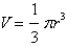
C)
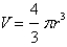
D)

E)
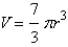

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
47
Use the method of cylindrical shells to find the volume of the solid generated by revolving the region about the indicated axis or line. 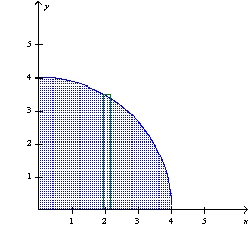 y-axis
y-axis
A)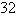
B)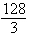
C)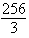
D)
 y-axis
y-axisA)
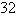
B)
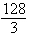
C)
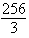
D)


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
48
Find the derivative of the function. 
A)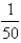
B)
C)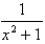
D)

A)
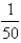
B)

C)
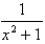
D)


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
49
Evaluate the limit by interpreting it as the limit of a Riemann sum of a function on the interval [a, b]. ![<strong>Evaluate the limit by interpreting it as the limit of a Riemann sum of a function on the interval [a, b]. </strong> A) B)- C)- D)](https://storage.examlex.com/TB5971/11eaa3e5_5581_80a5_9f8f_b36881526aab_TB5971_11.jpg)
A)![<strong>Evaluate the limit by interpreting it as the limit of a Riemann sum of a function on the interval [a, b]. </strong> A) B)- C)- D)](https://storage.examlex.com/TB5971/11eaa3e5_5581_80a6_9f8f_0bbccb3abfac_TB5971_11.jpg)
B)-![<strong>Evaluate the limit by interpreting it as the limit of a Riemann sum of a function on the interval [a, b]. </strong> A) B)- C)- D)](https://storage.examlex.com/TB5971/11eaa3e5_5581_a7b7_9f8f_a5c254928ff6_TB5971_11.jpg)
C)-![<strong>Evaluate the limit by interpreting it as the limit of a Riemann sum of a function on the interval [a, b]. </strong> A) B)- C)- D)](https://storage.examlex.com/TB5971/11eaa3e5_5581_a7b8_9f8f_8bf58e50132e_TB5971_11.jpg)
D)![<strong>Evaluate the limit by interpreting it as the limit of a Riemann sum of a function on the interval [a, b]. </strong> A) B)- C)- D)](https://storage.examlex.com/TB5971/11eaa3e5_5581_cec9_9f8f_c9e9405a2566_TB5971_11.jpg)
![<strong>Evaluate the limit by interpreting it as the limit of a Riemann sum of a function on the interval [a, b]. </strong> A) B)- C)- D)](https://storage.examlex.com/TB5971/11eaa3e5_5581_80a5_9f8f_b36881526aab_TB5971_11.jpg)
A)
![<strong>Evaluate the limit by interpreting it as the limit of a Riemann sum of a function on the interval [a, b]. </strong> A) B)- C)- D)](https://storage.examlex.com/TB5971/11eaa3e5_5581_80a6_9f8f_0bbccb3abfac_TB5971_11.jpg)
B)-
![<strong>Evaluate the limit by interpreting it as the limit of a Riemann sum of a function on the interval [a, b]. </strong> A) B)- C)- D)](https://storage.examlex.com/TB5971/11eaa3e5_5581_a7b7_9f8f_a5c254928ff6_TB5971_11.jpg)
C)-
![<strong>Evaluate the limit by interpreting it as the limit of a Riemann sum of a function on the interval [a, b]. </strong> A) B)- C)- D)](https://storage.examlex.com/TB5971/11eaa3e5_5581_a7b8_9f8f_8bf58e50132e_TB5971_11.jpg)
D)
![<strong>Evaluate the limit by interpreting it as the limit of a Riemann sum of a function on the interval [a, b]. </strong> A) B)- C)- D)](https://storage.examlex.com/TB5971/11eaa3e5_5581_cec9_9f8f_c9e9405a2566_TB5971_11.jpg)

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
50
If 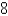 J of work are needed to stretch a spring from 8 cm to 14 cm and another
J of work are needed to stretch a spring from 8 cm to 14 cm and another 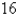 J are needed to stretch it from 14 cm to 19 cm, what is the natural length of the spring?
J are needed to stretch it from 14 cm to 19 cm, what is the natural length of the spring?
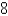 J of work are needed to stretch a spring from 8 cm to 14 cm and another
J of work are needed to stretch a spring from 8 cm to 14 cm and another 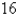 J are needed to stretch it from 14 cm to 19 cm, what is the natural length of the spring?
J are needed to stretch it from 14 cm to 19 cm, what is the natural length of the spring?
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
51
Find the volume of the solid generated by revolving the region bounded by the graphs of the equations about the indicated line. y = 1 - x2, y = 0; the line y = 4
A)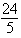
B)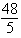
C)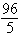
D)
A)
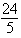
B)
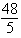
C)
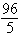
D)


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
52
The tank shown is full of water. Given that water weighs 62.5 lb/ft and R = 5, find the work (in lb-ft) required to pump the water out of the tank. 


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
53
Evaluate the integral 
A)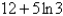
B)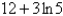
C)
D)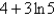

A)
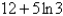
B)
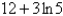
C)

D)
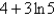

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
54
Evaluate the integral 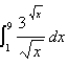
A)
B)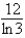
C)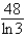
D)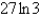
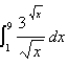
A)

B)
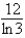
C)
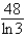
D)
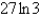

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
55
Use the method of cylindrical shells to find the volume of solid obtained by rotating the region bounded by the given curves about the x-axis. 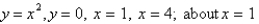
A)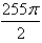
B)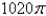
C)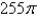
D)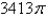
E)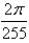
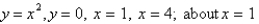
A)
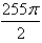
B)
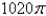
C)
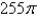
D)
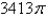
E)
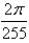

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
56
A chain weighing 3 lb/ft hangs vertically from a winch located 13 ft above the ground, and the free end of the chain is just touching the ground. Find the work done by the winch in pulling in the whole chain.

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
57
Evaluate the integral 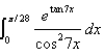
A)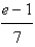
B)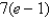
C)
D)
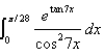
A)
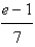
B)
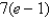
C)

D)


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
58
Find the volume of the solid generated by revolving the region bounded by the graphs of the equations about the x-axis. y = sin x + 1, x = 0, y = 0, x =
A)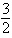 2 +2
2 +2
B) 2+2
C)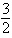 2+4
2+4
D) 2+4
A)
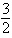 2 +2
2 +2 B) 2+2
C)
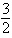 2+4
2+4 D) 2+4

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
59
Find the volume of the solid generated by revolving the region bounded by the graphs of the equations and inequalities about the y-axis. x2 - y2 = 36, x 0, y = -6, y = 6
A) 432
B) 288
C) 864
D) 576
A) 432
B) 288
C) 864
D) 576

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
60
Evaluate the integral. 
A)14
B)10
C)-11
D)-14

A)14
B)10
C)-11
D)-14

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
61
Find the volume of a pyramid with height  and base an equilateral triangle with side a =
and base an equilateral triangle with side a = 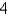 .
. 
A)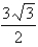
B)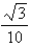
C)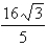
D)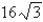
E)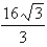
 and base an equilateral triangle with side a =
and base an equilateral triangle with side a = 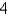 .
. 
A)
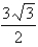
B)
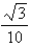
C)
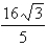
D)
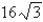
E)
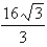

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
62
The Mean Value Theorem for Integrals says that if ![The Mean Value Theorem for Integrals says that if is continuous on [ , ], then there exists a number m in [ , ] such that .](https://storage.examlex.com/TB5971/11eaa3e5_5586_3bd6_9f8f_f5d3b3ce71e4_TB5971_11.jpg) is continuous on [
is continuous on [ ![The Mean Value Theorem for Integrals says that if is continuous on [ , ], then there exists a number m in [ , ] such that .](https://storage.examlex.com/TB5971/11eaa3e5_5586_3bd7_9f8f_fd1ad04291eb_TB5971_11.jpg) ,
, ![The Mean Value Theorem for Integrals says that if is continuous on [ , ], then there exists a number m in [ , ] such that .](https://storage.examlex.com/TB5971/11eaa3e5_5586_3bd8_9f8f_6f5aff8dadbe_TB5971_11.jpg) ], then there exists a number m in [
], then there exists a number m in [ ![The Mean Value Theorem for Integrals says that if is continuous on [ , ], then there exists a number m in [ , ] such that .](https://storage.examlex.com/TB5971/11eaa3e5_5586_3bd9_9f8f_6b10138f1849_TB5971_11.jpg) ,
, ![The Mean Value Theorem for Integrals says that if is continuous on [ , ], then there exists a number m in [ , ] such that .](https://storage.examlex.com/TB5971/11eaa3e5_5586_62ea_9f8f_41f9c2e03dc4_TB5971_11.jpg) ] such that
] such that ![The Mean Value Theorem for Integrals says that if is continuous on [ , ], then there exists a number m in [ , ] such that .](https://storage.examlex.com/TB5971/11eaa3e5_5586_62eb_9f8f_095a590db5af_TB5971_11.jpg) .
.
![The Mean Value Theorem for Integrals says that if is continuous on [ , ], then there exists a number m in [ , ] such that .](https://storage.examlex.com/TB5971/11eaa3e5_5586_3bd6_9f8f_f5d3b3ce71e4_TB5971_11.jpg) is continuous on [
is continuous on [ ![The Mean Value Theorem for Integrals says that if is continuous on [ , ], then there exists a number m in [ , ] such that .](https://storage.examlex.com/TB5971/11eaa3e5_5586_3bd7_9f8f_fd1ad04291eb_TB5971_11.jpg) ,
, ![The Mean Value Theorem for Integrals says that if is continuous on [ , ], then there exists a number m in [ , ] such that .](https://storage.examlex.com/TB5971/11eaa3e5_5586_3bd8_9f8f_6f5aff8dadbe_TB5971_11.jpg) ], then there exists a number m in [
], then there exists a number m in [ ![The Mean Value Theorem for Integrals says that if is continuous on [ , ], then there exists a number m in [ , ] such that .](https://storage.examlex.com/TB5971/11eaa3e5_5586_3bd9_9f8f_6b10138f1849_TB5971_11.jpg) ,
, ![The Mean Value Theorem for Integrals says that if is continuous on [ , ], then there exists a number m in [ , ] such that .](https://storage.examlex.com/TB5971/11eaa3e5_5586_62ea_9f8f_41f9c2e03dc4_TB5971_11.jpg) ] such that
] such that ![The Mean Value Theorem for Integrals says that if is continuous on [ , ], then there exists a number m in [ , ] such that .](https://storage.examlex.com/TB5971/11eaa3e5_5586_62eb_9f8f_095a590db5af_TB5971_11.jpg) .
.
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
63
Use the method of disks or washers, or the method of cylindrical shells to find the volume of the solid generated by revolving the region bounded by the graphs of the equations about the indicated axis. Sketch the region and a representative rectangle.
y = 2x2, y = 4x - 2, y = 8; the y-axis
y = 2x2, y = 4x - 2, y = 8; the y-axis

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
64
Use the method of cylindrical shells to find the volume of solid obtained by rotating the region bounded by the given curves about the x axis. 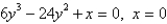
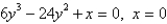

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
65
Find the volume of the solid obtained by rotating the region bounded by the given curves about the specified axis. 
A)
B)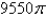
C)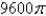
D)
E)None of these

A)

B)
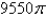
C)
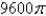
D)

E)None of these

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
66
Use 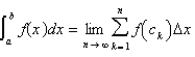 to evaluate the integral.
to evaluate the integral. 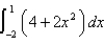
A)22
B)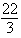
C)
D)54
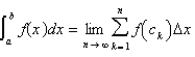 to evaluate the integral.
to evaluate the integral. 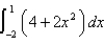
A)22
B)
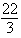
C)

D)54

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
67
The height of a monument is  m. A horizontal cross-section at a distance x meters from the top is an equilateral triangle with side
m. A horizontal cross-section at a distance x meters from the top is an equilateral triangle with side 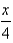 meters. Find the volume of the monument.
meters. Find the volume of the monument.
A)
B)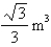
C)
D)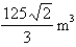
E)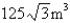
 m. A horizontal cross-section at a distance x meters from the top is an equilateral triangle with side
m. A horizontal cross-section at a distance x meters from the top is an equilateral triangle with side 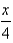 meters. Find the volume of the monument.
meters. Find the volume of the monument.A)

B)
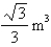
C)

D)
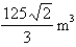
E)
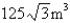

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
68
Use the method of cylindrical shells to find the volume of the solid generated by revolving the region bounded by the graphs of the equations about the indicated axis. Sketch the region and a representative rectangle.
y =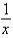 , y = 0, x = 2, x = 5; the y-axis
, y = 0, x = 2, x = 5; the y-axis
y =
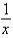 , y = 0, x = 2, x = 5; the y-axis
, y = 0, x = 2, x = 5; the y-axis
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
69
The given expression is the limit of a Riemann sum of a function f on [a, b]. Write this expression as a definite integral on [a, b]. ![<strong>The given expression is the limit of a Riemann sum of a function f on [a, b]. Write this expression as a definite integral on [a, b]. </strong> A) B) C) D)](https://storage.examlex.com/TB5971/11eaa3e5_558a_0ba1_9f8f_03b52bb058ea_TB5971_11.jpg)
A)![<strong>The given expression is the limit of a Riemann sum of a function f on [a, b]. Write this expression as a definite integral on [a, b]. </strong> A) B) C) D)](https://storage.examlex.com/TB5971/11eaa3e5_558a_0ba2_9f8f_cda0622b86bd_TB5971_11.jpg)
B)![<strong>The given expression is the limit of a Riemann sum of a function f on [a, b]. Write this expression as a definite integral on [a, b]. </strong> A) B) C) D)](https://storage.examlex.com/TB5971/11eaa3e5_558a_32b3_9f8f_29767d2f11a6_TB5971_11.jpg)
C)![<strong>The given expression is the limit of a Riemann sum of a function f on [a, b]. Write this expression as a definite integral on [a, b]. </strong> A) B) C) D)](https://storage.examlex.com/TB5971/11eaa3e5_558a_32b4_9f8f_777e04d5cf0d_TB5971_11.jpg)
D)![<strong>The given expression is the limit of a Riemann sum of a function f on [a, b]. Write this expression as a definite integral on [a, b]. </strong> A) B) C) D)](https://storage.examlex.com/TB5971/11eaa3e5_558a_32b5_9f8f_b747950d1f08_TB5971_11.jpg)
![<strong>The given expression is the limit of a Riemann sum of a function f on [a, b]. Write this expression as a definite integral on [a, b]. </strong> A) B) C) D)](https://storage.examlex.com/TB5971/11eaa3e5_558a_0ba1_9f8f_03b52bb058ea_TB5971_11.jpg)
A)
![<strong>The given expression is the limit of a Riemann sum of a function f on [a, b]. Write this expression as a definite integral on [a, b]. </strong> A) B) C) D)](https://storage.examlex.com/TB5971/11eaa3e5_558a_0ba2_9f8f_cda0622b86bd_TB5971_11.jpg)
B)
![<strong>The given expression is the limit of a Riemann sum of a function f on [a, b]. Write this expression as a definite integral on [a, b]. </strong> A) B) C) D)](https://storage.examlex.com/TB5971/11eaa3e5_558a_32b3_9f8f_29767d2f11a6_TB5971_11.jpg)
C)
![<strong>The given expression is the limit of a Riemann sum of a function f on [a, b]. Write this expression as a definite integral on [a, b]. </strong> A) B) C) D)](https://storage.examlex.com/TB5971/11eaa3e5_558a_32b4_9f8f_777e04d5cf0d_TB5971_11.jpg)
D)
![<strong>The given expression is the limit of a Riemann sum of a function f on [a, b]. Write this expression as a definite integral on [a, b]. </strong> A) B) C) D)](https://storage.examlex.com/TB5971/11eaa3e5_558a_32b5_9f8f_b747950d1f08_TB5971_11.jpg)

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
70
The region bounded by the given curves is rotated about the specified axis. Find the volume of the resulting solid by any method. 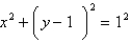 about the y-axis
about the y-axis
A)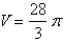
B)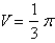
C)
D)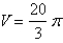
E)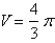
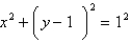 about the y-axis
about the y-axisA)
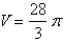
B)
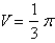
C)

D)
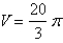
E)
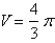

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
71
Find the volume of the solid generated by revolving the region bounded by the graphs of the equations about the indicated line. Sketch the region and a representative rectangle. y = 25 - x2, y = 0; the line 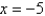
A)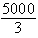
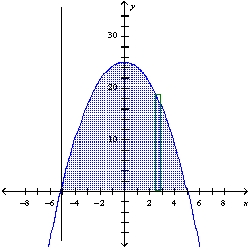
B)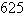
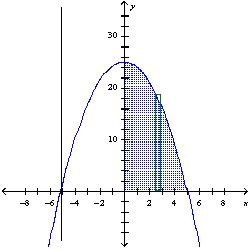
C)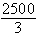
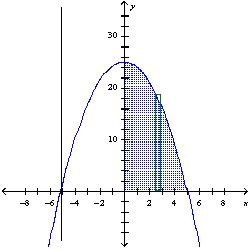
D)
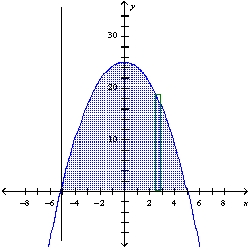
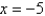
A)
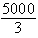

B)
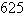

C)
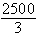

D)



Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
72
Use the method of cylindrical shells to find the volume of the solid generated by revolving the region bounded by the graphs of the equations about the indicated axis. Sketch the region and a representative rectangle.
y = 3x2, y = 0, x = 1; the y-axis
y = 3x2, y = 0, x = 1; the y-axis

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
73
Use the method of disks or washers, or the method of cylindrical shells to find the volume of the solid generated by revolving the region bounded by the graphs of the equations about the indicated axis. Sketch the region and a representative rectangle.  ,
, 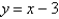 ,
,  , the x-axis
, the x-axis
A)

B)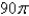
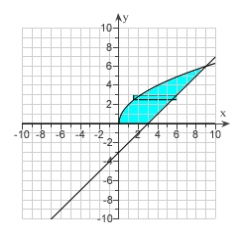
C)
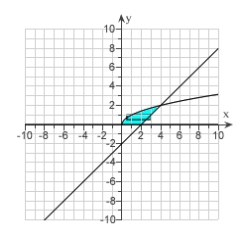
D)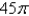
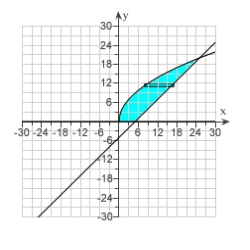
 ,
, 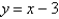 ,
,  , the x-axis
, the x-axisA)


B)
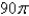

C)


D)
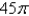


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
74
Find the volume of the solid obtained by rotating the region bounded by  about the x-axis.
about the x-axis.
A)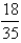
B)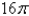
C)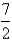
D)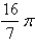
E)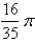
 about the x-axis.
about the x-axis.A)
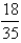
B)
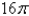
C)
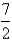
D)
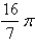
E)
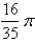

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
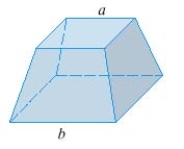
75
The volume of the frustum of a pyramid with square base of side b = 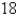 , square top of side a =
, square top of side a = 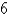 , and height h =
, and height h = 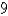 is
is 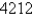 .
. 
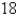 , square top of side a =
, square top of side a = 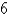 , and height h =
, and height h =  is
is  .
. 

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
76
Sketch a plane region and indicate the axis about which it is revolved so that the resulting solid of revolution (found using the shell method) is given by the integral. 2 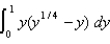
A)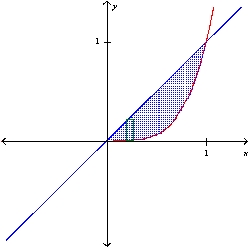 y-axis
y-axis
B)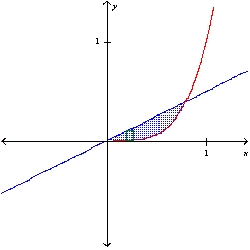 y-axis
y-axis
C)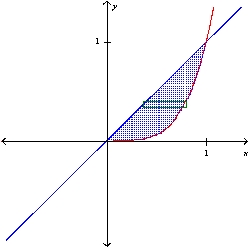 x-axis
x-axis
D)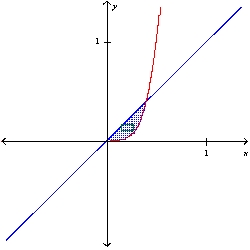 x-axis
x-axis
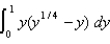
A)
 y-axis
y-axisB)
 y-axis
y-axisC)
 x-axis
x-axisD)
 x-axis
x-axis
Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
77
Use a graphing utility to (a) plot the graphs of the given functions, (b) find the approximate x-coordinates of the points of intersection of the graphs, and (c) find an approximation of the volume of the solid obtained by revolving the region bounded by the graphs of the functions about the y-axis. Round answers to two decimal places.
y = x, y = x5 - x2, x 0
y = x, y = x5 - x2, x 0

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
78
The base of a solid is a circular disk with radius 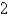 . Find the volume of the solid if parallel cross-sections perpendicular to the base are isosceles right triangles with hypotenuse lying along the base.
. Find the volume of the solid if parallel cross-sections perpendicular to the base are isosceles right triangles with hypotenuse lying along the base.
A)7
B)16
C)49
D)6
E)None of these
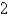 . Find the volume of the solid if parallel cross-sections perpendicular to the base are isosceles right triangles with hypotenuse lying along the base.
. Find the volume of the solid if parallel cross-sections perpendicular to the base are isosceles right triangles with hypotenuse lying along the base.A)7
B)16
C)49
D)6
E)None of these

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
79
Find the volume of the solid obtained by rotating the region bounded by 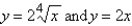 about the line
about the line 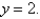
A)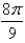
B)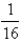
C)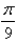
D)
E)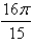
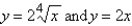 about the line
about the line 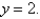
A)
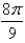
B)
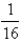
C)
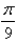
D)

E)
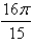

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
80
Use the method of cylindrical shells to find the volume of the solid generated by revolving the region bounded by the graphs of the equations about the indicated axis. Sketch the region and a representative rectangle. y = 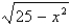 , y = -x + 5; the y-axis
, y = -x + 5; the y-axis
A)250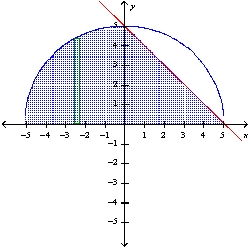
B)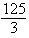
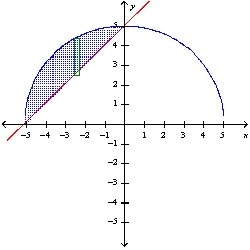
C)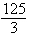
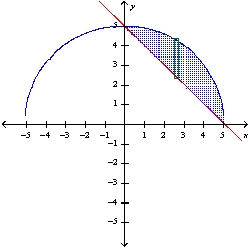
D)250
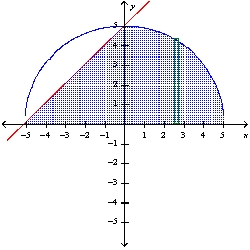
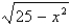 , y = -x + 5; the y-axis
, y = -x + 5; the y-axisA)250

B)
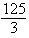

C)
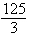

D)250


Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck


