Deck 14: Sampling and Simulation
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/58
Play
Full screen (f)
Deck 14: Sampling and Simulation
1
A new product is being introduced, and a nationwide survey to determine the public's opinion of the product is to be conducted. The country is divided into six regions, then two states are randomly selected from each region, and then each state is divided into rural and urban markets. A number of these markets are selected, and randomly selected people is these markets are surveyed about the new product. What type of sampling technique is being employed here?
A) sequence sampling
B) double sampling
C) multistage sampling
D) systematic sampling
A) sequence sampling
B) double sampling
C) multistage sampling
D) systematic sampling
C
2
Which of the following methods will give a truly random sampling of the general population?
A) Poll random people on the street.
B) Mail out questionnaires to be filled out and returned.
C) Ask a question on a radio station and ask the general public to call in and respond.
D) Identify all them members of the population then select the desired number randomly from among them.
A) Poll random people on the street.
B) Mail out questionnaires to be filled out and returned.
C) Ask a question on a radio station and ask the general public to call in and respond.
D) Identify all them members of the population then select the desired number randomly from among them.
D
3
In stratified sampling, the researcher selects intact, preexisting, or natural groups to use as samples.
False
4
A(n) ______________ is a sample obtained by dividing the population into subgroups according to various homogeneous characteristics and then selecting members from each subgroup for the sample.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
5
Sampling techniques are often used
A) when the population is too large to be analyzed.
B) when the population is too small to be accurately analyzed.
C) when the entire population can be analyzed.
D) when survey questions are difficult to compose.
A) when the population is too large to be analyzed.
B) when the population is too small to be accurately analyzed.
C) when the entire population can be analyzed.
D) when survey questions are difficult to compose.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
6
Sampling methods often use random numbers to select samples.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
7
An example of _________________ sampling could involve sampling successive units from a production line to ensure that products continue to meet certain standards.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
8
An example of a good technique for getting a random sample of the population would be to ask a question over the radio on a station that covers the region where the entire population lives, and have the listeners phone in with an answer.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
9
One major disadvantage of cluster sampling is that the elements in a cluster may not have the same variation in characteristics as elements selected individually from a population.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
10
For a sample to be _______________, every member of the population must have an equal chance of being selected.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
11
Samples are said to be biased when some type of systematic error has been made in the selection of the subjects.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
12
Identify the type of sample obtained by selecting a preexisting or natural group and using the members in the group as the sample.
A) biased sample
B) cluster sample
C) systematic sample
D) stratified sample
A) biased sample
B) cluster sample
C) systematic sample
D) stratified sample

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
13
A(n) __________ is defined as all subjects (human or otherwise) that are being studied.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
14
One limitation of random sampling is that if the population is extremely large, numbering and selecting sample elements could be costly and time consuming.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
15
If all possible samples of a specific size have the same chance of being selected, what type of sampling is being used?
A) random sampling
B) cluster sampling
C) systematic sampling
D) stratified sampling
A) random sampling
B) cluster sampling
C) systematic sampling
D) stratified sampling

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
16
What term is given to a sample chosen at random from a population?
A) biased sample
B) cluster sample
C) unbiased sample
D) stratified sample
A) biased sample
B) cluster sample
C) unbiased sample
D) stratified sample

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
17
If a third of a population is female, then approximately a third of the representative sample should be female.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
18
In double sampling, the researcher uses a combination of sampling methods.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
19
In multistage sampling, a very large population is given a questionnaire to determine those who meet the qualifications for the study.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
20
Identify the type of sample obtained by numbering each element in the population and then selecting every third or fifth or tenth, etc., number from the population to be included in the sample.
A) biased sample
B) cluster sample
C) systematic sample
D) stratified sample
A) biased sample
B) cluster sample
C) systematic sample
D) stratified sample

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
21
A pollster wanted to determine how much support a new library tax would have in a major metropolitan area. The metro area is divided into regions, Southeast, Southwest, Northeast, Northwest, and Central and 100 voters in each region are surveyed. What type of sampling technique is being employed?
A) stratified sampling
B) random sampling
C) cluster sampling
D) systematic sampling
A) stratified sampling
B) random sampling
C) cluster sampling
D) systematic sampling

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
22
A statistics teacher taught Introductory Statistics to a large lecture class of 200 students. She wanted to estimate the average on the first examination by selecting every eighth student's test score and finding the average of these scores. What type of sampling technique is being employed?
A) stratified sampling
B) random sampling
C) cluster sampling
D) systematic sampling
A) stratified sampling
B) random sampling
C) cluster sampling
D) systematic sampling

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
23
A pollster wants to determine which local news channel is watched most frequently in New York City. Two city blocks in Manhattan are used as a sample. What type of sampling technique is being used?

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
24
Which of the following is not a valid advantage for studying a sample rather than an entire population?
A) Sampling saves the researcher time.
B) Sampling enables the researcher to get more detailed information about a particular subject.
C) Sampling is more costly than using the population.
D) Sampling allows the researcher to obtain information that may not be gained otherwise.
A) Sampling saves the researcher time.
B) Sampling enables the researcher to get more detailed information about a particular subject.
C) Sampling is more costly than using the population.
D) Sampling allows the researcher to obtain information that may not be gained otherwise.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
25
"What are your three favorite flavors of ice cream?" would be an example of which type of question?
A) close-ended
B) open-ended
C) faulty
D) biased
A) close-ended
B) open-ended
C) faulty
D) biased

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
26
Which of the following examples of a survey question is not meant to bias the response?
A) "Do you feel that it is inappropriate for record companies to censor music?"
B) "Are you in favor of raising the speed limit on interstate highways?"
C) "At what age should airline pilots be forced into retirement?"
D) "Are you in favor of a small tax to increase social security payments to the elderly?"
A) "Do you feel that it is inappropriate for record companies to censor music?"
B) "Are you in favor of raising the speed limit on interstate highways?"
C) "At what age should airline pilots be forced into retirement?"
D) "Are you in favor of a small tax to increase social security payments to the elderly?"

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
27
A survey could be biased if the identity of those being surveyed is known.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
28
If a researcher chooses a name at random in the phone book and then chooses every 100th name thereafter as a sample, the researcher would be employing the method of systematic sampling.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
29
The survey question "Since everybody likes baseball, you support the construction of the new ball field, don't you?" is a poor survey question because it is
A) too open-ended of a question.
B) a biased question.
C) a question that uses double negatives.
D) a question that is ordered improperly.
A) too open-ended of a question.
B) a biased question.
C) a question that uses double negatives.
D) a question that is ordered improperly.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
30
A small-town politician is running for the district council seat. If the politician decides to poll three blocks in the district to determine his chance of winning the seat, what type of sampling is being used?
A) multistage sampling
B) cluster sampling
C) systematic sampling
D) stratified sampling
A) multistage sampling
B) cluster sampling
C) systematic sampling
D) stratified sampling

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
31
___________ sampling involves giving a questionnaire to a very large population, and then after the questionnaires are reviewed, a smaller population is formed and a sample selected from it.
A) Double
B) Cluster
C) Systematic
D) Stratified
A) Double
B) Cluster
C) Systematic
D) Stratified

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
32
The PAC-10 Conference consists of ten schools. 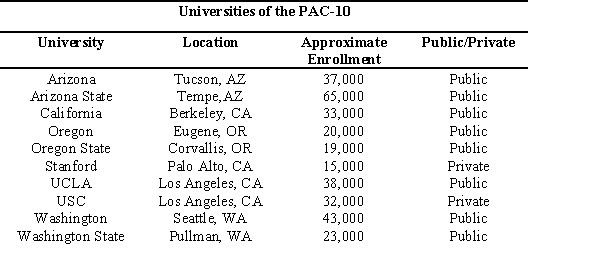 A researcher wants to study and compare the attitudes of the private and public university students within the PAC-10. He wants to select 200 students to interview and wants to use stratified sampling. Which of the following strategies will enable him to accomplish his goal?
A researcher wants to study and compare the attitudes of the private and public university students within the PAC-10. He wants to select 200 students to interview and wants to use stratified sampling. Which of the following strategies will enable him to accomplish his goal?
A) Randomly select 100 students from UCLA and 100 from USC since they are in the same city.
B) Select 100 students out of a large lecture section (more than 200) at California and Stanford since the students are naturally collected there already..
C) Select 100 students out the combined enrollments of USC and Standford, and 100 students out of the combined enrollments of the other eight universities, since he is not interested in cluster sampling.
D) Randomly select 100 students from a randomly selected public PAC-10 university outside California and 100 students from either USC or Stanford, since he is not interested in having all the students in the same state.
 A researcher wants to study and compare the attitudes of the private and public university students within the PAC-10. He wants to select 200 students to interview and wants to use stratified sampling. Which of the following strategies will enable him to accomplish his goal?
A researcher wants to study and compare the attitudes of the private and public university students within the PAC-10. He wants to select 200 students to interview and wants to use stratified sampling. Which of the following strategies will enable him to accomplish his goal?A) Randomly select 100 students from UCLA and 100 from USC since they are in the same city.
B) Select 100 students out of a large lecture section (more than 200) at California and Stanford since the students are naturally collected there already..
C) Select 100 students out the combined enrollments of USC and Standford, and 100 students out of the combined enrollments of the other eight universities, since he is not interested in cluster sampling.
D) Randomly select 100 students from a randomly selected public PAC-10 university outside California and 100 students from either USC or Stanford, since he is not interested in having all the students in the same state.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
33
Which of the following is not an advantage of cluster sampling over the other sampling techniques?
A) cost reduction
B) convenience
C) samples can be more homogeneous
D) simplified field work
A) cost reduction
B) convenience
C) samples can be more homogeneous
D) simplified field work

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
34
A sample is said to be ______________ if it is a randomly chosen representation of the population.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
35
The time and place where a survey is conducted could influence the results.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
36
What type of question gives the subject a list of possible responses from which to choose?
A) close-ended
B) open-ended
C) faulty
D) unbiased
A) close-ended
B) open-ended
C) faulty
D) unbiased

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
37
A researcher wants to learn about unemployment rates across the country. She takes an alphabetical list of the 50 states, numbers the states in order, and selects one state at random. She surveys that state and every every tenth state after it, cycling through the list. What type of sampling technique is the researcher employing?

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
38
The PAC-10 Conference consists of ten schools. 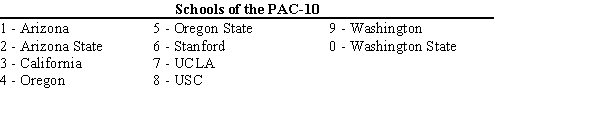 Use the following random number table to select two schools at random from the conference to play the first game of the season. Presume the highlighted entry has been randomly selected as your first school, and you are working down the columns then left to right from column to column to select the next school.
Use the following random number table to select two schools at random from the conference to play the first game of the season. Presume the highlighted entry has been randomly selected as your first school, and you are working down the columns then left to right from column to column to select the next school.  From the table, the first school chosen is California. What will the second school be ?
From the table, the first school chosen is California. What will the second school be ?
A) also California
B) Arizona State
C) Oregon State
D) Oregon
 Use the following random number table to select two schools at random from the conference to play the first game of the season. Presume the highlighted entry has been randomly selected as your first school, and you are working down the columns then left to right from column to column to select the next school.
Use the following random number table to select two schools at random from the conference to play the first game of the season. Presume the highlighted entry has been randomly selected as your first school, and you are working down the columns then left to right from column to column to select the next school.  From the table, the first school chosen is California. What will the second school be ?
From the table, the first school chosen is California. What will the second school be ?A) also California
B) Arizona State
C) Oregon State
D) Oregon

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
39
The PAC-10 Conference consists of ten schools. 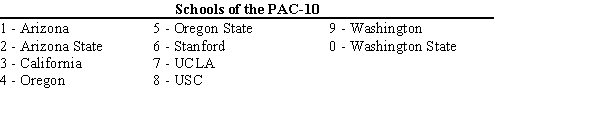 Use systematic sampling selecting every second number from the population to identify five schools out of the ten as a sample. The following random numbers table has already been used to randomly select the first school, USC. What are the other four schools in the sample?
Use systematic sampling selecting every second number from the population to identify five schools out of the ten as a sample. The following random numbers table has already been used to randomly select the first school, USC. What are the other four schools in the sample? 
A) Washington, Washington State, Arizona, Arizona State
B) Arizona, California, Oregon State, UCLA
C) Washington State, Arizona State, Oregon, Stanford
D) USC, USC, California, Arizona State
 Use systematic sampling selecting every second number from the population to identify five schools out of the ten as a sample. The following random numbers table has already been used to randomly select the first school, USC. What are the other four schools in the sample?
Use systematic sampling selecting every second number from the population to identify five schools out of the ten as a sample. The following random numbers table has already been used to randomly select the first school, USC. What are the other four schools in the sample? 
A) Washington, Washington State, Arizona, Arizona State
B) Arizona, California, Oregon State, UCLA
C) Washington State, Arizona State, Oregon, Stanford
D) USC, USC, California, Arizona State

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
40
The survey question "Why do you think people break laws?" is a poor survey question because it
A) is too open-ended of a question.
B) is too close-ended of a question.
C) uses double negatives.
D) is ordered improperly.
A) is too open-ended of a question.
B) is too close-ended of a question.
C) uses double negatives.
D) is ordered improperly.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
41
List two advantages of using simulation techniques instead of actual situations?

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
42
Simulation techniques go back to ancient times when the game of chess was invented to simulate warfare.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
43
Five students were scheduled to take a five-question true-false quiz on the history of the Peloponnesian War. Since they didn't know anything about the subject, they were faced with a decision of whether or not to study. Before they decided to study they decided to run a simulation to see how many of them would likely pass the quiz if they all just guessed randomly. At least three correct answers would be needed to pass. They made up a hypothetical set of correct answers as shown in the following table. 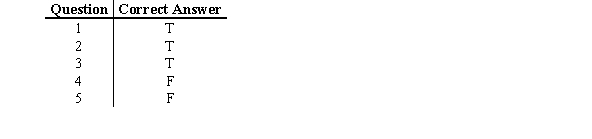 They then used the following random numbers table to ensure that the simulated responses would be truly random. Each simulated set of quiz responses began with a different highlighted number from the table and included the four numbers beneath it. An odd number was said to correspond to an answer of True, and an even number was said to correspond to an answer of False. How many passing grades were there in the five simulated responses?
They then used the following random numbers table to ensure that the simulated responses would be truly random. Each simulated set of quiz responses began with a different highlighted number from the table and included the four numbers beneath it. An odd number was said to correspond to an answer of True, and an even number was said to correspond to an answer of False. How many passing grades were there in the five simulated responses? 
A) 3
B) 4
C) 5
D) 6
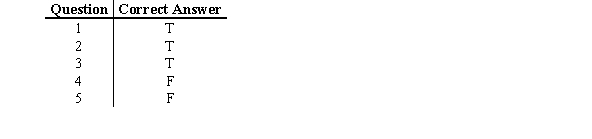 They then used the following random numbers table to ensure that the simulated responses would be truly random. Each simulated set of quiz responses began with a different highlighted number from the table and included the four numbers beneath it. An odd number was said to correspond to an answer of True, and an even number was said to correspond to an answer of False. How many passing grades were there in the five simulated responses?
They then used the following random numbers table to ensure that the simulated responses would be truly random. Each simulated set of quiz responses began with a different highlighted number from the table and included the four numbers beneath it. An odd number was said to correspond to an answer of True, and an even number was said to correspond to an answer of False. How many passing grades were there in the five simulated responses? 
A) 3
B) 4
C) 5
D) 6

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
44
Which of the following is a common simulation technique?
A) ANOVA
B) Monte Carlo method
C) stratified sampling
D) multistage sampling
A) ANOVA
B) Monte Carlo method
C) stratified sampling
D) multistage sampling

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
45
A bin at the store is filled with T-shirts. They are all sized either small or large, and there are approximately equal numbers of each, so the chance of picking either size is 0.5. You need to buy four T-shirts for your friends, all wear small. You want to know how many shirts you must pull out of the bin before you have at least four smalls. Use the random numbers table below to simulate your T-shirt selections. The highlighted numbers were randomly chosen and serve as the first selections for the different trials. Repeat the simulation five times, beginning each trial at a different highlighted number. Work down the columns, and move from left to right, column to column as needed.
Let the even digits correspond to selecting a small, and the odd digits correspond to selecting a large. What is the greatest number of shirts your experiments indicate you need to pull out of the bin before you have four smalls?
What is the greatest number of shirts your experiments indicate you need to pull out of the bin before you have four smalls? 
A) 7
B) 9
C) 11
D) 13
Let the even digits correspond to selecting a small, and the odd digits correspond to selecting a large.
 What is the greatest number of shirts your experiments indicate you need to pull out of the bin before you have four smalls?
What is the greatest number of shirts your experiments indicate you need to pull out of the bin before you have four smalls? 
A) 7
B) 9
C) 11
D) 13

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
46
A researcher is using Monte Carlo techniques to simulate wins and losses in a particular game. When the number 1, 2, or 3 appears, player A is the winner. When the number 4, 5, or 6 appears, player B is the winner. If the number 7, 8, 9, or 0 appears, the game is a draw. Given the following sequence of randomly generated numbers, how many games did player A win?
4, 5, 8, 5, 9, 3, 6, 4, 5, 1, 8, 3, 6
A) 3
B) 4
C) 5
D) 6
4, 5, 8, 5, 9, 3, 6, 4, 5, 1, 8, 3, 6
A) 3
B) 4
C) 5
D) 6

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
47
The survey question "Do you feel that, if people were not allowed to park along this street, that children would not be able to play there?" is a poor survey question because it
A) is too close-ended of a question.
B) is a biased question.
C) uses double negatives.
D) is too open-ended of a question.
A) is too close-ended of a question.
B) is a biased question.
C) uses double negatives.
D) is too open-ended of a question.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
48
In many women's tennis matches they play best-out-of-three sets. In other words, they play until one player wins two sets, and there are no ties. Lourdes and Mariana are two rivals, though Lourdes seems to win about six sets for every four that Mariana wins. Use the random numbers table below to simulate 10 matches between Lourdes and Marian. Let an entry in the table correspond to one set, 0-5 corresponds to Lourdes winning the set, and 6-9 corresponds to Mariana winning the set. Begin each match with a different highlighted number, and use it with up to two entries below it to represent a match.
In how many of the ten simulated matches did Mariana win?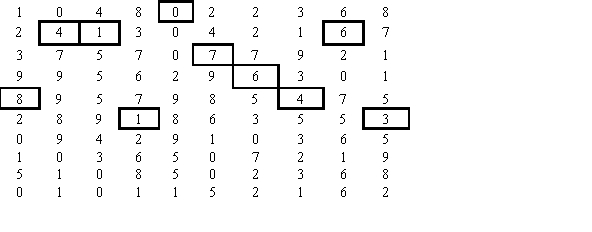
A) 0
B) 1
C) 2
D) 3
In how many of the ten simulated matches did Mariana win?

A) 0
B) 1
C) 2
D) 3

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
49
A flip of a fair coin is used to simulate the gender of a series of simulated students.  Given the following four flips, how many female students were there?
Given the following four flips, how many female students were there?
H H T H
A) 3
B) 4
C) 1
D) 2
 Given the following four flips, how many female students were there?
Given the following four flips, how many female students were there?H H T H
A) 3
B) 4
C) 1
D) 2

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
50
The _______________ method is a simulation technique using random numbers.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
51
In a free-throw relay, one member of a team of four shoots free-throws (a type of shot in basketball) until she makes one, then the next team member shoots until she makes one. This continues through the third and forth team members. In a competition, the first team to finish wins. The question arises whether it is better to have four average shooters or two good shooters and two not-so-good ones, if the overall shooting percentage of the teams is the same. Use a Monte Carlo simulation to help answer this question. Each number in the random numbers table below will correspond to one free throw.
One simulated team has four 50% free-throw shooters (the 50's). The order of the shooters does not matter. The digits 0-4 will correspond to making a free throw and the digits 5-9 will correspond to missing.
The other simulated team has two 80% free-throw shooters and two 20% free-throw shooters (the 80/20's). Let the 80% shooters be the first two of the four to shoot. For the 80% shooters, the digits 0-7 will correspond to making the free throw, and the digits 8-9 will correspond to missing. For the 20% shooters, the digits 0-1 will correspond to making the free throw and 2-9 will correspond to missing.
Work down the columns, moving to the column to the right as needed. The highlighted numbers were selected at random. Begin the 80/20's team at the 6, and begin the 50's team at the 7.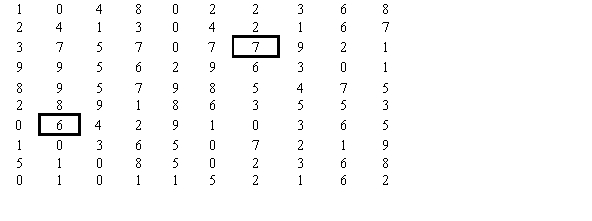 Simulate one race for each team. Count the number of shots each team needs to finish.
Simulate one race for each team. Count the number of shots each team needs to finish.
A) The 80's/20's needed 10, while the 50's needed 10
B) The 80's/20's needed 8, while the 50's needed 5
C) The 80's/20's needed 5, while the 50's needed 7
D) The 80's/20's needed 4, while the 50's needed 8
One simulated team has four 50% free-throw shooters (the 50's). The order of the shooters does not matter. The digits 0-4 will correspond to making a free throw and the digits 5-9 will correspond to missing.
The other simulated team has two 80% free-throw shooters and two 20% free-throw shooters (the 80/20's). Let the 80% shooters be the first two of the four to shoot. For the 80% shooters, the digits 0-7 will correspond to making the free throw, and the digits 8-9 will correspond to missing. For the 20% shooters, the digits 0-1 will correspond to making the free throw and 2-9 will correspond to missing.
Work down the columns, moving to the column to the right as needed. The highlighted numbers were selected at random. Begin the 80/20's team at the 6, and begin the 50's team at the 7.
 Simulate one race for each team. Count the number of shots each team needs to finish.
Simulate one race for each team. Count the number of shots each team needs to finish.A) The 80's/20's needed 10, while the 50's needed 10
B) The 80's/20's needed 8, while the 50's needed 5
C) The 80's/20's needed 5, while the 50's needed 7
D) The 80's/20's needed 4, while the 50's needed 8

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
52
In simulating experiments using the Monte Carlo method, one must define a correspondence between the random numbers and the outcomes.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
53
The basic simulation technique using random numbers is referred to as the Las Vegas method.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
54
Why would researchers want to use a simulation rather than study the actual situation?
A) Simulations demonstrate many additional features which the actual situation doesn't possess.
B) The actual situation may be too dangerous.
C) Simulations usually employ more sophisticated computer software than the actual situations.
D) The actual situation may not give the desired outcomes.
A) Simulations demonstrate many additional features which the actual situation doesn't possess.
B) The actual situation may be too dangerous.
C) Simulations usually employ more sophisticated computer software than the actual situations.
D) The actual situation may not give the desired outcomes.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
55
A particular baseball player gets, on average, three hits for every ten times he comes to bat. In a typical game, he comes to bat five times. Use the random numbers table below to simulate his hitting in ten games. Let an entry in the table correspond to one time at bat, 0-2 corresponds to getting a hit, and 3-9 corresponds to not getting a hit. The highlighted numbers were selected at random, and a different one should be used to start each of the player's simulated games. That is, use a highlighted number and the four numbers below it to represent his batting in one game.
In how many of the ten simulated games did he get three or more hits?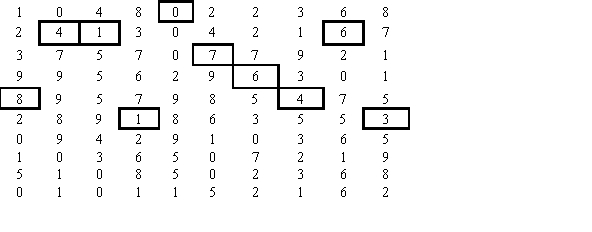
A) 2
B) 3
C) 4
D) 5
In how many of the ten simulated games did he get three or more hits?

A) 2
B) 3
C) 4
D) 5

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
56
A researcher is using a random numbers table to pick between two groups labeled X and Y. X is to be chosen with probability 5/7 and Y with probability 2/7. The researcher assigns the digits 1, 2, 3, 4, and 5 to X, and she assigns the digits 6 and 7 to Y. The remaining digits are neglected. The researcher uses this section of a random number table
6 8 5 1 7 4 4 0 4 0
What are the first and second choices?
A) The first selection is X, and the second is X.
B) The first selection is X, and the second is Y.
C) The first selection is Y, and the second is X.
D) The first selection is Y, and the second is Y.
6 8 5 1 7 4 4 0 4 0
What are the first and second choices?
A) The first selection is X, and the second is X.
B) The first selection is X, and the second is Y.
C) The first selection is Y, and the second is X.
D) The first selection is Y, and the second is Y.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
57
Simulate ten coin tosses using the following random numbers table. Represent the first toss with the highlighted number, then move down the column number-by-number moving from the bottom a column to the top of the next column to the right until ten numbers have been chosen. If the odd numbers represent tails, and the even numbers represent heads, how many tails are there in the group of ten simulated tosses? 


Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
58
Five-digit random numbers are used to simulate scores on each hole in an 18-hole round of golf. The correspondence between the random numbers and scores on each hole is chosen to be as follows. 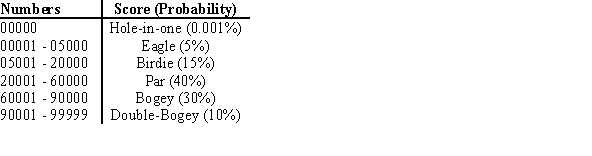 Use the following portion of a random numbers table starting with the highlighted number, and determine how many holes the simulated golfer made par or better (i.e., how many holes-in-one, eagles, birdies, and pars were obtained in the 18 holes). Use the highlighted number as the score on the first hole; work down a column moving from left to right, column to column as needed.
Use the following portion of a random numbers table starting with the highlighted number, and determine how many holes the simulated golfer made par or better (i.e., how many holes-in-one, eagles, birdies, and pars were obtained in the 18 holes). Use the highlighted number as the score on the first hole; work down a column moving from left to right, column to column as needed. 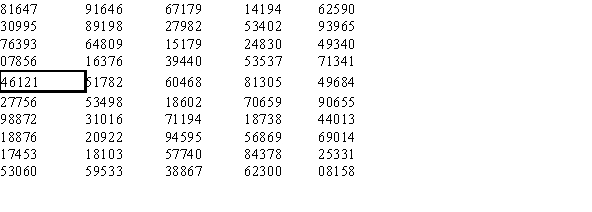
A) 11
B) 12
C) 13
D) 14
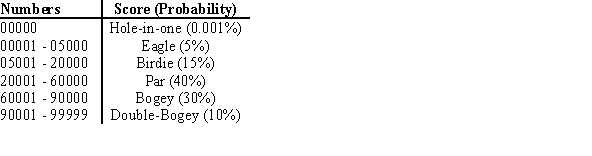 Use the following portion of a random numbers table starting with the highlighted number, and determine how many holes the simulated golfer made par or better (i.e., how many holes-in-one, eagles, birdies, and pars were obtained in the 18 holes). Use the highlighted number as the score on the first hole; work down a column moving from left to right, column to column as needed.
Use the following portion of a random numbers table starting with the highlighted number, and determine how many holes the simulated golfer made par or better (i.e., how many holes-in-one, eagles, birdies, and pars were obtained in the 18 holes). Use the highlighted number as the score on the first hole; work down a column moving from left to right, column to column as needed. 
A) 11
B) 12
C) 13
D) 14

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck


