Deck 8: Hypothesis Testing
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/83
Play
Full screen (f)
Deck 8: Hypothesis Testing
1
Which type of null hypothesis is used in the figure below? 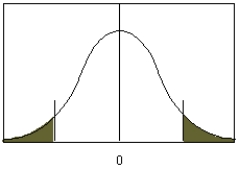
A)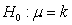
B)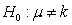
C)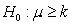
D)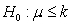

A)
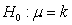
B)
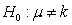
C)
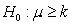
D)
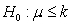
A
2
The null hypothesis states that there is no difference between a parameter and a specific value, or that there is no difference between two parameters.
True
3
Are the following statements 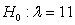 and
and 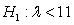 a valid pair of null and alternative hypothesis?
a valid pair of null and alternative hypothesis?
A) No, the null hypothesis should not state an equality.
B) No,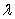 cannot be a parameter
cannot be a parameter
C) Yes, the null hypothesis specifies an equality and the alternative specifies a difference.
D) Yes, the alternative hypothesis specifies an equality and the null hypothesis specifies a difference.
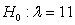 and
and 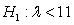 a valid pair of null and alternative hypothesis?
a valid pair of null and alternative hypothesis?A) No, the null hypothesis should not state an equality.
B) No,
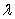 cannot be a parameter
cannot be a parameterC) Yes, the null hypothesis specifies an equality and the alternative specifies a difference.
D) Yes, the alternative hypothesis specifies an equality and the null hypothesis specifies a difference.
C
4
Sandra Johnson, a researcher, believes her pulse rate will decrease with exercise. Her alternative hypothesis would contain an equal sign.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
5
Which type of null hypothesis is used in the figure below? 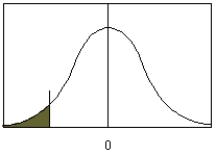
A)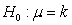
B)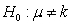
C)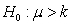
D)

A)
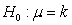
B)
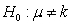
C)
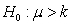
D)


Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
6
In a(n) _____________ test, the null hypothesis should be rejected when the test value is in either of the two critical regions.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
7
Which type of alternative hypothesis is used in the figure below? 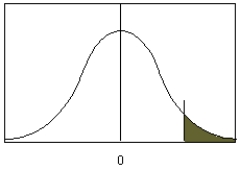
A)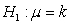
B)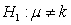
C)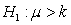
D)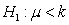

A)
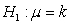
B)
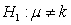
C)
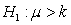
D)
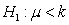

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
8
A type I error occurs if one rejects the null hypothesis when it is __________.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
9
If the null hypothesis is not rejected when it is false, a type II error has occurred.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
10
A right-tailed test is used when  .
.
 .
.
Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
11
Is the statement 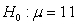 a valid null hypothesis?
a valid null hypothesis?
A) Yes, this is a statement that compares a parameter to a value.
B) Yes, this is a statement that compares two parameters.
C) No, equalities are not permitted in a null hypothesis.
D) No, there is no parameter contained in this statement.
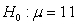 a valid null hypothesis?
a valid null hypothesis?A) Yes, this is a statement that compares a parameter to a value.
B) Yes, this is a statement that compares two parameters.
C) No, equalities are not permitted in a null hypothesis.
D) No, there is no parameter contained in this statement.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
12
The probability of a type I error is represented by which of the following symbols?
A) φ
B) β
C) η
D) α
A) φ
B) β
C) η
D) α

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
13
Stating the hypothesis should be the first step used in hypothesis testing.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
14
Are the following statements 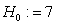 and
and 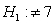 valid null and alternative hypotheses?
valid null and alternative hypotheses?
A) No, there are no parameters contained in these statements.
B) No, the alternative hypothesis cannot contain numeric values.
C) Yes, these statements are two non-overlapping hypotheses and compare two parameters.
D) Yes, these statements are two non-overlapping hypotheses and compare a parameter to a value.
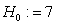 and
and 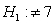 valid null and alternative hypotheses?
valid null and alternative hypotheses?A) No, there are no parameters contained in these statements.
B) No, the alternative hypothesis cannot contain numeric values.
C) Yes, these statements are two non-overlapping hypotheses and compare two parameters.
D) Yes, these statements are two non-overlapping hypotheses and compare a parameter to a value.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
15
In performing a hypothesis test, one should decide whether to reject or not reject the null hypothesis before summarizing the results.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
16
A type II error occurs if one does not reject the null hypothesis when it is __________.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
17
Is the statement 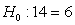 a valid null hypothesis?
a valid null hypothesis?
A) Yes, this is a statement that compares a parameter to a value.
B) Yes, this is a statement that compares two parameters.
C) No, equalities are not permitted in a null hypothesis.
D) No, there is no parameter contained in this statement.
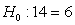 a valid null hypothesis?
a valid null hypothesis?A) Yes, this is a statement that compares a parameter to a value.
B) Yes, this is a statement that compares two parameters.
C) No, equalities are not permitted in a null hypothesis.
D) No, there is no parameter contained in this statement.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
18
Using the z table, find the critical value (or values) for an  = 0.03 two-tailed test.
= 0.03 two-tailed test.
A) 1.88
B) 2.17
C)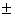 1.88
1.88
D) 2.17
2.17
 = 0.03 two-tailed test.
= 0.03 two-tailed test.A) 1.88
B) 2.17
C)
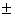 1.88
1.88D)
 2.17
2.17
Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
19
The numerical value obtained from a statistical test is called the _______________.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
20
Is the statement 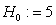 a valid null hypothesis?
a valid null hypothesis?
A) Yes, this is a statement that compares a parameter to a value.
B) Yes, this is a statement that compares two parameters.
C) No, equalities are not permitted in a null hypothesis.
D) No, there is no parameter contained in this statement.
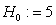 a valid null hypothesis?
a valid null hypothesis?A) Yes, this is a statement that compares a parameter to a value.
B) Yes, this is a statement that compares two parameters.
C) No, equalities are not permitted in a null hypothesis.
D) No, there is no parameter contained in this statement.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
21
Using the z table, determine the critical values for a two-tailed test when α = 0.03.
A) ± 1.88
B) ± 2.17
C) ± 0.18
D) ± 0.06
A) ± 1.88
B) ± 2.17
C) ± 0.18
D) ± 0.06

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
22
Alyson Silvastein, a scientist, reports that the average number of hours a person can go without sleep and still function normally is 29 with a standard deviation of five hours. A sample of 49 people had an average of 31 hours that they could go without sleep and still function normally. The test value for this hypothesis would be 2.8.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
23
A garbage collector believes that he averages picking up more than four tons of garbage per day. What is the null hypothesis for his statement?
A)
B)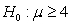
C)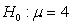
D)
A)

B)
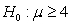
C)
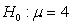
D)


Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
24
Using the z table, find the critical value (or values) for an 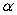 = 0.12 right-tailed test.
= 0.12 right-tailed test.
A) 0.59
B) 0.78
C) 1.18
D) 1.56
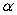 = 0.12 right-tailed test.
= 0.12 right-tailed test.A) 0.59
B) 0.78
C) 1.18
D) 1.56

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
25
If the sample mean is 7, the hypothesized population mean is 4, and the population standard deviation is 2, compute the test value needed for the z test.
A) 1.50
B) 3.00
C) -3.00
D) -1.50
A) 1.50
B) 3.00
C) -3.00
D) -1.50

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
26
A recent survey of gasoline prices indicated that the national average was $4.098 per gallon. The Dallas Automobile Club claimed that gasoline in Texas was significantly lower than the national average. A survey covering 10 different suburbs in Dallas found the average price of gasoline to be $3.924 per gallon with a population standard deviation of $0.053. What critical value should be used to test the claim using α = 0.01?
A) 1.26
B) 2.33
C) -1.26
D) -2.33
A) 1.26
B) 2.33
C) -1.26
D) -2.33

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
27
For the conjecture "The average age of students in this class is 20", the null hypothesis is:
A) We accept the hypothesis that the average age of students in this class is 20.
B) The average age of students in this class is 20.
C) The average age of students in this class is not 20.
D) We reject the hypothesis that the average age of students in this class is 20.
A) We accept the hypothesis that the average age of students in this class is 20.
B) The average age of students in this class is 20.
C) The average age of students in this class is not 20.
D) We reject the hypothesis that the average age of students in this class is 20.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
28
When conducting a two-tailed z test with α = 0.01, the test value was computed to be 2.07. The decision would be to not reject the null hypothesis.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
29
For the conjecture "The average weight of a cuckoo bird is less than 1.3 pounds", the null and alternative hypotheses are:
A)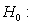 The average weight of a cuckoo bird is less than or equal to 1.3 pounds.
The average weight of a cuckoo bird is less than or equal to 1.3 pounds. 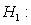 The average weight of a cuckoo bird is less than 1.3 pounds.
The average weight of a cuckoo bird is less than 1.3 pounds.
B)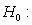 The average weight of a cuckoo bird is equal to 1.3 pounds.
The average weight of a cuckoo bird is equal to 1.3 pounds. 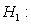 The average weight of a cuckoo bird is less than 1.3 pounds.
The average weight of a cuckoo bird is less than 1.3 pounds.
C)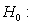 The average weight of a cuckoo bird is more than 1.3 pounds.
The average weight of a cuckoo bird is more than 1.3 pounds. 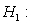 The average weight of a cuckoo bird is less than 1.3 pounds.
The average weight of a cuckoo bird is less than 1.3 pounds.
D)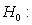 The average weight of a cuckoo bird is less than $1.3 pounds.
The average weight of a cuckoo bird is less than $1.3 pounds. 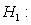 The average weight of a cuckoo bird is more than or equal to $1.3 pounds.
The average weight of a cuckoo bird is more than or equal to $1.3 pounds.
A)
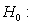 The average weight of a cuckoo bird is less than or equal to 1.3 pounds.
The average weight of a cuckoo bird is less than or equal to 1.3 pounds. 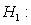 The average weight of a cuckoo bird is less than 1.3 pounds.
The average weight of a cuckoo bird is less than 1.3 pounds.B)
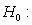 The average weight of a cuckoo bird is equal to 1.3 pounds.
The average weight of a cuckoo bird is equal to 1.3 pounds. 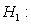 The average weight of a cuckoo bird is less than 1.3 pounds.
The average weight of a cuckoo bird is less than 1.3 pounds.C)
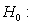 The average weight of a cuckoo bird is more than 1.3 pounds.
The average weight of a cuckoo bird is more than 1.3 pounds. 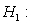 The average weight of a cuckoo bird is less than 1.3 pounds.
The average weight of a cuckoo bird is less than 1.3 pounds.D)
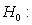 The average weight of a cuckoo bird is less than $1.3 pounds.
The average weight of a cuckoo bird is less than $1.3 pounds. 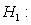 The average weight of a cuckoo bird is more than or equal to $1.3 pounds.
The average weight of a cuckoo bird is more than or equal to $1.3 pounds.
Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
30
Use the following to answer questions :
The Eagle Ridge Contractors Association claims the average price of a home in their subdivision is $125,150 with a standard deviation of $7,350. A sample of 36 homes for sale in this subdivision had an average selling price of $123,550. The Eagle Ridge Home Owners Association is interested in knowing if the costs of homes for sale in this subdivision are actually lower than claimed?
What is the p-value for this left-tailed test?
A) 0.0853
B) 0.0951
C) 0.1327
D) 0.0036
The Eagle Ridge Contractors Association claims the average price of a home in their subdivision is $125,150 with a standard deviation of $7,350. A sample of 36 homes for sale in this subdivision had an average selling price of $123,550. The Eagle Ridge Home Owners Association is interested in knowing if the costs of homes for sale in this subdivision are actually lower than claimed?
What is the p-value for this left-tailed test?
A) 0.0853
B) 0.0951
C) 0.1327
D) 0.0036

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
31
Use the following to answer questions:
A recent survey indicated that the average amount spent for breakfast by business managers was $7.58 with a standard deviation of $0.42. It was felt that breakfasts on the West Coast were higher than $7.58. A sample of 81 business managers on the West Coast had an average breakfast cost of $7.65.
At α = 0.05, what is the critical value?
A) 1.96
B) 1.5
C) -1.5
D) 1.65
A recent survey indicated that the average amount spent for breakfast by business managers was $7.58 with a standard deviation of $0.42. It was felt that breakfasts on the West Coast were higher than $7.58. A sample of 81 business managers on the West Coast had an average breakfast cost of $7.65.
At α = 0.05, what is the critical value?
A) 1.96
B) 1.5
C) -1.5
D) 1.65

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
32
Using the z table, determine the critical value for the left-tailed test with α = 0.02.
A) 2.33
B) 2.05
C) -2.33
D) -2.05
A) 2.33
B) 2.05
C) -2.33
D) -2.05

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
33
If the sample mean is 8, the hypothesized population mean is 11, and the population standard deviation is 2, compute the test value needed for the z test.
A) 1.50
B) 3.00
C) -3.00
D) -1.50
A) 1.50
B) 3.00
C) -3.00
D) -1.50

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
34
Dr. Christina Cuttleman, a nutritionist, claims that the average number of calories in a serving of popcorn is 75 with a standard deviation of 7. A sample of 50 servings of popcorn was found to have an average of 78 calories. Check Dr. Cuttleman's claim at α = 0.05.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
35
Using the z table, determine the critical value for the right-tailed test with α = 0.035.
A) 0.35
B) 0.70
C) 1.81
D) 2.43
A) 0.35
B) 0.70
C) 1.81
D) 2.43

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
36
Using the z table, find the critical value (or values) for an  = 0.021 left-tailed test.
= 0.021 left-tailed test.
A) -2.03
B) -2.31
C) -1.01
D) -1.15
 = 0.021 left-tailed test.
= 0.021 left-tailed test.A) -2.03
B) -2.31
C) -1.01
D) -1.15

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
37
The critical value for a right-tailed test with α = 0.03 is 1.88.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
38
For the conjecture "The average rent of an apartment is more than $900 per month," the alternative hypothesis is:
A) The average rent of an apartment is less than or equal to $900 per month.
B) The average rent of an apartment is greater than $900 per month.
C) We accept the hypothesis that the average rent of an apartment is more than $900 per month.
D) We reject the hypothesis that the average rent of an apartment is more than $900 per month.
A) The average rent of an apartment is less than or equal to $900 per month.
B) The average rent of an apartment is greater than $900 per month.
C) We accept the hypothesis that the average rent of an apartment is more than $900 per month.
D) We reject the hypothesis that the average rent of an apartment is more than $900 per month.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
39
Use the following to answer questions :
The Eagle Ridge Contractors Association claims the average price of a home in their subdivision is $125,150 with a standard deviation of $7,350. A sample of 36 homes for sale in this subdivision had an average selling price of $123,550. The Eagle Ridge Home Owners Association is interested in knowing if the costs of homes for sale in this subdivision are actually lower than claimed?
Compute the test value?
A) -1.31
B) 1.31
C) -1.52
D) 1.52
The Eagle Ridge Contractors Association claims the average price of a home in their subdivision is $125,150 with a standard deviation of $7,350. A sample of 36 homes for sale in this subdivision had an average selling price of $123,550. The Eagle Ridge Home Owners Association is interested in knowing if the costs of homes for sale in this subdivision are actually lower than claimed?
Compute the test value?
A) -1.31
B) 1.31
C) -1.52
D) 1.52

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
40
Use the following to answer questions:
A recent survey indicated that the average amount spent for breakfast by business managers was $7.58 with a standard deviation of $0.42. It was felt that breakfasts on the West Coast were higher than $7.58. A sample of 81 business managers on the West Coast had an average breakfast cost of $7.65.
Find the P-value for the test.
A) 0.4332
B) 0.2734
C) 0.1325
D) 0.0668
A recent survey indicated that the average amount spent for breakfast by business managers was $7.58 with a standard deviation of $0.42. It was felt that breakfasts on the West Coast were higher than $7.58. A sample of 81 business managers on the West Coast had an average breakfast cost of $7.65.
Find the P-value for the test.
A) 0.4332
B) 0.2734
C) 0.1325
D) 0.0668

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
41
An average department store sells 150 men's suits per year. The men's suit departments at a particular national chain of stores claim they sell more than the industry average. In 45 of their stores, they sold, on the average, 190 suits per year with a population standard deviation of 30. What is the test value for their claim?
A) 0.20
B) 1.33
C) 5.14
D) 8.94
A) 0.20
B) 1.33
C) 5.14
D) 8.94

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
42
In a particular city, the average annual salary for secretaries is $31,000. A sample of 45 secretaries from Company A shows an average annual salary of $27,500 with a population standard deviation of $5,500. Secretaries at Company A claim they are paid less than the city average. What is the test value for this claim?
A) -4.27
B) -0.64
C) 0.64
D) 4.27
A) -4.27
B) -0.64
C) 0.64
D) 4.27

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
43
Reginald Brown, an inspector from the Department of Weights and Measures, weighed 15 eighteen-ounce cereal boxes of corn flakes. He found their mean weight to be 17.8 ounces with a standard deviation of 0.4 ounces. At α = 0.01, are the cereal boxes lighter than they should be?

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
44
State whether the null hypothesis should be rejected on the basis of the given P-value.
P-value = 0.235,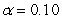 , two-tailed test
, two-tailed test
A) Do not reject.
B) Reject.
P-value = 0.235,
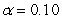 , two-tailed test
, two-tailed testA) Do not reject.
B) Reject.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
45
At a certain university, the average attendance at basketball games has been 2925. This year the attendance for the first 11 games has been 2715 with a standard deviation of 535. The athletic director claims that the attendance is the same as last year. If 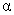 = .05, what are the critical values for this two-tailed t test?
= .05, what are the critical values for this two-tailed t test?
A)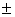 1.812
1.812
B)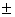 2.228
2.228
C) 1.796
1.796
D)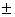 2.201
2.201
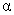 = .05, what are the critical values for this two-tailed t test?
= .05, what are the critical values for this two-tailed t test?A)
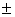 1.812
1.812B)
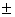 2.228
2.228C)
 1.796
1.796D)
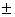 2.201
2.201
Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
46
The average greyhound can reach a top speed of 18.5 meters per second. A particular greyhound breeder claims her dogs are faster than the average greyhound. A sample of 40 of her dogs ran, on average, 19.2 meters per second with a population standard deviation of 1.2 meters per second. With α = 0.05, is her claim correct?
A) Yes, because the test value 0.09 falls in the noncritical region.
B) No, because the test value 0.09 falls in the critical region.
C) Yes, because the test value 3.69 falls in the critical region.
D) No, because the test value 0.70 falls in the critical region.
A) Yes, because the test value 0.09 falls in the noncritical region.
B) No, because the test value 0.09 falls in the critical region.
C) Yes, because the test value 3.69 falls in the critical region.
D) No, because the test value 0.70 falls in the critical region.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
47
At a certain university, the average cost of books was $320 per student last semester and the population standard deviation was $85. This semester a sample of 55 students revealed an average cost of books of $355 per student. The Dean of Students believes that the costs are greater this semester. What is the test value for this hypothesis?
A) 0.41
B) 0.64
C) 3.05
D) 22.65
A) 0.41
B) 0.64
C) 3.05
D) 22.65

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
48
An average computer mouse inspector can inspect 45 mice per hour with a population standard deviation of 14 mice per hour. The 44 computer mice inspectors at a particular factory can only inspect 38 mice per hour. Does the company have reason to believe that these inspectors are slower than average at α = 0.10?
A) Yes, because the test value -3.32 falls in the noncritical region.
B) No, because the test value -1.91 falls in the critical region.
C) Yes, because the test value -3.32 falls in the critical region.
D) No, because the test value -1.91 falls in the noncritical region.
A) Yes, because the test value -3.32 falls in the noncritical region.
B) No, because the test value -1.91 falls in the critical region.
C) Yes, because the test value -3.32 falls in the critical region.
D) No, because the test value -1.91 falls in the noncritical region.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
49
A manufacturer claims that its televisions have an average lifetime of at least five years (60 months) with a population standard deviation of seven months. Eighty-one televisions were selected at random, and the average lifetime was found to be 59 months. With α = 0.025, is the manufacturer's claim supported?

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
50
State whether the null hypothesis should be rejected on the basis of the given P-value.
P-value = 0.172,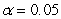 , one-tailed test
, one-tailed test
A) Do not reject.
B) Reject.
P-value = 0.172,
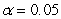 , one-tailed test
, one-tailed testA) Do not reject.
B) Reject.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
51
A recent report by the American Medical Association stated the average annual salary of psychiatrists is $189,121 with a population standard deviation of $26,975. A group of hospital administrators randomly sampled 64 psychiatrists and found an average annual salary of $198,630. The group claims that the average annual salary is actually higher than what the American Medical Association reported. What is the P-value for this one-sided test?
A) 0.0024
B) 0.0035
C) 0.1328
D) 0.0237
A) 0.0024
B) 0.0035
C) 0.1328
D) 0.0237

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
52
Science fiction novels average 250 pages in length. The average length of 10 randomly chosen novels written by I. M. Wordy was 295 pages in length with a standard deviation of 60. At 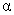 = .05, are Wordy's novels significantly longer than the average science fiction novel?
= .05, are Wordy's novels significantly longer than the average science fiction novel?
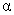 = .05, are Wordy's novels significantly longer than the average science fiction novel?
= .05, are Wordy's novels significantly longer than the average science fiction novel?
Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
53
According to Beautiful Bride magazine, the average age of a groom is now 26.2 years. A sample of 16 prospective grooms in Chicago revealed that their average age was 26.6 years with a standard deviation of 5.3 years. What is the test value for a t test of the claim?
A) 1.81
B) 0.30
C) 0.59
D) 2.13
A) 1.81
B) 0.30
C) 0.59
D) 2.13

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
54
What is the critical value for a right-tailed t test when α = 0.025 and n = 13?
A) 2.179
B) 0.697
C) 0.695
D) 2.201
A) 2.179
B) 0.697
C) 0.695
D) 2.201

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
55
The average magnesium concentration in ground water around Metro City is 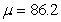 parts per million (ppm) with a standard deviation of
parts per million (ppm) with a standard deviation of 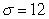 . The table below shows the ground-water magnesium concentrations (ppm) from random sites in a suburb located 15 miles away. At
. The table below shows the ground-water magnesium concentrations (ppm) from random sites in a suburb located 15 miles away. At 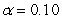 , can it be concluded that the average magnesium concentration in the suburb differs from 86.2 ppm?
, can it be concluded that the average magnesium concentration in the suburb differs from 86.2 ppm?  a. State the hypotheses and identify the claim.
a. State the hypotheses and identify the claim.
b. Find the critical values.
c. Compute the test statistic.
d. Make a decision.
e. Summarize the results.
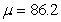 parts per million (ppm) with a standard deviation of
parts per million (ppm) with a standard deviation of 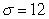 . The table below shows the ground-water magnesium concentrations (ppm) from random sites in a suburb located 15 miles away. At
. The table below shows the ground-water magnesium concentrations (ppm) from random sites in a suburb located 15 miles away. At 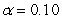 , can it be concluded that the average magnesium concentration in the suburb differs from 86.2 ppm?
, can it be concluded that the average magnesium concentration in the suburb differs from 86.2 ppm?  a. State the hypotheses and identify the claim.
a. State the hypotheses and identify the claim.b. Find the critical values.
c. Compute the test statistic.
d. Make a decision.
e. Summarize the results.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
56
Find the P-value for the test value.
T = 1.581, n = 23, right-tailed
A) 0.0321
B) 0.1282
C) 0.0641
D) 0.0687
T = 1.581, n = 23, right-tailed
A) 0.0321
B) 0.1282
C) 0.0641
D) 0.0687

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
57
A recent study of business travelers claims they spend an average of $41.00 per day on meals. As a test of this claim, a random sampling of 16 business travelers found they had spent an average of $45.00 per day with a standard deviation of $3.65. What are the critical values for a two-tailed t test of this claim with α = 0.05?
A) ± 2.120
B) ± 2.131
C) ± 1.753
D) ± 1.746
A) ± 2.120
B) ± 2.131
C) ± 1.753
D) ± 1.746

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
58
What is the critical value for a two-tailed t test when α = 0.02 and n = 19?
A) 2.878
B) 2.567
C) 2.552
D) 2.110
A) 2.878
B) 2.567
C) 2.552
D) 2.110

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
59
Sam Ying, a career counselor, claims the average number of years of schooling for an engineer is 15.8 years. A sample of 16 engineers had a mean of 15.0 years and a standard deviation of 1.5 years. The test value used in evaluating the claim would be -2.68.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
60
Nationwide, the average waiting time until a electric utility customer service representative answers a call is 210 seconds. The Gigantic Kilowatt Energy Company randomly sampled 30 calls and found that, on average, they were answered in 183 seconds with a population standard deviation of 30 seconds. Can the company claim that they are faster than the average utility at α = 0.05?
A) Yes, because the test value -4.93 falls in the critical region.
B) No, because the test value -0.90 falls in the critical region.
C) Yes, because the test value -0.16 falls in the critical region.
D) No, because the test value -0.16 falls in the critical region.
A) Yes, because the test value -4.93 falls in the critical region.
B) No, because the test value -0.90 falls in the critical region.
C) Yes, because the test value -0.16 falls in the critical region.
D) No, because the test value -0.16 falls in the critical region.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
61
A political strategist claims that 56% of voters in Madison County support his candidate. In a poll of 200 randomly selected voters, 100 of them support the strategist's candidate. At 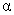 = .05, is the political strategist's claim warranted?
= .05, is the political strategist's claim warranted?
A) No, because the test value -1.01 is in the noncritical region.
B) No, because the test value -2.22 is in the critical region.
C) Yes, because the test value -2.22 is in the noncritical region.
D) Yes, because the test value -1.71 is in the noncritical region.
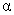 = .05, is the political strategist's claim warranted?
= .05, is the political strategist's claim warranted?A) No, because the test value -1.01 is in the noncritical region.
B) No, because the test value -2.22 is in the critical region.
C) Yes, because the test value -2.22 is in the noncritical region.
D) Yes, because the test value -1.71 is in the noncritical region.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
62
The formula for the test value in a z test for proportions is __________.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
63
The χ2 critical value for n = 16 and α = 0.01 for a left-tailed test is 5.812.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
64
A statistician claims that the standard deviation of the weights of firemen is less than 25 pounds. A sample of 20 randomly chosen firemen had a standard deviation of their weights of 26.2 pounds. Assume the variable is normally distributed. At α = 0.05, what is the critical value χ2 for this test?
A) 31.410
B) 30.144
C) 10.851
D) 10.117
A) 31.410
B) 30.144
C) 10.851
D) 10.117

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
65
It has been claimed that at UCLA at least 40% of the students live on campus. In a random sample of 250 students, 90 were found to live on campus. Does the evidence support the claim at 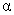 = .01?
= .01?
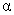 = .01?
= .01?
Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
66
A doctor claims that the standard deviation of systolic blood pressure is 12 mmHg. A random sample of 24 patients found a standard deviation of 14 mmHg. Assume the variable is normally distributed. At α = 0.01, what are the critical χ2 values?
A) 9.262 and 44.181
B) 13.091 and 35.172
C) 13.848 and 36.415
D) 11.524 and 44.314
A) 9.262 and 44.181
B) 13.091 and 35.172
C) 13.848 and 36.415
D) 11.524 and 44.314

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
67
Assume that a 99% confidence interval for the mean is  . The null hypothesis
. The null hypothesis 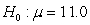 at
at 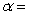 0.01 would
0.01 would
A) not be rejected because 11.0 is less than 15.0.
B) not be rejected because 11.0 is between 9.0 and 15.0.
C) be rejected because 11.0 is more than 9.0.
D) be rejected because 11.0 is not equal to 12.00.
 . The null hypothesis
. The null hypothesis 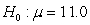 at
at 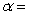 0.01 would
0.01 wouldA) not be rejected because 11.0 is less than 15.0.
B) not be rejected because 11.0 is between 9.0 and 15.0.
C) be rejected because 11.0 is more than 9.0.
D) be rejected because 11.0 is not equal to 12.00.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
68
Assume that a 90% confidence interval for the mean is 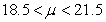 . The null hypothesis
. The null hypothesis 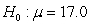 at
at 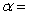 0.10 would
0.10 would
A) be rejected because 17.0 is less than 18.5.
B) not be rejected because 17.0 is less than 21.5.
C) not be rejected because 21.5 is more than 18.5.
D) be rejected because 17.0 is not equal equal to 20.0.
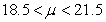 . The null hypothesis
. The null hypothesis 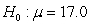 at
at 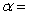 0.10 would
0.10 wouldA) be rejected because 17.0 is less than 18.5.
B) not be rejected because 17.0 is less than 21.5.
C) not be rejected because 21.5 is more than 18.5.
D) be rejected because 17.0 is not equal equal to 20.0.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
69
A lab technician is tested for her consistency by making multiple measurements of the cholesterol level in one blood sample. The target precision is a standard deviation of 1.4 mg/dL or less. If 16 measurements are taken and the standard deviation is 2.1 mg/dL, is there enough evidence to support the claim that her standard deviation is greater than the target, at 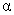 = .01?
= .01?
A) Yes, since the χ2 test value 33.750 is greater than the critical value 32.000.
B) No, since the χ2 test value 22.50 is less than the critical value 30.578.
C) Yes, since the χ2 test value 33.750 is greater than the critical value 30.578.
D) No, since the χ2 test value 22.50 is less than the critical value 32.000.
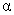 = .01?
= .01?A) Yes, since the χ2 test value 33.750 is greater than the critical value 32.000.
B) No, since the χ2 test value 22.50 is less than the critical value 30.578.
C) Yes, since the χ2 test value 33.750 is greater than the critical value 30.578.
D) No, since the χ2 test value 22.50 is less than the critical value 32.000.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
70
A scientist claims that only 65% of geese in his area fly south for the winter. He tags 55 random geese in the summer and finds that 17 of them do not fly south in the winter. If 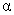 = .05, is the scientist's belief warranted?
= .05, is the scientist's belief warranted?
A) Yes, because the test value 0.64 is in the noncritical region.
B) No, because the test value 0.70 is in the critical region.
C) Yes, because the test value -0.70 is in the noncritical region.
D) No, because the test value -0.64 is in the noncritical region.
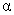 = .05, is the scientist's belief warranted?
= .05, is the scientist's belief warranted?A) Yes, because the test value 0.64 is in the noncritical region.
B) No, because the test value 0.70 is in the critical region.
C) Yes, because the test value -0.70 is in the noncritical region.
D) No, because the test value -0.64 is in the noncritical region.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
71
A type I error is only a possibility when the null hypothesis is not rejected.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
72
If the null hypothesis 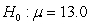 is rejected at
is rejected at 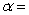 0.05 when a mean of 15.0 is obtained from a random sample, one could also say that
0.05 when a mean of 15.0 is obtained from a random sample, one could also say that
A) the 5% confidence interval for the population mean contains the value 15.0.
B) the 95% confidence interval for the population mean contains the value 15.0.
C) the 95% confidence interval for the population mean does not contain the value 13.0.
D) the 5% confidence interval for the population mean does not contain the value 13.0.
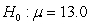 is rejected at
is rejected at 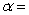 0.05 when a mean of 15.0 is obtained from a random sample, one could also say that
0.05 when a mean of 15.0 is obtained from a random sample, one could also say thatA) the 5% confidence interval for the population mean contains the value 15.0.
B) the 95% confidence interval for the population mean contains the value 15.0.
C) the 95% confidence interval for the population mean does not contain the value 13.0.
D) the 5% confidence interval for the population mean does not contain the value 13.0.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
73
A lumber mill is tested for consistency by measuring the variance of board thickness. The target accuracy is a variance of 0.0035 square inches or less. If 30 measurements are made and their variance is 0.006 square inches, is there enough evidence to reject the claim that the standard deviation is within the limit at 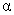 = .01?
= .01?
A) Yes, since the χ2 test value 49.71 is greater than the critical value 49.588.
B) No, since the χ2 test value 9.23 is less than the critical value 49.588.
C) Yes, since the χ2 test value 49.71 is less than the critical value 50.892.
D) No, since the χ2 test value 9.23 is less than the critical value 50.892.
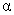 = .01?
= .01?A) Yes, since the χ2 test value 49.71 is greater than the critical value 49.588.
B) No, since the χ2 test value 9.23 is less than the critical value 49.588.
C) Yes, since the χ2 test value 49.71 is less than the critical value 50.892.
D) No, since the χ2 test value 9.23 is less than the critical value 50.892.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
74
A random sample of 450 shoppers at Quincy Mall found that 125 favored longer shopping hours. Is this sufficient evidence at the 0.05 level of significance to conclude that less than 30% of the shoppers at Quincy Mall favor longer hours?

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
75
The probability of committing a type II error is denoted as β.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
76
At a certain university, the average attendance at basketball games has been 3225. Due to the dismal showing of the team this year, the attendance for the first 12 games has averaged only 2865 with a standard deviation of 735. The athletic director claims that the attendance is the same as last year. What is the test value needed to evaluate the claim?
A) -1.70
B) -3.79
C) -4.48
D) -5.88
A) -1.70
B) -3.79
C) -4.48
D) -5.88

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
77
A machine fills 12-ounce bottles with soda. For the machine to function properly, the standard deviation of the sample must be less than or equal to 0.02 ounce. A sample of 8 bottles is selected, and the number of ounces of soda in each bottle is given. At  , can you reject the claim that the machine is functioning properly? Justify your answer. (Assume that the variables are approximately normally distributed.)
, can you reject the claim that the machine is functioning properly? Justify your answer. (Assume that the variables are approximately normally distributed.) 
A)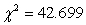 ,
, 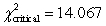 ; There is evidence to reject the claim that the machine is working properly.
; There is evidence to reject the claim that the machine is working properly.
B)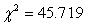 ,
,  ; There is evidence to reject the claim that the machine is working properly.
; There is evidence to reject the claim that the machine is working properly.
C)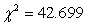 ,
, 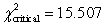 ; There is not enough evidence to reject the claim that the machine is working properly.
; There is not enough evidence to reject the claim that the machine is working properly.
D) ,
, 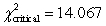 ; There is evidence to reject the claim that the machine is working properly.
; There is evidence to reject the claim that the machine is working properly.
 , can you reject the claim that the machine is functioning properly? Justify your answer. (Assume that the variables are approximately normally distributed.)
, can you reject the claim that the machine is functioning properly? Justify your answer. (Assume that the variables are approximately normally distributed.) 
A)
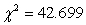 ,
, 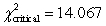 ; There is evidence to reject the claim that the machine is working properly.
; There is evidence to reject the claim that the machine is working properly.B)
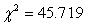 ,
,  ; There is evidence to reject the claim that the machine is working properly.
; There is evidence to reject the claim that the machine is working properly.C)
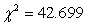 ,
, 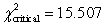 ; There is not enough evidence to reject the claim that the machine is working properly.
; There is not enough evidence to reject the claim that the machine is working properly.D)
 ,
, 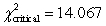 ; There is evidence to reject the claim that the machine is working properly.
; There is evidence to reject the claim that the machine is working properly.
Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
78
Using Table G, find the P-value interval for the 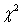 test value.
test value. 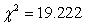 , n = 11, right-tailed
, n = 11, right-tailed
A) 0.05 < P-value < 0.10
B) 0.1 < P-value < 0.20
C) 0.025 < P-value < 0.05
D) 0.90 < P-value < 0.95
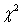 test value.
test value. 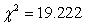 , n = 11, right-tailed
, n = 11, right-tailedA) 0.05 < P-value < 0.10
B) 0.1 < P-value < 0.20
C) 0.025 < P-value < 0.05
D) 0.90 < P-value < 0.95

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
79
If the null hypothesis 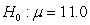 is not rejected at
is not rejected at 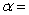 0.01 when a mean of 7.0 is obtained from a random sample, one could also say that
0.01 when a mean of 7.0 is obtained from a random sample, one could also say that
A) the 1% confidence interval for the population mean contains the value 7.0.
B) the 99% confidence interval for the population mean contains the value 11.0.
C) the 99% confidence interval for the population mean does not contain the value 11.0.
D) the 1% confidence interval for the population mean does not contain the value 11.0.
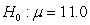 is not rejected at
is not rejected at 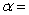 0.01 when a mean of 7.0 is obtained from a random sample, one could also say that
0.01 when a mean of 7.0 is obtained from a random sample, one could also say thatA) the 1% confidence interval for the population mean contains the value 7.0.
B) the 99% confidence interval for the population mean contains the value 11.0.
C) the 99% confidence interval for the population mean does not contain the value 11.0.
D) the 1% confidence interval for the population mean does not contain the value 11.0.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck
80
Doctors nationally believe that 74% of a certain type of operation are successful. In a particular hospital, 57 of these operations were observed and 47 of them were successful. At 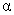 = .05, is this hospital's success rate different from the national average?
= .05, is this hospital's success rate different from the national average?
A) No, because the test value 0.86 is in the noncritical region.
B) Yes, because the test value 0.86 is in the critical region.
C) No, because the test value 1.46 is in the noncritical region.
D) Yes, because the test value 1.46 is in the noncritical region.
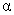 = .05, is this hospital's success rate different from the national average?
= .05, is this hospital's success rate different from the national average?A) No, because the test value 0.86 is in the noncritical region.
B) Yes, because the test value 0.86 is in the critical region.
C) No, because the test value 1.46 is in the noncritical region.
D) Yes, because the test value 1.46 is in the noncritical region.

Unlock Deck
Unlock for access to all 83 flashcards in this deck.
Unlock Deck
k this deck


