Deck 8: Probability: the Mathematics of Chance
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/108
Play
Full screen (f)
Deck 8: Probability: the Mathematics of Chance
1
Suppose a trial consists of rolling two dice and reporting the sum of the numbers rolled. What are the possible outcomes? Are they equally likely?
Possible outcomes are 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, or 12. They are not equally likely.
2
A pizza can be made with any of the following toppings: cheese, pepperoni, sausage, mushrooms, anchovies, green peppers, or olives. How many different three-topping pizzas can be made? Doubling of any topping is not allowed.
35
3
We wish to make a spinner that will be numbered 1 to 4, but will have the probability of spinning a "1" be 0.5. Draw the face for such a spinner.
Answers may vary. Any spinner face which shows half the face labeled "1" is correct.
4
A pair of dice is rolled. Sam says there are 36 outcomes in the sample space for this procedure and Sally says there are 11 outcomes in the sample space. Explain how they could both be correct.

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
5
A spinner with regions numbered 1 to 4 is spun and a coin is tossed. Both the region number and heads or tails is recorded. Write the sample space.

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
6
A pizza can be made with any of the following toppings: cheese, pepperoni, sausage, mushrooms, anchovies, green peppers, or olives. Doubling of any topping is not allowed. What is the probability that a randomly created three-topping pizza will contain mushrooms?

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
7
Three coins are flipped simultaneously and it is recorded whether each coin lands heads or tails. List the sample space.

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
8
Suppose a game has four outcomes: A, B, C, and D. The probability of outcome A is 0.4, the probabilities of each of the other outcomes is 0.2. A player receives $2 if outcome A occurs, $3 if outcome B occurs, $1 if outcome C occurs, and must pay $5 if outcome D occurs. What is the mean value of one trial of this game?

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
9
You roll two dice and record the sum shown. What is the probability of the event "the sum is greater than 2"?

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
10
If the probability that Kerry gets an A in English class is 0.82, what is the probability that Kerry does not get an A?

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
11
A student is taking a five-question true/false test. If the student chooses answers at random, what is the probability of getting all questions correct?

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
12
Below is a probability model for the number of automobiles owned by a randomly chosen family in a large town. What is the mean number of automobiles owned? 


Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
13
Suppose a trial consists of rolling a single die and reporting the number that is rolled. What are the possible outcomes? Are they equally likely?

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
14
A license plate code consists of two letters followed by three digits. The letters cannot repeat, but the digits can. What is the probability that a randomly chosen plate has all three digits the same?

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
15
The mean value of one trial of a carnival game is −$.05. Explain what this means.

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
16
We must create a license plate code that consists of two letters followed by three digits. The letters cannot repeat, but the digits may. How many such license plate codes can be formed?

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
17
Exactly one of three contestants will win a game show. The probability that Terry wins is 0.25 and the probability that Chris wins is 0.65. What is the probability that Toni, the remaining contestant wins?

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
18
A pair of fair dice is rolled and the sum of the faces showing is recorded. What is the probability of getting a sum greater than 9?

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
19
Find the probability of drawing a three or a heart from a regular (bridge) deck of cards. (Such a deck consists of four suits of 13 cards each. The suits are hearts, spades, diamonds, and clubs. The cards are 1 through 10, Jack, Queen, and King.)

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
20
Suppose three fair coins are tossed and the number of heads that appear is recorded. What is the probability of getting exactly two heads?

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
21
For a sample space {2,3,4,5,6}, two events are defined as follows:
Event A: A chosen number is greater than 4
Event B: A chosen number is a prime number; that is, 2, 3, or 5
Are those events A and B dependent or independent?
Event A: A chosen number is greater than 4
Event B: A chosen number is a prime number; that is, 2, 3, or 5
Are those events A and B dependent or independent?

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
22
Suppose a trial consists of rolling two dice and reporting the smaller of the two numbers rolled. What are the possible outcomes? Are they equally likely?

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
23
A game consists of tossing a coin and rolling a six-sided die. The results can be recorded easily; for example, if heads shows on the coin and a 4 shows on the die, you can record this as H4. List the sample space for the results of this game.

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
24
How many possible ways are there for drawing three spades from a regular (bridge) deck of cards?

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
25
A spinner numbered 1 through 10 is spun and one die is tossed simultaneously. The number spun and the number rolled are recorded. How many outcomes are in the sample space?
A) 60
B) 16
C) 10
D) 6
A) 60
B) 16
C) 10
D) 6

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
26
What is the probability of drawing three cards that are all spades from a regular (bridge) deck of cards?

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
27
Suppose you use a spinner to choose a random number between 0 and 1. Which is more likely-choosing a number between 1/3 and 2/3 or between 1/2 and 3/4?

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
28
Three dice are tossed. The number rolled on each die is recorded. How many outcomes are in the sample space?
A) 18
B) 72
C) 216
D) 42
A) 18
B) 72
C) 216
D) 42

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
29
A continuous probability model has a constant density curve on the range 0 to 12. What is the height of the density curve?

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
30
A spinner numbered 1 through 10 is spun and one die is rolled simultaneously. The sum of the number spun and the number rolled is recorded. How many outcomes are in the sample space?
A) 60
B) 16
C) 15
D) 10
A) 60
B) 16
C) 15
D) 10

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
31
A game consists of tossing three coins. Find the probability model for the number of coins showing heads.

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
32
For a sample space {1,2,3,4,5,6,7,8}, two events are defined as follows:
Event A: A chosen number is greater than 4
Event B: A chosen number is a prime number; that is, 2, 3, 5, or 7
Are those events A and B dependent or independent?
Event A: A chosen number is greater than 4
Event B: A chosen number is a prime number; that is, 2, 3, 5, or 7
Are those events A and B dependent or independent?

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
33
A die is rolled and a coin is flipped simultaneously. The number rolled on the die and whether the coin lands heads or tails is recorded. How many outcomes are in the sample space?
A) 8
B) 6
C) 10
D) 12
A) 8
B) 6
C) 10
D) 12

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
34
According to the central limit theorem, how does the standard deviation of averages over four observations compare with the standard deviation of individual observations?

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
35
Two coins are flipped at the same time and heads or tails is recorded. The sample space for this is:
A) {H, T}.
B) {HH, HT, TT}.
C) {HH, HT, TH, TT}.
D) {H, H, T, T}.
A) {H, T}.
B) {HH, HT, TT}.
C) {HH, HT, TH, TT}.
D) {H, H, T, T}.

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
36
A football stadium snack bar conducted a survey and found that 30 customers bought one item, 52 customers bought two items, 21 customers bought three items, and 12 customers bought four items. What is the mean number of items purchased per customer? Round your answer to the nearest tenth.

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
37
The mean weight of a collection of potatoes in a shipment to a fruit market is 1.3 pounds, with a standard deviation of 0.35 pounds. The distribution of weights is approximately Normal. What is the probability that one potato chosen at random will weigh more than 1.65 pounds?

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
38
In the manufacturing process for ball bearings, the mean diameter is 5 mm with a standard deviation of 0.002 mm. Every hour a sample of 20 bearings is drawn, measured, and the mean diameter of the sample found. What is the standard deviation x of the sample mean?

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
39
The mean weight of a collection of potatoes in a shipment to a fruit market is 1.3 pounds, with a standard deviation of 0.35 pounds. The distribution of weights is approximately Normal. What fraction of the potatoes in the shipment will weigh between 0.25 and 2.35 pounds?

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
40
In the manufacturing process for ball bearings, the mean diameter is 5 mm with a standard deviation of 0.002 mm. Between what two measurements will 95% of all diameters of ball bearings be found?

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
41
Two coins are flipped and the number that landed on heads is recorded. The outcomes in the sample space are all equally likely.

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
42
Use the following to answer
You roll two dice and record the sum shown. The sample space is the set of all possible sums, which are 2, 3, . . . , 11, 12.
What is the complement of the event "the sum is less than 6 or greater than 7"?
A) The outcome is 6.
B) The outcome is 6 or 7.
C) The outcome is less than 7.
D) The outcome is 6 or larger.
You roll two dice and record the sum shown. The sample space is the set of all possible sums, which are 2, 3, . . . , 11, 12.
What is the complement of the event "the sum is less than 6 or greater than 7"?
A) The outcome is 6.
B) The outcome is 6 or 7.
C) The outcome is less than 7.
D) The outcome is 6 or larger.

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
43
Use the following to answer
You roll two dice and record the sum shown. The sample space is the set of all possible sums, which are 2, 3, . . . , 11, 12.
The probability of the event "the sum is 5" is:
A) 1/6.
B) 1/8.
C) 1/9.
D) 1/12.
You roll two dice and record the sum shown. The sample space is the set of all possible sums, which are 2, 3, . . . , 11, 12.
The probability of the event "the sum is 5" is:
A) 1/6.
B) 1/8.
C) 1/9.
D) 1/12.

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
44
If you roll two dice and record their sum, the events "even" and "less than or equal to 6" are:
A) disjoint.
B) independent.
C) Both A and B is correct.
D) Neither A nor B is correct.
A) disjoint.
B) independent.
C) Both A and B is correct.
D) Neither A nor B is correct.

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
45
If you randomly select a committee of two from a group of two men and two women, what is the probability that the group is all female?
A) 1/2
B) 1/4
C) 1/6
D) 1/12
A) 1/2
B) 1/4
C) 1/6
D) 1/12

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
46
To function correctly, each of two parts of a circuit must work. If an event denotes that the circuit functions correctly, what is the complement of the event?
A) Exactly one of two parts is defective.
B) At least one of two parts is defective.
C)
D) Both parts are defective.
None of parts is defective.
A) Exactly one of two parts is defective.
B) At least one of two parts is defective.
C)
D) Both parts are defective.
None of parts is defective.

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
47
If a fair die is rolled once, what is the probability of getting a number less than five?
A) 1/6
B) 1/2
C) 2/3
D) 5/6
A) 1/6
B) 1/2
C) 2/3
D) 5/6

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
48
Three dice are rolled and the number rolled on each die is recorded. The outcomes in the sample space are all equally likely.

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
49
Use the following to answer
You roll two dice and record the sum shown. The sample space is the set of all possible sums, which are 2, 3, . . . , 11, 12.
Are the 11 outcomes equally likely?
A) Yes
B) No
You roll two dice and record the sum shown. The sample space is the set of all possible sums, which are 2, 3, . . . , 11, 12.
Are the 11 outcomes equally likely?
A) Yes
B) No

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
50
Three dice are tossed. The sum of the numbers rolled is recorded. How many outcomes are in the sample space?
A) 18
B) 12
C) 16
D) 72
A) 18
B) 12
C) 16
D) 72

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
51
A fair coin is tossed three times. Find the probability of getting exactly two heads.
A) 1/2
B) 1/3
C) 2/3
D) 3/8
A) 1/2
B) 1/3
C) 2/3
D) 3/8

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
52
Use the following to answer
You roll two dice and record the sum shown. The sample space is the set of all possible sums, which are 2, 3, . . . , 11, 12.
What other outcome has a probability equal to the probability of "the sum is 2"?
A) The sum is greater than 2.
B) The sum is less than 10.
C) The sum is greater than 11.
D) None of the above.
You roll two dice and record the sum shown. The sample space is the set of all possible sums, which are 2, 3, . . . , 11, 12.
What other outcome has a probability equal to the probability of "the sum is 2"?
A) The sum is greater than 2.
B) The sum is less than 10.
C) The sum is greater than 11.
D) None of the above.

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
53
You use a random number generator that produces the digits 1 through 9 with equal probability. You draw four digits and make them into a number; for example, 0, 2, 4, 4 would be the number 0244 or just 244. What is the probability of getting a number strictly greater than 1000?
A) 1/10
B) 1001/10,000
C) 1000/9999
D) None of the above.
A) 1/10
B) 1001/10,000
C) 1000/9999
D) None of the above.

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
54
Two fair dice are rolled and the sum rolled is recorded. Find the probability that the sum is 4.
A) 1/3
B) 1/12
C) 4/11
D) 1/9
A) 1/3
B) 1/12
C) 4/11
D) 1/9

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
55
A computer is programmed to randomly print two letters in a row without repeating a letter. What is the probability that the first combination printed is the word "DO"?
A) 1/650
B) 1/325
C) 2/325
D) 1/26
A) 1/650
B) 1/325
C) 2/325
D) 1/26

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
56
Three dice are rolled and the sum of the numbers rolled is recorded. The outcomes in the sample space are all equally likely.

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
57
There are seven blue and six black socks in a drawer. One is pulled out at random. Find the probability that it is black.
A) 6/13
B) 6/7
C) 1/2
D) 1/6
A) 6/13
B) 6/7
C) 1/2
D) 1/6

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
58
We need to create three-digit code numbers that must begin with a 7. How many such codes can be made?
A) 700
B) 107
C) 100
D) 27
A) 700
B) 107
C) 100
D) 27

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
59
Two coins are flipped and heads or tails is recorded. The outcomes in the sample space are all equally likely.

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
60
Two dice are rolled and the sum is recorded. What sis the most likely outcome?
A) 7
B) 8
C) 9
D) 12
A) 7
B) 8
C) 9
D) 12

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
61
For a casino game, you pay $4 and roll a die. The casino keeps your $4 but pays you back the number of dollars you roll. (Roll a 6 and you get $6.) Your average loss per bet is:
A) $0.
B) $0.50.
C) $1.
D) $4.
A) $0.
B) $0.50.
C) $1.
D) $4.

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
62
If two events A and B have probabilities as P(A) = 0.45 and P(B) = 0.7, then what is the smallest possible value for P(A and
A) 0.1
B) 0.15
B)?
C) 0.3
D) 0.35
A) 0.1
B) 0.15
B)?
C) 0.3
D) 0.35

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
63
A fair die is rolled. If a number 1 or 2 appears, you will receive $5. If any other number appears, you will pay $2. What is the mean value of one trial of this game?
A) $1/3
B) $3
C) $3/2
D) −$3
A) $1/3
B) $3
C) $3/2
D) −$3

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
64
Use the following information for Questions
A random number generator is modeled by a uniform probability model with a density curve that has height 1/10 between 0 and 10 and height 0 everywhere else.
For this model, what is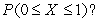
A) 0
B) 1/2
C) 1
D) None of the above.
A random number generator is modeled by a uniform probability model with a density curve that has height 1/10 between 0 and 10 and height 0 everywhere else.
For this model, what is
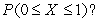
A) 0
B) 1/2
C) 1
D) None of the above.

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
65
Use the following information for Questions
A random number generator is modeled by a uniform probability model with a density curve that has height 1/10 between 0 and 10 and height 0 everywhere else.
For this model, what is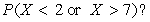
A) 0
B) 1/2
C) 1
D) None of the above .
A random number generator is modeled by a uniform probability model with a density curve that has height 1/10 between 0 and 10 and height 0 everywhere else.
For this model, what is
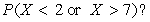
A) 0
B) 1/2
C) 1
D) None of the above .

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
66
At a certain discount store, the number of people in checkout lines varies. The probability model for the number of people in a randomly chosen line is:  What is the mean number of people in a line?
What is the mean number of people in a line?
A) 2.5
B) 15.92
C) 2.81
D) 2.89
 What is the mean number of people in a line?
What is the mean number of people in a line?A) 2.5
B) 15.92
C) 2.81
D) 2.89

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
67
Suppose a game has three outcomes, A, B, and C with probabilities P(A) = 0.2, P(B) = 0.3, and P(C) = 0.5. A player will receive $3 when outcome A occurs, $4 when outcome B occurs, and will have to pay $2 when outcome C occurs. What is the mean value of one trial of the game?
A) $0.80
B) $1.66
C) $1.00
D) $5.00
A) $0.80
B) $1.66
C) $1.00
D) $5.00

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
68
The Olympic flag consists of five intertwined circles, one in each of the colors black, blue, green, red, and yellow. What is the probability that a random coloring of the five circles using these colors will produce the exact match of the Olympic symbol?
A) 1/5
B) 1/3125
C) 5/120
D) 1/120
A) 1/5
B) 1/3125
C) 5/120
D) 1/120

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
69
A continuous probability model has the following density curve: 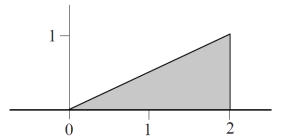 What is
What is 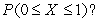
A) 1/8
B) 1/4
C) 1/2
D) None of the above.
 What is
What is 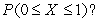
A) 1/8
B) 1/4
C) 1/2
D) None of the above.

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
70
We need to create serial numbers that start with one of the letters a, b, c, d, or f followed by three nonrepeating digits. How many serial numbers can be created?
A) 5000
B) 2520
C) 725
D) 3600
A) 5000
B) 2520
C) 725
D) 3600

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
71
The marketing department of an electronics manufacturer has done research on the number of television sets owned by families in a large town. The probability model for the number of sets owned by a randomly chosen family is given below. What is the mean number of sets per family? 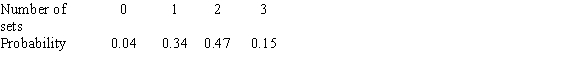
A) 0.96
B) 1.5
C) 1.77
D) 1.73

A) 0.96
B) 1.5
C) 1.77
D) 1.73

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
72
A sample space consists of three outcomes, X, Y, and Z. Which of the following could be a legitimate assignment of probabilities to the outcomes?
A)
B)
C)
D)
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
73
Use the following information for Questions
A random number generator is modeled by a uniform probability model with a density curve that has height 1/10 between 0 and 10 and height 0 everywhere else.
For this model, what is
A) 0
B) 1/2
C) 1
D) None of the above.
A random number generator is modeled by a uniform probability model with a density curve that has height 1/10 between 0 and 10 and height 0 everywhere else.
For this model, what is

A) 0
B) 1/2
C) 1
D) None of the above.

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
74
A computer system requires users to have an access code that consists of a three-digit number that is not allowed to start with zero and cannot repeat digits. How many such codes are possible?
A) 990
B) 648
C) 729
D) 720
A) 990
B) 648
C) 729
D) 720

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
75
A raffle ticket costs $5. First-prize and second-prize winners will be drawn at random. The probability of winning the $100 first prize is 1/40, and the probability of winning the $25 second prize is 1/20. What is the mean winnings for one play, taking into account the $5 cost of the ticket?
A) −$1.25
B) −$0.875
C) $3.375
D) $3.75
A) −$1.25
B) −$0.875
C) $3.375
D) $3.75

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
76
We need to create code words that use three letters of the alphabet. Repeating of letters is allowed. How many code words can be created?
A) 17,576
B) 15,600
C) 78
D) 75
A) 17,576
B) 15,600
C) 78
D) 75

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
77
Exactly one of Terry, Chris, and Kim will attend a party. The probability Terry attends is 0.31 and the probability Chris attends is 0.5. What is the probability that Kim attends?
A) 0.33
B) 0.5
C) 0.81
D) 0.19
A) 0.33
B) 0.5
C) 0.81
D) 0.19

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
78
Use the following information for Questions
A random number generator is modeled by a uniform probability model with a density curve that has height 1/10 between 0 and 10 and height 0 everywhere else.
For this model, what is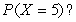
A) 0
B) 1/2
C) 1
D) None of the above.
A random number generator is modeled by a uniform probability model with a density curve that has height 1/10 between 0 and 10 and height 0 everywhere else.
For this model, what is
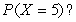
A) 0
B) 1/2
C) 1
D) None of the above.

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
79
A sample space contains three outcomes, A, B, and C. Which of the following could be a legitimate assignment of probabilities to the outcomes?
A)
B)
C)
D)
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck
80
If there is a 0.8 probability of rain today, what is the probability it will not rain?
A) 0.8
B) 0.5
C) 0.2
D) 0.1
A) 0.8
B) 0.5
C) 0.2
D) 0.1

Unlock Deck
Unlock for access to all 108 flashcards in this deck.
Unlock Deck
k this deck


