Deck 6: Discrete Probability Distributions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/114
Play
Full screen (f)
Deck 6: Discrete Probability Distributions
1
The variance of a binomial distribution is found by nπ (1 - π).
True
2
The probability of a particular outcome must always be between 0.0 and 1.0 inclusive.
True
3
The variance measures the skewness of a probability distribution.
False
4
The probability distribution for the number of automobiles lined up at a Lakeside Olds at opening time (7:30 a.m.) for service is: 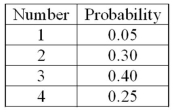 On a typical day, how many automobiles should Lakeside Olds expect to be lined up at opening time?
On a typical day, how many automobiles should Lakeside Olds expect to be lined up at opening time?
A)10.00
B)1.00
C)2.85
D)1.96
 On a typical day, how many automobiles should Lakeside Olds expect to be lined up at opening time?
On a typical day, how many automobiles should Lakeside Olds expect to be lined up at opening time?A)10.00
B)1.00
C)2.85
D)1.96

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
5
To construct a binomial probability distribution, the mean must be known.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
6
Sweetwater & Associates write weekend trip insurance at a very nominal charge. Records show that the probability that a motorist will have an accident during the weekend and file a claim is 0.0005. Suppose they wrote 400 policies for the coming weekend, what is the probability that exactly two claims will be filed?
A)0.8187
B)0.2500
C)0.0164
D)0.0001
A)0.8187
B)0.2500
C)0.0164
D)0.0001

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
7
In a Poisson distribution, the probability of success may vary from trial to trial.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
8
To construct a binomial distribution, it is necessary to know the total number of trials and the probability of success on each trial.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
9
The mean of a binomial distribution is the product of n and π.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
10
On a very hot summer day, 5% of the production employees at Midland States Steel are absent from work. The production employees are randomly selected for a special in-depth study on absenteeism. What is the probability of randomly selecting 10 production employees on a hot summer day and finding that none of them are absent?
A)0.002
B)0.344
C)0.599
D)0.100
A)0.002
B)0.344
C)0.599
D)0.100

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
11
A random variable represents the outcome of an experiment.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
12
A probability distribution is a mutually exclusive and collectively exhaustive listing of experimental outcomes that can occur by chance, and their corresponding probabilities.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
13
A listing of all possible outcomes of an experiment and their corresponding probabilities of occurrence is called a ____________.
A)Random variable
B)Probability distribution
C)Subjective probability
D)Frequency distribution
A)Random variable
B)Probability distribution
C)Subjective probability
D)Frequency distribution

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
14
A total of 60% of the customers of a fast food chain order a hamburger, French fries, and a drink. If a random sample of 15 cash register receipts is selected, what is the probability that 10 or more will show that the above three food items were ordered?
A)1.000
B)0.186
C)0.403
D)0.000
A)1.000
B)0.186
C)0.403
D)0.000

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
15
Judging from recent experience, 5% of the computer keyboards produced by an automatic, high-speed machine are defective. If six keyboards are randomly selected, what is the probability that none of the keyboards are defective?
A)0.001
B)0.167
C)0.735
D)0.500
A)0.001
B)0.167
C)0.735
D)0.500

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
16
The binomial probability distribution is always negatively skewed.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
17
The variance of a probability distribution is based on the sum or squared differences from the mean.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
18
The mean of a probability distribution is called its expected value.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
19
The random variable for a Poisson probability distribution can assume an infinite number of values.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
20
If the variance is 3.6 grams, what is the standard deviation?
A)0.600
B)1.897
C)6.000
D)12.96
A)0.600
B)1.897
C)6.000
D)12.96

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
21
A farmer who grows genetically engineered corn is experiencing trouble with corn borers. A random check of 5,000 ears revealed the following: Many of the ears contained no borers. Some ears had one borer; A few had two borers, and so on. The distribution of the number of borers per ear approximated the Poisson distribution. The farmer counted 3,500 borers in the 5,000 ears. What is the probability that an ear of corn selected at random will contain no borers?
A)0.3476
B)0.4966
C)1.000
D)0.0631
A)0.3476
B)0.4966
C)1.000
D)0.0631

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
22
The production department has installed a new spray machine to paint automobile doors. As is common with most spray guns, unsightly blemishes often appear because of improper mixture or other problems. A worker counted the number of blemishes on each door. Most doors had no blemishes; a few had one; a very few had two; and so on. The average number was 0.5 per door. The distribution of blemishes followed the Poisson distribution. Out of 10,000 doors painted, about how many would have no blemishes?
A)About 6,065
B)About 3,935
C)About 5,000
D)About 500
A)About 6,065
B)About 3,935
C)About 5,000
D)About 500

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
23
In a large metropolitan area, past records revealed that 30% of all the high school graduates go to college. From 20 graduates selected at random, what is the probability that exactly 8 will go to college?
A)0.114
B)0.887
C)0.400
D)0.231
A)0.114
B)0.887
C)0.400
D)0.231

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
24
Which shape describes a Poisson distribution?
A)Positively skewed
B)Negatively skewed
C)Symmetrical
D)All apply
A)Positively skewed
B)Negatively skewed
C)Symmetrical
D)All apply

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
25
A tennis match requires that a player win three of five sets to win the match. If a player wins the first two sets, what is the probability that the player wins the match, assuming that each player is equally likely to win each set?
A)0.500
B)0.125
C)0.875
D)0.000
A)0.500
B)0.125
C)0.875
D)0.000

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
26
A true/false test consists of six questions. If you guess the answer to each question, what is the probability of getting all six questions correct?
A)0
B)0.016
C)0.062
D)0.250
A)0
B)0.016
C)0.062
D)0.250

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
27
What is the only variable in the Poisson probability formula?
A)π
B)x
C)e
D)P
A)π
B)x
C)e
D)P

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
28
A coin is tossed four times. The following table summarizes the experiment. What is the following table called? 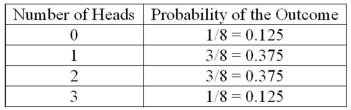
A)Probability distribution
B)Cumulative frequency distribution
C)Standard deviation
D)Frequency table

A)Probability distribution
B)Cumulative frequency distribution
C)Standard deviation
D)Frequency table

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
29
Data show that the weight of an offensive linesman may be any weight between 200 and 350 pounds. The distribution of weight is based on a ______________.
A)Continuous random variable
B)Discrete random variable
C)Qualitative variable
D)All apply
A)Continuous random variable
B)Discrete random variable
C)Qualitative variable
D)All apply

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
30
Carlson Jewelers permits the return of their diamond wedding rings, provided the return occurs within two weeks of the purchase date. Their records reveal that 10% of the diamond wedding rings are returned. Five different customers buy a wedding ring. What is the probability that none of the customers return a ring?
A)0.250
B)0.073
C)0.590
D)0.500
A)0.250
B)0.073
C)0.590
D)0.500

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
31
Which of the following is correct about a probability distribution?
A)The sum of all possible outcomes must equal 1.0.
B)Outcomes must be mutually exclusive.
C)The probability of each outcome must be between 0.0 and 1.0 inclusive.
D)All apply.
A)The sum of all possible outcomes must equal 1.0.
B)Outcomes must be mutually exclusive.
C)The probability of each outcome must be between 0.0 and 1.0 inclusive.
D)All apply.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
32
Chances are 50-50 that a newborn baby will be a girl. For families with five children, what is the probability that all the children are girls?
A)0.900
B)0.031
C)0.001
D)0.250
A)0.900
B)0.031
C)0.001
D)0.250

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
33
Which of the following is NOT a characteristic of a binomial probability distribution?
A)Each outcome is mutually exclusive.
B)Each trial is independent.
C)The probability of success remains constant from trial to trial.
D)The number of trials is limited to two.
A)Each outcome is mutually exclusive.
B)Each trial is independent.
C)The probability of success remains constant from trial to trial.
D)The number of trials is limited to two.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
34
Which is true for a binomial distribution?
A)There are ten or more possible outcomes.
B)The probability of success remains the same from trial to trial.
C)The value of π is equal to 1.50.
D)It approximates the Poisson distribution.
A)There are ten or more possible outcomes.
B)The probability of success remains the same from trial to trial.
C)The value of π is equal to 1.50.
D)It approximates the Poisson distribution.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
35
What kind of distributions are the binomial and Poisson probability distributions?
A)Discrete
B)Continuous
C)Both discrete and continuous
D)Neither discrete or continuous
A)Discrete
B)Continuous
C)Both discrete and continuous
D)Neither discrete or continuous

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
36
A machine shop has 100 drill presses and other machines in constant use. The probability that a machine will become inoperative during a given day is 0.002. During some days, no machines are inoperative, but during some days, one, two, three, or more are broken down. What is the probability that fewer than two machines will be inoperative during a particular day?
A)0.0200
B)0.1637
C)0.8187
D)0.9824
A)0.0200
B)0.1637
C)0.8187
D)0.9824

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
37
A new car was put into production. It involved many assembly tasks. Each car was inspected at the end of the assembly line and the number of defects per unit was recorded. For the first 100 cars produced, there were 40 defective cars. Some of the cars had no defects, a few had one defect, and so on. The distribution of defects followed a Poisson distribution. Based on the first 100 cars produced, about how many out of every 1,000 cars assembled should have one or more defects?
A)About 660
B)About 165
C)About 630
D)About 330
A)About 660
B)About 165
C)About 630
D)About 330

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
38
Sponsors of a local charity decided to attract wealthy patrons to its $500-a-plate dinner by allowing each patron to buy a set of 20 tickets for the gaming tables. The chance of winning a prize for each of the 20 plays is 50-50. If you bought 20 tickets, what is the chance of winning 15 or more prizes?
A)0.250
B)0.021
C)0.006
D)0.750
A)0.250
B)0.021
C)0.006
D)0.750

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
39
Which one of the following is NOT a condition of the binomial distribution?
A)Independent trials
B)Only two outcomes
C)The probability of success remains constant from trial to trial
D)Sampling at least 10 trials
A)Independent trials
B)Only two outcomes
C)The probability of success remains constant from trial to trial
D)Sampling at least 10 trials

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
40
A manufacturer of headache medicine claims it is 70% effective within a few minutes. That is, out of every 100 users, 70 get relief within a few minutes. A group of 12 patients are given the medicine. If the claim is true, what is the probability that eight have relief within a few minutes?
A)0.001
B)0.168
C)0.667
D)0.231
A)0.001
B)0.168
C)0.667
D)0.231

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
41
A company is studying the number of monthly absences among its 125 employees. The following probability distribution shows the likelihood that people were absent 0, 1, 2, 3, 4, or 5 days last month. 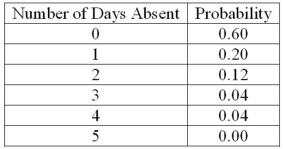 Given the probability distribution, which of the following predictions is correct?
Given the probability distribution, which of the following predictions is correct?
A)60% of the employees will have more than one day absent per month.
B)There is a 0.04 probability that an employee will be absent one day per month.
C)There is a 0.12 probability that an employee will be absent two days per month.
D)There is a 0.50 probability that an employee will be absent 0.72 days per month.
 Given the probability distribution, which of the following predictions is correct?
Given the probability distribution, which of the following predictions is correct?A)60% of the employees will have more than one day absent per month.
B)There is a 0.04 probability that an employee will be absent one day per month.
C)There is a 0.12 probability that an employee will be absent two days per month.
D)There is a 0.50 probability that an employee will be absent 0.72 days per month.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
42
In a Poisson distribution, the variance is equal to ___________.
A)nπ
B)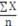
C)e-x
D)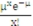
A)nπ
B)
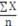
C)e-x
D)
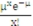

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
43
A statistics professor receives an average of five e-mail messages per day from students. Assume the number of messages approximates a Poisson distribution. What is the probability that on a randomly selected day she will have five messages?
A)0.0067
B)0.8750
C)0.1755
D)1.0000
A)0.0067
B)0.8750
C)0.1755
D)1.0000

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
44
For the following distribution: 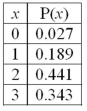 What is the variance of the distribution?
What is the variance of the distribution?
A)2.1
B)0.63
C)3.9
D)2.754
 What is the variance of the distribution?
What is the variance of the distribution?A)2.1
B)0.63
C)3.9
D)2.754

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
45
A company is studying the number of monthly absences among its 125 employees. The following probability distribution shows the likelihood that people were absent 0, 1, 2, 3, 4, or 5 days last month. 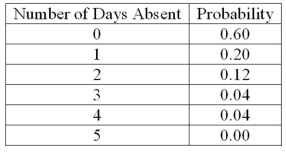 What is the variance of the number of days absent?
What is the variance of the number of days absent?
A)1.1616
B)1.41
C)5.00
D)55.52
 What is the variance of the number of days absent?
What is the variance of the number of days absent?A)1.1616
B)1.41
C)5.00
D)55.52

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
46
What must you know to develop a binomial probability distribution?
A)The probability of success
B)The probability of success and the number of trials
C)The probability of success and the number of successes
D)The number of trials and the number of successes
A)The probability of success
B)The probability of success and the number of trials
C)The probability of success and the number of successes
D)The number of trials and the number of successes

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
47
For the following distribution: 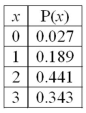 What is the mean of the distribution?
What is the mean of the distribution?
A)2.1
B)1.5
C)0.441
D)2
 What is the mean of the distribution?
What is the mean of the distribution?A)2.1
B)1.5
C)0.441
D)2

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
48
A statistics professor receives an average of five e-mail messages per day from students. Assume the number of messages approximates a Poisson distribution. What is the probability that on a randomly selected day she will have no messages?
A)0.0067
B)Zero
C)0.0335
D)Impossible to have no messages
A)0.0067
B)Zero
C)0.0335
D)Impossible to have no messages

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
49
For the following distribution: 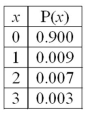 What is the mean of the distribution?
What is the mean of the distribution?
A)2.1
B)1.13
C)0.113
D)1.5
 What is the mean of the distribution?
What is the mean of the distribution?A)2.1
B)1.13
C)0.113
D)1.5

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
50
For the following distribution:  What is the mean of the distribution?
What is the mean of the distribution?
A)1
B)2.5
C)1.604
D)3
 What is the mean of the distribution?
What is the mean of the distribution?A)1
B)2.5
C)1.604
D)3

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
51
A company is studying the number of monthly absences among its 125 employees. The following probability distribution shows the likelihood that people were absent 0, 1, 2, 3, 4, or 5 days last month. 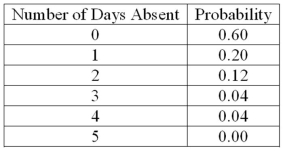 What is the standard deviation of the number of days absent?
What is the standard deviation of the number of days absent?
A)1.1616
B)0
C)1.6595
D)1.0778
 What is the standard deviation of the number of days absent?
What is the standard deviation of the number of days absent?A)1.1616
B)0
C)1.6595
D)1.0778

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
52
David's gasoline station offers 4 cents off per gallon if the customer pays in cash and does not use a credit card. Past evidence indicates that 40% of all customers pay in cash. During a one-hour period, 15 customers buy gasoline at this station. What is the probability that more than 8 and less than 12 customers pay in cash?
A)0.210
B)0.212
C)0.092
D)0.562
A)0.210
B)0.212
C)0.092
D)0.562

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
53
For the following distribution: 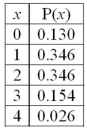 What is the variance of the distribution?
What is the variance of the distribution?
A)1.1616
B)0.964
C)0.982
D)1.000
 What is the variance of the distribution?
What is the variance of the distribution?A)1.1616
B)0.964
C)0.982
D)1.000

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
54
A statistics professor receives an average of five e-mail messages per day from students. Assume the number of messages approximates a Poisson distribution. What is the probability that on a randomly selected day she will have two messages?
A)0.0067
B)0.0014
C)0.4200
D)0.0842
A)0.0067
B)0.0014
C)0.4200
D)0.0842

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
55
David's gasoline station offers 4 cents off per gallon if the customer pays in cash and does not use a credit card. Past evidence indicates that 40% of all customers pay in cash. During a one-hour period, 15 customers buy gasoline at this station. What is the probability that at least 10 pay in cash?
A)0.024
B)0.033
C)0.009
D)0.976
A)0.024
B)0.033
C)0.009
D)0.976

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
56
A company is studying the number of monthly absences among its 125 employees. The following probability distribution shows the likelihood that people were absent 0, 1, 2, 3, 4, or 5 days last month. 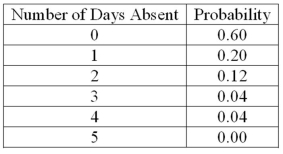 What is the mode of the distribution?
What is the mode of the distribution?
A)0.72 days
B)2.5 days
C)0 days
D)3 days
 What is the mode of the distribution?
What is the mode of the distribution?A)0.72 days
B)2.5 days
C)0 days
D)3 days

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
57
David's gasoline station offers 4 cents off per gallon if the customer pays in cash and does not use a credit card. Past evidence indicates that 40% of all customers pay in cash. During a one-hour period, 15 customers buy gasoline at this station. This situation is an example of what type of discrete probability distribution?
A)Continuous probability distribution
B)Poisson probability distribution
C)Binomial probability distribution
D)Hypergeometric probability distribution
A)Continuous probability distribution
B)Poisson probability distribution
C)Binomial probability distribution
D)Hypergeometric probability distribution

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
58
David's gasoline station offers 4 cents off per gallon if the customer pays in cash and does not use a credit card. Past evidence indicates that 40% of all customers pay in cash. During a one-hour period, 15 customers buy gasoline at this station. What is the probability that all 15 pay in cash?
A)0.0
B)0.1
C)0.9
D)1.0
A)0.0
B)0.1
C)0.9
D)1.0

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
59
A company is studying the number of monthly absences among its 125 employees. The following probability distribution shows the likelihood that people were absent 0, 1, 2, 3, 4, or 5 days last month. 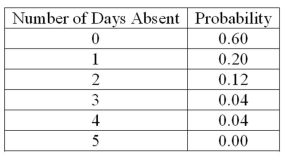 What is the mean number of days absent?
What is the mean number of days absent?
A)1.00
B)0.40
C)0.72
D)2.5
 What is the mean number of days absent?
What is the mean number of days absent?A)1.00
B)0.40
C)0.72
D)2.5

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
60
To apply a Poisson probability distribution, the mean can be computed as __________.
A)nπ
B)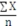
C)e-x
D)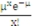
A)nπ
B)
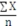
C)e-x
D)
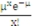

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
61
The following is a Poisson probability distribution with µ = 0.1.  The variance of the distribution is ______.
The variance of the distribution is ______.
A)1.0
B)0.9046
C)3.0
D)0.1
 The variance of the distribution is ______.
The variance of the distribution is ______.A)1.0
B)0.9046
C)3.0
D)0.1

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
62
A _______________ random variable can assume only a certain number of separated values.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
63
If π = 1/5 and n = 100, the standard deviation of this binomial distribution is _____.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
64
A probability distribution shows the outcomes of an experiment and the ___________________ of each one occurring.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
65
To construct a binomial distribution, we need to know the ___________ and the probability of a success.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
66
In a binomial experiment, the probability of a failure equals _________.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
67
A binomial probability distribution approaches a greater degree of symmetry as the probability of success remains constant and the number of trials becomes ___________.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
68
For a trial, the number of possible outcomes for the binomial experiment is _____.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
69
For the following distribution:  What is the variance of the distribution?
What is the variance of the distribution?
A)2.1
B)0.132
C)0.364
D)1.000
 What is the variance of the distribution?
What is the variance of the distribution?A)2.1
B)0.132
C)0.364
D)1.000

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
70
If n = 900 and π = ⅓, the variance of this binomial distribution is ______.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
71
The following is a Poisson probability distribution with µ = 0.1. 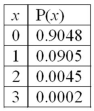 The mean of the distribution is _____.
The mean of the distribution is _____.
A)1.5
B)0.1
C)0.25
D)1.0
 The mean of the distribution is _____.
The mean of the distribution is _____.A)1.5
B)0.1
C)0.25
D)1.0

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
72
In a binomial experiment, the probability of a _________ remains constant.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
73
For a binomial distribution, the mean is 0.6 and n = 2. What is π for this distribution?
A)0.5
B)1.00
C)0.3
D)0.1
A)0.5
B)1.00
C)0.3
D)0.1

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
74
For any probability distribution, the mean is calculated as a weighted mean. The weights are the ______________.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
75
If π = 1/3 and n = 900, the mean of this binomial distribution is ______.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
76
The Poisson distribution or, the law of improbable events, is _______________ skewed.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
77
For a binomial distribution, the mean is 4.0 and n = 8. What is π for this distribution?
A)0.5
B)1.00
C)4.0
D)0.1
A)0.5
B)1.00
C)4.0
D)0.1

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
78
A probability distribution is a listing of the expected outcomes of an experiment and the probability of each outcome occurring. The sum of the probabilities is ______.

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
79
The following is a binomial probability distribution with n = 3 and π = 0.20. 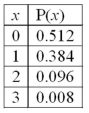 The mean of the distribution is _______.
The mean of the distribution is _______.
A)1.50
B)0.60
C)0.25
D)0.00
 The mean of the distribution is _______.
The mean of the distribution is _______.A)1.50
B)0.60
C)0.25
D)0.00

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck
80
The following is a binomial probability distribution with n = 3 and π = 0.20. 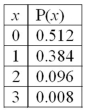 The variance of the distribution is _________.
The variance of the distribution is _________.
A)1.5
B)3.0
C)0.69
D)0.48
 The variance of the distribution is _________.
The variance of the distribution is _________.A)1.5
B)3.0
C)0.69
D)0.48

Unlock Deck
Unlock for access to all 114 flashcards in this deck.
Unlock Deck
k this deck


