Deck 12: Chi-Square Tests
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/120
Play
Full screen (f)
Deck 12: Chi-Square Tests
1
What are the degrees of freedom for the goodness-of-fit test for normality?
A) 2
B) k−3
C) k−2
D) k−1
A) 2
B) k−3
C) k−2
D) k−1
B
2
For the Jarque-Bera test for normality,the test statistic is assumed to have a chi-square distribution with two degrees of freedom.
True
3
For the chi-square test of a contingency table,the expected cell frequencies are found as ________________.
A) the row total multiplied by the column total divided by the sample size
B) the observed cell frequency
C) (r−1)(c−1)
D) rc
A) the row total multiplied by the column total divided by the sample size
B) the observed cell frequency
C) (r−1)(c−1)
D) rc
A
4
When applying the goodness-of-fit test for normality,the quantitative data must be converted into a qualitative format.

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
5
For the goodness-of-fit test,the expected category frequencies found are the _________________________.
A) sample proportions
B) hypothesized proportions
C) average of the hypothesized and sample proportions
D) proportions specified under the alternative hypothesis
A) sample proportions
B) hypothesized proportions
C) average of the hypothesized and sample proportions
D) proportions specified under the alternative hypothesis

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
6
The chi-square test statistic measures the difference between the observed frequencies and the expected frequencies assuming the null hypothesis is true.

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
7
For a chi-square test of a contingency table,each observation may be counted in multiple cells of the contingency table.

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
8
For a chi-square test of a contingency table,the degrees of freedom are calculated as (r−1)(c−1)where r and c are the number of rows and columns in the contingency table.

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
9
For a chi-square goodness-of-fit test,the expected category frequencies are calculated using the sample category proportions.

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
10
A goodness-of-fit test analyzes for two qualitative variables whereas a chi-square test of a contingency table is for a single qualitative variable.

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
11
The chi-square test of a contingency table is valid when the expected cell frequencies are _______________.
A) equal to 0
B) more than 0 but less than 5
C) at least 5
D) negative
A) equal to 0
B) more than 0 but less than 5
C) at least 5
D) negative

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
12
For a multinomial experiment with k categories,the goodness-of-fit test statistic is assumed to follow a chi-square distribution with k degrees of freedom.

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
13
Which of the following null hypotheses is used to test if five population proportions are the same?
A) H0:p1 = p2 = p3 = p4 = p5 = 0.25
B) H0:p1 = p2 = p3 = p4 = 0.25
C) H0:p1 = p2 = p3 = p4 = 0.20
D) H0:p1 = p2 = p3 = p4 = p5 = 0.20
A) H0:p1 = p2 = p3 = p4 = p5 = 0.25
B) H0:p1 = p2 = p3 = p4 = 0.25
C) H0:p1 = p2 = p3 = p4 = 0.20
D) H0:p1 = p2 = p3 = p4 = p5 = 0.20

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
14
For the chi-square test of a contingency table,the expected cell frequencies are found as 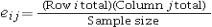 which is the same as ___________________________.
which is the same as ___________________________.
A) the observed cell frequencies
B) the cell probability multiplied by the sample size
C) the row total
D) the column total
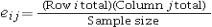 which is the same as ___________________________.
which is the same as ___________________________.A) the observed cell frequencies
B) the cell probability multiplied by the sample size
C) the row total
D) the column total

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
15
For a multinomial experiment,which of the following is not true?
A) The number of categories is at least two,k ≥ 2.
B) The trials are dependent.
C) The sum of the cell probabilities is P1 + P2 + ...+ Pk = 1.
D) The category probabilities are the same for each trial.
A) The number of categories is at least two,k ≥ 2.
B) The trials are dependent.
C) The sum of the cell probabilities is P1 + P2 + ...+ Pk = 1.
D) The category probabilities are the same for each trial.

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
16
If the null hypothesis is rejected by the goodness-of-fit test,the alternative hypothesis specifies which of the population proportions differ from their hypothesized values.

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
17
For the goodness-of-fit test,the chi-square test statistic will __________________.
A) always equal zero
B) always be negative
C) be at least zero
D) always be equal ton
A) always equal zero
B) always be negative
C) be at least zero
D) always be equal ton

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
18
For the goodness-of-fit test,the sum of the expected frequencies must equal ___.
A) 1
B) n
C) k
D) k−1
A) 1
B) n
C) k
D) k−1

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
19
For a chi-square test of a contingency table,the expected frequencies for each cell are calculated assuming the null hypothesis is true.

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
20
The chi-square test of a contingency table is a test of independence for __________________.
A) a single qualitative variable
B) two qualitative variables
C) two quantitative variables
D) three or more quantitative variables
A) a single qualitative variable
B) two qualitative variables
C) two quantitative variables
D) three or more quantitative variables

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
21
For the Jarque-Bera test for normality,the null and alternative hypotheses are __________________________.
A) H0:S < 0 and K > 0,HA:S < 0 or K < 0
B) H0:S < 0 and K = 0,HA:S > 0 or K ≠ 0
C) H0:S = 0 and K = 0,HA:S ≠ 0 or K ≠ 0
D) H0:S = 0 and K > 0,HA:S ≠ 0 or K < 0
A) H0:S < 0 and K > 0,HA:S < 0 or K < 0
B) H0:S < 0 and K = 0,HA:S > 0 or K ≠ 0
C) H0:S = 0 and K = 0,HA:S ≠ 0 or K ≠ 0
D) H0:S = 0 and K > 0,HA:S ≠ 0 or K < 0

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
22
The calculation of the Jarque-Bera test statistic involves___________.
A) only the sample size
B) the sample size,standard deviation,and average
C) the sample size,skewness coefficient,and kurtosis coefficient
D) the sample average,skewness coefficient,and kurtosis coefficient
A) only the sample size
B) the sample size,standard deviation,and average
C) the sample size,skewness coefficient,and kurtosis coefficient
D) the sample average,skewness coefficient,and kurtosis coefficient

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
23
For the goodness-of-fit test for normality to be applied,what is the minimum number of qualitative intervals the quantitative data can be converted to?
A) 2
B) 4
C) 5
D) 10
A) 2
B) 4
C) 5
D) 10

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
24
A university has six colleges and takes a poll to gauge student support for a tuition increase.The university wants to ensure each college is represented fairly.The below table shows the observed number of students who participate in the poll from each college and the actual proportion of students in each college. 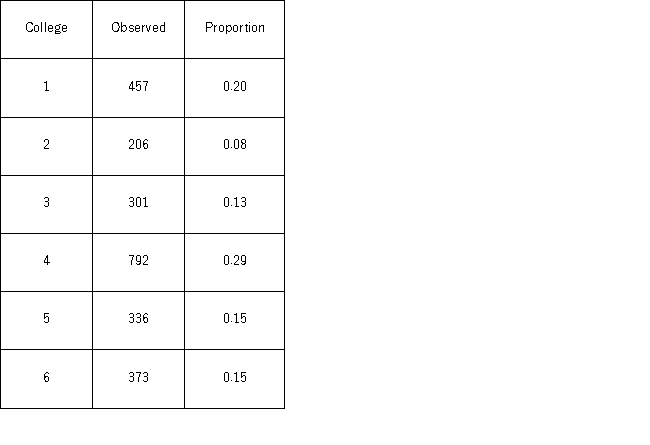 Which of the following is the value of the goodness-of-fit test statistic?
Which of the following is the value of the goodness-of-fit test statistic?
A) 3.08
B) 15.09
C) 15.64
D) 16.75
 Which of the following is the value of the goodness-of-fit test statistic?
Which of the following is the value of the goodness-of-fit test statistic?A) 3.08
B) 15.09
C) 15.64
D) 16.75

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
25
Packaged candies have three different types of colors.Suppose you want to determine if the population proportion of each color is the same.The most appropriate test is the ______________________________________.
A) goodness-of-fit test for a multinomial experiment
B) chi-square test for independence
C) goodness-of-fit test for normality
D) Jarque-Bera test for normality
A) goodness-of-fit test for a multinomial experiment
B) chi-square test for independence
C) goodness-of-fit test for normality
D) Jarque-Bera test for normality

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
26
A card-dealing machine deals spades (1),hearts (2),clubs (3),and diamonds (4)at random as if from an infinite deck.In a randomness check,1,600 cards were dealt and counted.The results are shown below. 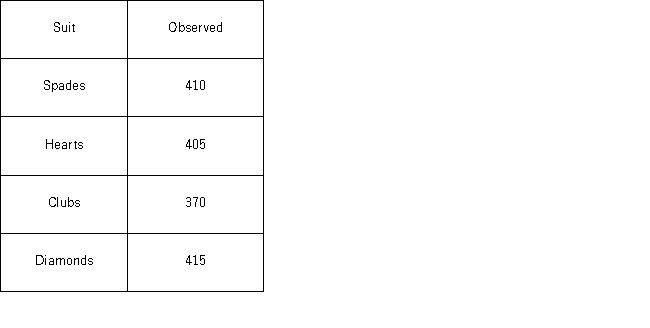 At the 5% significance level,the critical value is ______.
At the 5% significance level,the critical value is ______.
A) 6.251
B) 7.815
C) 9.348
D) 11.345
 At the 5% significance level,the critical value is ______.
At the 5% significance level,the critical value is ______.A) 6.251
B) 7.815
C) 9.348
D) 11.345

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
27
Suppose you want to determine if the quarterly returns for mutual funds have a normal distribution using quantitative summary statistics.The most appropriate test is the _________________________________.
A) goodness-of-fit test for a multinomial experiment
B) chi-square test for independence
C) goodness-of-fit test for normality
D) Jarque-Bera test for normality
A) goodness-of-fit test for a multinomial experiment
B) chi-square test for independence
C) goodness-of-fit test for normality
D) Jarque-Bera test for normality

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
28
A card-dealing machine deals spades (1),hearts (2),clubs (3),and diamonds (4)at random as if from an infinite deck.In a randomness check,1,600 cards were dealt and counted.The results are shown below. 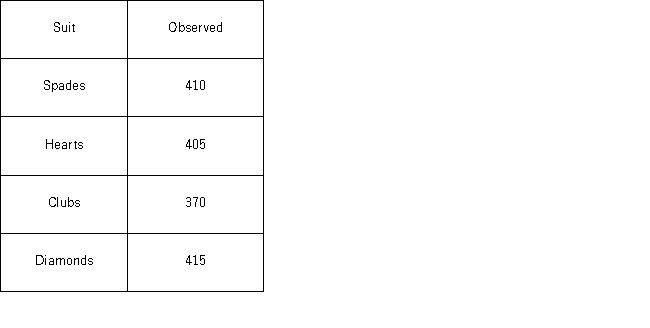 To test if the poker-dealing machine deals cards at random,the null and alternative hypotheses are ________________________________________.
To test if the poker-dealing machine deals cards at random,the null and alternative hypotheses are ________________________________________.
A) H0:p1 = p2 = p3 = p4 = 0,HA: Not all population proportions are equal to 0,25
B) H0:p1 = p2 = p3 = p4 = 0.25,HA: Not all population proportions are equal to 0,25
C) H0:p1 = p2 = p3 = p4 = 1,HA: Not all population proportions are equal to 0,25
D) H0:p1 = p2 = p3 = p4 = 0.20,HA: Not all population proportions are equal to 0,20
 To test if the poker-dealing machine deals cards at random,the null and alternative hypotheses are ________________________________________.
To test if the poker-dealing machine deals cards at random,the null and alternative hypotheses are ________________________________________.A) H0:p1 = p2 = p3 = p4 = 0,HA: Not all population proportions are equal to 0,25
B) H0:p1 = p2 = p3 = p4 = 0.25,HA: Not all population proportions are equal to 0,25
C) H0:p1 = p2 = p3 = p4 = 1,HA: Not all population proportions are equal to 0,25
D) H0:p1 = p2 = p3 = p4 = 0.20,HA: Not all population proportions are equal to 0,20

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
29
A university has six colleges and takes a poll to gauge student support for a tuition increase.The university wants to ensure each college is represented fairly.The below table shows the observed number of students who participate in the poll from each college and the actual proportion of students in each college. 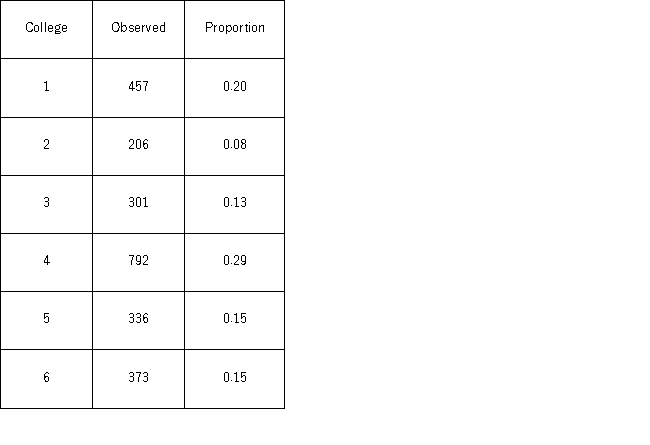 For the goodness-of-fit test,the assumed degrees of freedom are _____.
For the goodness-of-fit test,the assumed degrees of freedom are _____.
A) 2
B) 3
C) 4
D) 5
 For the goodness-of-fit test,the assumed degrees of freedom are _____.
For the goodness-of-fit test,the assumed degrees of freedom are _____.A) 2
B) 3
C) 4
D) 5

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
30
A card-dealing machine deals spades (1),hearts (2),clubs (3),and diamonds (4)at random as if from an infinite deck.In a randomness check,1,600 cards were dealt and counted.The results are shown below. 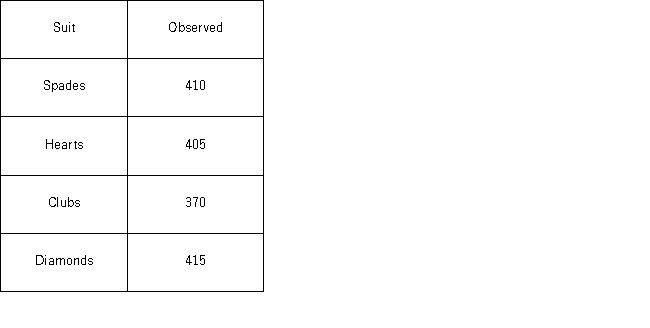 Using the p-value approach and α = 0.05,the decision and conclusion are _________________________________.
Using the p-value approach and α = 0.05,the decision and conclusion are _________________________________.
A) do not reject the null hypothesis;all of the population proportions are the same
B) reject the null hypothesis;conclude that not all proportions are equal to 0.20
C) reject the null hypothesis;conclude that not all proportions are equal to 0.25
D) do not reject the null hypothesis;we cannot conclude that not all of the proportions are equal to 0.25
 Using the p-value approach and α = 0.05,the decision and conclusion are _________________________________.
Using the p-value approach and α = 0.05,the decision and conclusion are _________________________________.A) do not reject the null hypothesis;all of the population proportions are the same
B) reject the null hypothesis;conclude that not all proportions are equal to 0.20
C) reject the null hypothesis;conclude that not all proportions are equal to 0.25
D) do not reject the null hypothesis;we cannot conclude that not all of the proportions are equal to 0.25

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
31
A card-dealing machine deals spades (1),hearts (2),clubs (3),and diamonds (4)at random as if from an infinite deck.In a randomness check,1,600 cards were dealt and counted.The results are shown below. 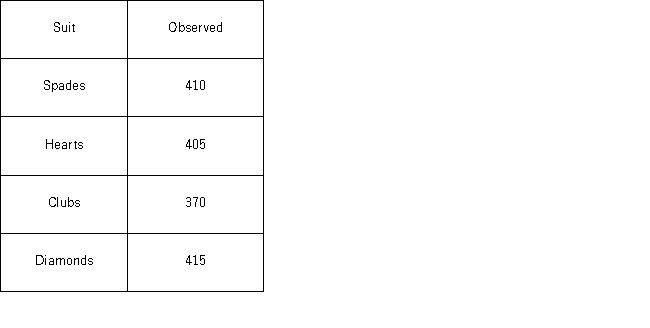 For the goodness-of-fit test,the value of the test statistic is _____.
For the goodness-of-fit test,the value of the test statistic is _____.
A) 2.25
B) 3.125
C) 6.45
D) 7.815
 For the goodness-of-fit test,the value of the test statistic is _____.
For the goodness-of-fit test,the value of the test statistic is _____.A) 2.25
B) 3.125
C) 6.45
D) 7.815

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
32
A university has six colleges and takes a poll to gauge student support for a tuition increase.The university wants to ensure each college is represented fairly.The below table shows the observed number of students who participate in the poll from each college and the actual proportion of students in each college. 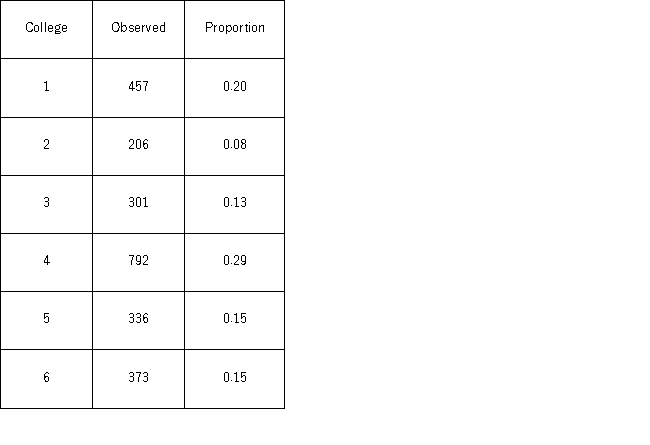 For the goodness-of-fit test,the alternative hypothesis states that __________________________________________.
For the goodness-of-fit test,the alternative hypothesis states that __________________________________________.
A) HA: Not all population proportions are equal to 0,20
B) HA: At least one of the population proportions is different from its hypothesized value
C) HA: Not all population proportions are the same
D) HA: Not all population proportions are equal to 0,17
 For the goodness-of-fit test,the alternative hypothesis states that __________________________________________.
For the goodness-of-fit test,the alternative hypothesis states that __________________________________________.A) HA: Not all population proportions are equal to 0,20
B) HA: At least one of the population proportions is different from its hypothesized value
C) HA: Not all population proportions are the same
D) HA: Not all population proportions are equal to 0,17

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
33
For the chi-square test for normality,the expected frequencies for each interval must be __________.
A) exactly 2
B) k−3
C) at least 5
D) k−1
A) exactly 2
B) k−3
C) at least 5
D) k−1

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
34
A card-dealing machine deals spades (1),hearts (2),clubs (3),and diamonds (4)at random as if from an infinite deck.In a randomness check,1,600 cards were dealt and counted.The results are shown below. 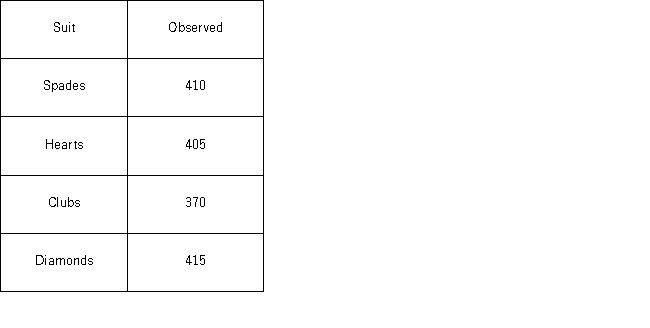 The p-value is _______________.
The p-value is _______________.
A) less than 0.01
B) between 0.01 and 0.05
C) between 0.05 and 0.10
D) greater than 0.10
 The p-value is _______________.
The p-value is _______________.A) less than 0.01
B) between 0.01 and 0.05
C) between 0.05 and 0.10
D) greater than 0.10

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
35
Suppose you want to determine if gender and major are independent.Which of the following tests should you use?
A) Goodness-of-fit test for a multinomial experiment
B) Chi-square test for independence
C) Goodness-of-fit test for normality
D) Jarque-Bera test for normality
A) Goodness-of-fit test for a multinomial experiment
B) Chi-square test for independence
C) Goodness-of-fit test for normality
D) Jarque-Bera test for normality

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
36
A card-dealing machine deals spades (1),hearts (2),clubs (3),and diamonds (4)at random as if from an infinite deck.In a randomness check,1,600 cards were dealt and counted.The results are shown below. 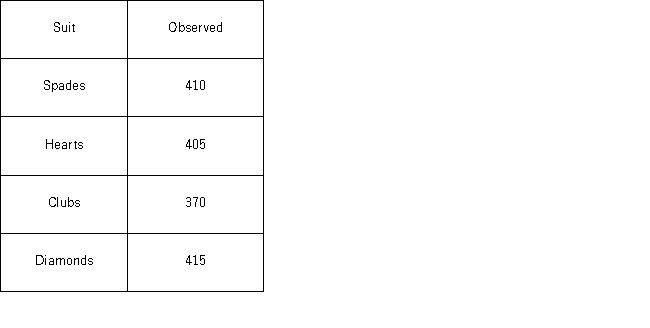 For the goodness-of-fit test,the degrees of freedom are ____.
For the goodness-of-fit test,the degrees of freedom are ____.
A) 2
B) 3
C) 4
D) 5
 For the goodness-of-fit test,the degrees of freedom are ____.
For the goodness-of-fit test,the degrees of freedom are ____.A) 2
B) 3
C) 4
D) 5

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
37
Suppose you want to determine if the quarterly returns for mutual funds have a normal distribution when your available data is partitioned into some non-overlapping intervals with given frequencies.The most appropriate test is the _____________________________________.
A) goodness-of-fit test for a multinomial experiment
B) chi-square test for independence
C) goodness-of-fit test for normality
D) Jarque-Bera test for normality
A) goodness-of-fit test for a multinomial experiment
B) chi-square test for independence
C) goodness-of-fit test for normality
D) Jarque-Bera test for normality

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
38
For the goodness-of-fit test for normality,the null and alternative hypotheses are _____________________.
A) H0: Data does not follow a normal distribution,HA: Data follows a normal distribution
B) H0: Data follows a normal distribution,HA: Data does not follow a normal distribution
C) H0: Data follows a normal distribution,HA: Data are skewed right
D) H0: Data follows a normal distribution,HA: Data are skewed left
A) H0: Data does not follow a normal distribution,HA: Data follows a normal distribution
B) H0: Data follows a normal distribution,HA: Data does not follow a normal distribution
C) H0: Data follows a normal distribution,HA: Data are skewed right
D) H0: Data follows a normal distribution,HA: Data are skewed left

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
39
A card-dealing machine deals spades (1),hearts (2),clubs (3),and diamonds (4)at random as if from an infinite deck.In a randomness check,1,600 cards were dealt and counted.The results are shown below.  Using the critical value approach,the decision and conclusion are _________________________________________.
Using the critical value approach,the decision and conclusion are _________________________________________.
A) do not reject the null hypothesis;we cannot conclude that not all of the proportions are equal to 0.25
B) do not reject the null hypothesis;all of the population proportions are the same
C) reject the null hypothesis;conclude that not all proportions are equal to 0.25
D) reject the null hypothesis;conclude that not all proportions are equal to 0.20
 Using the critical value approach,the decision and conclusion are _________________________________________.
Using the critical value approach,the decision and conclusion are _________________________________________.A) do not reject the null hypothesis;we cannot conclude that not all of the proportions are equal to 0.25
B) do not reject the null hypothesis;all of the population proportions are the same
C) reject the null hypothesis;conclude that not all proportions are equal to 0.25
D) reject the null hypothesis;conclude that not all proportions are equal to 0.20

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
40
If a test statistic has a value of X and is assumed to be χ2 distributed with df degrees of freedom,then the p-value for a right-tailed test found by using Excel's command ________________________.
A) '=CHISQ.DIST.RT(X,Deg_freedom)'
B) '=CHISQ.DIST.RT(Deg_freedom,X)'
C) '=1-CHISQ.DIST.RT(X,Deg_freedom)'
D) '=1-CHISQ.DIST.RT(Deg_freedom,X)'
A) '=CHISQ.DIST.RT(X,Deg_freedom)'
B) '=CHISQ.DIST.RT(Deg_freedom,X)'
C) '=1-CHISQ.DIST.RT(X,Deg_freedom)'
D) '=1-CHISQ.DIST.RT(Deg_freedom,X)'

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
41
In the following table,likely voters' preferences of two candidates are cross-classified by gender. 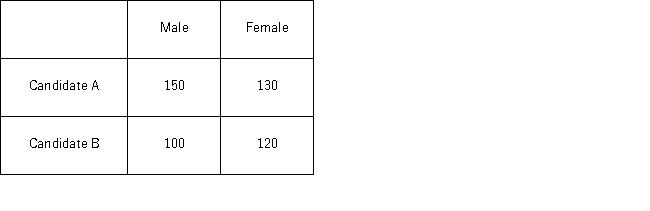 At the 10% significance level,the critical value is _______.
At the 10% significance level,the critical value is _______.
A) 6.635
B) 5.024
C) 3.841
D) 2.706
 At the 10% significance level,the critical value is _______.
At the 10% significance level,the critical value is _______.A) 6.635
B) 5.024
C) 3.841
D) 2.706

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
42
A university has six colleges and takes a poll to gauge student support for a tuition increase.The university wants to ensure each college is represented fairly.The below table shows the observed number of students who participate in the poll from each college and the actual proportion of students in each college. 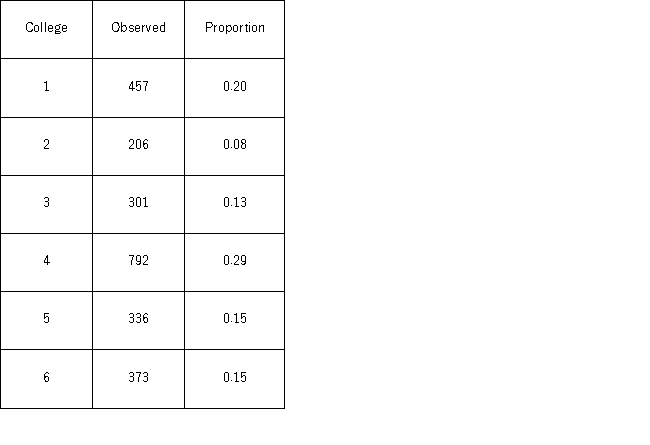 The p-value is ______________.
The p-value is ______________.
A) less than 0.01
B) between 0.01 and 0.05
C) between 0.05 and 0.10
D) greater than 0.10
 The p-value is ______________.
The p-value is ______________.A) less than 0.01
B) between 0.01 and 0.05
C) between 0.05 and 0.10
D) greater than 0.10

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
43
A fund manager wants to know if it is equally likely that the Dow Jones Industrial Average will go up each day of the week.For each day of the week,the fund manager observes the following number of days when the Dow Jones Industrial Average goes up. 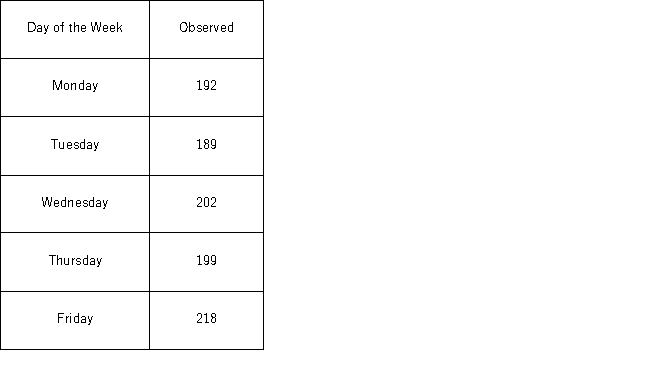 Which of the following is the value of goodness-of-fit chi-square test statistic?
Which of the following is the value of goodness-of-fit chi-square test statistic?
A) 0.605
B) 0.632
C) 1.62
D) 2.57
 Which of the following is the value of goodness-of-fit chi-square test statistic?
Which of the following is the value of goodness-of-fit chi-square test statistic?A) 0.605
B) 0.632
C) 1.62
D) 2.57

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
44
In the following table,individuals are cross-classified by their age group and income level. 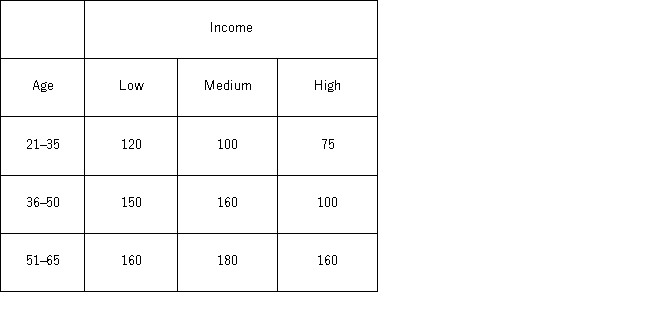 Which of the following is the expected joint probability for the "low income and 21-35 age group" cell assuming age group and income are independent?
Which of the following is the expected joint probability for the "low income and 21-35 age group" cell assuming age group and income are independent?
A) 0.0830
B) 0.0874
C) 0.0996
D) 0.1328
 Which of the following is the expected joint probability for the "low income and 21-35 age group" cell assuming age group and income are independent?
Which of the following is the expected joint probability for the "low income and 21-35 age group" cell assuming age group and income are independent?A) 0.0830
B) 0.0874
C) 0.0996
D) 0.1328

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
45
In the following table,likely voters' preferences of two candidates are cross-classified by gender.  To test that gender and candidate preference are independent,the null hypothesis is ___________________________.
To test that gender and candidate preference are independent,the null hypothesis is ___________________________.
A) H0: Gender and candidate preference are independent
B) H0: Gender and candidate preference are mutually exclusive
C) H0: Gender and candidate preference are not mutually exclusive
D) H0: Gender and candidate preference are dependent
 To test that gender and candidate preference are independent,the null hypothesis is ___________________________.
To test that gender and candidate preference are independent,the null hypothesis is ___________________________.A) H0: Gender and candidate preference are independent
B) H0: Gender and candidate preference are mutually exclusive
C) H0: Gender and candidate preference are not mutually exclusive
D) H0: Gender and candidate preference are dependent

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
46
A fund manager wants to know if it is equally likely that the Dow Jones Industrial Average will go up each day of the week.For each day of the week,the fund manager observes the following number of days when the Dow Jones Industrial Average goes up. 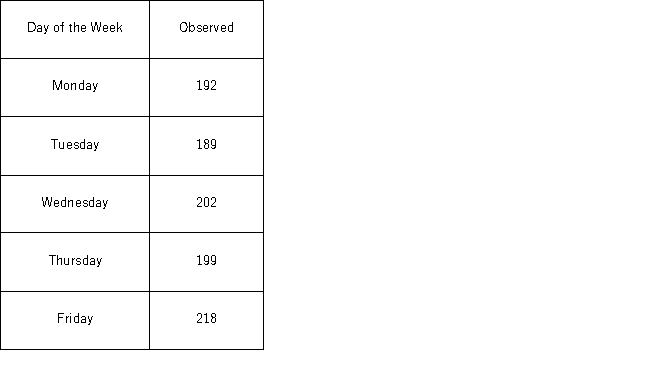 For the goodness-of-fit test,the null and alternative hypotheses are ________________________________________.
For the goodness-of-fit test,the null and alternative hypotheses are ________________________________________.
A) H0:p1 = p2 = p3 = p4 = 1/4,HA: Not all population proportions are equal to 1/4
B) H0:p1 = p2 = p3 = p4 = p5 = 1/5,HA: Not all population proportions are equal to 1/5
C) H0:p1 = p2 = p3 = p4 = p5 = 1/4,HA: Not all population proportions are equal to 1/4
D) H0:p1 = p2 = p3 = p4 = 1/5,HA: Not all population proportions are equal to 1/5
 For the goodness-of-fit test,the null and alternative hypotheses are ________________________________________.
For the goodness-of-fit test,the null and alternative hypotheses are ________________________________________.A) H0:p1 = p2 = p3 = p4 = 1/4,HA: Not all population proportions are equal to 1/4
B) H0:p1 = p2 = p3 = p4 = p5 = 1/5,HA: Not all population proportions are equal to 1/5
C) H0:p1 = p2 = p3 = p4 = p5 = 1/4,HA: Not all population proportions are equal to 1/4
D) H0:p1 = p2 = p3 = p4 = 1/5,HA: Not all population proportions are equal to 1/5

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
47
A fund manager wants to know if it is equally likely that the Dow Jones Industrial Average will go up each day of the week.For each day of the week,the fund manager observes the following number of days when the Dow Jones Industrial Average goes up. 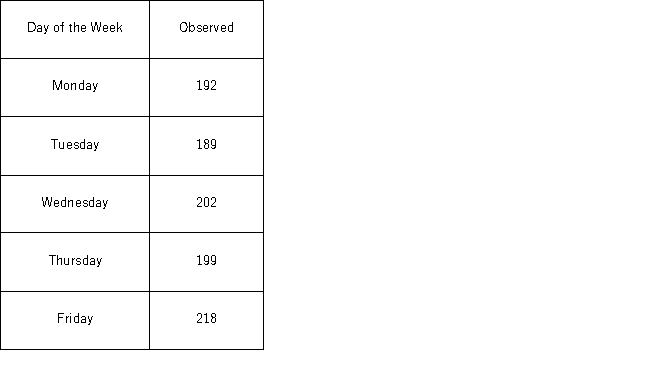 Using the critical value approach,the decision and conclusion are
Using the critical value approach,the decision and conclusion are
A) reject the null hypothesis;not all of the proportions are the same
B) reject the null hypothesis;all of the proportions are not the same
C) do not reject the null hypothesis;all of the proportions are the same
D) do not reject the null hypothesis;we cannot conclude that not all of the proportions are the same
 Using the critical value approach,the decision and conclusion are
Using the critical value approach,the decision and conclusion areA) reject the null hypothesis;not all of the proportions are the same
B) reject the null hypothesis;all of the proportions are not the same
C) do not reject the null hypothesis;all of the proportions are the same
D) do not reject the null hypothesis;we cannot conclude that not all of the proportions are the same

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
48
A fund manager wants to know if it is equally likely that the Dow Jones Industrial Average will go up each day of the week.For each day of the week,the fund manager observes the following number of days when the Dow Jones Industrial Average goes up. 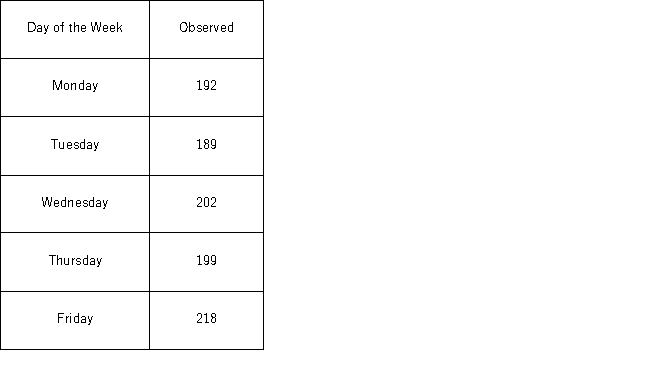 Using the p-value approach and α = 0.05,the decision and conclusion are ____________________________________.
Using the p-value approach and α = 0.05,the decision and conclusion are ____________________________________.
A) do not reject the null hypothesis;all of the proportions are the same
B) do not reject the null hypothesis;we cannot conclude that not all of the proportions are the same
C) reject the null hypothesis;not all of the proportions are the same
D) reject the null hypothesis;all of the proportions are not the same
 Using the p-value approach and α = 0.05,the decision and conclusion are ____________________________________.
Using the p-value approach and α = 0.05,the decision and conclusion are ____________________________________.A) do not reject the null hypothesis;all of the proportions are the same
B) do not reject the null hypothesis;we cannot conclude that not all of the proportions are the same
C) reject the null hypothesis;not all of the proportions are the same
D) reject the null hypothesis;all of the proportions are not the same

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
49
A fund manager wants to know if it is equally likely that the Dow Jones Industrial Average will go up each day of the week.For each day of the week,the fund manager observes the following number of days when the Dow Jones Industrial Average goes up. 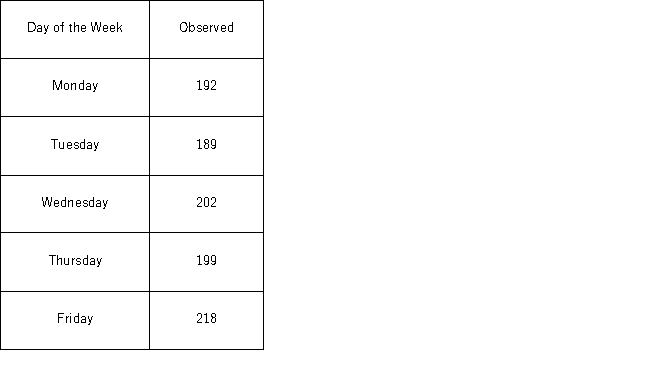 The p-value is ________________.
The p-value is ________________.
A) less than 0.01
B) between 0.01 and 0.05
C) between 0.05 and 0.10
D) greater than 0.10
 The p-value is ________________.
The p-value is ________________.A) less than 0.01
B) between 0.01 and 0.05
C) between 0.05 and 0.10
D) greater than 0.10

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
50
In the following table,likely voters' preferences of two candidates are cross-classified by gender. 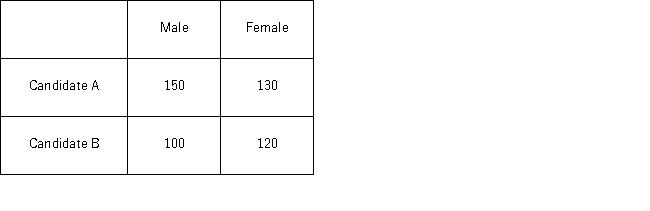 Using the critical value approach,the decision and conclusion are ________________________________________.
Using the critical value approach,the decision and conclusion are ________________________________________.
A) reject the null hypothesis;gender and candidate preference are dependent
B) do not reject the null hypothesis;gender and candidate preference are independent
C) reject the null hypothesis;gender and candidate preference are independent
D) do not reject the null hypothesis;gender and candidate preference are dependent
 Using the critical value approach,the decision and conclusion are ________________________________________.
Using the critical value approach,the decision and conclusion are ________________________________________.A) reject the null hypothesis;gender and candidate preference are dependent
B) do not reject the null hypothesis;gender and candidate preference are independent
C) reject the null hypothesis;gender and candidate preference are independent
D) do not reject the null hypothesis;gender and candidate preference are dependent

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
51
A fund manager wants to know if it is equally likely that the Dow Jones Industrial Average will go up each day of the week.For each day of the week,the fund manager observes the following number of days when the Dow Jones Industrial Average goes up.  For the goodness-of-fit test,what are the degrees of freedom for the chi-squared test statistic?
For the goodness-of-fit test,what are the degrees of freedom for the chi-squared test statistic?
A) 4
B) 5
C) 6
D) 7
 For the goodness-of-fit test,what are the degrees of freedom for the chi-squared test statistic?
For the goodness-of-fit test,what are the degrees of freedom for the chi-squared test statistic?A) 4
B) 5
C) 6
D) 7

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
52
In the following table,likely voters' preferences of two candidates are cross-classified by gender. 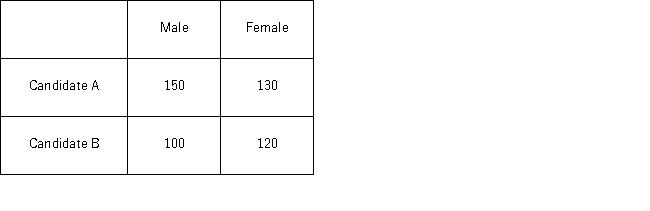 For the chi-square test of independence,the value of the test statistic is _______.
For the chi-square test of independence,the value of the test statistic is _______.
A) 2.34
B) 1.62
C) 3.25
D) 4
 For the chi-square test of independence,the value of the test statistic is _______.
For the chi-square test of independence,the value of the test statistic is _______.A) 2.34
B) 1.62
C) 3.25
D) 4

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
53
In the following table,likely voters' preferences of two candidates are cross-classified by gender. 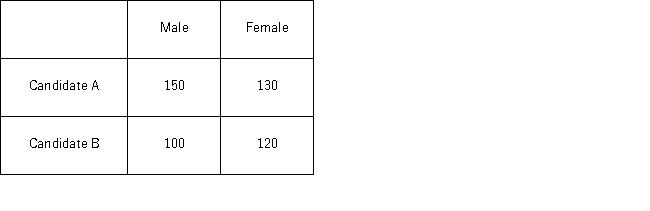 Using the p-value approach and α = 0.10,the decision and conclusion are ___________________________________.
Using the p-value approach and α = 0.10,the decision and conclusion are ___________________________________.
A) reject the null hypothesis;gender and candidate preference are dependent
B) do not reject the null hypothesis;gender and candidate preference are independent
C) reject the null hypothesis;gender and candidate preference are independent
D) do not reject null hypothesis;gender and candidate preference are dependent
 Using the p-value approach and α = 0.10,the decision and conclusion are ___________________________________.
Using the p-value approach and α = 0.10,the decision and conclusion are ___________________________________.A) reject the null hypothesis;gender and candidate preference are dependent
B) do not reject the null hypothesis;gender and candidate preference are independent
C) reject the null hypothesis;gender and candidate preference are independent
D) do not reject null hypothesis;gender and candidate preference are dependent

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
54
In the following table,likely voters' preferences of two candidates are cross-classified by gender.  For the chi-square test of independence,the assumed degrees of freedom are ____.
For the chi-square test of independence,the assumed degrees of freedom are ____.
A) 1
B) 2
C) 3
D) 4
 For the chi-square test of independence,the assumed degrees of freedom are ____.
For the chi-square test of independence,the assumed degrees of freedom are ____.A) 1
B) 2
C) 3
D) 4

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
55
In the following table,likely voters' preferences of two candidates are cross-classified by gender.  The p-value is _______________.
The p-value is _______________.
A) less than 0.01
B) between 0.01 and 0.05
C) between 0.05 and 0.10
D) greater than 0.10
 The p-value is _______________.
The p-value is _______________.A) less than 0.01
B) between 0.01 and 0.05
C) between 0.05 and 0.10
D) greater than 0.10

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
56
A fund manager wants to know if it is equally likely that the Dow Jones Industrial Average will go up each day of the week.For each day of the week,the fund manager observes the following number of days when the Dow Jones Industrial Average goes up.  At the 5% significance level,the critical value is _______.
At the 5% significance level,the critical value is _______.
A) 7.779
B) 9.488
C) 11.143
D) 13.277
 At the 5% significance level,the critical value is _______.
At the 5% significance level,the critical value is _______.A) 7.779
B) 9.488
C) 11.143
D) 13.277

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
57
In the following table,individuals are cross-classified by their age group and income level. 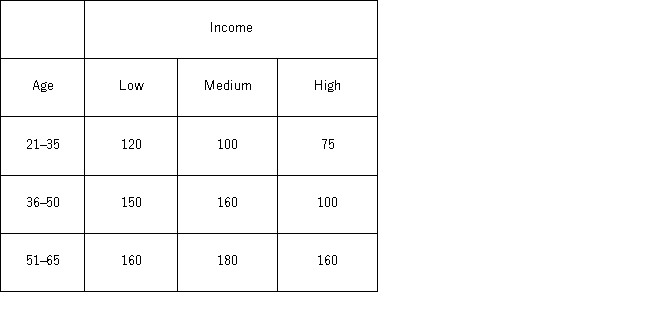 Which of the following is the estimated joint probability for the "low income and 21-35 age group" cell?
Which of the following is the estimated joint probability for the "low income and 21-35 age group" cell?
A) 0.0830
B) 0.0874
C) 0.0996
D) 0.1328
 Which of the following is the estimated joint probability for the "low income and 21-35 age group" cell?
Which of the following is the estimated joint probability for the "low income and 21-35 age group" cell?A) 0.0830
B) 0.0874
C) 0.0996
D) 0.1328

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
58
A university has six colleges and takes a poll to gauge student support for a tuition increase.The university wants to ensure each college is represented fairly.The below table shows the observed number of students who participate in the poll from each college and the actual proportion of students in each college. 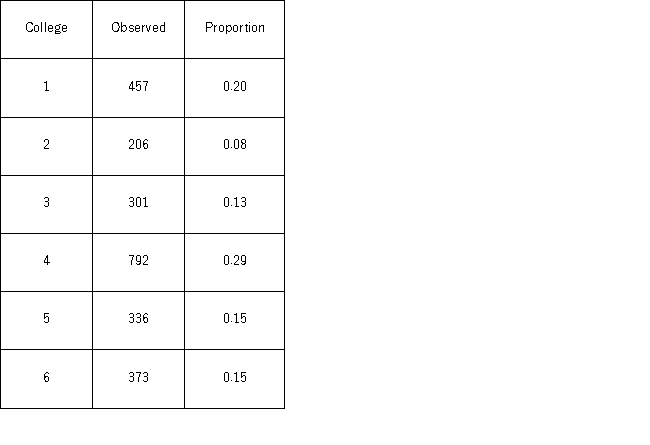 At the 1% significance level,the critical value is _______.
At the 1% significance level,the critical value is _______.
A) 9.236
B) 11.070
C) 12.833
D) 15.086
 At the 1% significance level,the critical value is _______.
At the 1% significance level,the critical value is _______.A) 9.236
B) 11.070
C) 12.833
D) 15.086

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
59
A university has six colleges and takes a poll to gauge student support for a tuition increase.The university wants to ensure each college is represented fairly.The below table shows the observed number of students who participate in the poll from each college and the actual proportion of students in each college. 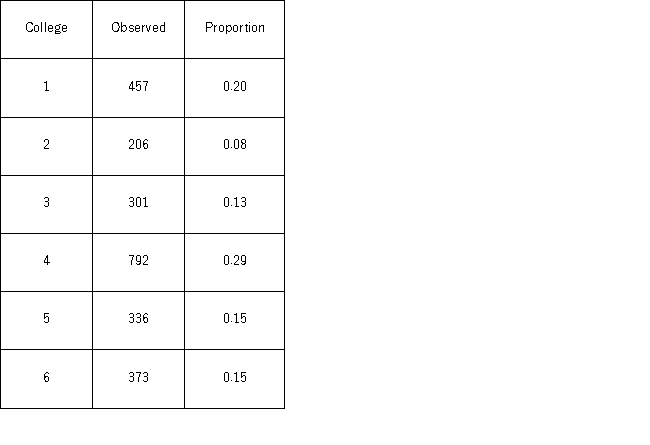 Using the p-value approach and α = 0.01,the decision and conclusion are ___________________________________.
Using the p-value approach and α = 0.01,the decision and conclusion are ___________________________________.
A) do not reject the null hypothesis;all proportions are equal to 0.20
B) do not reject the null hypothesis;we cannot conclude that not all of the proportions are the same
C) reject the null hypothesis;at least one of the proportions is different from its hypothesized value
D) reject the null hypothesis;all of the proportions are not the same
 Using the p-value approach and α = 0.01,the decision and conclusion are ___________________________________.
Using the p-value approach and α = 0.01,the decision and conclusion are ___________________________________.A) do not reject the null hypothesis;all proportions are equal to 0.20
B) do not reject the null hypothesis;we cannot conclude that not all of the proportions are the same
C) reject the null hypothesis;at least one of the proportions is different from its hypothesized value
D) reject the null hypothesis;all of the proportions are not the same

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
60
A university has six colleges and takes a poll to gauge student support for a tuition increase.The university wants to ensure each college is represented fairly.The below table shows the observed number of students that participate in the poll from each college and the actual proportion of students in each college. 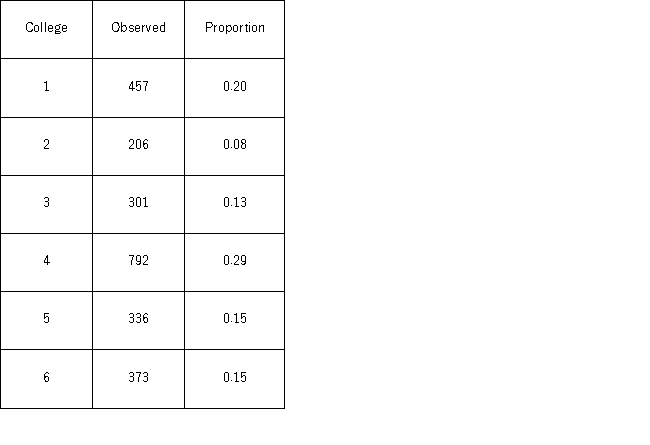 Using the critical value approach,the decision and conclusion are _________________________________________.
Using the critical value approach,the decision and conclusion are _________________________________________.
A) reject the null hypothesis;at least one of the proportions is different from its hypothesized value
B) reject the null hypothesis;all of the proportions are not the same
C) do not reject the null hypothesis;all proportions are equal to 0.20
D) do not reject the null hypothesis;we cannot conclude not all of the proportions are the same
 Using the critical value approach,the decision and conclusion are _________________________________________.
Using the critical value approach,the decision and conclusion are _________________________________________.A) reject the null hypothesis;at least one of the proportions is different from its hypothesized value
B) reject the null hypothesis;all of the proportions are not the same
C) do not reject the null hypothesis;all proportions are equal to 0.20
D) do not reject the null hypothesis;we cannot conclude not all of the proportions are the same

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
61
In the following table,individuals are cross-classified by their age group and income level. 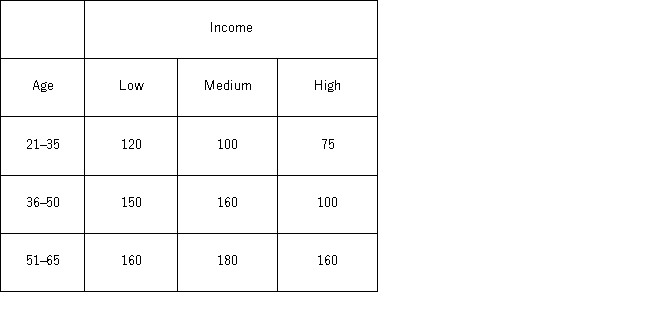 For the chi-square test of independence,the degrees of freedom are __________.
For the chi-square test of independence,the degrees of freedom are __________.
A) 2
B) 4
C) 9
D) 8
 For the chi-square test of independence,the degrees of freedom are __________.
For the chi-square test of independence,the degrees of freedom are __________.A) 2
B) 4
C) 9
D) 8

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
62
The following table shows the distribution of employees in an organization.Martha Foreman,an analyst,wants to see if race has a bearing on the position a person holds with this company. 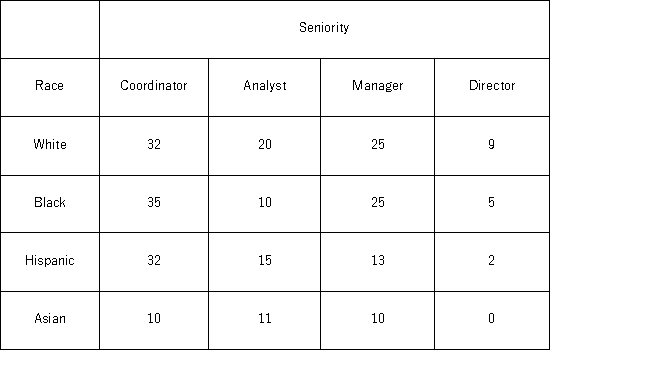 Using the critical value approach,the decision and conclusion are _______________________________________________________________.
Using the critical value approach,the decision and conclusion are _______________________________________________________________.
A) reject the hypothesis;conclude race and seniority are dependent
B) reject the null hypothesis;conclude race and seniority are independent
C) do not reject the null hypothesis;conclude race and seniority are dependent
D) do not reject the null hypothesis;conclude race and seniority are independent
 Using the critical value approach,the decision and conclusion are _______________________________________________________________.
Using the critical value approach,the decision and conclusion are _______________________________________________________________.A) reject the hypothesis;conclude race and seniority are dependent
B) reject the null hypothesis;conclude race and seniority are independent
C) do not reject the null hypothesis;conclude race and seniority are dependent
D) do not reject the null hypothesis;conclude race and seniority are independent

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
63
In the following table,individuals are cross-classified by their age group and income level. 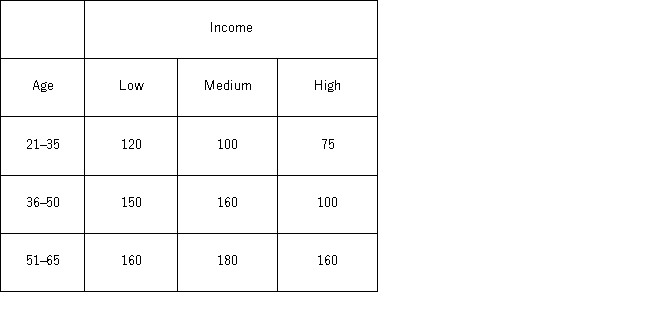 Assuming age group and income are independent,the expected "low income and 21-35 age group" cell frequency is ________.
Assuming age group and income are independent,the expected "low income and 21-35 age group" cell frequency is ________.
A) 105.27
B) 107.72
C) 146.31
D) 178.42
 Assuming age group and income are independent,the expected "low income and 21-35 age group" cell frequency is ________.
Assuming age group and income are independent,the expected "low income and 21-35 age group" cell frequency is ________.A) 105.27
B) 107.72
C) 146.31
D) 178.42

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
64
The following table shows the distribution of employees in an organization.Martha Foreman,an analyst,wants to see if race has a bearing on the position a person holds with this company. 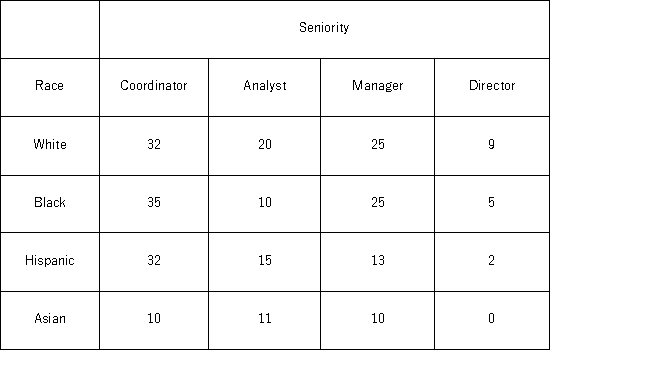 The row total for Asians is _____.
The row total for Asians is _____.
A) 86
B) 75
C) 62
D) 31
 The row total for Asians is _____.
The row total for Asians is _____.A) 86
B) 75
C) 62
D) 31

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
65
In the following table,individuals are cross-classified by their age group and income level.  For the chi-square test of independence,the value of the test statistic is ________.
For the chi-square test of independence,the value of the test statistic is ________.
A) 8.779
B) 10.840
C) 13.243
D) 16.159
 For the chi-square test of independence,the value of the test statistic is ________.
For the chi-square test of independence,the value of the test statistic is ________.A) 8.779
B) 10.840
C) 13.243
D) 16.159

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
66
The following table shows the distribution of employees in an organization.Martha Foreman,an analyst,wants to see if race has a bearing on the position a person holds with this company.  Using the p-value approach and α = 0.05,the decision and conclusion are ________________________________________________________________________.
Using the p-value approach and α = 0.05,the decision and conclusion are ________________________________________________________________________.
A) reject the null hypothesis;conclude race and seniority are dependent
B) reject the null hypothesis;conclude race and seniority are independent
C) do not reject the null hypothesis;cannot conclude race and seniority are dependent
D) do not reject the null hypothesis;conclude race and seniority are independent
 Using the p-value approach and α = 0.05,the decision and conclusion are ________________________________________________________________________.
Using the p-value approach and α = 0.05,the decision and conclusion are ________________________________________________________________________.A) reject the null hypothesis;conclude race and seniority are dependent
B) reject the null hypothesis;conclude race and seniority are independent
C) do not reject the null hypothesis;cannot conclude race and seniority are dependent
D) do not reject the null hypothesis;conclude race and seniority are independent

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
67
The following table shows the distribution of employees in an organization.Martha Foreman,an analyst,wants to see if race has a bearing on the position a person holds with this company. 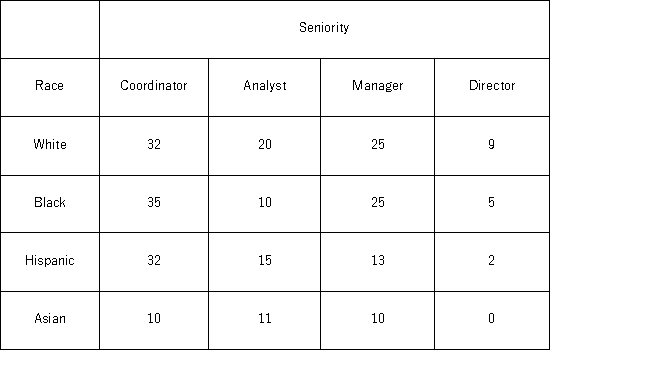 The column total for directors is _____.
The column total for directors is _____.
A) 16
B) 56
C) 73
D) 109
 The column total for directors is _____.
The column total for directors is _____.A) 16
B) 56
C) 73
D) 109

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
68
In the following table,individuals are cross-classified by their age group and income level. 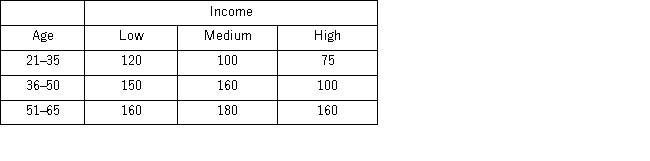 The p-value is
The p-value is
A) Less than 0.01
B) Between 0.01 and 0.05
C) Between 0.05 and 0.10
D) Greater than 0.10
 The p-value is
The p-value isA) Less than 0.01
B) Between 0.01 and 0.05
C) Between 0.05 and 0.10
D) Greater than 0.10

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
69
The following table shows the distribution of employees in an organization.Martha Foreman,an analyst,wants to see if race has a bearing on the position a person holds with this company. 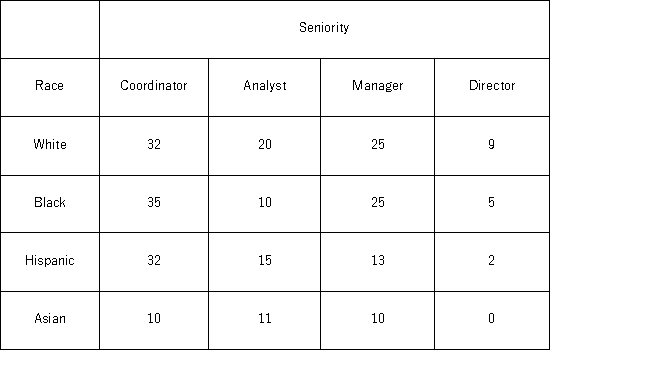 For the chi-square test of independence,the value of the test statistic is _______.
For the chi-square test of independence,the value of the test statistic is _______.
A) 12.221
B) 15.378
C) 17.853
D) 20.154
 For the chi-square test of independence,the value of the test statistic is _______.
For the chi-square test of independence,the value of the test statistic is _______.A) 12.221
B) 15.378
C) 17.853
D) 20.154

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
70
In the following table,individuals are cross-classified by their age group and income level. 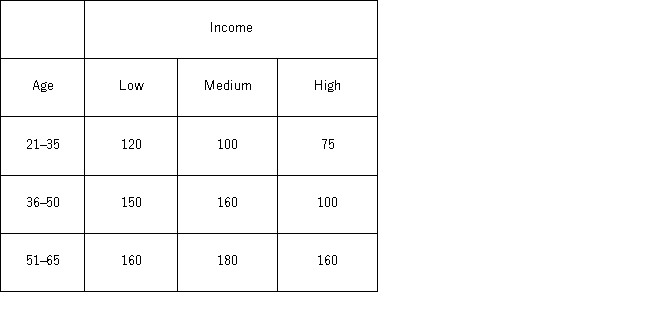 Using the p-value approach and α = 0.05,the decision and conclusion are __________________________________.
Using the p-value approach and α = 0.05,the decision and conclusion are __________________________________.
A) do not reject the null hypothesis;age and income are dependent
B) do not reject the null hypothesis;age and income are independent
C) reject the null hypothesis;age and income are dependent
D) reject the null hypothesis;age and income are independent
 Using the p-value approach and α = 0.05,the decision and conclusion are __________________________________.
Using the p-value approach and α = 0.05,the decision and conclusion are __________________________________.A) do not reject the null hypothesis;age and income are dependent
B) do not reject the null hypothesis;age and income are independent
C) reject the null hypothesis;age and income are dependent
D) reject the null hypothesis;age and income are independent

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
71
The following table shows the distribution of employees in an organization.Martha Foreman,an analyst,wants to see if race has a bearing on the position a person holds with this company. 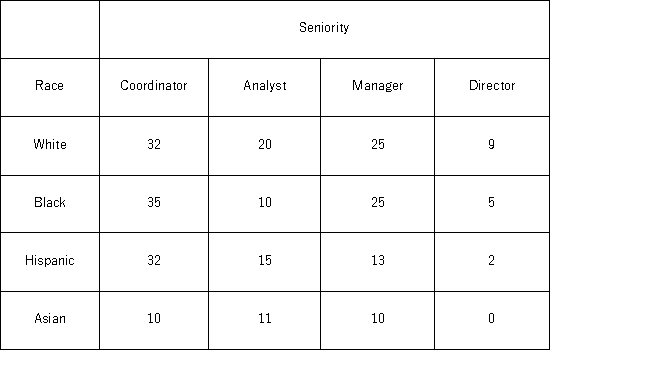 To test that race and seniority are independent,the null and alternative hypothesis are _______________________________________________________________________.
To test that race and seniority are independent,the null and alternative hypothesis are _______________________________________________________________________.
A) H0: Race and seniority are independent;HA: Race and seniority are dependent
B) H0: Race and seniority are mutually exclusive;HA: Race and seniority are not mutually exclusive
C) H0: Race and seniority are not mutually exclusive;HA: Race and seniority are mutually exclusive
D) H0: Race and seniority are dependent;HA: Race and seniority are independent
 To test that race and seniority are independent,the null and alternative hypothesis are _______________________________________________________________________.
To test that race and seniority are independent,the null and alternative hypothesis are _______________________________________________________________________.A) H0: Race and seniority are independent;HA: Race and seniority are dependent
B) H0: Race and seniority are mutually exclusive;HA: Race and seniority are not mutually exclusive
C) H0: Race and seniority are not mutually exclusive;HA: Race and seniority are mutually exclusive
D) H0: Race and seniority are dependent;HA: Race and seniority are independent

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
72
The following table shows the distribution of employees in an organization.Martha Foreman,an analyst,wants to see if race has a bearing on the position a person holds with this company. 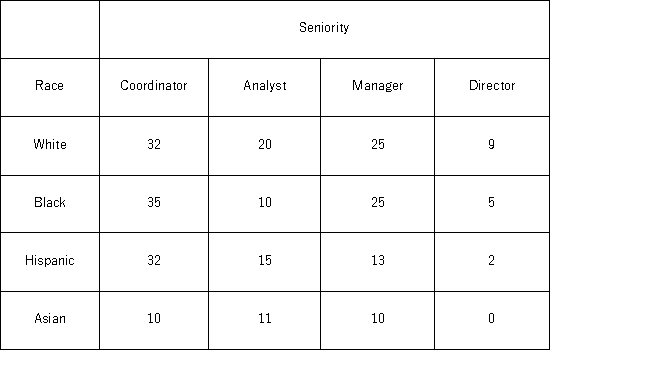 The p-value is ________________.
The p-value is ________________.
A) less than 0.01
B) between 0.01 and 0.05
C) between 0.05 and 0.10
D) greater than 0.10
 The p-value is ________________.
The p-value is ________________.A) less than 0.01
B) between 0.01 and 0.05
C) between 0.05 and 0.10
D) greater than 0.10

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
73
The heights (in cm)for a random sample of 60 males were measured.The sample mean is 166.55,the standard deviation is 12.57,the sample kurtosis is 0.12,and the sample skewness is −0.23.The following table shows the heights subdivided into non-overlapping intervals. 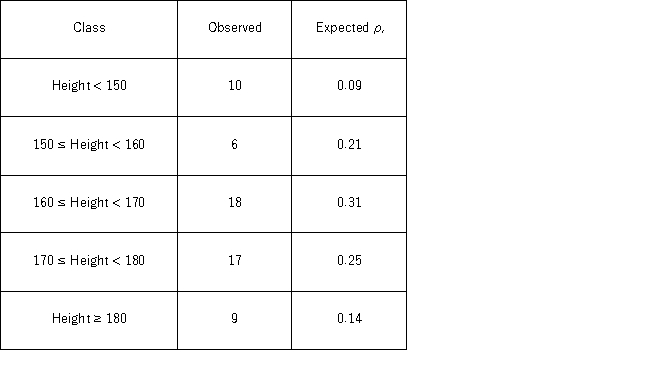 The heights are subdivided into five intervals.The degrees of freedom for the goodness-of-fit test for normality is ____.
The heights are subdivided into five intervals.The degrees of freedom for the goodness-of-fit test for normality is ____.
A) 2
B) 3
C) 4
D) 5
 The heights are subdivided into five intervals.The degrees of freedom for the goodness-of-fit test for normality is ____.
The heights are subdivided into five intervals.The degrees of freedom for the goodness-of-fit test for normality is ____.A) 2
B) 3
C) 4
D) 5

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
74
The following table shows the distribution of employees in an organization.Martha Foreman,an analyst,wants to see if race has a bearing on the position a person holds with this company. 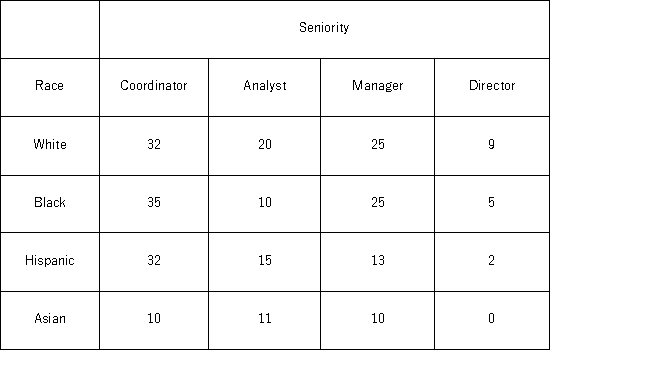 For the chi-square test for independence,the degrees of freedom used are _____.
For the chi-square test for independence,the degrees of freedom used are _____.
A) 2
B) 16
C) 9
D) 8
 For the chi-square test for independence,the degrees of freedom used are _____.
For the chi-square test for independence,the degrees of freedom used are _____.A) 2
B) 16
C) 9
D) 8

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
75
In the following table,individuals are cross-classified by their age group and income level.  At the 5% significance level,the critical value is _______.
At the 5% significance level,the critical value is _______.
A) 13.277
B) 11.143
C) 9.488
D) 7.779
 At the 5% significance level,the critical value is _______.
At the 5% significance level,the critical value is _______.A) 13.277
B) 11.143
C) 9.488
D) 7.779

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
76
The following table shows the distribution of employees in an organization.Martha Foreman,an analyst,wants to see if race has a bearing on the position a person holds with this company.  Assuming that race and seniority are independent,which of the following is the expected frequency of Asian directors?
Assuming that race and seniority are independent,which of the following is the expected frequency of Asian directors?
A) 0
B) 1.95
C) 3.91
D) 5.42
 Assuming that race and seniority are independent,which of the following is the expected frequency of Asian directors?
Assuming that race and seniority are independent,which of the following is the expected frequency of Asian directors?A) 0
B) 1.95
C) 3.91
D) 5.42

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
77
The heights (in cm)for a random sample of 60 males were measured.The sample mean is 166.55,the standard deviation is 12.57,the sample kurtosis is 0.12,and the sample skewness is −0.23.The following table shows the heights subdivided into non-overlapping intervals. 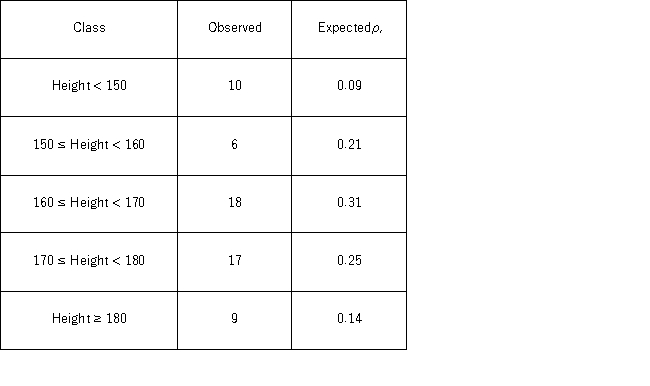 For the goodness-of-fit test for normality,the null and alternative hypothesis are ______________________________________________________________________.
For the goodness-of-fit test for normality,the null and alternative hypothesis are ______________________________________________________________________.
A) H0: Heights follow a normal distribution with mean 166.55 and standard deviation 12.46,HA: Heights do not follow a normal distribution with mean 166.55 and standard deviation 12.46
B) H0: Heights do not follow a normal distribution with mean 166.55 and standard deviation 12.46,HA: Heights follow a normal distribution with mean 166.55 and standard deviation 12.46
C) H0: Heights follow a normal distribution with mean 166.55 and standard deviation 12.57,HA: Heights do not follow a normal distribution with mean 166.55 and standard deviation 12.57
D) H0: Heights do not follow a normal distribution with mean 166.55 and standard deviation 12.57,HA: Heights follow a normal distribution with mean 166.55 and standard deviation 12.57
 For the goodness-of-fit test for normality,the null and alternative hypothesis are ______________________________________________________________________.
For the goodness-of-fit test for normality,the null and alternative hypothesis are ______________________________________________________________________.A) H0: Heights follow a normal distribution with mean 166.55 and standard deviation 12.46,HA: Heights do not follow a normal distribution with mean 166.55 and standard deviation 12.46
B) H0: Heights do not follow a normal distribution with mean 166.55 and standard deviation 12.46,HA: Heights follow a normal distribution with mean 166.55 and standard deviation 12.46
C) H0: Heights follow a normal distribution with mean 166.55 and standard deviation 12.57,HA: Heights do not follow a normal distribution with mean 166.55 and standard deviation 12.57
D) H0: Heights do not follow a normal distribution with mean 166.55 and standard deviation 12.57,HA: Heights follow a normal distribution with mean 166.55 and standard deviation 12.57

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
78
The following table shows the distribution of employees in an organization.Martha Foreman,an analyst,wants to see if race has a bearing on the position a person holds with this company. 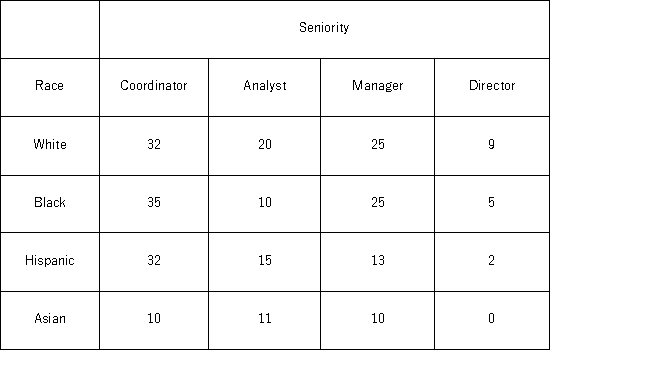 At the 5% significance level,the critical value is ________.
At the 5% significance level,the critical value is ________.
A) 14.684
B) 16.919
C) 19.023
D) 21.666
 At the 5% significance level,the critical value is ________.
At the 5% significance level,the critical value is ________.A) 14.684
B) 16.919
C) 19.023
D) 21.666

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
79
In the following table,individuals are cross-classified by their age group and income level. 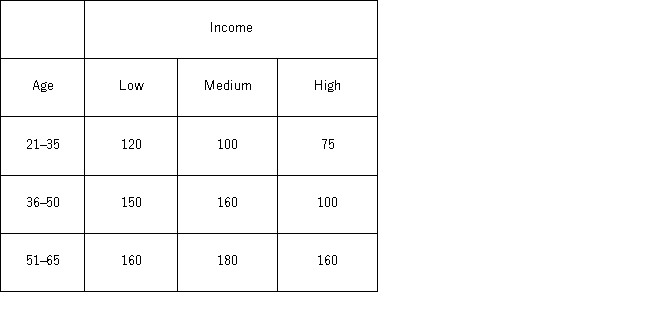 To test that age group and income are independent,the null and alternative hypothesis are _________________________________________________________________________.
To test that age group and income are independent,the null and alternative hypothesis are _________________________________________________________________________.
A) H0: Age group and income are dependent;HA: Age group and income are independent
B) H0: Age group and income are mutually exclusive;HA: Age group and income are not mutually exclusive
C) H0: Age group and income are not mutually exclusive;HA: Age group and income are mutually exclusive
D) H0: Age group and income are independent;HA: Age group and income are dependent
 To test that age group and income are independent,the null and alternative hypothesis are _________________________________________________________________________.
To test that age group and income are independent,the null and alternative hypothesis are _________________________________________________________________________.A) H0: Age group and income are dependent;HA: Age group and income are independent
B) H0: Age group and income are mutually exclusive;HA: Age group and income are not mutually exclusive
C) H0: Age group and income are not mutually exclusive;HA: Age group and income are mutually exclusive
D) H0: Age group and income are independent;HA: Age group and income are dependent

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck
80
In the following table,individuals are cross-classified by their age group and income level.  Using the critical value approach,the decision and conclusion are _________________________________________.
Using the critical value approach,the decision and conclusion are _________________________________________.
A) do not reject the null hypothesis;age and income are dependent
B) do not reject the null hypothesis;age and income are independent
C) reject the null hypothesis;age and income are dependent
D) reject the null hypothesis;age and income are independent
 Using the critical value approach,the decision and conclusion are _________________________________________.
Using the critical value approach,the decision and conclusion are _________________________________________.A) do not reject the null hypothesis;age and income are dependent
B) do not reject the null hypothesis;age and income are independent
C) reject the null hypothesis;age and income are dependent
D) reject the null hypothesis;age and income are independent

Unlock Deck
Unlock for access to all 120 flashcards in this deck.
Unlock Deck
k this deck


