Introduction to Management Science 12th Edition by Bernard Taylor

Exercise 29

For the linear programming model for Southern Sporting Goods Company, formulated in Problem 1 and solved graphically in Problem 2:
Problem 1
Southern Sporting Goods Company makes basketballs and footballs. Each product is produced from two resources-rubber and leather. The resource requirements for each product and the total resources available are as follows:
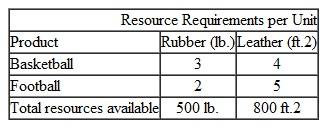Each basketball produced results in a profit of $12, and each football earns $16 in profit.
a. Formulate a linear programming model to determine the number of basketballs and footballs to produce in order to maximize profit.
b. Transform this model into standard form.
Problem 2
Solve the model formulated in Problem for Southern Sporting Goods Company graphically.
a. Identify the amount of unused resources (i.e., slack) at each of the graphical extreme points.
b. What would be the effect on the optimal solution if the profit for a basketball changed from $12 to $13 What would be the effect if the profit for a football changed from $16 to $15
c. What would be the effect on the optimal solution if 500 additional pounds of rubber could be obtained What would be the effect if 500 additional square feet of leather could be obtained
a. Determine the sensitivity ranges for the objective function coefficients and constraint quantity values, using graphical analysis.
b. Verify the sensitivity ranges determined in (a) by using the computer.
c. Using the computer, determine the shadow prices for the resources and explain their meaning.
Problem
Southern Sporting Goods Company makes basketballs and footballs. Each product is produced from two resources-rubber and leather. The resource requirements for each product and the total resources available are as follows:
Each basketball produced results in a profit of $12, and each football earns $16 in profit.
a. Formulate a linear programming model to determine the number of basketballs and footballs to produce in order to maximize profit.
b. Transform this model into standard form.