Introduction to Management Science 12th Edition by Bernard Taylor

Exercise 14

In Problem, assume that the students at Randolph College have redefined the probability distributions of their dates at Salem College as follows: Physical attractiveness is uniformly distributed from 1 to 5; intelligence is defined by a triangular distribution with a minimum rating of 1, a maximum of 5, and a most likely of 2; and personality is defined by a triangular distribution with a minimum of 1, a maximum of 5, and a most likely rating of 3. Develop a simulation model by using Crystal Ball and determine the average date rating (for 1,000 trials). Also compute the probability that the rating will be "better" than 3.0.
Problem
Randolph College and Salem College are within 20 miles of each other, and the students at each college frequently date each other. The students at Randolph College are debating how good their dates are at Salem College. The Randolph students have sampled several hundred of their fellow students and asked them to rate their dates from 1 to 5 (in which 1 is excellent and 5 is poor) according to physical attractiveness, intelligence, and personality. Following are the resulting probability distributions for these three traits for students at Salem College:


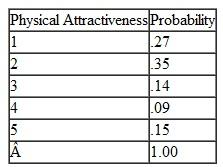
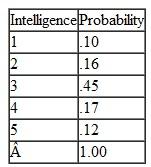
Simulate 20 dates and compute an average overall rating of the Salem students.