Deck 12: Waiting Lines and Queuing Theory Models

Question 1

The goal of most waiting line problems is to identify the service level that minimizes service cost.

Answer 1

False

Question 2

Service times often follow a Poisson distribution.

Answer 2

False

Question 3

Two characteristics of arrivals are the line length and queue discipline.

Answer 3

False

Question 4

A transient state is the normal operating condition of the queuing system.

Question 5

A finite population model differs from an infinite population model because there is a random relationship between the length of the queue and the arrival rate.

Question 6

An (M/D/1)queuing model is classified as probabilistic.

Question 7

On a practical note-if we are using waiting line analysis to study customers calling a telephone number for service, balking is probably not an issue.

Question 8

An automatic car wash is an example of a constant service time model.

Question 9

One difficulty in waiting line analysis is that it is sometimes difficult to place a value on customer waiting time.

Question 10

A bank with a single queue to move customers to several tellers is an example of a single-channel system.

Question 11

In the multichannel model (M/M/m), we must assume that the average service time for all channels is the same.

Question 12

An "infinite calling population" occurs when the likelihood of a new arrival depends upon the number of past arrivals.

Question 13

On a practical note-if we were to study the waiting lines in a hair salon that had only five chairs for patrons waiting, we should use an infinite queue waiting line model.

Question 14

A goal of many waiting line problems is to help a firm find the ideal level of services that minimize the cost of waiting and the cost of providing the service.

Question 15

On a practical note-if we are using waiting line analysis to study cars passing through a single tollbooth, reneging is probably not an issue.

Question 16

An M/M/2 model has Poisson arrivals exponential service times and two channels.

Question 17

On a practical note-we should probably view the checkout counters in a grocery store as a set of single channel systems.

Question 18

In a single-channel, single-phase system, reducing the service time only reduces the total amount of time spent in the system, not the time spent in the queue.

Question 19

A hospital ward with only 30 beds could be modeled using a finite population model.

Question 20

If we are studying the arrival of automobiles at a highway toll station, we can assume an infinite calling population.

Question 21

The customer who arrives at a bank, enters a line, and decides to leave is

A)balking.
B)cropping.
C)reneging.
D)blithering.

Question 22

The customer who arrives at a bank, sees a long line, and leaves to return another time is

A)balking.
B)cropping.
C)reneging.
D)blithering.

Question 23

The rule of thumb for employing a finite population queuing model is if the calling population is eight or fewer.

Question 24

The three major characteristics of the input source that generates arrivals or customers for the service system are

A)size, demographics, and behavior.
B)size, pattern, and behavior.
C)demographics, pattern, and behavior.
D)size, demographics, and pattern.

Question 25

In order to analyze a queue using a single channel single phase model, customer arrivals must be independent of one another but the average arrival rate is invariant.

Question 26

Little's Flow Equations are advantageous because if one characteristic of the operating system is known, the other characteristics can be easily found.

Question 27

A queue system is in a transient state before the steady state is reached.

Question 28

If inventory increases, then flow rate must also increase.

Question 29

In queuing theory, the calling population is another name for

A)the queue size.
B)the servers.
C)the arrivals.
D)the service rate.

Question 30

An arrival in a queue that reneges is one who

A)after joining the queue, becomes impatient and leaves.
B)refuses to join the queue because it is too long.
C)goes through the queue, but never returns.
D)jumps from one queue to another, trying to get through as quickly as possible.

Question 31

The term queue "discipline" describes the

A)degree to which members of the queue renege.
B)sequence in which members of the queue arrived.
C)degree to which members of the queue are orderly and quiet.
D)sequence in which members of the queue are serviced.

Question 32

Little's Law concepts are applicable to all queueing models presented by the authors except the finite calling population situation.

Question 33

Using a simulation model allows one to ignore the common assumptions required to use analytical models.

Question 34

Many real-world systems are too complex to be handled analytically.In this case a simulation model is the most appropriate analysis tool.

Question 35

Percent idle time plus percent utilization equals one.

Question 36

In order to analyze a queue using a single channel single phase model, customer interarrival time must be lower than service time.

Question 37

Little's Flow Equations are applicable for single-channel systems only.

Question 38

Moving from a single channel queuing system to a multichannel queuing system with two servers will cut the queue length and waiting time in half.

Question 39

In queuing analysis, total expected cost is the sum of expected ________ plus expected ________.

A)service costs, arrival costs
B)facility costs, calling costs
C)calling costs, waiting costs
D)service costs, waiting costs

Question 40

Assume that we are using a waiting line model to analyze the number of service technicians required to maintain machines in a factory.Our goal should be to

A)minimize the number of machines needing repair.
B)minimize the downtime for individual machines.
C)minimize the percent of idle time of the technicians.
D)minimize the total cost (cost of maintenance plus cost of downtime).

Question 41

The initial state of a queuing system (for example, when a restaurant first opens), is referred to as the ________ state.

A)steady
B)primary
C)introductory
D)transient

Question 42

A professor holds office hours during final exam week and discovers much to his dismay that his sudden popularity affords him little time to engage in his favorite activity, watching cat videos.Fifteen students per hour arrive at his office and he patiently answers questions, averaging three minutes per student.On average, how many students are waiting to ask questions?

A)2.00
B)2.25
C)2.50
D)2.75

Question 43

A vendor selling newspapers on a street corner is an example of a

A)single-channel, multiphase system.
B)single-channel, single-phase system.
C)multichannel, multiphase system.
D)multichannel, single-phase system.

Question 44

A professor holds office hours during final exam week and discovers much to his dismay that his sudden popularity affords him little time to engage in his favorite activity, watching cat videos.Fifteen students per hour arrive at his office and he patiently answers questions, averaging three minutes per student.On average, how long must a student wait in line to get their last-minute questions answered?

A)3 minutes
B)6 minutes
C)9 minutes
D)12 minutes

Question 45

Customers enter the waiting line at a cafeteria on a first-come, first-served basis.The arrival rate follows a Poisson distribution, while service times follow an exponential distribution.If the average number of arrivals is four per minute and the average service rate of a single server is seven per minute, what is the average number of customers in the system?

A)0.43
B)1.67
C)0.57
D)1.33

Question 46

Customers enter the waiting line to pay for food as they leave a cafeteria on a first-come, first-served basis.The arrival rate follows a Poisson distribution, while service times follow an exponential distribution.If the average number of arrivals is four per minute and the average service rate of a single server is seven per minute, what proportion of the time is the server busy?

A)0.43
B)0.57
C)0.75
D)0.25

Question 47

Which of the following distributions is most often used to estimate service times?

A)negative exponential
B)normal
C)Poisson
D)Erlang

Question 48

At a local fast-food joint, cars arrive randomly at a rate of 12 every 30 minutes.The fast food joint takes an average of 2 minutes to serve each arrival.The utilization factor for this system is

A)0.467.
B)0.547.
C)0.800.
D)0.133.

Question 49

At a local fast food joint, cars arrive randomly at a rate of 12 every 30 minutes.Service times are random (exponential)and average 2 minutes per arrival.The average time in the queue for each arrival is

A)2 minutes.
B)4 minutes.
C)6 minutes.
D)8 minutes.

Question 50

Most systems use a queue discipline known as

A)shortest processing time.
B)longest processing time.
C)FIFO.
D)earliest due date.

Question 51

A professor holds office hours during final exam week and discovers much to his dismay that his sudden popularity affords him little time to engage in his favorite activity, watching cat videos.Fifteen students per hour arrive at his office and he patiently answers questions, averaging three minutes per student.A student joins the line at 9:45 am and the test is at 10:00 am the same morning.How much time does the student have after getting his question answered to get to the exam room?

A)12 minutes
B)9 minutes
C)6 minutes
D)3 minutes

Question 52

A single automatic car wash with one bay and a service time that is normally distributed is what type of model?

A)M/M/1
B)M/D/2
C)M/D/1
D)M/G/1

Question 53

Which of the following distributions is most often used to estimate the arrival pattern?

A)negative exponential
B)normal
C)Poisson
D)Erlang

Question 54

A suburban specialty restaurant has developed a single drive-thru window.Customers order, pay, and pick up their food at the same window.Arrivals follow a Poisson distribution, while service times follow an exponential distribution.What type of queuing model is exhibited in this problem?

A)M/M/1
B)M/M/2
C)M/D/2
D)M/D/1

Question 55

A queuing system with a normally distributed arrival pattern, exponential service times, and three servers would be described as

A)G/G/3.
B)M/M/3.
C)G/M/3.
D)M/G/3.

Question 56

A single automatic car wash with one bay and a cycle time of 2.5 minutes is what type of model?

A)M/M/1
B)M/M/2
C)M/D/2
D)M/D/1

Question 57

Customers enter the waiting line to pay for food as they leave a cafeteria on a first-come, first-served basis.The arrival rate follows a Poisson distribution, while service times follow an exponential distribution.If the average number of arrivals is four per minute and the average service rate of a single server is seven per minute, on average, how much time will elapse from the time a customer enters the line until he/she leaves the cafeteria?

A)0.67 minute
B)0.50 minute
C)0.75 minute
D)0.33 minute

Question 58

Customers enter the waiting line at a cafeteria on a first-come, first-served basis.The arrival rate follows a Poisson distribution, while service times follow an exponential distribution.If the average number of arrivals is four per minute and the average service rate of a single server is seven per minute, what is the average number of customers waiting in line behind the person being served?

A)0.76
B)0.19
C)1.33
D)1.67

Question 59

Lines at banks where customers wait for a teller window are usually representative of a

A)single-channel, multiphase system.
B)single-channel, single-phase system.
C)multichannel, multiphase system.
D)multichannel, single-phase system.

Question 60

A professor holds office hours during final exam week and discovers much to his dismay that his sudden popularity affords him little time to engage in his favorite activity, watching cat videos.Fifteen students per hour arrive at his office and he patiently answers questions, averaging three minutes per student.If the average cat video is 60 seconds, how many videos can the professor watch in an hour?

A)15
B)18
C)20
D)24

Question 61

If everything else remains constant, including the mean arrival rate and service rate, except that the service time becomes constant instead of exponential

A)the average queue length will be halved.
B)the average waiting time will be doubled.
C)the average queue length will be doubled.
D)There is not enough information to know what will happen to the queue length and waiting time.

Question 62

A post office has a single line for customers waiting for the next available postal clerk.There are two postal clerks who work at the same rate.The arrival rate of customers follows a Poisson distribution, while the service time follows an exponential distribution.What type of queuing model applies here?

A)M/M/1
B)M/M/2
C)M/D/2
D)M/D/1

Question 63

Table 12-3

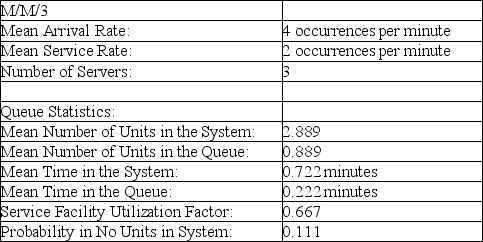
According to the information provided in Table 12-3, which presents a queuing problem solution, what proportion of time is the system totally empty?

A)0.111
B)0.333
C)0.889
D)0.667

Question 64

Table 12-2

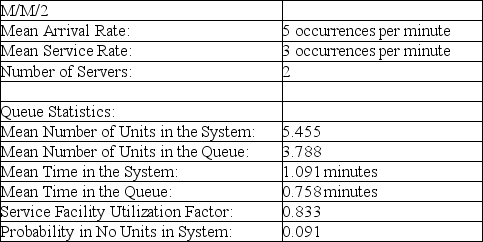
According to the information provided in Table 12-2, which presents a queuing problem solution, there are two servers in this system.Counting each person being served and the people in line, on average, how many people would be in this system?

A)5.455
B)3.788
C)9.243
D)10.900

Question 65

Table 12-1

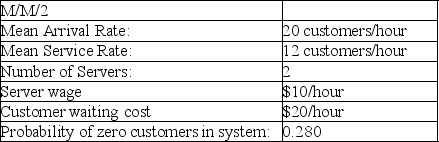
According to the information provided in Table 12-1, what percentage of the total available service time is being used?

A)83.0%
B)87.0%
C)78.3%
D)60.0%

Question 66

Table 12-2

According to the information provided in Table 12-2, which presents a queuing problem solution, on average, how many units are in the line?

A)5.455
B)3.788
C)1.091
D)0.758
E)0.833

Question 67

Table 12-1

According to the information provided in Table 12-1, how long does a customer wait on average?

A)11 minutes
B)11 minutes 30 seconds
C)12 minutes 15 seconds
D)12 minutes 45 seconds

Question 68

Table 12-1

According to the information provided in Table 12-1, on average, how many customers are in the line?

A)3.79
B)3.33
C)4.25
D)4.33

Question 69

A post office has a single line for customers waiting for the next available postal clerk.There are two postal clerks who work at the same rate.The arrival rate of customers follows a Poisson distribution, while the service time follows a normal distribution.What type of queuing model applies here?

A)M/M/2
B)M/D/2
C)M/D/1
D)M/G/2

Question 70

Table 12-2

According to the information provided in Table 12-2, which presents a queuing problem solution, what proportion of time is at least one server busy?

A)0.833
B)0.758
C)0.091
D)0.909

Question 71

A post office has a single line for customers waiting for the next available postal clerk.There are two postal clerks who work at the same rate.The arrival rate of customers follows a Poisson distribution, while the service time follows an exponential distribution.The average arrival rate is three per minute and the average service rate is two per minute for each of the two clerks.How long does the average person spend waiting for a clerk?

A)3.429 minutes
B)1.929 minutes
C)1.143 minutes
D)0.643 minute

Question 72

At an automatic car wash, cars arrive randomly at a rate of 7 every 30 minutes.The car wash takes exactly 4 minutes (this is constant).On average, how long would each car spend at the car wash?

A)28 minutes
B)32 minutes
C)17 minutes
D)24 minutes

Question 73

At an automatic car wash, cars arrive randomly at a rate of 7 cars every 30 minutes.The car wash takes exactly 4 minutes (this is constant).On average, what would be the length of the line?

A)8.171
B)7.467
C)6.53
D)0.467

Question 74

Table 12-1

According to the information provided in Table 12-1, what is the hourly total cost of the system for customers and servers? Assume that servers are paid only when they are helping customers.

A)$117.67
B)$121.47
C)$129.09
D)$125.76

Question 75

Table 12-1

Using the information provided in Table 12-1, counting each person being served and the people in line, on average, how many customers would be in this system?

A)4.85
B)5.05
C)5.45
D)6.15

Question 76

Table 12-3

According to the information provided in Table 12-3, which presents a queuing problem solution, what is the utilization rate of the service facility?

A)0.889
B)0.222
C)0.722
D)0.667

Question 77

A post office has a single line for customers waiting for the next available postal clerk.There are two postal clerks who work at the same rate.The arrival rate of customers follows a Poisson distribution, while the service time follows an exponential distribution.The average arrival rate is three per minute and the average service rate is two per minute for each of the two clerks.What proportion of time are both clerks idle?

A)0.643
B)0.250
C)0.750
D)0.143

Question 78

Table 12-1

According to the information provided in Table 12-1, what is the average amount of time a customer waits and is served?

A)15 minutes 40 seconds
B)16 minutes 20 seconds
C)17 minutes
D)17 minutes 40 seconds

Question 79

Table 12-3

According to the information provided in Table 12-3, which presents a queuing problem solution, on average, how long does each customer spend waiting in line?

A)0.333 minute
B)0.889 minute
C)0.222 minute
D)0.722 minute

Question 80

A post office has a single line for customers waiting for the next available postal clerk.There are two postal clerks who work at the same rate.The arrival rate of customers follows a Poisson distribution, while the service time follows an exponential distribution.The average arrival rate is three per minute and the average service rate is two per minute for each of the two clerks.What is the average length of the line?

A)3.429
B)1.929
C)1.143
D)0.643