Deck 11: Analysis of Variance
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/40
Play
Full screen (f)
Deck 11: Analysis of Variance
1
Use the following sample data to answer the next questions. For a study on
Type 1 diabetes, medical graduate students subdivided the United States into four
study regions (Northeast, Southeast, Southwest, and Northwest). The students
randomly selected seven patients per region and recorded the number of times
during a randomly selected month that each patient used insulin shots to regulate
blood sugar levels. Use One-Way ANOVA at a .05 significance level to test the
claim that the means from the different regions are not the same.
Mean number of times patients used insulin shots to regulate blood sugar levels
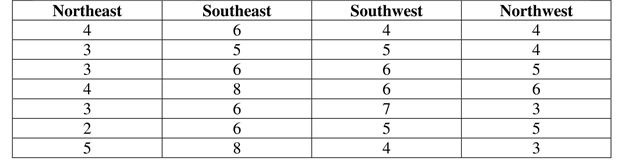
Determine the value of the F test statistic.
Type 1 diabetes, medical graduate students subdivided the United States into four
study regions (Northeast, Southeast, Southwest, and Northwest). The students
randomly selected seven patients per region and recorded the number of times
during a randomly selected month that each patient used insulin shots to regulate
blood sugar levels. Use One-Way ANOVA at a .05 significance level to test the
claim that the means from the different regions are not the same.
Mean number of times patients used insulin shots to regulate blood sugar levels

Determine the value of the F test statistic.
test statistic F = 9.94
2
Determine whether the following statement is true or false.
Assuming calculations for a One-Way ANOVA with equal sample sizes, if each data value in one of the samples is increased by a fixed amount, then any change in the F test statistic and the P-value is attributable only to the change in the sample mean.
Assuming calculations for a One-Way ANOVA with equal sample sizes, if each data value in one of the samples is increased by a fixed amount, then any change in the F test statistic and the P-value is attributable only to the change in the sample mean.
True
3
Use the following technology display from a Two-Way ANOVA to answer the next
questions. Wildlife biologists studying beaver habitats measured the number
of beaver colonies per square mile identified at two randomly selected wetland
habitats, under three densities of tree growth (sparse, average, or dense growth).
Use a .05 significance level.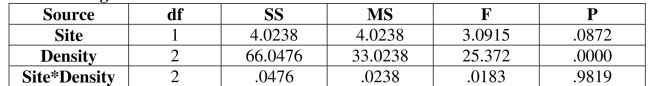
For the density effect, state a conclusion about the null hypothesis.
questions. Wildlife biologists studying beaver habitats measured the number
of beaver colonies per square mile identified at two randomly selected wetland
habitats, under three densities of tree growth (sparse, average, or dense growth).
Use a .05 significance level.

For the density effect, state a conclusion about the null hypothesis.
Reject 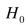 .
.
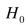 .
. 4
Use the following sample data to answer the next questions. For a study on
Type 1 diabetes, medical graduate students subdivided the United States into four
study regions (Northeast, Southeast, Southwest, and Northwest). The students
randomly selected seven patients per region and recorded the number of times
during a randomly selected month that each patient used insulin shots to regulate
blood sugar levels. Use One-Way ANOVA at a .05 significance level to test the
claim that the means from the different regions are not the same.
Mean number of times patients used insulin shots to regulate blood sugar levels

State a final conclusion that addresses the original claim.
Type 1 diabetes, medical graduate students subdivided the United States into four
study regions (Northeast, Southeast, Southwest, and Northwest). The students
randomly selected seven patients per region and recorded the number of times
during a randomly selected month that each patient used insulin shots to regulate
blood sugar levels. Use One-Way ANOVA at a .05 significance level to test the
claim that the means from the different regions are not the same.
Mean number of times patients used insulin shots to regulate blood sugar levels

State a final conclusion that addresses the original claim.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
5
Use the following technology display from a Two-Way ANOVA to answer the next
questions. Wildlife biologists studying beaver habitats measured the number
of beaver colonies per square mile identified at two randomly selected wetland
habitats, under three densities of tree growth (sparse, average, or dense growth).
Use a .05 significance level.
For the interaction effect, state a conclusion about the null hypothesis.
questions. Wildlife biologists studying beaver habitats measured the number
of beaver colonies per square mile identified at two randomly selected wetland
habitats, under three densities of tree growth (sparse, average, or dense growth).
Use a .05 significance level.

For the interaction effect, state a conclusion about the null hypothesis.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
6
Determine whether the following statement is true or false.
Assuming that differences exist among the population means, the One-Way ANOVA can determine which population mean(s) differ from the others.
Assuming that differences exist among the population means, the One-Way ANOVA can determine which population mean(s) differ from the others.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
7
Determine whether the following statement is true or false.
One method to reduce the effect of extraneous factors is to design the experiment so that it has a completely randomized design.
One method to reduce the effect of extraneous factors is to design the experiment so that it has a completely randomized design.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
8
Use the following technology display from a Two-Way ANOVA to answer the next
questions. Wildlife biologists studying beaver habitats measured the number
of beaver colonies per square mile identified at two randomly selected wetland
habitats, under three densities of tree growth (sparse, average, or dense growth).
Use a .05 significance level.
State a final conclusion about the overall study.
questions. Wildlife biologists studying beaver habitats measured the number
of beaver colonies per square mile identified at two randomly selected wetland
habitats, under three densities of tree growth (sparse, average, or dense growth).
Use a .05 significance level.

State a final conclusion about the overall study.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
9
Use the following technology display from a Two-Way ANOVA to answer the next
questions. Wildlife biologists studying beaver habitats measured the number
of beaver colonies per square mile identified at two randomly selected wetland
habitats, under three densities of tree growth (sparse, average, or dense growth).
Use a .05 significance level.
For the site effect, state a conclusion about the null hypothesis.
questions. Wildlife biologists studying beaver habitats measured the number
of beaver colonies per square mile identified at two randomly selected wetland
habitats, under three densities of tree growth (sparse, average, or dense growth).
Use a .05 significance level.

For the site effect, state a conclusion about the null hypothesis.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
10
Determine whether the following statement is true or false.
If there is only one observation per cell in a Two-Way ANOVA, and it can be assumed there is an interaction between factors, then we can proceed to interpret the results of the row and column effects.
If there is only one observation per cell in a Two-Way ANOVA, and it can be assumed there is an interaction between factors, then we can proceed to interpret the results of the row and column effects.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
11
Use the following sample data to answer the next questions. For a study on
Type 1 diabetes, medical graduate students subdivided the United States into four
study regions (Northeast, Southeast, Southwest, and Northwest). The students
randomly selected seven patients per region and recorded the number of times
during a randomly selected month that each patient used insulin shots to regulate
blood sugar levels. Use One-Way ANOVA at a .05 significance level to test the
claim that the means from the different regions are not the same.
Mean number of times patients used insulin shots to regulate blood sugar levels

Identify the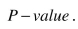
Type 1 diabetes, medical graduate students subdivided the United States into four
study regions (Northeast, Southeast, Southwest, and Northwest). The students
randomly selected seven patients per region and recorded the number of times
during a randomly selected month that each patient used insulin shots to regulate
blood sugar levels. Use One-Way ANOVA at a .05 significance level to test the
claim that the means from the different regions are not the same.
Mean number of times patients used insulin shots to regulate blood sugar levels

Identify the
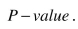

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
12
Use the following sample data to answer the next questions. For a study on
Type 1 diabetes, medical graduate students subdivided the United States into four
study regions (Northeast, Southeast, Southwest, and Northwest). The students
randomly selected seven patients per region and recorded the number of times
during a randomly selected month that each patient used insulin shots to regulate
blood sugar levels. Use One-Way ANOVA at a .05 significance level to test the
claim that the means from the different regions are not the same.
Mean number of times patients used insulin shots to regulate blood sugar levels

Identify the alternative hypothesis.
Type 1 diabetes, medical graduate students subdivided the United States into four
study regions (Northeast, Southeast, Southwest, and Northwest). The students
randomly selected seven patients per region and recorded the number of times
during a randomly selected month that each patient used insulin shots to regulate
blood sugar levels. Use One-Way ANOVA at a .05 significance level to test the
claim that the means from the different regions are not the same.
Mean number of times patients used insulin shots to regulate blood sugar levels

Identify the alternative hypothesis.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
13
Use the following technology display from a Two-Way ANOVA to answer the next
questions. Wildlife biologists studying beaver habitats measured the number
of beaver colonies per square mile identified at two randomly selected wetland
habitats, under three densities of tree growth (sparse, average, or dense growth).
Use a .05 significance level.
For the interaction effect, identify the null hypothesis, the value of the F test statistic, and the .
.
questions. Wildlife biologists studying beaver habitats measured the number
of beaver colonies per square mile identified at two randomly selected wetland
habitats, under three densities of tree growth (sparse, average, or dense growth).
Use a .05 significance level.

For the interaction effect, identify the null hypothesis, the value of the F test statistic, and the
 .
.
Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
14
Use the following sample data to answer the next questions. For a study on
Type 1 diabetes, medical graduate students subdivided the United States into four
study regions (Northeast, Southeast, Southwest, and Northwest). The students
randomly selected seven patients per region and recorded the number of times
during a randomly selected month that each patient used insulin shots to regulate
blood sugar levels. Use One-Way ANOVA at a .05 significance level to test the
claim that the means from the different regions are not the same.
Mean number of times patients used insulin shots to regulate blood sugar levels

Identify the null hypothesis.
Type 1 diabetes, medical graduate students subdivided the United States into four
study regions (Northeast, Southeast, Southwest, and Northwest). The students
randomly selected seven patients per region and recorded the number of times
during a randomly selected month that each patient used insulin shots to regulate
blood sugar levels. Use One-Way ANOVA at a .05 significance level to test the
claim that the means from the different regions are not the same.
Mean number of times patients used insulin shots to regulate blood sugar levels

Identify the null hypothesis.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
15
Use the following sample data to answer the next questions. For a study on
Type 1 diabetes, medical graduate students subdivided the United States into four
study regions (Northeast, Southeast, Southwest, and Northwest). The students
randomly selected seven patients per region and recorded the number of times
during a randomly selected month that each patient used insulin shots to regulate
blood sugar levels. Use One-Way ANOVA at a .05 significance level to test the
claim that the means from the different regions are not the same.
Mean number of times patients used insulin shots to regulate blood sugar levels

State a conclusion about the null hypothesis.
Type 1 diabetes, medical graduate students subdivided the United States into four
study regions (Northeast, Southeast, Southwest, and Northwest). The students
randomly selected seven patients per region and recorded the number of times
during a randomly selected month that each patient used insulin shots to regulate
blood sugar levels. Use One-Way ANOVA at a .05 significance level to test the
claim that the means from the different regions are not the same.
Mean number of times patients used insulin shots to regulate blood sugar levels

State a conclusion about the null hypothesis.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
16
Use the following technology display from a Two-Way ANOVA to answer the next
questions. Wildlife biologists studying beaver habitats measured the number
of beaver colonies per square mile identified at two randomly selected wetland
habitats, under three densities of tree growth (sparse, average, or dense growth).
Use a .05 significance level.
For the density effect, identify the null hypothesis, the value of the F test statistic, and the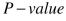 .
.
questions. Wildlife biologists studying beaver habitats measured the number
of beaver colonies per square mile identified at two randomly selected wetland
habitats, under three densities of tree growth (sparse, average, or dense growth).
Use a .05 significance level.

For the density effect, identify the null hypothesis, the value of the F test statistic, and the
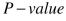 .
.
Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
17
Use the following technology display from a Two-Way ANOVA to answer the next
questions. Wildlife biologists studying beaver habitats measured the number
of beaver colonies per square mile identified at two randomly selected wetland
habitats, under three densities of tree growth (sparse, average, or dense growth).
Use a .05 significance level.
For the site effect, identify the null hypothesis, the value of the F test statistic, and the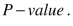
questions. Wildlife biologists studying beaver habitats measured the number
of beaver colonies per square mile identified at two randomly selected wetland
habitats, under three densities of tree growth (sparse, average, or dense growth).
Use a .05 significance level.

For the site effect, identify the null hypothesis, the value of the F test statistic, and the
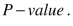

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
18
Determine whether the following statement is true or false.
The reason we cannot use two One-Way ANOVAs instead of using a Two-Way ANOVA is that this would ignore the effect of interaction between the two factors.
The reason we cannot use two One-Way ANOVAs instead of using a Two-Way ANOVA is that this would ignore the effect of interaction between the two factors.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
19
Determine whether the following statement is true or false.
For a Two-Way ANOVA, assuming there is an interaction, we can continue to interpret the results of the row and column effects.
For a Two-Way ANOVA, assuming there is an interaction, we can continue to interpret the results of the row and column effects.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
20
Determine whether the following statement is true or false.
The reason we cannot use multiple t-tests to claim that four populations have the same mean is that we increase the likelihood of a type II error.
The reason we cannot use multiple t-tests to claim that four populations have the same mean is that we increase the likelihood of a type II error.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
21
Use the following technology display from a Two-Way ANOVA to answer the next
questions. Biologists studying habitat use in Lepidopteran moths measured
the number of savannah moths found at three randomly selected prairie sites with
two potential habitat interferences (expansion of row crops and grazing). Use a .05
significance level.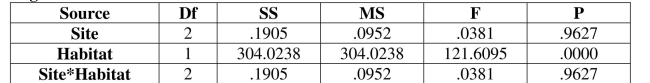
For the habitat effect, state a conclusion about the null hypothesis.
questions. Biologists studying habitat use in Lepidopteran moths measured
the number of savannah moths found at three randomly selected prairie sites with
two potential habitat interferences (expansion of row crops and grazing). Use a .05
significance level.

For the habitat effect, state a conclusion about the null hypothesis.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
22
Use the following sample data to answer the next questions. Geneticists
studying carriers of genetic diseases followed subjects subdivided by race.
Researchers randomly selected seven patients per race who had been identified as
carrying a certain gene for a genetic disease; these patients were followed to
determine the number of their siblings who also carried the gene for the genetic
disease. Use a One-Way ANOVA at a .05 significance level to test the claim that
the means from the different races are not all the same.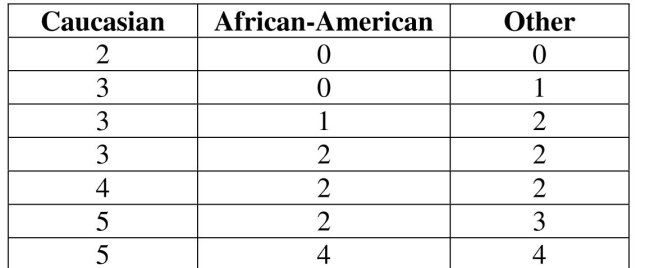
Identify the alternative hypothesis.
studying carriers of genetic diseases followed subjects subdivided by race.
Researchers randomly selected seven patients per race who had been identified as
carrying a certain gene for a genetic disease; these patients were followed to
determine the number of their siblings who also carried the gene for the genetic
disease. Use a One-Way ANOVA at a .05 significance level to test the claim that
the means from the different races are not all the same.

Identify the alternative hypothesis.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
23
Use the following technology display from a Two-Way ANOVA to answer the next
questions. Biologists studying habitat use in Lepidopteran moths measured
the number of savannah moths found at three randomly selected prairie sites with
two potential habitat interferences (expansion of row crops and grazing). Use a .05
significance level.
State a final conclusion about the overall study.
questions. Biologists studying habitat use in Lepidopteran moths measured
the number of savannah moths found at three randomly selected prairie sites with
two potential habitat interferences (expansion of row crops and grazing). Use a .05
significance level.

State a final conclusion about the overall study.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
24
Use the following technology display from a Two-Way ANOVA to answer the next
questions. Biologists studying habitat use in Lepidopteran moths measured
the number of savannah moths found at three randomly selected prairie sites with
two potential habitat interferences (expansion of row crops and grazing). Use a .05
significance level.
For the site effect, state a conclusion about the null hypothesis.
questions. Biologists studying habitat use in Lepidopteran moths measured
the number of savannah moths found at three randomly selected prairie sites with
two potential habitat interferences (expansion of row crops and grazing). Use a .05
significance level.

For the site effect, state a conclusion about the null hypothesis.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
25
Determine whether the following statement is true or false.
Assuming calculations for a One-Way ANOVA with equal sample sizes, if each data value in one of the samples is increased by a fixed amount, then any change in the F test statistic and the P-value is attributable only to the change in the sample variances.
Assuming calculations for a One-Way ANOVA with equal sample sizes, if each data value in one of the samples is increased by a fixed amount, then any change in the F test statistic and the P-value is attributable only to the change in the sample variances.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
26
Use the following sample data to answer the next questions. Geneticists
studying carriers of genetic diseases followed subjects subdivided by race.
Researchers randomly selected seven patients per race who had been identified as
carrying a certain gene for a genetic disease; these patients were followed to
determine the number of their siblings who also carried the gene for the genetic
disease. Use a One-Way ANOVA at a .05 significance level to test the claim that
the means from the different races are not all the same.
State a final conclusion that addresses the original claim.
studying carriers of genetic diseases followed subjects subdivided by race.
Researchers randomly selected seven patients per race who had been identified as
carrying a certain gene for a genetic disease; these patients were followed to
determine the number of their siblings who also carried the gene for the genetic
disease. Use a One-Way ANOVA at a .05 significance level to test the claim that
the means from the different races are not all the same.

State a final conclusion that addresses the original claim.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
27
Determine whether the following statement is true or false.
Assuming differences exist among the population means, the Bonferroni test can determine which population means differ from the others.
Assuming differences exist among the population means, the Bonferroni test can determine which population means differ from the others.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
28
Determine whether the following statement is true or false.
If there is only one observation per cell in a Two-Way ANOVA, and it can be assumed there is not an interaction between factors, then we can proceed to interpret the results of the row and column effects.
If there is only one observation per cell in a Two-Way ANOVA, and it can be assumed there is not an interaction between factors, then we can proceed to interpret the results of the row and column effects.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
29
Determine whether the following statement is true or false.
The reason we cannot use multiple t-tests to claim that four populations have the same mean is that we increase the likelihood of a type I error.
The reason we cannot use multiple t-tests to claim that four populations have the same mean is that we increase the likelihood of a type I error.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
30
Determine whether the following statement is true or false.
Another way to conduct a Two-Way ANOVA is to conduct two separate One-Way ANOVAs.
Another way to conduct a Two-Way ANOVA is to conduct two separate One-Way ANOVAs.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
31
Use the following technology display from a Two-Way ANOVA to answer the next
questions. Biologists studying habitat use in Lepidopteran moths measured
the number of savannah moths found at three randomly selected prairie sites with
two potential habitat interferences (expansion of row crops and grazing). Use a .05
significance level.
For the interaction effect, state a conclusion about the null hypothesis.
questions. Biologists studying habitat use in Lepidopteran moths measured
the number of savannah moths found at three randomly selected prairie sites with
two potential habitat interferences (expansion of row crops and grazing). Use a .05
significance level.

For the interaction effect, state a conclusion about the null hypothesis.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
32
Determine whether the following statement is true or false.
For a Two-Way ANOVA, assuming there is not an interaction, we can continue to interpret the results of the row and column effects.
For a Two-Way ANOVA, assuming there is not an interaction, we can continue to interpret the results of the row and column effects.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
33
Use the following technology display from a Two-Way ANOVA to answer the next
questions. Biologists studying habitat use in Lepidopteran moths measured
the number of savannah moths found at three randomly selected prairie sites with
two potential habitat interferences (expansion of row crops and grazing). Use a .05
significance level.
For the interaction effect, identify the null hypothesis, the value of the F test statistic, and the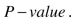
questions. Biologists studying habitat use in Lepidopteran moths measured
the number of savannah moths found at three randomly selected prairie sites with
two potential habitat interferences (expansion of row crops and grazing). Use a .05
significance level.

For the interaction effect, identify the null hypothesis, the value of the F test statistic, and the
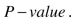

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
34
Use the following technology display from a Two-Way ANOVA to answer the next
questions. Biologists studying habitat use in Lepidopteran moths measured
the number of savannah moths found at three randomly selected prairie sites with
two potential habitat interferences (expansion of row crops and grazing). Use a .05
significance level.
For the site effect, identify the null hypothesis, the value of the F test statistic, and the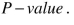
questions. Biologists studying habitat use in Lepidopteran moths measured
the number of savannah moths found at three randomly selected prairie sites with
two potential habitat interferences (expansion of row crops and grazing). Use a .05
significance level.

For the site effect, identify the null hypothesis, the value of the F test statistic, and the
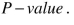

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
35
Use the following sample data to answer the next questions. Geneticists
studying carriers of genetic diseases followed subjects subdivided by race.
Researchers randomly selected seven patients per race who had been identified as
carrying a certain gene for a genetic disease; these patients were followed to
determine the number of their siblings who also carried the gene for the genetic
disease. Use a One-Way ANOVA at a .05 significance level to test the claim that
the means from the different races are not all the same.
State a conclusion about the null hypothesis.
studying carriers of genetic diseases followed subjects subdivided by race.
Researchers randomly selected seven patients per race who had been identified as
carrying a certain gene for a genetic disease; these patients were followed to
determine the number of their siblings who also carried the gene for the genetic
disease. Use a One-Way ANOVA at a .05 significance level to test the claim that
the means from the different races are not all the same.

State a conclusion about the null hypothesis.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
36
Determine whether the following statement is true or false.
One method to reduce the effect of extraneous factors is to design the experiment so that it has a convenience sampling design.
One method to reduce the effect of extraneous factors is to design the experiment so that it has a convenience sampling design.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
37
Use the following sample data to answer the next questions. Geneticists
studying carriers of genetic diseases followed subjects subdivided by race.
Researchers randomly selected seven patients per race who had been identified as
carrying a certain gene for a genetic disease; these patients were followed to
determine the number of their siblings who also carried the gene for the genetic
disease. Use a One-Way ANOVA at a .05 significance level to test the claim that
the means from the different races are not all the same.
Identify the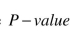 .
.
studying carriers of genetic diseases followed subjects subdivided by race.
Researchers randomly selected seven patients per race who had been identified as
carrying a certain gene for a genetic disease; these patients were followed to
determine the number of their siblings who also carried the gene for the genetic
disease. Use a One-Way ANOVA at a .05 significance level to test the claim that
the means from the different races are not all the same.

Identify the
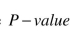 .
.
Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
38
Use the following technology display from a Two-Way ANOVA to answer the next
questions. Biologists studying habitat use in Lepidopteran moths measured
the number of savannah moths found at three randomly selected prairie sites with
two potential habitat interferences (expansion of row crops and grazing). Use a .05
significance level.
For the habitat effect, identify the null hypothesis, the value of the F test statistic, and the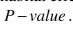
questions. Biologists studying habitat use in Lepidopteran moths measured
the number of savannah moths found at three randomly selected prairie sites with
two potential habitat interferences (expansion of row crops and grazing). Use a .05
significance level.

For the habitat effect, identify the null hypothesis, the value of the F test statistic, and the
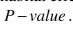

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
39
Use the following sample data to answer the next questions. Geneticists
studying carriers of genetic diseases followed subjects subdivided by race.
Researchers randomly selected seven patients per race who had been identified as
carrying a certain gene for a genetic disease; these patients were followed to
determine the number of their siblings who also carried the gene for the genetic
disease. Use a One-Way ANOVA at a .05 significance level to test the claim that
the means from the different races are not all the same.
Identify the null hypothesis.
studying carriers of genetic diseases followed subjects subdivided by race.
Researchers randomly selected seven patients per race who had been identified as
carrying a certain gene for a genetic disease; these patients were followed to
determine the number of their siblings who also carried the gene for the genetic
disease. Use a One-Way ANOVA at a .05 significance level to test the claim that
the means from the different races are not all the same.

Identify the null hypothesis.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck
40
Use the following sample data to answer the next questions. Geneticists
studying carriers of genetic diseases followed subjects subdivided by race.
Researchers randomly selected seven patients per race who had been identified as
carrying a certain gene for a genetic disease; these patients were followed to
determine the number of their siblings who also carried the gene for the genetic
disease. Use a One-Way ANOVA at a .05 significance level to test the claim that
the means from the different races are not all the same.
Determine the value of the F test statistic.
studying carriers of genetic diseases followed subjects subdivided by race.
Researchers randomly selected seven patients per race who had been identified as
carrying a certain gene for a genetic disease; these patients were followed to
determine the number of their siblings who also carried the gene for the genetic
disease. Use a One-Way ANOVA at a .05 significance level to test the claim that
the means from the different races are not all the same.

Determine the value of the F test statistic.

Unlock Deck
Unlock for access to all 40 flashcards in this deck.
Unlock Deck
k this deck


