Deck 6: Conic Sections
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/97
Play
Full screen (f)
Deck 6: Conic Sections
1
Find the vertex, focus, and directrix of the parabola with the given equation.
A) vertex:
focus:
directrix:
B) vertex:
focus:
directrix:
C) vertex:
focus:
directrix:
D) vertex:
focus:
directrix:
A) vertex:
focus:
directrix:
B) vertex:
focus:
directrix:
C) vertex:
focus:
directrix:
D) vertex:
focus:
directrix:
D
2
Find the vertex, focus, and directrix of the parabola with the given equation.
A) vertex:
focus:
directrix:
B) vertex:
focus:
directrix:
C) vertex:
focus:
directrix:
D) vertex:
focus:
directrix:
A) vertex:
focus:
directrix:
B) vertex:
focus:
directrix:
C) vertex:
focus:
directrix:
D) vertex:
focus:
directrix:
A
3
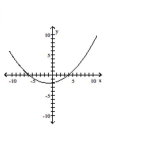
Graph the equation.

A)
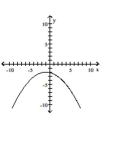
B)
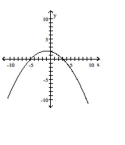
C)
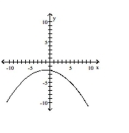
D)

A)

B)

C)

D)

A
4
Find the vertex, focus, and directrix of the parabola. Graph the equation.
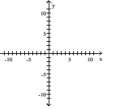
A) vertex:
focus:
directrix:
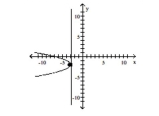
B) vertex:
focus:
directrix:
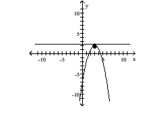
C) vertex:
focus:
directrix:
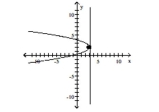
D) vertex:
focus:
directrix:


A) vertex:
focus:
directrix:

B) vertex:
focus:
directrix:

C) vertex:
focus:
directrix:

D) vertex:
focus:
directrix:


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
5
Determine if each ordered pair is a solution to the given system of inequalities in two variables.
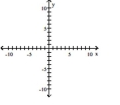
A)
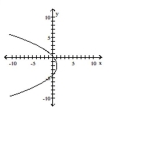
B)
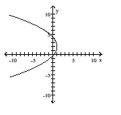
C)

D)
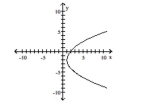

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
6
Find the vertex, focus, and directrix of the parabola with the given equation.
A) vertex:
focus:
directrix:
B) vertex:
focus:
directrix:
C) vertex:
focus:
directrix:
D) vertex:
focus:
directrix:
A) vertex:
focus:
directrix:
B) vertex:
focus:
directrix:
C) vertex:
focus:
directrix:
D) vertex:
focus:
directrix:

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
7
Determine if each ordered pair is a solution to the given system of inequalities in two variables.
The vertex has coordinates (0, 0), and the focus has coordinates (6, 0). A)
B)
C)
D)
The vertex has coordinates (0, 0), and the focus has coordinates (6, 0). A)
B)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
8
Determine if each ordered pair is a solution to the given system of inequalities in two variables.
The vertex has coordinates (6, 9), and the focus has coordinates (7, 9). A)
B)
C)
D)
The vertex has coordinates (6, 9), and the focus has coordinates (7, 9). A)
B)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
9
Determine if each ordered pair is a solution to the given system of inequalities in two variables.
The focus has coordinates (-15, 0), and the equation of the directrix is x = 15. A)
B)
C)
D)
The focus has coordinates (-15, 0), and the equation of the directrix is x = 15. A)
B)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
10
Find the vertex, focus, and directrix of the parabola with the given equation.
A) vertex:
focus:
directrix:
B) vertex:
focus:
directrix:
C) vertex:
focus:
directrix:
D) vertex:
focus:
directrix:
A) vertex:
focus:
directrix:
B) vertex:
focus:
directrix:
C) vertex:
focus:
directrix:
D) vertex:
focus:
directrix:

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
11
Graph the equation.
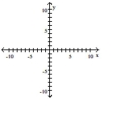
A)
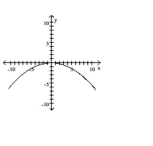
B)
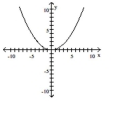
C)

D)
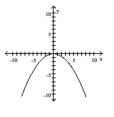

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
12
Find the vertex, focus, and directrix of the parabola. Graph the equation.
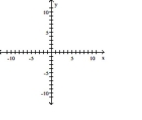
A)
vertex:
focus:
directrix:
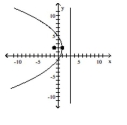
B) vertex:
focus:
directrix:
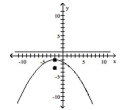
C) vertex:
focus:
directrix:
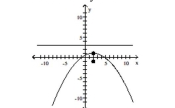
D) vertex:
focus:
directrix:
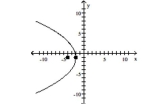

A)
vertex:
focus:
directrix:

B) vertex:
focus:
directrix:

C) vertex:
focus:
directrix:

D) vertex:
focus:
directrix:


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
13
Graph the equation.
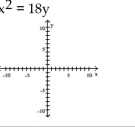 A)
A)
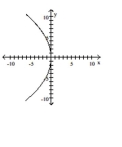
B)
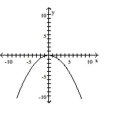
C)
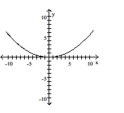
D)
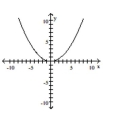
 A)
A)
B)

C)

D)


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
14
Graph the equation.
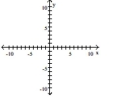
A)

B)
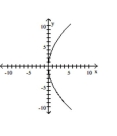
C)
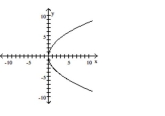
D)
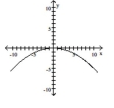

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
15
Graph the equation.
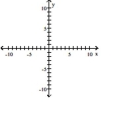
A)

B)

C)

D)
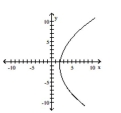

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
16
Graph the equation.
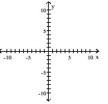
A)

B)
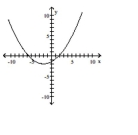
C)
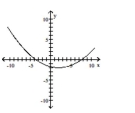
D)
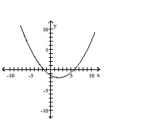

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
17
Determine if each ordered pair is a solution to the given system of inequalities in two variables.
The focus has coordinates (0, 19), and the equation of the directrix is y = -19. A)
B)
C)
D)
The focus has coordinates (0, 19), and the equation of the directrix is y = -19. A)
B)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
18
Find the vertex, focus, and directrix of the parabola. Graph the equation.
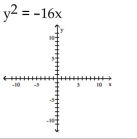 A) vertex:
A) vertex:
focus:
directrix:
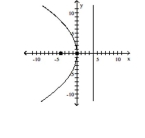
B) vertex:
focus:
directrix:
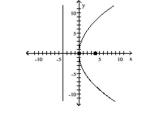
C) vertex:
focus:
directrix:
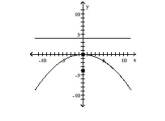
D) vertex:
focus:
directrix:
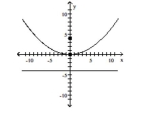
 A) vertex:
A) vertex: focus:
directrix:

B) vertex:
focus:
directrix:

C) vertex:
focus:
directrix:

D) vertex:
focus:
directrix:


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
19
Graph the equation.
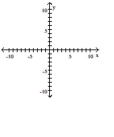
A)
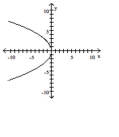
B)
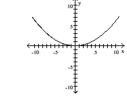
C)
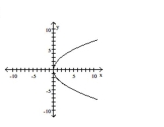
D)


A)

B)

C)

D)


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
20
Find the vertex, focus, and directrix of the parabola. Graph the equation.
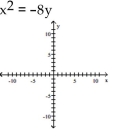 A) vertex:
A) vertex:
focus:
directrix:

B) vertex:
focus:
directrix:


 A) vertex:
A) vertex: focus:
directrix:

B) vertex:
focus:
directrix:



Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
21
Graph the ellipse and locate the foci.
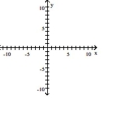
A) foci at and
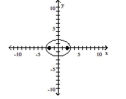
B) foci at and
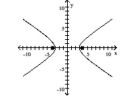
C) foci at and

D) foci at and
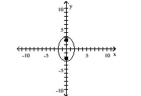

A) foci at and

B) foci at and

C) foci at and

D) foci at and


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
22
Determine if each ordered pair is a solution to the given system of inequalities in two variables.
The vertex has coordinates (5, 6), and the focus has coordinates (5, 3). A)
B)
C)
D)
The vertex has coordinates (5, 6), and the focus has coordinates (5, 3). A)
B)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
23
Find the center, foci, and vertices of the ellipse.
A) center at
foci at and
vertices at
B) center at
foci at and
vertices at
C) center at
foci at and
vertices at
D) center at
foci at and
vertices at
A) center at
foci at and
vertices at
B) center at
foci at and
vertices at
C) center at
foci at and
vertices at
D) center at
foci at and
vertices at

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
24
Determine if each ordered pair is a solution to the given system of inequalities in two variables.
The vertex has coordinates (9, -3), and the focus has coordinates (9, -7). A)
B)
C)
D)
The vertex has coordinates (9, -3), and the focus has coordinates (9, -7). A)
B)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
25
Solve the problem.
A reflecting telescope contains a parabolic mirror. If the mirror is 24 inches across at its opening and is 4 feet deep, where will the light be concentrated?
A)0.3 in. from the vertex
B)0.8 in. from the vertex
C)0.7 in. from the vertex
D)9 in. from the vertex
A reflecting telescope contains a parabolic mirror. If the mirror is 24 inches across at its opening and is 4 feet deep, where will the light be concentrated?
A)0.3 in. from the vertex
B)0.8 in. from the vertex
C)0.7 in. from the vertex
D)9 in. from the vertex

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
26
Find the center, foci, and vertices of the ellipse.
A) center at
foci at
vertices at
B) center at
foci at
vertices at
C) center at
foci at
vertices at
D) center at
foci at
vertices at
A) center at
foci at
vertices at
B) center at
foci at
vertices at
C) center at
foci at
vertices at
D) center at
foci at
vertices at

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
27
Graph the equation.
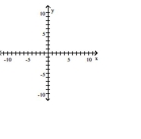
A)
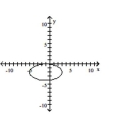
B)
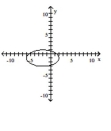
C)
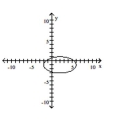
D)

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
28
Solve the problem.
A searchlight is shaped like a parabola. If the light source is located 3 feet from the base along the axis of symmetry and the opening is 8 feet across, how deep should the searchlight be?
A)0.6 ft
B)1.3 ft
C)5.3 ft
D)4 ft
A searchlight is shaped like a parabola. If the light source is located 3 feet from the base along the axis of symmetry and the opening is 8 feet across, how deep should the searchlight be?
A)0.6 ft
B)1.3 ft
C)5.3 ft
D)4 ft

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
29
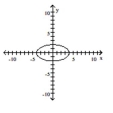
Graph the ellipse and locate the foci.
A) foci at and
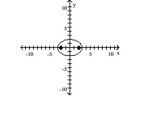
B) foci at and
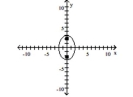
C) foci at and
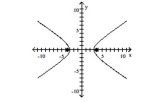
D) foci at and
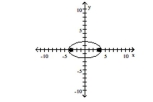

A) foci at and

B) foci at and

C) foci at and

D) foci at and


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
30
Find the center, foci, and vertices of the ellipse.
A) center at
foci at and
vertices at
B) center at
foci at and
vertices at
C) center at
foci at and
vertices at
D) center at
foci at and
vertices at
A) center at
foci at and
vertices at
B) center at
foci at and
vertices at
C) center at
foci at and
vertices at
D) center at
foci at and
vertices at

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
31
Find the center, foci, and vertices of the ellipse.
A) center at
foci at and
vertices at
B) center at
foci at and
vertices at
C) center at
foci at and
vertices at
D) center at
foci at and
vertices at and
A) center at
foci at and
vertices at
B) center at
foci at and
vertices at
C) center at
foci at and
vertices at
D) center at
foci at and
vertices at and

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
32
Find the vertex, focus, and directrix of the parabola. Graph the equation.
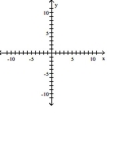
A) vertex:
focus:
directrix:
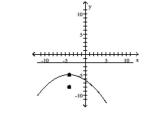
B) vertex:
focus:
directrix:
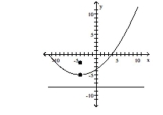
C) vertex:
focus:
directrix:
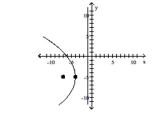
D) vertex:
focus:
directrix:
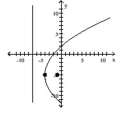

A) vertex:
focus:
directrix:

B) vertex:
focus:
directrix:

C) vertex:
focus:
directrix:

D) vertex:
focus:
directrix:


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
33
Determine if each ordered pair is a solution to the given system of inequalities in two variables.
The vertex has coordinates (3, -5), and the focus has coordinates (4, -5). A)
B)
C)
D)
The vertex has coordinates (3, -5), and the focus has coordinates (4, -5). A)
B)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
34
Graph the ellipse and locate the foci.
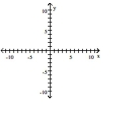
A) foci at and
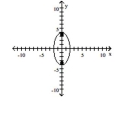
B) foci at and
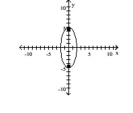
C) foci at and
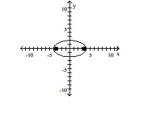
D) foci at and
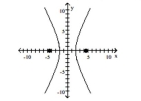

A) foci at and

B) foci at and

C) foci at and

D) foci at and


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
35
Find the center, foci, and vertices of the ellipse.
A) center at
foci at and
vertices at
B) center at
foci at and
vertices at
C) center at
foci at and
vertices at
D) center at
foci at and
vertices at
A) center at
foci at and
vertices at
B) center at
foci at and
vertices at
C) center at
foci at and
vertices at
D) center at
foci at and
vertices at

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
36
Find the vertex, focus, and directrix of the parabola. Graph the equation.
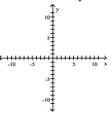
A) vertex:
focus:
directrix:

B) vertex:
focus:
directrix:
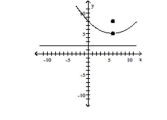


A) vertex:
focus:
directrix:

B) vertex:
focus:
directrix:



Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
37
Find the center, foci, and vertices of the ellipse.
A) center at
foci at
vertices at
B) center at
foci at
vertices at
C) center at
foci at
vertices at
D) center at
foci at
vertices at
A) center at
foci at
vertices at
B) center at
foci at
vertices at
C) center at
foci at
vertices at
D) center at
foci at
vertices at

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
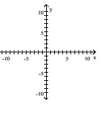
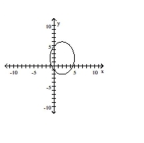
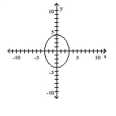
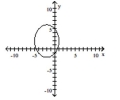
38
Graph the equation.
A)
B)
C)

D)

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
39
Graph the ellipse and locate the foci.

A) foci at and
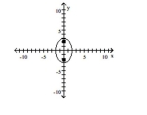
B) foci at and
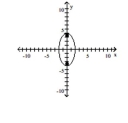
C) foci at and

D) foci at and

A) foci at and

B) foci at and

C) foci at and

D) foci at and


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
40
Solve the problem.
A bridge is built in the shape of a parabolic arch. The bridge arch has a span of 160 feet and a maximum height of 40 feet. Find the height of the arch at 10 feet from its center.
A)2.5 ft
B)39.4 ft
C)0.2 ft
D)5 ft
A bridge is built in the shape of a parabolic arch. The bridge arch has a span of 160 feet and a maximum height of 40 feet. Find the height of the arch at 10 feet from its center.
A)2.5 ft
B)39.4 ft
C)0.2 ft
D)5 ft

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
41
Find an equation for the ellipse described.
Foci at (1, 4)and (-5, 4); vertex at (-8, 4) A)
B)
C)
D)
Foci at (1, 4)and (-5, 4); vertex at (-8, 4) A)
B)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
42
Find an equation for the ellipse described.
Foci at (1, 4)and (7, 4); length of major axis is 10 A)
В)
C)
D)
Foci at (1, 4)and (7, 4); length of major axis is 10 A)
В)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
43
Find an equation for the ellipse described.
Focus at (-2, 0); vertices at (-8, 0)and (8, 0) A)
B)
C)
D)
Focus at (-2, 0); vertices at (-8, 0)and (8, 0) A)
B)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
44
Find the equation in standard form of the parabola described.
Center at (-4, 5); focus at (-6, 5); contains the point (-9, 5) A)
B)
D)
Center at (-4, 5); focus at (-6, 5); contains the point (-9, 5) A)
B)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
45
Find an equation for the ellipse described.
Vertices at (5, -4)and (5, 8); length of minor axis is 6 A)
B)
C)
D)
Vertices at (5, -4)and (5, 8); length of minor axis is 6 A)
B)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
46
Find the equation in standard form of the parabola described.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
47
Find an equation for the ellipse described.
Center at (0, 0); focus at (-2, 0); vertex at (3, 0) A)
B)
C)
D)
Center at (0, 0); focus at (-2, 0); vertex at (3, 0) A)
B)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
48
Find an equation for the ellipse described.
Foci at (-3, 4)and (-3, -2); length of major axis is 10 A)
B)
C)
D)
Foci at (-3, 4)and (-3, -2); length of major axis is 10 A)
B)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
49
Find an equation for the ellipse described.
Foci at (0, -3)and (0, 3); length of the major axis is 12 A)
B)
C)
D)
Foci at (0, -3)and (0, 3); length of the major axis is 12 A)
B)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
50
Find an equation for the ellipse described.
Center at (3, 3); focus at (9, 3); vertex at (11, 3) A)
B)
C)
D)
Center at (3, 3); focus at (9, 3); vertex at (11, 3) A)
B)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
51
Graph the equation.
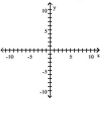
A)
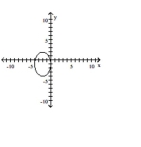
B)
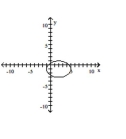
C)
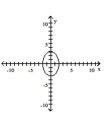
D)
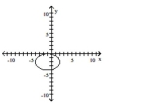

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
52
Write an equation for the graph.
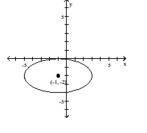
A)
B)
C)
D)

A)
B)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
53
Find an equation for the ellipse described.
Focus at (0, -6); vertices at (0, -7)and (0, 7) A)
B)
C)
D)
Focus at (0, -6); vertices at (0, -7)and (0, 7) A)
B)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
54
Find an equation for the ellipse described.
Center at (0, 0); focus at (0, -5); vertex at (0, 8) A)
B)
C)
D)
Center at (0, 0); focus at (0, -5); vertex at (0, 8) A)
B)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
55
Graph the equation.
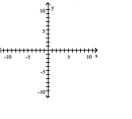
A)
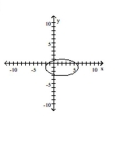
B)
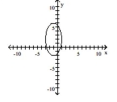
C)
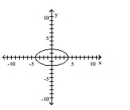
D)
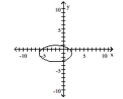

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
56
Find an equation for the ellipse described.
Center (0, 0); major axis horizontal with length 8; length of minor axis is 4 A)
B)
C)
D)
Center (0, 0); major axis horizontal with length 8; length of minor axis is 4 A)
B)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
57
Find an equation for the ellipse described.
Center at (0, 0); focus at (-5, 0); vertex at (8, 0) A)
B)
C)
D)
Center at (0, 0); focus at (-5, 0); vertex at (8, 0) A)
B)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
58
Find an equation for the ellipse described.
Vertices at (-6, 2)and (14, 2); focus at (12, 2) A)
B)
C)
D)
Vertices at (-6, 2)and (14, 2); focus at (12, 2) A)
B)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
59
Find an equation for the ellipse described.
Center at (0, 0); focus at (5, 0); vertex at (8, 0) A)
B)
C)
D)
Center at (0, 0); focus at (5, 0); vertex at (8, 0) A)
B)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
60
Find an equation for the ellipse described.
Center (0, 0); major axis vertical with length 12; length of minor axis is 8 A)
В)
C)
D)
Center (0, 0); major axis vertical with length 12; length of minor axis is 8 A)
В)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
61
Find the center, transverse axis, vertices, foci, and asymptotes of the hyperbola.
A) center at
transverse axis is -axis
vertices:
foci:
asymptotes of and
B) center at
transverse axis is -axis
vertices:
foci:
asymptotes of and
C) center at
transverse axis is -axis
vertices:
foci:
asymptotes of and
D) center at
transverse axis is -axis
vertices:
foci:
asymptotes of and
A) center at
transverse axis is -axis
vertices:
foci:
asymptotes of and
B) center at
transverse axis is -axis
vertices:
foci:
asymptotes of and
C) center at
transverse axis is -axis
vertices:
foci:
asymptotes of and
D) center at
transverse axis is -axis
vertices:
foci:
asymptotes of and

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
62
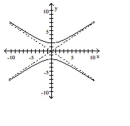
Graph the hyperbola.

A)
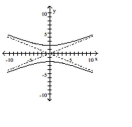
B)
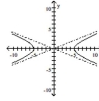
C)
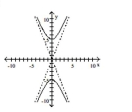
D)

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
63
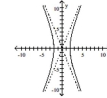
Graph the hyperbola.

A)
B)
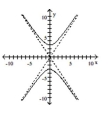
C)
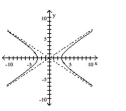
D)
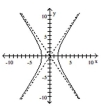

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
64
Graph the hyperbola.

A)
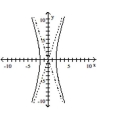
B)

C)
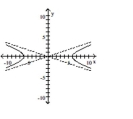
D)
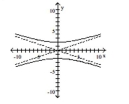

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
65
Find the center, transverse axis, vertices, and foci of the hyperbola.
A) center at
transverse axis is -axis
vertices:
foci:
B) center at
transverse axis is -axis
vertices:
foci:
C) center at
transverse axis is -axis
vertices:
foci:
D) center at
transverse axis is -axis
vertices:
foci:
A) center at
transverse axis is -axis
vertices:
foci:
B) center at
transverse axis is -axis
vertices:
foci:
C) center at
transverse axis is -axis
vertices:
foci:
D) center at
transverse axis is -axis
vertices:
foci:

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
66
Find the equation in standard form of the parabola described.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
67
Find the center, transverse axis, vertices, foci, and asymptotes of the hyperbola.
A) center:
transverse axis is parallel to -axis
vertices: and
foci: and
asymptotes of and
B) center:
transverse axis is parallel to -axis
vertices: and
foci: and
asymptotes of and
C) center:
transverse axis is parallel to -axis
vertices: and
foci: and
asymptotes of and
D) center:
transverse axis is parallel to -axis
vertices: and
foci: and
asymptotes of and
A) center:
transverse axis is parallel to -axis
vertices: and
foci: and
asymptotes of and
B) center:
transverse axis is parallel to -axis
vertices: and
foci: and
asymptotes of and
C) center:
transverse axis is parallel to -axis
vertices: and
foci: and
asymptotes of and
D) center:
transverse axis is parallel to -axis
vertices: and
foci: and
asymptotes of and

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
68
Graph the hyperbola.
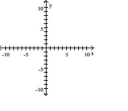
A)
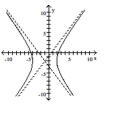
B)
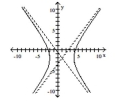
C)
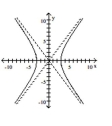
D)


A)

B)

C)

D)


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
69
Find the center, transverse axis, vertices, foci, and asymptotes of the hyperbola.
A) center at
transverse axis is parallel to -axis
vertices at and
foci at and
asymptotes of and
B) center at
transverse axis is parallel to -axis
vertices at and
foci at and
asymptotes of and
C) center at
transverse axis is parallel to -axis
vertices at and
foci at and
asymptotes of and
D) center at
transverse axis is parallel to -axis
vertices at and
foci at and
asymptotes of and
A) center at
transverse axis is parallel to -axis
vertices at and
foci at and
asymptotes of and
B) center at
transverse axis is parallel to -axis
vertices at and
foci at and
asymptotes of and
C) center at
transverse axis is parallel to -axis
vertices at and
foci at and
asymptotes of and
D) center at
transverse axis is parallel to -axis
vertices at and
foci at and
asymptotes of and

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
70
Graph the hyperbola.
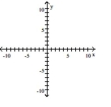
A)
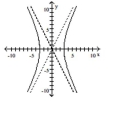
B)
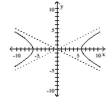
C)
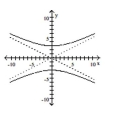
D)
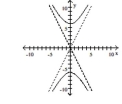

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
71
Graph the hyperbola.
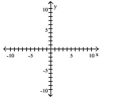
A)
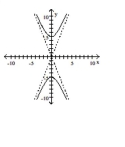
B)

C)

D)
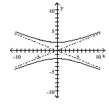

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
72
Find the center, transverse axis, vertices, foci, and asymptotes of the hyperbola.
A) center at
transverse axis is parallel to -axis
vertices at and ,
foci at and ,
asymptotes of and
B) center at
transverse axis is parallel to -axis
vertices at and
foci at and
asymptotes of and
C) center at
transverse axis is parallel to -axis
vertices at and
foci at and
asymptotes of and
D) center at
transverse axis is parallel to -axis
vertices at and
foci at and
asymptotes of and
A) center at
transverse axis is parallel to -axis
vertices at and ,
foci at and ,
asymptotes of and
B) center at
transverse axis is parallel to -axis
vertices at and
foci at and
asymptotes of and
C) center at
transverse axis is parallel to -axis
vertices at and
foci at and
asymptotes of and
D) center at
transverse axis is parallel to -axis
vertices at and
foci at and
asymptotes of and

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
73
Find the center, transverse axis, vertices, foci, and asymptotes of the hyperbola.
A) center:
transverse axis is parallel to -axis
vertices: and ;
foci: and
asymptotes of and
B) center:
transverse axis is parallel to -axis
vertices: and
foci: and
asymptotes of and
C) center:
transverse axis is parallel to -axis
vertices: and
foci: and
asymptotes of and
D) center:
transverse axis is parallel to -axis
vertices: and
foci: and
asymptotes of and
A) center:
transverse axis is parallel to -axis
vertices: and ;
foci: and
asymptotes of and
B) center:
transverse axis is parallel to -axis
vertices: and
foci: and
asymptotes of and
C) center:
transverse axis is parallel to -axis
vertices: and
foci: and
asymptotes of and
D) center:
transverse axis is parallel to -axis
vertices: and
foci: and
asymptotes of and

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
74
Graph the hyperbola.

A)
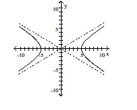
B)
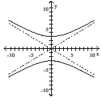
C)
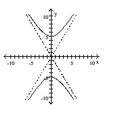
D)
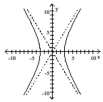

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
75
Find the center, transverse axis, vertices, and foci of the hyperbola.
A) center at
transverse axis is -axis
vertices:
foci:
B) center at
transverse axis is -axis
vertices:
foci:
C) center at
transverse axis is -axis
vertices:
foci:
D) center at
transverse axis is -axis
vertices at and
foci at and
A) center at
transverse axis is -axis
vertices:
foci:
B) center at
transverse axis is -axis
vertices:
foci:
C) center at
transverse axis is -axis
vertices:
foci:
D) center at
transverse axis is -axis
vertices at and
foci at and

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
76
Find the center, transverse axis, vertices, foci, and asymptotes of the hyperbola.
A) center at
transverse axis is -axis
vertices:
foci:
asymptotes of and
B) center at
transverse axis is -axis
vertices:
foci:
asymptotes of and
C) center at
transverse axis is -axis
vertices:
foci:
asymptotes of and
D) center at
transverse axis is -axis
vertices:
foci:
asymptotes of and
A) center at
transverse axis is -axis
vertices:
foci:
asymptotes of and
B) center at
transverse axis is -axis
vertices:
foci:
asymptotes of and
C) center at
transverse axis is -axis
vertices:
foci:
asymptotes of and
D) center at
transverse axis is -axis
vertices:
foci:
asymptotes of and

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
77
Solve the problem.
An arch for a bridge over a highway is in the form of a semiellipse. The top of the arch is 35 feet above ground (the major axis). What should the span of the bridge be (the length of its minor axis)if the height 27 feet from the center is to be 16 feet above ground?
A)60.72 ft
B)30.36 ft
C)50.29 ft
D)118.13 ft
An arch for a bridge over a highway is in the form of a semiellipse. The top of the arch is 35 feet above ground (the major axis). What should the span of the bridge be (the length of its minor axis)if the height 27 feet from the center is to be 16 feet above ground?
A)60.72 ft
B)30.36 ft
C)50.29 ft
D)118.13 ft

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
78
Find the center, transverse axis, vertices, and foci of the hyperbola.
A) center at
transverse axis is -axis
vertices:
oci:
B) center at
transverse axis is -axis
vertices: f
foci:
C) center at
transverse axis is -axis
vertices:
foci:
D) center at
transverse axis is -axis
vertices:
foci:
A) center at
transverse axis is -axis
vertices:
oci:
B) center at
transverse axis is -axis
vertices: f
foci:
C) center at
transverse axis is -axis
vertices:
foci:
D) center at
transverse axis is -axis
vertices:
foci:

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
79
Solve the problem.
A bridge is built in the shape of a semielliptical arch. It has a span of 102 feet. The height of the arch 27 feet from the center is to be 11 feet. Find the height of the arch at its center.
A)11.41 ft
B)20.78 ft
C)12.97 ft
D)27.65 ft
A bridge is built in the shape of a semielliptical arch. It has a span of 102 feet. The height of the arch 27 feet from the center is to be 11 feet. Find the height of the arch at its center.
A)11.41 ft
B)20.78 ft
C)12.97 ft
D)27.65 ft

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck
80
Find the center, transverse axis, vertices, and foci of the hyperbola.
A) center at
transverse axis is -axis
vertices at and
foci at and
B) center at
transverse axis is -axis
vertices at and
foci at and
C) center at
transverse axis is -axis
vertices at and
foci at and
D) center at
transverse axis is -axis
vertices at and
foci at and
A) center at
transverse axis is -axis
vertices at and
foci at and
B) center at
transverse axis is -axis
vertices at and
foci at and
C) center at
transverse axis is -axis
vertices at and
foci at and
D) center at
transverse axis is -axis
vertices at and
foci at and

Unlock Deck
Unlock for access to all 97 flashcards in this deck.
Unlock Deck
k this deck


