Deck 10: Developing Number Operations With Whole Numbers
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/48
Play
Full screen (f)
Deck 10: Developing Number Operations With Whole Numbers
1
Modeling the inverse nature of multiplication and divisions might be done by:
A)putting five beans into each of 6 cups and separating 30 beans into 6 cups.
B)showing that 3 × 5 is 15 and 5 × 3 is 15.
C)showing 273 as 2 hundred, 7 tens, and 3 ones with base-10 blocks.
D)giving 4 children 3 cookies each.
A)putting five beans into each of 6 cups and separating 30 beans into 6 cups.
B)showing that 3 × 5 is 15 and 5 × 3 is 15.
C)showing 273 as 2 hundred, 7 tens, and 3 ones with base-10 blocks.
D)giving 4 children 3 cookies each.
putting five beans into each of 6 cups and separating 30 beans into 6 cups.
2
The problem below is an example of what?
Huang has 3 shirts, 4 pairs of shorts, 2 pairs of socks, and a pair of shoes. How many different outfits can he make with these items of clothing?
A)repeated addition model for multiplication
B)geometric interpretation or array model for multiplication
C)Cartesian cross-product or combinations model for multiplication
D)partitive or sharing model for division
Huang has 3 shirts, 4 pairs of shorts, 2 pairs of socks, and a pair of shoes. How many different outfits can he make with these items of clothing?
A)repeated addition model for multiplication
B)geometric interpretation or array model for multiplication
C)Cartesian cross-product or combinations model for multiplication
D)partitive or sharing model for division
Cartesian cross-product or combinations model for multiplication
3
The problem below is an example of which type of subtraction situation?Tamar found 18 ballpoint pens in a desk at her house. Eleven were out of ink. How many usable pens did she find?
A)takeaway
B)comparison
C)completion
D)whole-part-part
A)takeaway
B)comparison
C)completion
D)whole-part-part
takeaway
4
Subtraction is represented by which four different stories or situations?
A)takeaway, whole-part-part, comparison, and completion
B)commutative, inverse, associative, and identity
C)fluency, algorithms, estimation, and mental computation
D)difference, remainder, subtrahend, and minuend
A)takeaway, whole-part-part, comparison, and completion
B)commutative, inverse, associative, and identity
C)fluency, algorithms, estimation, and mental computation
D)difference, remainder, subtrahend, and minuend

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
5
The most difficult multiplication and division facts for most children to learn are:
A)multiplication by 0, 1, and 2.
B)multiplication by 5 and 10.
C)facts for the square numbers.
D)multiplication facts with 7, 8, and 9 as factors.
A)multiplication by 0, 1, and 2.
B)multiplication by 5 and 10.
C)facts for the square numbers.
D)multiplication facts with 7, 8, and 9 as factors.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
6
Which question is associated with the completion situation of subtraction?
A)"How many are left?"
B)"How many can you see?"
C)"What is the difference?"
D)"How many more are needed?"
A)"How many are left?"
B)"How many can you see?"
C)"What is the difference?"
D)"How many more are needed?"

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
7
The problem below is an example of what?
Hannah had 37 cookies and decided to give each friend 3 cookies. How many friends could she give 3 cookies to?
A)repeated addition model for multiplication.
B)geometric interpretation or array model for multiplication.
C)partitive or sharing model for division.
D) measurement or repeated subtraction model for subtraction.
Hannah had 37 cookies and decided to give each friend 3 cookies. How many friends could she give 3 cookies to?
A)repeated addition model for multiplication.
B)geometric interpretation or array model for multiplication.
C)partitive or sharing model for division.
D) measurement or repeated subtraction model for subtraction.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
8
The problem below is an example of what?
A bag of ready-to-pop popcorn costs 59 cents. How much do 15 bags cost?
A)repeated addition model for multiplication.
B)geometric interpretation or array model for multiplication
C)Cartesian cross-products or combinations model for multiplication
D)measurement or repeated subtraction model for division
A bag of ready-to-pop popcorn costs 59 cents. How much do 15 bags cost?
A)repeated addition model for multiplication.
B)geometric interpretation or array model for multiplication
C)Cartesian cross-products or combinations model for multiplication
D)measurement or repeated subtraction model for division

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
9
The commutative property may be modeled by:
A)moving back and forth on a number line.
B)rotating a domino by 180 degrees.
C)plus one and plus zero facts.
D)showing combinations of 10 using Unifix cubes.
A)moving back and forth on a number line.
B)rotating a domino by 180 degrees.
C)plus one and plus zero facts.
D)showing combinations of 10 using Unifix cubes.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
10
The problem below is an example of what?
Eric has 23 cookies. He has 4 friends coming to his party. How many cookies does each friend get?
A)repeated addition model for multiplication
B)geometric interpretation or array model for multiplication
C)partitive or sharing model for division
D)measurement or repeated subtraction model for subtraction
Eric has 23 cookies. He has 4 friends coming to his party. How many cookies does each friend get?
A)repeated addition model for multiplication
B)geometric interpretation or array model for multiplication
C)partitive or sharing model for division
D)measurement or repeated subtraction model for subtraction

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
11
When learning the meanings for multiplication and division, students:
A)concentrate on learning the facts.
B)model situations with stories, objects, and pictures.
C)are too mature to need manipulatives.
D)must wait until they understand place value.
A)concentrate on learning the facts.
B)model situations with stories, objects, and pictures.
C)are too mature to need manipulatives.
D)must wait until they understand place value.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
12
Skip counting by 3s, 4s and 5s:
A)is not helpful to students learning multiplication.
B)may help children learn the multiplication facts.
C)is best done with a special calculator.
D)relates to the associative property of multiplication.
A)is not helpful to students learning multiplication.
B)may help children learn the multiplication facts.
C)is best done with a special calculator.
D)relates to the associative property of multiplication.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
13
Which is the least effective way for children to learn the 100 multiplication facts?
A)Children discover relationships and make generalizations about fact combinations.
B)Children show how an 8 by 4 array is the same as (4 × 4)+ (4 × 4).
C)Children complete speed practices every day for 30 minutes.
D)Children see the relationship between 9 × 8 = 72 and 8 × 9 = 72.
A)Children discover relationships and make generalizations about fact combinations.
B)Children show how an 8 by 4 array is the same as (4 × 4)+ (4 × 4).
C)Children complete speed practices every day for 30 minutes.
D)Children see the relationship between 9 × 8 = 72 and 8 × 9 = 72.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
14
When children are learning basic addition facts, they can reduce the learning demands by:
A)concentrating on the 20 to 30 hard facts.
B)learning strategies that apply to many facts.
C)memorizing only 2 or 3 facts each day.
D)writing each fact 20 times.
A)concentrating on the 20 to 30 hard facts.
B)learning strategies that apply to many facts.
C)memorizing only 2 or 3 facts each day.
D)writing each fact 20 times.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
15
Addition is represented in stories, action, and drawings as:
A)the union of sets.
B)the decomposition of sets.
C)the elaboration of sets.
D)the common elements of sets.
A)the union of sets.
B)the decomposition of sets.
C)the elaboration of sets.
D)the common elements of sets.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
16
The problem below is an example of what?Penelope's 40-page stamp book has spaces for 35 stamps on each page. How many stamps can she put in the book?
A)repeated addition model for multiplication
B)geometric interpretation or array model for multiplication
C)Cartesian cross-product or combination model for multiplication
D)measurement model for division
A)repeated addition model for multiplication
B)geometric interpretation or array model for multiplication
C)Cartesian cross-product or combination model for multiplication
D)measurement model for division

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
17
For which situation would the remainder 12, remainder 4 be interpreted as 13?
A)How many cars are needed for 64 children when 5 can ride in each car?
B)How many dollars per person when $64 is divided fairly among 5 people?
C)How many miles per gallon when a truck goes 64 miles on 5 gallons?
D)How many marbles per person when 64 marbles are shared fairly by 5 people?
A)How many cars are needed for 64 children when 5 can ride in each car?
B)How many dollars per person when $64 is divided fairly among 5 people?
C)How many miles per gallon when a truck goes 64 miles on 5 gallons?
D)How many marbles per person when 64 marbles are shared fairly by 5 people?

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
18
The problem below is an example of which type of subtraction situation?Shafi has 21 rookie baseball cards, and Bandar has 12 cards. How many more cards does Shafi have than Bandar?
A)takeaway
B)comparison
C)completion
D)whole-part-part
A)takeaway
B)comparison
C)completion
D)whole-part-part

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
19
The equations 7 + 2 = 9 and 9 - 2 = 7 demonstrate:
A)commutative property.
B)associative property.
C)identity element for addition and subtraction.
D)inverse operations.
A)commutative property.
B)associative property.
C)identity element for addition and subtraction.
D)inverse operations.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
20
Assessment shows that many primary children interpret the equal sign as:
A)meaning "3 + 4 write the answer now 7."
B)meaning "3 + 4 is the same as 7."
C)meaning "3 + 4 is the opposite of 4 + 3."
D)meaningless.
A)meaning "3 + 4 write the answer now 7."
B)meaning "3 + 4 is the same as 7."
C)meaning "3 + 4 is the opposite of 4 + 3."
D)meaningless.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
21
What is the role of drill and practice in learning basic number facts? What are cautions you would take with drill and practice?

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
22
Which number makes the following number sentence true? 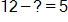
A)4
B)7
C)9
D)17
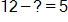
A)4
B)7
C)9
D)17

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
23
Match the number operations with examples related to that operation.
Santino can run to the fence in 55 seconds. Estela can run the distance in 52 seconds. Who is faster?
A)addition
B)subtraction
C)multiplication
D)division
Santino can run to the fence in 55 seconds. Estela can run the distance in 52 seconds. Who is faster?
A)addition
B)subtraction
C)multiplication
D)division

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
24
Mr. Horowitz wants to buy 48 cans of dog food to give to the animal shelter. There are 6 cans of dog food in a package. How many packages of dog food should Mr. Horowitz buy?
A)7 packages
B)8 packages
C)42 packages
D)54 packages
A)7 packages
B)8 packages
C)42 packages
D)54 packages

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
25
Match the number operations with examples related to that operation.
John has 3 pets and Sally has 4 pets. Who has more pets?
A)addition
B)subtraction
C)multiplication
D)division
John has 3 pets and Sally has 4 pets. Who has more pets?
A)addition
B)subtraction
C)multiplication
D)division

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
26
In primary grades, the emphasis is on the first three phases of number operations, which are:
A)concepts, memorization of number facts, and practice.
B)learning strategies, practice, and maintenance.
C)integration of number with language arts, social studies, and science.
D)concepts, strategies for number combinations, and accuracy with speed.
A)concepts, memorization of number facts, and practice.
B)learning strategies, practice, and maintenance.
C)integration of number with language arts, social studies, and science.
D)concepts, strategies for number combinations, and accuracy with speed.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
27
Kayla has 15 strawberries. She gives 4 strawberries to each of her 3 friends. How many strawberries are left for Kayla?
A)1
B)2
C)3
D)4
A)1
B)2
C)3
D)4

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
28
Use of compatible numbers makes computation easier because children:
A)work with numbers less than 100.
B)look for numbers with sums such as 10, 100, or 1,000.
C)the sum of compatible numbers is always even.
D)the product of compatible numbers ends in 5 or 0.
A)work with numbers less than 100.
B)look for numbers with sums such as 10, 100, or 1,000.
C)the sum of compatible numbers is always even.
D)the product of compatible numbers ends in 5 or 0.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
29
Emma wants to give 8 stickers to 4 friends. She wrote this number sentence to find out how many stickers she needs.
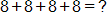
Write a multiplication number sentence that Emma could use to find out how many stickers she needs. Be sure to show your work.
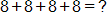
Write a multiplication number sentence that Emma could use to find out how many stickers she needs. Be sure to show your work.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
30
Match the number operations with examples related to that operation.
Huang has 3 pants and 4 shirts. How many days can he wear a different outfit before repeating?
A)addition
B)subtraction
C)multiplication
D)division
Huang has 3 pants and 4 shirts. How many days can he wear a different outfit before repeating?
A)addition
B)subtraction
C)multiplication
D)division

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
31
Sample Test Items
What is the greatest number of 40-cent apples that can be purchased with $5.00?
A)6
B)12
C)15
D)20
What is the greatest number of 40-cent apples that can be purchased with $5.00?
A)6
B)12
C)15
D)20

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
32
How should 3 children share 12 cookies equally?
A)3 portions of 4 cookies each
B)4 portions of 3 cookies each
C)1 portion of 5 cookies, 1 portion of 4 cookies, and 1 portion of 3 cookies
D)2 portions of 6 cookies each
A)3 portions of 4 cookies each
B)4 portions of 3 cookies each
C)1 portion of 5 cookies, 1 portion of 4 cookies, and 1 portion of 3 cookies
D)2 portions of 6 cookies each

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
33
Drew entered four numbers less than 10 on his calculator. He forgot what his second and fourth numbers were. This is what he remembered doing.
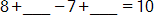
List a pair of numbers that could have been the second and fourth numbers. (You may use number tiles to help you.)
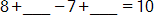
List a pair of numbers that could have been the second and fourth numbers. (You may use number tiles to help you.)

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
34
Match the number operations with examples related to that operation.
A bag of ready-to-pop popcorn costs 59 cents. How much do 15 bags cost?
A)addition
B)subtraction
C)multiplication
D)division
A bag of ready-to-pop popcorn costs 59 cents. How much do 15 bags cost?
A)addition
B)subtraction
C)multiplication
D)division

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
35
Match the number operations with examples related to that operation.
Counting by 2s, 3s, 4s, or 7s
A)addition
B)subtraction
C)multiplication
D)division
Counting by 2s, 3s, 4s, or 7s
A)addition
B)subtraction
C)multiplication
D)division

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
36
What is computational fluency? What must children know and be able to do to achieve computational fluency?

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
37
Describe two activities you could use to build children's understanding of addition or subtraction.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
38
Match the number operations with examples related to that operation.
John has 3 pets and Sally has 4 pets. How many pets do they have?
A)addition
B)subtraction
C)multiplication
D)division
John has 3 pets and Sally has 4 pets. How many pets do they have?
A)addition
B)subtraction
C)multiplication
D)division

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
39
Match the number operations with examples related to that operation.
The bag of cookies has 28 cookies. How many children can be served if they each get 3?
A)addition
B)subtraction
C)multiplication
D)division
The bag of cookies has 28 cookies. How many children can be served if they each get 3?
A)addition
B)subtraction
C)multiplication
D)division

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
40
Match the number operations with examples related to that operation.
Eight children get 3 cookies each.
A)addition
B)subtraction
C)multiplication
D)division
Eight children get 3 cookies each.
A)addition
B)subtraction
C)multiplication
D)division

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
41
Explain why division by zero is undefined.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
42
Define and give examples for the two types of division.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
43
What roles do the commutative and associative properties play in learning the basic facts?

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
44
What is the value of teaching strategies rather than memorization for learning basic facts?

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
45
John multiplied 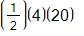 and obtained the product (2)(10). Explain his error in terms of properties or in terms of the concept of multiplication being a binary operation.
and obtained the product (2)(10). Explain his error in terms of properties or in terms of the concept of multiplication being a binary operation.
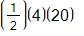 and obtained the product (2)(10). Explain his error in terms of properties or in terms of the concept of multiplication being a binary operation.
and obtained the product (2)(10). Explain his error in terms of properties or in terms of the concept of multiplication being a binary operation.
Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
46
Define and give examples for the three types of multiplication.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
47
Sketch all possible rectangular areas for rectangles of area 6 and 5 square units, respectively. Explain how the areas relate to prime and composite numbers.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
48
The owner of the bookstore gave the school 17 books. The principal is going to divide the books equally among 5 classes and give the rest to the school library. Draw a picture that shows how many books each class will get and how many the library will receive. Show your work, then explain in words how you got your answer.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck


