Deck 12: Vector Functions and Curves
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/87
Play
Full screen (f)
Deck 12: Vector Functions and Curves
1
Let r(t) = 4t i + 3sin(t) j - 3cos(t) k be a differentiable vector function giving the position r of a particle at time t. Find the speed of the particle at time t =  seconds.
seconds.
A) 5.5 units/s
B) 5.0 units/s
C) 4.5 units/s
D) 4.0 units/s
E) 3.5 units/s
 seconds.
seconds.A) 5.5 units/s
B) 5.0 units/s
C) 4.5 units/s
D) 4.0 units/s
E) 3.5 units/s
5.0 units/s
2
Find the velocity at time t = -2 of a particle whose position at time t is given by r(t) = 3t i - 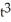 j.
j.
A) v(-2) = - 3i + 12j
B) v(-2) =- 3i - 12j
C) v(-2) =3i + 12j
D) v(-2) = 3i - 12j
E) v(-2) = 3i - 4j
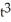 j.
j.A) v(-2) = - 3i + 12j
B) v(-2) =- 3i - 12j
C) v(-2) =3i + 12j
D) v(-2) = 3i - 12j
E) v(-2) = 3i - 4j
v(-2) = 3i - 12j
3
Find the acceleration at time t = -1 of a particle whose position at time t is given by r(t) = 4t i + 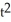 j.
j.
A) a(-1) = -2j
B) a(-1) = 2j
C) a(-1) = i - 2j
D) a(-1) = i + 2j
E) a(-1) = 2i + j
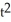 j.
j.A) a(-1) = -2j
B) a(-1) = 2j
C) a(-1) = i - 2j
D) a(-1) = i + 2j
E) a(-1) = 2i + j
a(-1) = 2j
4
A moving particle starts at an initial position (1, 0, 0) with initial velocity i - j + k. Its acceleration at time t is a(t) = 4t i + 6t j + k. Find its velocity and position at time t > 0.
A)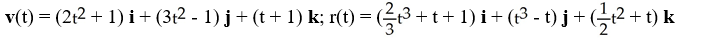
B)
C)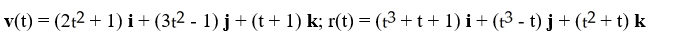
D)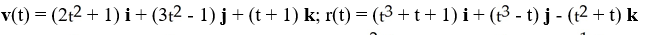
E)
A)
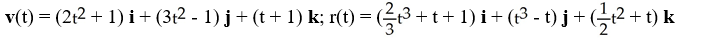
B)

C)
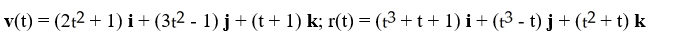
D)
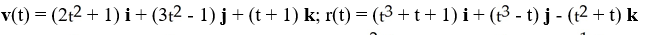
E)


Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
5
Find the velocity, speed, and acceleration at time t of a particle that has position function r(t) = (2sin t) i + 6t j + (2cos t) k.
A)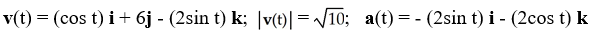
B)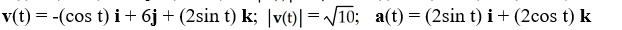
C)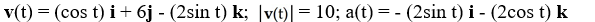
D)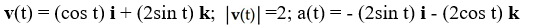
E)
A)
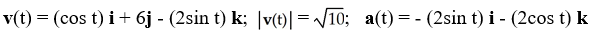
B)
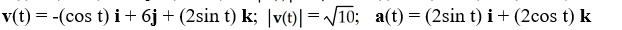
C)
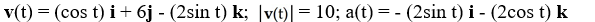
D)
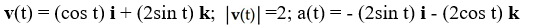
E)


Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
6
Find the velocity, speed, and acceleration at time t of a particle that has position function r(t) = 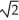 t i +
t i + 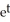 j +
j + 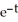 k.
k.
A)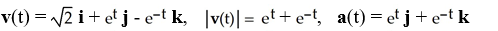
B)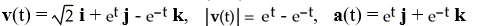
C)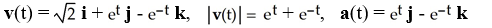
D)
E)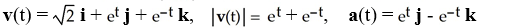
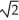 t i +
t i + 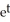 j +
j + 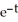 k.
k.A)
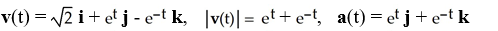
B)
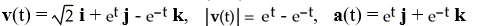
C)
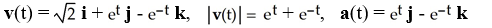
D)

E)
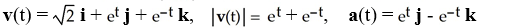

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
7
What kind of curve is r(t) = 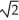 (sin t) i +
(sin t) i + 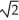 (sin t) j + 2(cos t) k?
(sin t) j + 2(cos t) k?
A) a circular helix
B) an oval plane curve that is, however, not an ellipse
C) an ellipse (but not a circle)
D) a circle
E) a parabola
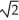 (sin t) i +
(sin t) i + 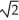 (sin t) j + 2(cos t) k?
(sin t) j + 2(cos t) k?A) a circular helix
B) an oval plane curve that is, however, not an ellipse
C) an ellipse (but not a circle)
D) a circle
E) a parabola

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
8
Describe the curve r(t) = (sin t) i + (cos t) j + 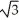 k.
k.
A) a helix wound around the cylinder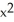 +
+ 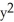 = 1
= 1
B) the circle (of radius 1) in which the plane z =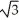 intersects the sphere
intersects the sphere  +
+ 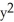 +
+ 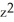 = 4
= 4
C) the circle (of radius ) in which the plane z = 1 intersects the sphere
) in which the plane z = 1 intersects the sphere  +
+ 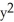 +
+ 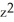 = 3
= 3
D) the circle (of radius ) in which the plane z =
) in which the plane z = 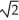 intersects the cylinder
intersects the cylinder  +
+ 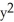 = 1
= 1
E) a helix wound around the cylinder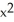 +
+ 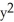 = 3
= 3
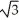 k.
k.A) a helix wound around the cylinder
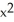 +
+ 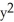 = 1
= 1B) the circle (of radius 1) in which the plane z =
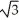 intersects the sphere
intersects the sphere  +
+ 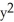 +
+ 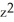 = 4
= 4C) the circle (of radius
 ) in which the plane z = 1 intersects the sphere
) in which the plane z = 1 intersects the sphere  +
+ 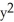 +
+ 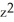 = 3
= 3D) the circle (of radius
 ) in which the plane z =
) in which the plane z = 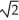 intersects the cylinder
intersects the cylinder  +
+ 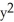 = 1
= 1E) a helix wound around the cylinder
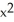 +
+ 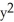 = 3
= 3
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
9
Find an equation of the line tangent to the parametric space curve
r(t) = (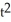 + 3t + 1) i + (2 - 7t j + (4sin(t) -3)k at the point on the curve corresponding to t = 0.
+ 3t + 1) i + (2 - 7t j + (4sin(t) -3)k at the point on the curve corresponding to t = 0.
A) r(u) = (1 + 3u) i + (2 - 7u) j + (- 3 + 4s) k, u R
R
B) 3x - 7y + 4z -23 = 0
C) x + y + z = 0
D) r(u) = (3 + u) i + (-7 + 2u) j + (4 - 3u) k, u 11ee7b17_3372_5854_ae82_d19d2ea0c252_TB9661_11 R
E) x + 2y - 3z - 14 = 0
r(t) = (
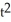 + 3t + 1) i + (2 - 7t j + (4sin(t) -3)k at the point on the curve corresponding to t = 0.
+ 3t + 1) i + (2 - 7t j + (4sin(t) -3)k at the point on the curve corresponding to t = 0.A) r(u) = (1 + 3u) i + (2 - 7u) j + (- 3 + 4s) k, u
 R
RB) 3x - 7y + 4z -23 = 0
C) x + y + z = 0
D) r(u) = (3 + u) i + (-7 + 2u) j + (4 - 3u) k, u 11ee7b17_3372_5854_ae82_d19d2ea0c252_TB9661_11 R
E) x + 2y - 3z - 14 = 0

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
10
A particle is moving to the right with constant speed 2 along the curve y = cos x in the xy-plane. Find its velocity at the instant when it crosses the vertical line x = 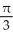 .
.
A) v = i -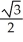 j
j
B) v =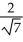 i -
i - 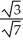 j
j
C) v = i -
i - 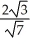 j
j
D) v = i -
i - 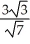 j
j
E) v = i +
i + 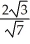 j
j
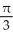 .
.A) v = i -
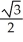 j
jB) v =
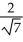 i -
i - 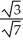 j
jC) v =
 i -
i - 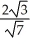 j
jD) v =
 i -
i - 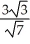 j
jE) v =
 i +
i + 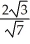 j
j
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
11
The position vector of a moving particle in space is given by the vector equation 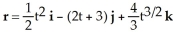 . When will the speed of the particle be 4 units?
. When will the speed of the particle be 4 units?
A) t = 6 and t = -2
B) t = 0
C) t = -6 and t = 2
D) t = 6
E) t = 2
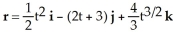 . When will the speed of the particle be 4 units?
. When will the speed of the particle be 4 units?A) t = 6 and t = -2
B) t = 0
C) t = -6 and t = 2
D) t = 6
E) t = 2

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
12
An object is moving to the right in the xy-plane along the curve y = 
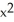 . At the instant when it is at the point
. At the instant when it is at the point 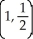 , its speed is 2 and that speed is decreasing at rate 1. What are the velocity and acceleration of the object at that instant?
, its speed is 2 and that speed is decreasing at rate 1. What are the velocity and acceleration of the object at that instant?
A) v =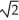 (i + j), a = (1 +
(i + j), a = (1 + 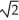 ) i + (1 -
) i + (1 - 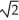 ) j
) j
B) v =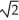 (i + j), a = i - j
(i + j), a = i - j
C) v = 2 (i + j), a = (1 +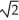 ) i + (1 -
) i + (1 - 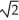 ) j
) j
D) v = 2 (i + j), a = -i + j
E) v =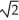 (i + j), a = -i + j
(i + j), a = -i + j

 . At the instant when it is at the point
. At the instant when it is at the point 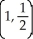 , its speed is 2 and that speed is decreasing at rate 1. What are the velocity and acceleration of the object at that instant?
, its speed is 2 and that speed is decreasing at rate 1. What are the velocity and acceleration of the object at that instant?A) v =
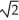 (i + j), a = (1 +
(i + j), a = (1 + 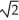 ) i + (1 -
) i + (1 - 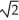 ) j
) jB) v =
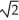 (i + j), a = i - j
(i + j), a = i - jC) v = 2 (i + j), a = (1 +
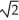 ) i + (1 -
) i + (1 - 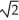 ) j
) jD) v = 2 (i + j), a = -i + j
E) v =
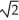 (i + j), a = -i + j
(i + j), a = -i + j
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
13
At a certain instant a moving object has velocity v = 2i - 3j - k (in cm/s) and acceleration 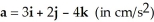 . How fast is its speed changing at that instant?
. How fast is its speed changing at that instant?
A) speed is decreasing at /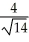 cm
cm 
B) speed is increasing at /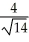 cm
cm 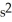
C) speed is decreasing at / cm
cm 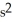
D) speed is increasing at /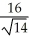 cm
cm 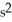
E) speed is unchanging
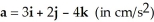 . How fast is its speed changing at that instant?
. How fast is its speed changing at that instant?A) speed is decreasing at /
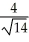 cm
cm 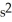
B) speed is increasing at /
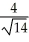 cm
cm 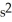
C) speed is decreasing at /
 cm
cm 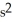
D) speed is increasing at /
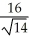 cm
cm 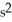
E) speed is unchanging

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
14
Suppose that the position r(t) and velocity v(t) of a moving object satisfy r(t) . v(t) = 0 for all t. What does this imply about the curve r(t)?
A) The curve lies on a sphere centred at the origin.
B) The curve lies in a plane.
C) The curve is a circle.
D) The curve is a helix.
E) The curve lies on a hyperboloid.
A) The curve lies on a sphere centred at the origin.
B) The curve lies in a plane.
C) The curve is a circle.
D) The curve is a helix.
E) The curve lies on a hyperboloid.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
15
The position function of a particle is given by r(t) = ,  When is the speed a minimum?
When is the speed a minimum?
A) t = 2
B) t = 3
C) t = 4
D) t = 5
E) t = 1
 When is the speed a minimum?
When is the speed a minimum?A) t = 2
B) t = 3
C) t = 4
D) t = 5
E) t = 1

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
16
The position of a particle at time t is given by r = (cos t) i + (sin t) j + t k. Find the speed and the magnitude of the acceleration at any time t. Describe the motion.
A)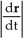 =
= 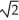 ;
; 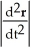 =
= 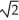 ; path is a circular helix
; path is a circular helix
B)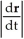 =
= 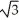 ;
; 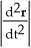 =
=  ; path is a circle
; path is a circle
C)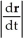 =
= 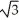 ;
; 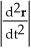 = 2; path is a circular helix
= 2; path is a circular helix
D)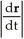 =
= 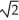 ;
; 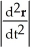 = 1; path is a circular helix
= 1; path is a circular helix
E)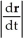 =
= 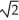 ;
; 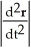 = 1; path is a circle
= 1; path is a circle
A)
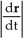 =
= 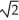 ;
; 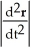 =
= 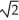 ; path is a circular helix
; path is a circular helixB)
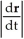 =
= 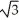 ;
; 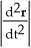 =
=  ; path is a circle
; path is a circleC)
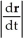 =
= 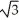 ;
; 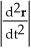 = 2; path is a circular helix
= 2; path is a circular helixD)
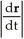 =
= 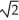 ;
; 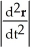 = 1; path is a circular helix
= 1; path is a circular helixE)
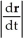 =
= 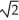 ;
; 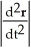 = 1; path is a circle
= 1; path is a circle
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
17
Solve the initial-value problem 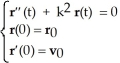
A) r(t) =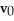 cos(kt) -
cos(kt) - 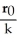 sin(kt)
sin(kt)
B) r(t) =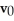 cos(kt) +
cos(kt) + 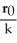 sin(kt)
sin(kt)
C) r(t) =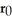 cos(kt) +
cos(kt) + 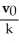 sin(kt)
sin(kt)
D) r(t) =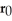 cos(kt) -
cos(kt) - 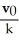 sin(kt)
sin(kt)
E) r(t) =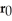 sin(kt) +
sin(kt) + 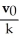 cos(kt)
cos(kt)
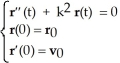
A) r(t) =
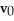 cos(kt) -
cos(kt) - 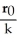 sin(kt)
sin(kt)B) r(t) =
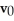 cos(kt) +
cos(kt) + 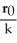 sin(kt)
sin(kt)C) r(t) =
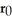 cos(kt) +
cos(kt) + 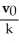 sin(kt)
sin(kt)D) r(t) =
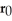 cos(kt) -
cos(kt) - 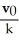 sin(kt)
sin(kt)E) r(t) =
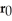 sin(kt) +
sin(kt) + 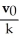 cos(kt)
cos(kt)
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
18
Suppose the position vector r(t) of an object moving in 3-space and the corresponding velocity and acceleration vectors v(t) and a(t) satisfy
(i) a(t) is parallel to r(t) for all t, and
(ii) r(0) × v(0) = c (a nonzero constant vector).
Find r(t) × v(t) and c . r(t) for all t.
(i) a(t) is parallel to r(t) for all t, and
(ii) r(0) × v(0) = c (a nonzero constant vector).
Find r(t) × v(t) and c . r(t) for all t.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
19
Assuming u(t) has continuous derivatives of all required orders, simplify the expression . 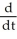

A)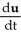 .
.  + u(t) .
+ u(t) . 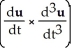
B) .
. 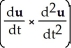
C) u(t) .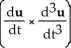
D) 0
E)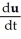 .
. 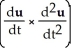 + u(t) .
+ u(t) . 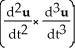

A)
 .
.  + u(t) .
+ u(t) . 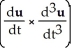
B)
 .
. 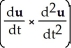
C) u(t) .
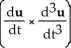
D) 0
E)
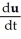 .
. 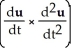 + u(t) .
+ u(t) . 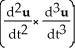

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
20
Assuming u(t) has continuous derivatives of all required orders, simplify the expression . 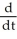

A) ×
× 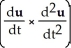 + u(t) ×
+ u(t) × 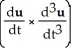
B)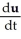 ×
× 
C) u(t) ×
D) 0
E)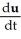 ×
× 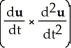 + u(t) ×
+ u(t) × 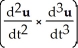
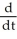

A)
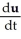 ×
× 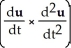 + u(t) ×
+ u(t) × 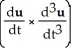
B)
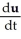 ×
× 
C) u(t) ×

D) 0
E)
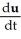 ×
× 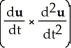 + u(t) ×
+ u(t) × 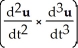

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
21
If u and v are differentiable functions of t, then 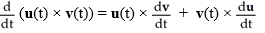
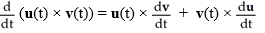

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
22
If r(t) , v(t) and a(t) are the position, velocity, and acceleration of a moving particle at any time t, respectively, and if the speed at any time t is a constant , then the velocity and acceleration vectors are perpendicular.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
23
A particle moves around the elliptical cylinder 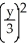 +
+ 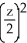 = 1 in such a way that its position at time t is
= 1 in such a way that its position at time t is 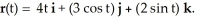 Find the maximum and minimum values of both its speed
Find the maximum and minimum values of both its speed  and its magnitude of its acceleration
and its magnitude of its acceleration 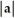 .
.
A) max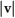 = 3, min
= 3, min 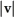 = 2; max
= 2; max 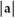 = 3, min
= 3, min 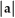 = 2
= 2
B) max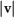 = 5, min
= 5, min  = 2
= 2 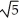 ; max
; max 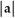 = 3, min
= 3, min 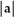 = 2
= 2
C) max = 5, min
= 5, min 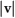 = 2
= 2 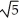 ; max
; max 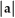 = 5, min
= 5, min 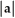 = 2
= 2 
D) max = 3, min
= 3, min 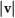 = 2; max
= 2; max 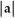 = 3, min
= 3, min 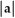 = 0
= 0
E) max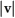 = 5, min
= 5, min 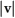 = 2
= 2 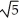 ; max
; max  = 3, min
= 3, min  = 0
= 0
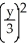 +
+ 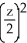 = 1 in such a way that its position at time t is
= 1 in such a way that its position at time t is 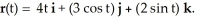 Find the maximum and minimum values of both its speed
Find the maximum and minimum values of both its speed 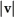 and its magnitude of its acceleration
and its magnitude of its acceleration 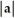 .
.A) max
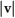 = 3, min
= 3, min 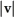 = 2; max
= 2; max 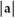 = 3, min
= 3, min 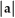 = 2
= 2B) max
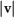 = 5, min
= 5, min  = 2
= 2 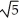 ; max
; max 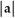 = 3, min
= 3, min 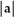 = 2
= 2C) max
 = 5, min
= 5, min 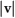 = 2
= 2 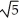 ; max
; max 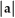 = 5, min
= 5, min 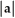 = 2
= 2 
D) max
 = 3, min
= 3, min 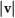 = 2; max
= 2; max 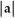 = 3, min
= 3, min 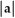 = 0
= 0E) max
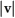 = 5, min
= 5, min 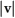 = 2
= 2 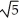 ; max
; max  = 3, min
= 3, min  = 0
= 0
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
24
A ball of ice having mass 100 g at time t = 0 s is melting, and therefore losing mass, at a steady rate of 1 g/s. The ball has velocity i + 2 j at time t = 0 and is subject to a constant force F = 3 i thereafter. What is its velocity after 1 minute?
A) 10 i + 5 j
B) 10 i + 10 j
C) 4 i + 5 j
D) 4 i + 8 j
E) 10 i + 8 j
A) 10 i + 5 j
B) 10 i + 10 j
C) 4 i + 5 j
D) 4 i + 8 j
E) 10 i + 8 j

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
25
Solve the initial-value problem 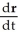 = k × r with initial condition r(0) = i + 2j + 3k. Describe the solution curve.
= k × r with initial condition r(0) = i + 2j + 3k. Describe the solution curve.
A) r(t) =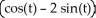 i +
i + 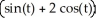 j + 3 k,a circle with centre (0, 0, 3) and radius 5 in the plane z = 3.
j + 3 k,a circle with centre (0, 0, 3) and radius 5 in the plane z = 3.
B) r(t) = i -
i - 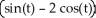 j + 3 k,a circle with centre (0, 0, 3) and radius
j + 3 k,a circle with centre (0, 0, 3) and radius 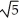 in the plane z = 3.
in the plane z = 3.
C) r(t) = i -
i -  j + 3 k,a circle with centre (0, 0, 3) and radius 5 in the plane z = 3.
j + 3 k,a circle with centre (0, 0, 3) and radius 5 in the plane z = 3.
D) r(t) = i +
i + 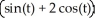 j + 3 k,a circle with centre (0, 0, 3) and radius
j + 3 k,a circle with centre (0, 0, 3) and radius 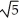 in the plane z = 3.
in the plane z = 3.
E) r(t) = i +
i + 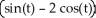 j + 3 k,a circle with centre (0, 0, 3) and radius 5 in the plane z = 3.
j + 3 k,a circle with centre (0, 0, 3) and radius 5 in the plane z = 3.
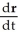 = k × r with initial condition r(0) = i + 2j + 3k. Describe the solution curve.
= k × r with initial condition r(0) = i + 2j + 3k. Describe the solution curve.A) r(t) =
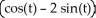 i +
i + 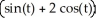 j + 3 k,a circle with centre (0, 0, 3) and radius 5 in the plane z = 3.
j + 3 k,a circle with centre (0, 0, 3) and radius 5 in the plane z = 3.B) r(t) =
 i -
i - 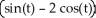 j + 3 k,a circle with centre (0, 0, 3) and radius
j + 3 k,a circle with centre (0, 0, 3) and radius 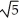 in the plane z = 3.
in the plane z = 3.C) r(t) =
 i -
i -  j + 3 k,a circle with centre (0, 0, 3) and radius 5 in the plane z = 3.
j + 3 k,a circle with centre (0, 0, 3) and radius 5 in the plane z = 3.D) r(t) =
 i +
i + 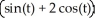 j + 3 k,a circle with centre (0, 0, 3) and radius
j + 3 k,a circle with centre (0, 0, 3) and radius 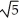 in the plane z = 3.
in the plane z = 3.E) r(t) =
 i +
i + 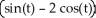 j + 3 k,a circle with centre (0, 0, 3) and radius 5 in the plane z = 3.
j + 3 k,a circle with centre (0, 0, 3) and radius 5 in the plane z = 3.
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
26
You are at the origin in the xy-plane. At time t = 0 an incoming missile is at position (1000, 500) and has velocity -30 i + 3 j. (All distances are in metres and time is measured in seconds.) At that instant you fire an anti-missile missile to intercept the incoming missile. If your missile has an initial speed of 100 m/s, at what angle of elevation above the horizontal should you fire your missile to ensure that it intercepts the incoming missile? Assume that gravity is the only force acting on the projectiles.
A) 0.42535 radians
B) 0.52535 radians
C) 0.62535 radians
D) 0.72535 radians
E) 0.56535 radians
A) 0.42535 radians
B) 0.52535 radians
C) 0.62535 radians
D) 0.72535 radians
E) 0.56535 radians

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
27
A rocket is fired vertically in a vacuum (free space where the gravitational field is negligible).During the burning process, the exhaust gases are ejected at a constant rate of 1000 kg/s and at a constant velocity with magnitude 400 m/s relative to the rocket.Let M be the total initial mass of the rocket, and assume the rocket starts motion from rest.In order to accelerate to the speed of 800 m/s, the rocket has to burn P % of the total initial mass M as a fuel. Assuming there is sufficient amount of fuel on board, the value of P is equal to:
A) 1 -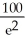
B) 100
C) 100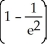
D)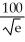
E) 100 -
A) 1 -
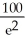
B) 100

C) 100
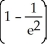
D)
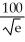
E) 100 -


Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
28
A rocket with mass 60,000 kilogram (kg), which includes 30,000 kg of fuel mixture, is fired vertically in a vacuum (free space where the gravitational field is negligible).During the burning process, the exhaust gases are ejected at a constant rate of 1000 kg/s and at a constant velocity with magnitude 400 m/s relative to the rocket.If the rocket was initially at rest, find its speed after 50 seconds.
A) 400 ln(3) m/s
B) 400 ln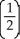 m/s
m/s
C) 400 m/s
D) 400 ln (2) m/s
E) 400 ln (6) m/s
A) 400 ln(3) m/s
B) 400 ln
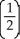 m/s
m/sC) 400 m/s
D) 400 ln (2) m/s
E) 400 ln (6) m/s

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
29
Which of the following parametrize the circle  +
+ 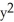 - 4y = 0 with clockwise orientation?
- 4y = 0 with clockwise orientation?
(a) r = (2 cos ) i + (2 + 2 sin ) j
(b) r = (2 cos ) i - (2 + 2 sin ) j
(c) r = (2 cos ) i + (2 - 2 sin ) j
(d) r = (2 sin ) i + (2 + 2 cos ) j
A) only (a)
B) only (b)
C) only (c)
D) only (d)
E) both (a) and (b)
F) both (c) and (d)
G) none of the above
 +
+ 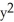 - 4y = 0 with clockwise orientation?
- 4y = 0 with clockwise orientation?(a) r = (2 cos ) i + (2 + 2 sin ) j
(b) r = (2 cos ) i - (2 + 2 sin ) j
(c) r = (2 cos ) i + (2 - 2 sin ) j
(d) r = (2 sin ) i + (2 + 2 cos ) j
A) only (a)
B) only (b)
C) only (c)
D) only (d)
E) both (a) and (b)
F) both (c) and (d)
G) none of the above

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
30
Find a parametrization of the ellipse in which the plane z = 3y intersects the cylinder ![<strong>Find a parametrization of the ellipse in which the plane z = 3y intersects the cylinder + = 4, using the polar angle \theta in the xy-plane as the parameter, [0, 2 \pi ] as parameter interval, and ensuring that the ellipse is oriented counterclockwise as viewed from high on the z-axis.</strong> A) r = 2 cos( \theta ) i + 2 sin( \theta ) j + 6 sin( \theta ) k B) r = 2 cos( \theta ) i - 2 sin( \theta ) j + 6 sin( \theta ) k C) r = 2 cos( \theta ) i + 2 sin( \theta ) j - 6 sin( \theta ) k D) r = 2 cos( \theta ) i - 2 sin( \theta ) j - 6 sin( \theta ) k E) r = 2 cos( \theta ) i + 2 sin( \theta ) j + 3 sin( \theta ) k](https://storage.examlex.com/TB9661/11ee77e1_77bc_4cc2_a0f8_47790a5bc80a_TB9661_11.jpg) +
+ ![<strong>Find a parametrization of the ellipse in which the plane z = 3y intersects the cylinder + = 4, using the polar angle \theta in the xy-plane as the parameter, [0, 2 \pi ] as parameter interval, and ensuring that the ellipse is oriented counterclockwise as viewed from high on the z-axis.</strong> A) r = 2 cos( \theta ) i + 2 sin( \theta ) j + 6 sin( \theta ) k B) r = 2 cos( \theta ) i - 2 sin( \theta ) j + 6 sin( \theta ) k C) r = 2 cos( \theta ) i + 2 sin( \theta ) j - 6 sin( \theta ) k D) r = 2 cos( \theta ) i - 2 sin( \theta ) j - 6 sin( \theta ) k E) r = 2 cos( \theta ) i + 2 sin( \theta ) j + 3 sin( \theta ) k](https://storage.examlex.com/TB9661/11ee77e1_77bc_4cc3_a0f8_6935ec97e78a_TB9661_11.jpg) = 4, using the polar angle in the xy-plane as the parameter, [0, 2 ] as parameter interval, and ensuring that the ellipse is oriented counterclockwise as viewed from high on the z-axis.
= 4, using the polar angle in the xy-plane as the parameter, [0, 2 ] as parameter interval, and ensuring that the ellipse is oriented counterclockwise as viewed from high on the z-axis.
A) r = 2 cos( ) i + 2 sin( ) j + 6 sin( ) k
B) r = 2 cos( ) i - 2 sin( ) j + 6 sin( ) k
C) r = 2 cos( ) i + 2 sin( ) j - 6 sin( ) k
D) r = 2 cos( ) i - 2 sin( ) j - 6 sin( ) k
E) r = 2 cos( ) i + 2 sin( ) j + 3 sin( ) k
![<strong>Find a parametrization of the ellipse in which the plane z = 3y intersects the cylinder + = 4, using the polar angle \theta in the xy-plane as the parameter, [0, 2 \pi ] as parameter interval, and ensuring that the ellipse is oriented counterclockwise as viewed from high on the z-axis.</strong> A) r = 2 cos( \theta ) i + 2 sin( \theta ) j + 6 sin( \theta ) k B) r = 2 cos( \theta ) i - 2 sin( \theta ) j + 6 sin( \theta ) k C) r = 2 cos( \theta ) i + 2 sin( \theta ) j - 6 sin( \theta ) k D) r = 2 cos( \theta ) i - 2 sin( \theta ) j - 6 sin( \theta ) k E) r = 2 cos( \theta ) i + 2 sin( \theta ) j + 3 sin( \theta ) k](https://storage.examlex.com/TB9661/11ee77e1_77bc_4cc2_a0f8_47790a5bc80a_TB9661_11.jpg) +
+ ![<strong>Find a parametrization of the ellipse in which the plane z = 3y intersects the cylinder + = 4, using the polar angle \theta in the xy-plane as the parameter, [0, 2 \pi ] as parameter interval, and ensuring that the ellipse is oriented counterclockwise as viewed from high on the z-axis.</strong> A) r = 2 cos( \theta ) i + 2 sin( \theta ) j + 6 sin( \theta ) k B) r = 2 cos( \theta ) i - 2 sin( \theta ) j + 6 sin( \theta ) k C) r = 2 cos( \theta ) i + 2 sin( \theta ) j - 6 sin( \theta ) k D) r = 2 cos( \theta ) i - 2 sin( \theta ) j - 6 sin( \theta ) k E) r = 2 cos( \theta ) i + 2 sin( \theta ) j + 3 sin( \theta ) k](https://storage.examlex.com/TB9661/11ee77e1_77bc_4cc3_a0f8_6935ec97e78a_TB9661_11.jpg) = 4, using the polar angle in the xy-plane as the parameter, [0, 2 ] as parameter interval, and ensuring that the ellipse is oriented counterclockwise as viewed from high on the z-axis.
= 4, using the polar angle in the xy-plane as the parameter, [0, 2 ] as parameter interval, and ensuring that the ellipse is oriented counterclockwise as viewed from high on the z-axis.A) r = 2 cos( ) i + 2 sin( ) j + 6 sin( ) k
B) r = 2 cos( ) i - 2 sin( ) j + 6 sin( ) k
C) r = 2 cos( ) i + 2 sin( ) j - 6 sin( ) k
D) r = 2 cos( ) i - 2 sin( ) j - 6 sin( ) k
E) r = 2 cos( ) i + 2 sin( ) j + 3 sin( ) k

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
31
Parametrize the parabola in which the plane z = 1 + y intersects the cone  +
+ 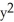 =
=  using x as the parameter.
using x as the parameter.
A) r = x i +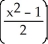 j +
j + 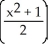 k, - < x <
k, - < x <
B) r = x i +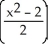 j +
j + 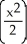 k, - < x <
k, - < x <
C) r = x i +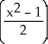 j +
j + 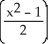 k, - < x <
k, - < x <
D) r = x i +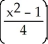 j +
j +  k, - < x <
k, - < x <
E) r = x i + j +
j + 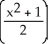 k, - < x <
k, - < x <
 +
+ 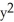 =
=  using x as the parameter.
using x as the parameter.A) r = x i +
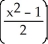 j +
j + 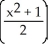 k, - < x <
k, - < x < B) r = x i +
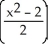 j +
j + 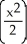 k, - < x <
k, - < x < C) r = x i +
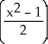 j +
j + 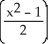 k, - < x <
k, - < x < D) r = x i +
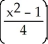 j +
j +  k, - < x <
k, - < x < E) r = x i +
 j +
j + 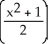 k, - < x <
k, - < x < 
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
32
Find the length of the arc r = u i +  j, 0 u
j, 0 u  .
.
A)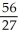 units
units
B)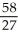 units
units
C)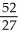 units
units
D)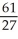 units
units
E)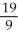 units
units
 j, 0 u
j, 0 u  .
.A)
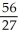 units
unitsB)
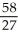 units
unitsC)
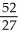 units
unitsD)
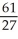 units
unitsE)
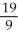 units
units
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
33
Find the perimeter of the astroid curve x = 2 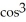 , y = 2
, y = 2 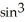 .
.
A) 16 units
B) 6 units
C) 3 units
D) 12 units
E) 9 units
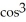 , y = 2
, y = 2 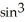 .
.A) 16 units
B) 6 units
C) 3 units
D) 12 units
E) 9 units

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
34
Find the arc length of the arc r = (ln (cos ) ) i + j, - 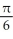 0.
0.
A)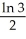 units
units
B) ln (2 +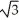 ) units
) units
C) 2 units
D)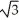 units
units
E)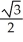 units
units
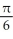 0.
0.A)
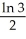 units
unitsB) ln (2 +
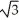 ) units
) unitsC) 2 units
D)
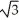 units
unitsE)
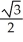 units
units
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
35
Find the length of the curve r(t) = ( 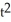 sin t) i + (
sin t) i + ( 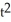 cos t) j + 2t k for 0 t 2 .
cos t) j + 2t k for 0 t 2 .
A) + 2 units
+ 2 units
B)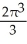 + 4 units
+ 4 units
C)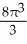 + 4 units
+ 4 units
D)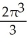 + 2 units
+ 2 units
E)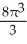 + 2 units
+ 2 units
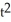 sin t) i + (
sin t) i + ( 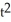 cos t) j + 2t k for 0 t 2 .
cos t) j + 2t k for 0 t 2 .A)
 + 2 units
+ 2 unitsB)
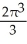 + 4 units
+ 4 unitsC)
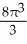 + 4 units
+ 4 unitsD)
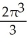 + 2 units
+ 2 unitsE)
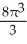 + 2 units
+ 2 units
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
36
Find the arc length of the space curve given by the vector equation r = t i + 2ln(t) j +(1 -  ) k, 1 t 2.
) k, 1 t 2.
A) 1 + 2ln(4) units
B)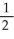 unit
unit
C) 2 units
D) 1 unit
E) 3 units
 ) k, 1 t 2.
) k, 1 t 2.A) 1 + 2ln(4) units
B)
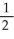 unit
unitC) 2 units
D) 1 unit
E) 3 units

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
37
Parametrize the curve of intersection of the cylinder y = 1 -  and the plane z = - x using as parameter the slope m =
and the plane z = - x using as parameter the slope m = 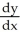 of the curve of intersection of the cylinder with the xy-plane.
of the curve of intersection of the cylinder with the xy-plane.
A) x = -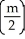 i +
i + 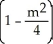 j + m k
j + m k
B) x = - m i +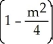 j + m k
j + m k
C) x = -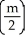 i +
i + 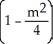 j +
j + 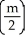 k
k
D) x = -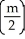 i +
i + 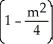 j -
j - 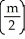 k
k
E) x = i +
i + 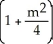 j -
j - 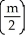 k
k
 and the plane z = - x using as parameter the slope m =
and the plane z = - x using as parameter the slope m = 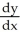 of the curve of intersection of the cylinder with the xy-plane.
of the curve of intersection of the cylinder with the xy-plane.A) x = -
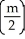 i +
i + 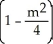 j + m k
j + m kB) x = - m i +
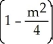 j + m k
j + m kC) x = -
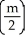 i +
i + 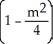 j +
j + 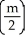 k
kD) x = -
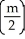 i +
i + 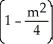 j -
j - 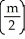 k
kE) x =
 i +
i + 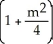 j -
j - 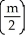 k
k
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
38
Find a parametric representation of the curve of intersection of the paraboloid z = x2 + y2 and the plane 8x - 4y - z - 11 = 0.
A) r = (3 - 4cos(t)) i + (3 + 2sin(t)) j + (1 - 32cos(t) - 8sin(t)) k, 0 t 2
B) r = (3 + 4cos(t)) i + (3 - 2sin(t)) j + (1 + 32cos(t) + 8sin(t)) k, 0 t 2
C) r = (4 + 3cos(t)) i + (-2 + 3sin(t)) j + (24cos(t) - 12sin(t) - 29) k, 0 t 2
D) r = (-4 + 3cos(t)) i + (2 + 3sin(t)) j + (24cos(t) - 12sin(t) - 51) k, 0 t 2
E) r = (-3 + 4cos(t)) i + (-3 - 2sin(t)) j + (32cos(t) + 8sin(t) - 23) k, 0 t 2
A) r = (3 - 4cos(t)) i + (3 + 2sin(t)) j + (1 - 32cos(t) - 8sin(t)) k, 0 t 2
B) r = (3 + 4cos(t)) i + (3 - 2sin(t)) j + (1 + 32cos(t) + 8sin(t)) k, 0 t 2
C) r = (4 + 3cos(t)) i + (-2 + 3sin(t)) j + (24cos(t) - 12sin(t) - 29) k, 0 t 2
D) r = (-4 + 3cos(t)) i + (2 + 3sin(t)) j + (24cos(t) - 12sin(t) - 51) k, 0 t 2
E) r = (-3 + 4cos(t)) i + (-3 - 2sin(t)) j + (32cos(t) + 8sin(t) - 23) k, 0 t 2

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
39
Reparametrize the curve r = 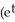 cos t) i + (
cos t) i + ( 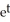 sin t) j +
sin t) j +  k in terms of arc length s measured from the point where t = 0.
k in terms of arc length s measured from the point where t = 0.
A) r = cos
cos 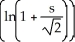 i +
i + 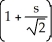 sin
sin 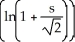 j +
j + 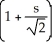 k
k
B) r =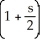 cos
cos 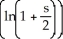 i +
i + 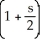 sin
sin 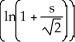 j +
j + 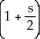 k
k
C) r =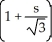 cos
cos  i +
i + 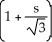 sin
sin 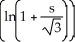 j +
j + 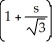 k
k
D) r =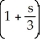 cos
cos  i +
i +  sin
sin 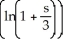 j +
j + 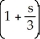 k
k
E) r =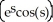 i +
i + 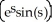 j +
j + 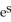 k
k
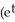 cos t) i + (
cos t) i + ( 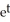 sin t) j +
sin t) j +  k in terms of arc length s measured from the point where t = 0.
k in terms of arc length s measured from the point where t = 0.A) r =
 cos
cos 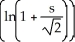 i +
i + 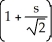 sin
sin 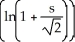 j +
j + 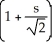 k
kB) r =
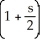 cos
cos 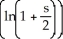 i +
i + 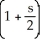 sin
sin 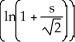 j +
j + 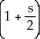 k
kC) r =
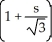 cos
cos  i +
i + 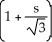 sin
sin 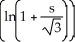 j +
j + 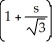 k
kD) r =
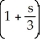 cos
cos  i +
i +  sin
sin 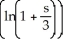 j +
j + 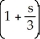 k
kE) r =
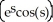 i +
i + 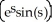 j +
j + 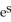 k
k
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
40
A parametric representation of the curve of intersection of the two surfaces 4x2 + y2 + z2 = 8 and  is given by which of the following vector equations?
is given by which of the following vector equations?
A) r = 2cos(t) i + sin(t) j - 2 k, 0 t 2
B) r = 2cos(t) i + sin(t) j + 2 k, 0 t 2
C) r = cos(t) i + 2sin(t) j +2 k, 0 t
D) r = cos(t) i + 2sin(t) j - 2 k, 0 t 2
E) r = 2cos(t) i + sin(t) j - 2 k, 0 t
 is given by which of the following vector equations?
is given by which of the following vector equations?A) r = 2cos(t) i + sin(t) j - 2 k, 0 t 2
B) r = 2cos(t) i + sin(t) j + 2 k, 0 t 2
C) r = cos(t) i + 2sin(t) j +2 k, 0 t
D) r = cos(t) i + 2sin(t) j - 2 k, 0 t 2
E) r = 2cos(t) i + sin(t) j - 2 k, 0 t

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
41
A recording tape 0.01 cm thick is wound around a reel whose inner radius is 1 cm and outer radius is 4 cm. How much tape is required to fill the reel?
A) 1885 cm
B) 1178 cm
C) 4712 cm
D) 2827 cm
E) 5238 cm
A) 1885 cm
B) 1178 cm
C) 4712 cm
D) 2827 cm
E) 5238 cm

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
42
Let 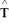 ,
,  , and
, and 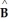 be the unit tangent, the principal unit normal, and the unit binormal, respectively.Which of the following equations is correct?
be the unit tangent, the principal unit normal, and the unit binormal, respectively.Which of the following equations is correct?
A)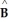 =
=  ×
× 
B)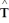 =
= 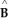 ×
× 
C)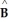 =
=  .
. 
D)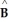 =
=  ×
× 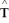
E)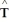 =
=  +
+ 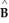
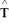 ,
,  , and
, and 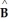 be the unit tangent, the principal unit normal, and the unit binormal, respectively.Which of the following equations is correct?
be the unit tangent, the principal unit normal, and the unit binormal, respectively.Which of the following equations is correct?A)
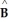 =
=  ×
× 
B)
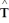 =
= 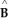 ×
× 
C)
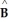 =
=  .
. 
D)
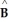 =
=  ×
× 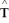
E)
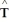 =
=  +
+ 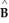

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
43
Find the curvature of a circle with radius a.
A)
B)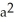
C) -
D) a
E)
A)

B)
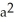
C) -

D) a
E)


Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
44
Find the radius of curvature of the plane curve 4x2 + 4y2 + 16x - 12y -11 = 0.
A) 6
B) 3
C) 2
D)
E)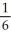
A) 6
B) 3
C) 2
D)

E)

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
45
Find 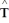 and
and  for the circular motion described by r(t) = (cos bt) i + (sin bt) j.
for the circular motion described by r(t) = (cos bt) i + (sin bt) j.
A) = -(sin bt) i + (cos bt) j and
= -(sin bt) i + (cos bt) j and  = -(cos bt) i - (sin bt) j
= -(cos bt) i - (sin bt) j
B)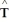 = (sin bt) i + (cos bt) j and
= (sin bt) i + (cos bt) j and  = (cos bt) i - (sin bt) j
= (cos bt) i - (sin bt) j
C)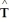 = -(sin bt) i + (cos bt) j and
= -(sin bt) i + (cos bt) j and  = -(cos bt) i + (sin bt) j
= -(cos bt) i + (sin bt) j
D)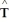 = -(sin bt) i - (cos bt) j and
= -(sin bt) i - (cos bt) j and  = -(cos bt) i + (sin bt) j
= -(cos bt) i + (sin bt) j
E)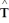 = (sin bt) i + (cos bt) j and
= (sin bt) i + (cos bt) j and  = -(cos bt) i + (sin bt) j
= -(cos bt) i + (sin bt) j
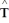 and
and  for the circular motion described by r(t) = (cos bt) i + (sin bt) j.
for the circular motion described by r(t) = (cos bt) i + (sin bt) j.A)
 = -(sin bt) i + (cos bt) j and
= -(sin bt) i + (cos bt) j and  = -(cos bt) i - (sin bt) j
= -(cos bt) i - (sin bt) jB)
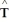 = (sin bt) i + (cos bt) j and
= (sin bt) i + (cos bt) j and  = (cos bt) i - (sin bt) j
= (cos bt) i - (sin bt) jC)
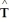 = -(sin bt) i + (cos bt) j and
= -(sin bt) i + (cos bt) j and  = -(cos bt) i + (sin bt) j
= -(cos bt) i + (sin bt) jD)
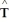 = -(sin bt) i - (cos bt) j and
= -(sin bt) i - (cos bt) j and  = -(cos bt) i + (sin bt) j
= -(cos bt) i + (sin bt) jE)
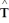 = (sin bt) i + (cos bt) j and
= (sin bt) i + (cos bt) j and  = -(cos bt) i + (sin bt) j
= -(cos bt) i + (sin bt) j
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
46
For what positive value of the constant c is the curve r = (3 cos cs) i + (3 sin cs) j + 4cs k parametrized in terms of arc length s? What is the curvature 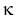 (s) and the torsion
(s) and the torsion  (s) for this curve? What kind of curve is it?
(s) for this curve? What kind of curve is it?
A) c =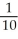 , 11ee7b4a_c535_8783_ae82_218a633b3a19_TB9661_11 =
, 11ee7b4a_c535_8783_ae82_218a633b3a19_TB9661_11 = 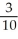 , 11ee7b4a_f17d_2194_ae82_9be00d89db23_TB9661_11 = 0, circle
, 11ee7b4a_f17d_2194_ae82_9be00d89db23_TB9661_11 = 0, circle
B) c = , 11ee7b4a_c535_8783_ae82_218a633b3a19_TB9661_11 =
, 11ee7b4a_c535_8783_ae82_218a633b3a19_TB9661_11 =  , 11ee7b4a_f17d_2194_ae82_9be00d89db23_TB9661_11 =
, 11ee7b4a_f17d_2194_ae82_9be00d89db23_TB9661_11 = 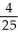 , circular helix
, circular helix
C) c = , 11ee7b4a_c535_8783_ae82_218a633b3a19_TB9661_11 =
, 11ee7b4a_c535_8783_ae82_218a633b3a19_TB9661_11 =  , 11ee7b4a_f17d_2194_ae82_9be00d89db23_TB9661_11=
, 11ee7b4a_f17d_2194_ae82_9be00d89db23_TB9661_11=  , circular helix
, circular helix
D) c =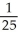 , 11ee7b4a_c535_8783_ae82_218a633b3a19_TB9661_11 =
, 11ee7b4a_c535_8783_ae82_218a633b3a19_TB9661_11 = 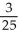 , 11ee7b4a_f17d_2194_ae82_9be00d89db23_TB9661_11 =
, 11ee7b4a_f17d_2194_ae82_9be00d89db23_TB9661_11 = 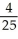 , circular helix
, circular helix
E) c = , 11ee7b4a_c535_8783_ae82_218a633b3a19_TB9661_11 =
, 11ee7b4a_c535_8783_ae82_218a633b3a19_TB9661_11 = 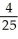 , 11ee7b4a_f17d_2194_ae82_9be00d89db23_TB9661_11 =
, 11ee7b4a_f17d_2194_ae82_9be00d89db23_TB9661_11 = 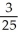 , circular helix
, circular helix
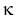 (s) and the torsion
(s) and the torsion  (s) for this curve? What kind of curve is it?
(s) for this curve? What kind of curve is it?A) c =
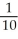 , 11ee7b4a_c535_8783_ae82_218a633b3a19_TB9661_11 =
, 11ee7b4a_c535_8783_ae82_218a633b3a19_TB9661_11 = 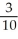 , 11ee7b4a_f17d_2194_ae82_9be00d89db23_TB9661_11 = 0, circle
, 11ee7b4a_f17d_2194_ae82_9be00d89db23_TB9661_11 = 0, circleB) c =
 , 11ee7b4a_c535_8783_ae82_218a633b3a19_TB9661_11 =
, 11ee7b4a_c535_8783_ae82_218a633b3a19_TB9661_11 =  , 11ee7b4a_f17d_2194_ae82_9be00d89db23_TB9661_11 =
, 11ee7b4a_f17d_2194_ae82_9be00d89db23_TB9661_11 = 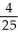 , circular helix
, circular helixC) c =
 , 11ee7b4a_c535_8783_ae82_218a633b3a19_TB9661_11 =
, 11ee7b4a_c535_8783_ae82_218a633b3a19_TB9661_11 =  , 11ee7b4a_f17d_2194_ae82_9be00d89db23_TB9661_11=
, 11ee7b4a_f17d_2194_ae82_9be00d89db23_TB9661_11=  , circular helix
, circular helixD) c =
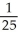 , 11ee7b4a_c535_8783_ae82_218a633b3a19_TB9661_11 =
, 11ee7b4a_c535_8783_ae82_218a633b3a19_TB9661_11 = 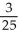 , 11ee7b4a_f17d_2194_ae82_9be00d89db23_TB9661_11 =
, 11ee7b4a_f17d_2194_ae82_9be00d89db23_TB9661_11 = 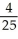 , circular helix
, circular helixE) c =
 , 11ee7b4a_c535_8783_ae82_218a633b3a19_TB9661_11 =
, 11ee7b4a_c535_8783_ae82_218a633b3a19_TB9661_11 = 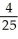 , 11ee7b4a_f17d_2194_ae82_9be00d89db23_TB9661_11 =
, 11ee7b4a_f17d_2194_ae82_9be00d89db23_TB9661_11 = 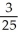 , circular helix
, circular helix
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
47
Let  : I
: I 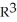 be the unit speed vector 11ee7b18_ef83_6016_ae82_a1fef198316c_TB9661_11 (s) =
be the unit speed vector 11ee7b18_ef83_6016_ae82_a1fef198316c_TB9661_11 (s) = 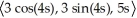 . Compute the curvature and torsion of https://storage.examlex.com/TB9661/11ee7b18_ef83_6016_ae82_a1fef198316c_TB9661_11.
. Compute the curvature and torsion of https://storage.examlex.com/TB9661/11ee7b18_ef83_6016_ae82_a1fef198316c_TB9661_11.
A) Curvature is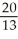 and torsion is
and torsion is 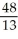
B) Curvature is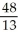 and torsion is
and torsion is 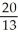
C) Curvature is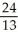 and torsion is
and torsion is 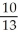
D) Curvature is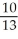 and torsion is
and torsion is 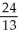
E) Curvature is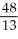 and torsion is -
and torsion is - 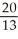
 : I
: I 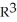 be the unit speed vector 11ee7b18_ef83_6016_ae82_a1fef198316c_TB9661_11 (s) =
be the unit speed vector 11ee7b18_ef83_6016_ae82_a1fef198316c_TB9661_11 (s) = 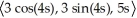 . Compute the curvature and torsion of https://storage.examlex.com/TB9661/11ee7b18_ef83_6016_ae82_a1fef198316c_TB9661_11.
. Compute the curvature and torsion of https://storage.examlex.com/TB9661/11ee7b18_ef83_6016_ae82_a1fef198316c_TB9661_11.A) Curvature is
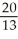 and torsion is
and torsion is 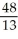
B) Curvature is
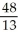 and torsion is
and torsion is 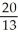
C) Curvature is
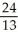 and torsion is
and torsion is 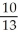
D) Curvature is
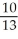 and torsion is
and torsion is 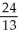
E) Curvature is
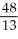 and torsion is -
and torsion is - 

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
48
Let r = r(s) be a curve parametrized in terms of arc length, let 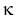 (s) and
(s) and  (s) be the curvature and torsion, and let {
(s) be the curvature and torsion, and let {  ,
,  ,
, 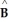 } be the Frenet frame for the curve. Suppose that
} be the Frenet frame for the curve. Suppose that  = 0 and
= 0 and 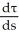 = 0 for
= 0 for  Calculate
Calculate 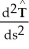 ,
,  , and
, and 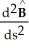 in terms of
in terms of 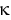 ,
, 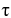 ,
, 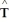 ,
,  , and
, and 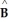 .
.
A)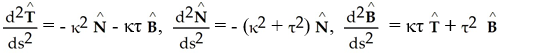
B)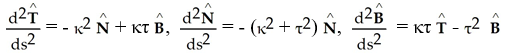
C)
D)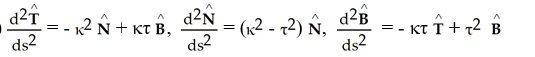
E)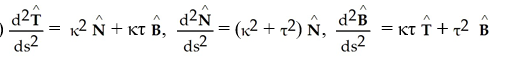
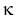 (s) and
(s) and  (s) be the curvature and torsion, and let {
(s) be the curvature and torsion, and let {  ,
,  ,
, 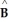 } be the Frenet frame for the curve. Suppose that
} be the Frenet frame for the curve. Suppose that  = 0 and
= 0 and 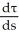 = 0 for
= 0 for 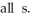 Calculate
Calculate 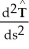 ,
,  , and
, and 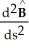 in terms of
in terms of 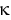 ,
, 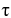 ,
, 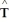 ,
,  , and
, and 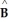 .
.A)
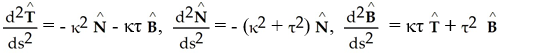
B)
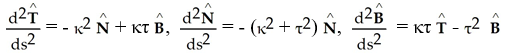
C)

D)
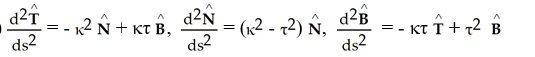
E)
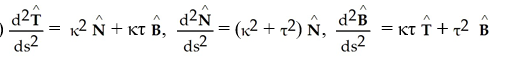

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
49
A curve with constant curvature and constant torsion is a circular helix. What is the radius of the cylinder on which the helix is wound if the curvature is 1 and the torsion is 2?
A)
B)
C)
D) 5
E) 1
A)

B)

C)

D) 5
E) 1

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
50
A curve can have constant curvature 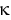 = 2 and constant torsion
= 2 and constant torsion 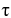 = 0.
= 0.
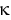 = 2 and constant torsion
= 2 and constant torsion 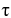 = 0.
= 0.
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
51
A curve can have constant curvature 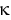 = 0 and constant torsion
= 0 and constant torsion 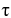 = 2.
= 2.
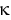 = 0 and constant torsion
= 0 and constant torsion 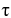 = 2.
= 2.
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
52
The curve r = r(s) is a straight line if and only if  (s) = 0 for all s.
(s) = 0 for all s.
 (s) = 0 for all s.
(s) = 0 for all s.
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
53
A curve r(s) parametrized in terms of arc length s is traced at unit speed (thus,  =1).
=1).
 =1).
=1).
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
54
Find the curvature of the parabola y = x2 at the point (0, 0).
A) 2
B) 1
C) 4
D)
E)
A) 2
B) 1
C) 4
D)

E)


Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
55
Find the curvature of the hyperbola xy = 1 at (1, 1).
A)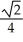
B) 2
C)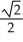
D)
E) 2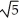
A)
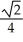
B) 2
C)
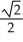
D)

E) 2
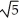

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
56
Find the maximum and minimum values of the curvature of the ellipse r = (3 cos t) i + (2 sin t) j.
A) max , min
, min 
B) max , min
, min 
C) max , min
, min 
D) max 2, min
E) max , min
, min 
A) max
 , min
, min 
B) max
 , min
, min 
C) max
 , min
, min 
D) max 2, min

E) max
 , min
, min 

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
57
At what value of x is the radius of curvature of y = ex smallest?
A) -
B)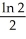
C) -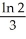
D)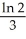
E)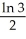
A) -

B)
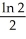
C) -
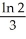
D)
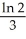
E)
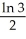

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
58
Find 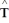 and
and  for the plane curve r(t) = (2t + 3) i + (5 -
for the plane curve r(t) = (2t + 3) i + (5 - 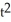 ) j.
) j.
A)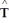 =
= 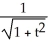 i +
i + 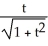 j,
j,  = -
= - 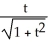 i +
i +  j
j
B)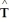 =
=  i +
i + 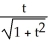 j,
j,  =
= 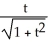 i -
i - 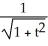 j
j
C)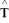 =
= 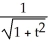 i -
i - 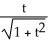 j,
j,  =
= 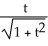 i +
i + 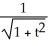 j
j
D)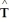 =
=  i -
i - 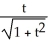 j,
j,  = -
= - 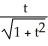 i -
i -  j
j
E)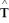 =
= 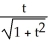 i -
i -  j,
j,  = -
= - 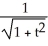 i -
i -  j
j
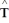 and
and  for the plane curve r(t) = (2t + 3) i + (5 -
for the plane curve r(t) = (2t + 3) i + (5 - 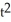 ) j.
) j.A)
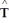 =
= 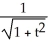 i +
i + 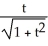 j,
j,  = -
= - 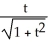 i +
i +  j
jB)
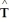 =
=  i +
i + 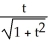 j,
j,  =
= 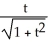 i -
i - 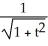 j
jC)
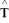 =
= 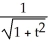 i -
i - 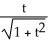 j,
j,  =
= 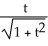 i +
i + 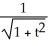 j
jD)
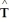 =
=  i -
i - 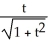 j,
j,  = -
= - 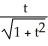 i -
i -  j
jE)
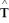 =
= 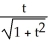 i -
i -  j,
j,  = -
= - 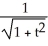 i -
i -  j
j
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
59
The acceleration, the unit tangent, and the principal unit normal of a moving particle in space at a particular time are respectively given by
a = 12 i + 3 j -12 k ,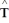 =
=  ( 2 i + j - 2 k ), and
( 2 i + j - 2 k ), and  =
= 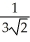 ( i -4 j - k )
( i -4 j - k )
The tangential component of the acceleration is equal to:
A) 2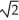 ( i - j + k )
( i - j + k )
B) 17
C) 8 i + j + 8 k
D) 2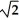
E) 0
a = 12 i + 3 j -12 k ,
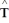 =
=  ( 2 i + j - 2 k ), and
( 2 i + j - 2 k ), and  =
= 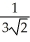 ( i -4 j - k )
( i -4 j - k )The tangential component of the acceleration is equal to:
A) 2
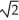 ( i - j + k )
( i - j + k )B) 17
C) 8 i + j + 8 k
D) 2
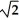
E) 0

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
60
Find the radius of curvature of the curve r = (2 cos t) i + (2 sin t) j + (2 sin t) k.
A)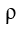 = 2
= 2 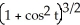
B) 11efb6fe_8167_da73_bb63_af6e34405a3b_TB9661_00 = 2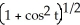
C) 11efb6fe_8167_da73_bb63_af6e34405a3b_TB9661_00 =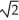
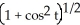
D) 11efb6fe_8167_da73_bb63_af6e34405a3b_TB9661_00 =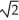

E)11efb6fe_8167_da73_bb63_af6e34405a3b_TB9661_00 =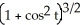
A)
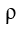 = 2
= 2 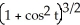
B) 11efb6fe_8167_da73_bb63_af6e34405a3b_TB9661_00 = 2
C) 11efb6fe_8167_da73_bb63_af6e34405a3b_TB9661_00 =
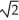
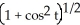
D) 11efb6fe_8167_da73_bb63_af6e34405a3b_TB9661_00 =
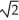

E)11efb6fe_8167_da73_bb63_af6e34405a3b_TB9661_00 =
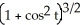

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
61
Find the curvature of r = ( 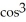 t) i + (
t) i + ( 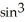 t) j at t =
t) j at t = 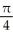 .
.
A)
B) -
C)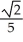
D)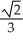
E)
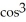 t) i + (
t) i + ( 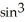 t) j at t =
t) j at t = 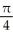 .
.A)

B) -

C)
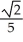
D)
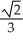
E)


Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
62
Let C be the space curve given by r(t) = (t - 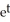 ) i + 3t j + (2t -
) i + 3t j + (2t - 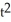 ) k. The unit binormal to curve C at t = 0 is given by
) k. The unit binormal to curve C at t = 0 is given by
A) - i -
i -  j +
j +  k
k
B) -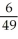 i +
i +  j +
j + 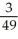 k
k
C) - i +
i +  j +
j +  k
k
D) - i -
i -  j +
j +  k
k
E) - k
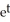 ) i + 3t j + (2t -
) i + 3t j + (2t - 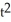 ) k. The unit binormal to curve C at t = 0 is given by
) k. The unit binormal to curve C at t = 0 is given byA) -
 i -
i -  j +
j +  k
kB) -
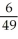 i +
i +  j +
j + 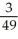 k
kC) -
 i +
i +  j +
j +  k
kD) -
 i -
i -  j +
j +  k
kE) - k

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
63
Find the curvature and the torsion of the curve of intersection of the surfaces x2 + y2 + z2 = 18 and 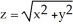 at an arbitrary point (x, y, z).
at an arbitrary point (x, y, z).
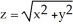 at an arbitrary point (x, y, z).
at an arbitrary point (x, y, z).
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
64
Find the point on the curve r(t) = (5 sin t) i + (5 cos t) j + 12t k at a distance 26 units along the curve from the point (0, 5, 0) when t > 0 corresponds to the direction of increasing arc length.
A) (-5, 0, 2 )
B) (5, 0, 12 )
C) (0, 5, 26 )
D) (0, 5, 24 )
E) (5, 0,12 )
A) (-5, 0, 2 )
B) (5, 0, 12 )
C) (0, 5, 26 )
D) (0, 5, 24 )
E) (5, 0,12 )

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
65
Find the radius of curvature of r = 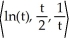 .
.
A)
B)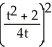
C)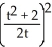
D)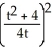
E)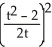
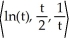 .
.A)

B)
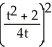
C)
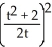
D)
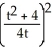
E)
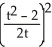

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
66
Find the Frenet frame for the curve r =  .
.
A)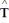 (t) =
(t) = 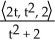 ,
,  (t) =
(t) = 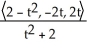 ,
,  (t) =
(t) = 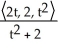
B) (t) =
(t) = 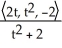 ,
,  (t) =
(t) =  ,
,  (t) =
(t) = 
C) (t) =
(t) = 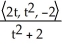 ,
,  (t) =
(t) = 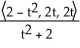 ,
, 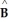 (t) =
(t) = 
D)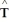 (t) =
(t) = 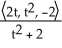 ,
,  (t) =
(t) = 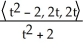 ,
, 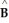 (t) =
(t) = 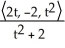
E)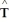 (t) =
(t) = 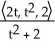 ,
,  (t) =
(t) = 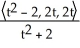 ,
,  (t) =
(t) = 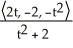
 .
.A)
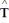 (t) =
(t) = 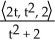 ,
,  (t) =
(t) = 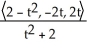 ,
,  (t) =
(t) = 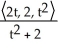
B)
 (t) =
(t) = 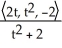 ,
,  (t) =
(t) =  ,
,  (t) =
(t) = 
C)
 (t) =
(t) = 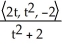 ,
,  (t) =
(t) = 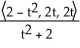 ,
, 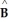 (t) =
(t) = 
D)
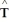 (t) =
(t) = 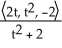 ,
,  (t) =
(t) = 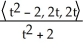 ,
, 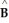 (t) =
(t) = 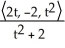
E)
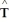 (t) =
(t) = 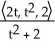 ,
,  (t) =
(t) = 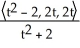 ,
,  (t) =
(t) = 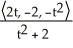

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
67
Find an equation for the osculating circle of the curve r = t i + (sin t) j at the point ( 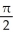 , 1).
, 1).
A)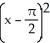 +
+ 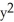 = 4
= 4
B) +
+ 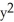 = 2
= 2
C)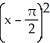 +
+ 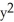 = 1
= 1
D)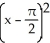 +
+ 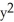 =
= 
E)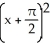 +
+ 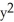 = 2
= 2
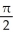 , 1).
, 1).A)
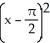 +
+ 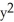 = 4
= 4B)
 +
+ 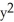 = 2
= 2C)
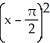 +
+ 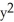 = 1
= 1D)
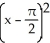 +
+ 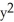 =
= 
E)
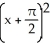 +
+ 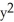 = 2
= 2
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
68
Find the radius of curvature of the cycloid r = a ( - sin ) i + a(1 - cos ) j at the point = . Assume that a > 0.
A) 4a
B)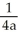
C) 2a
D)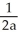
E) a
A) 4a
B)
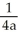
C) 2a
D)
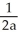
E) a

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
69
The speed and the magnitude of the acceleration of a moving particle at some point P are 3 units and 4 units, respectively. If the angle between the velocity and acceleration vectors at the point P is 30°, determine the radius of curvature of the particle's trajectory at P.
A)
B) 4.5
C)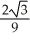
D)
E) 2.25
F)
A)

B) 4.5
C)
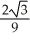
D)

E) 2.25
F)


Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
70
Find the tangential and normal components of the acceleration of a particle moving so that at time t its position is r(t) = (  cos t) i + (
cos t) i + ( 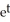 sin t) j.
sin t) j.
A)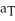 =
= 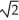
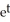 ,
, 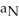 =
= 
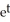
B)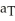 = 2
= 2 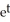 ,
, 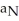 =
= 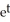
C)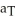 =
= 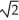
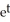 ,
, 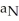 = 2
= 2 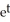
D)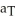 = 2
= 2 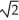
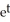 ,
, 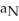 =
= 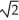
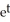
E)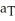 =
= 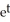 ,
, 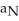 =
= 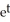
 cos t) i + (
cos t) i + ( 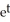 sin t) j.
sin t) j.A)
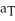 =
= 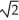
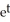 ,
, 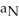 =
= 
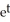
B)
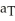 = 2
= 2 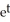 ,
,  =
= 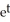
C)
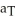 =
= 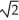
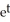 ,
, 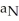 = 2
= 2 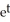
D)
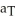 = 2
= 2 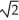
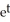 ,
, 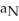 =
= 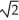
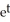
E)
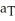 =
= 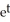 ,
, 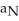 =
= 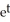

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
71
Find the evolute 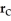 (t) of the curve r(t) = (
(t) of the curve r(t) = ( 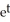 cos t) i + (
cos t) i + ( 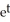 sin t) j + 2k.
sin t) j + 2k.
A)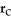 = - (
= - ( 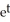 sin t) i + (
sin t) i + (  cos t) j + 2k
cos t) j + 2k
B) = (
= ( 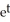 sin t) i + (
sin t) i + (  cos t) j + 2k
cos t) j + 2k
C)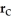 = (
= ( 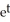 sin t) i - (
sin t) i - ( 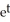 cos t) j
cos t) j
D)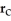 = - (
= - ( 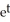 sin t) i - (
sin t) i - ( 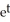 cos t) j
cos t) j
E)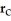 = - (
= - (  sin t) i - (
sin t) i - ( 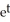 cos t) j + k
cos t) j + k
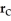 (t) of the curve r(t) = (
(t) of the curve r(t) = ( 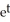 cos t) i + (
cos t) i + ( 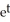 sin t) j + 2k.
sin t) j + 2k.A)
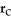 = - (
= - ( 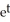 sin t) i + (
sin t) i + (  cos t) j + 2k
cos t) j + 2kB)
 = (
= ( 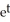 sin t) i + (
sin t) i + (  cos t) j + 2k
cos t) j + 2kC)
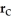 = (
= ( 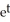 sin t) i - (
sin t) i - ( 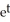 cos t) j
cos t) jD)
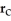 = - (
= - ( 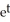 sin t) i - (
sin t) i - ( 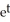 cos t) j
cos t) jE)
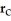 = - (
= - (  sin t) i - (
sin t) i - ( 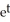 cos t) j + k
cos t) j + k
Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
72
Find the evolute  (t) of the curve r(t) = (2 cos t) i + (2 sin t) j + 3t k.
(t) of the curve r(t) = (2 cos t) i + (2 sin t) j + 3t k.
A)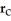 = -
= -  sin t i -
sin t i -  cos t j + 3t k
cos t j + 3t k
B)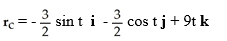
C)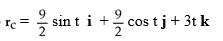
D)
E)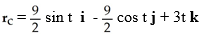
 (t) of the curve r(t) = (2 cos t) i + (2 sin t) j + 3t k.
(t) of the curve r(t) = (2 cos t) i + (2 sin t) j + 3t k.A)
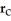 = -
= -  sin t i -
sin t i -  cos t j + 3t k
cos t j + 3t kB)
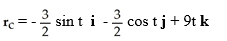
C)
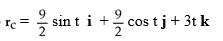
D)

E)
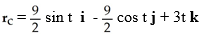

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
73
Find a polynomial f(x) of lowest possible degree such that the curve y = f(x), 0 x 1, can be used to join the straight line segments y = 0, x 0, and y = 1, x 1, to form a curve along which a particle can travel at constant speed without experiencing discontinuous acceleration.
A) f(x) = 10 - 15
- 15 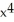 + 6
+ 6 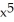
B) f(x) = 4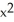 - 7
- 7 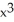 + 4
+ 4 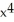
C) f(x) = 2 - 3
- 3  + 2
+ 2 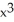
D) f(x) = 18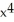 - 30
- 30 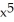 + 13
+ 13 
E) f(x) =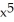 - 3
- 3 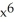 + 2
+ 2 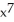
A) f(x) = 10
 - 15
- 15 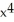 + 6
+ 6 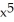
B) f(x) = 4
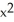 - 7
- 7 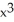 + 4
+ 4 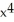
C) f(x) = 2
 - 3
- 3  + 2
+ 2 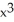
D) f(x) = 18
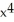 - 30
- 30 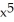 + 13
+ 13 
E) f(x) =
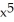 - 3
- 3 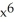 + 2
+ 2 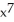

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
74
A curve with nonzero curvature lies in a plane if and only if the torsion of the curve is identically zero.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
75
A frictionless highway turn has a constant curvature 1.96 × 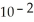
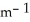 and is banked at an angle =
and is banked at an angle = 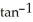 (0.2). What is the maximum safe speed for the turn in m/s?
(0.2). What is the maximum safe speed for the turn in m/s?
You may assume the gravitational acceleration g = 9.8 m/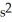 .
.
A) 100 m/s
B) 19.6 m/s
C) 20 m/s
D) 25 m/s
E) 10 m/s
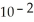
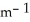 and is banked at an angle =
and is banked at an angle = 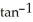 (0.2). What is the maximum safe speed for the turn in m/s?
(0.2). What is the maximum safe speed for the turn in m/s?You may assume the gravitational acceleration g = 9.8 m/
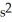 .
.A) 100 m/s
B) 19.6 m/s
C) 20 m/s
D) 25 m/s
E) 10 m/s

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
76
A frictionless road turn is approximately circular of radius 50 metres and is designed for a maximum safe speed of 10 m/s. Determine the banking angle of the turn to the nearest degree.You may assume the gravitational acceleration g = 9.8 m/s2.

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
77
The period of the moon's orbit around the Earth is approximately 27.32 days, and the semi-major axis of its orbit is about 385 000 km. Find the radius and location of the circular orbit of a geosynchronous satellite (i.e., one that remains above the same point on the Earth's surface).
A) 69 742 km, in the equatorial plane
B) 35 016 km, in the equatorial plane
C) 42 443 km, in the equatorial plane
D) 45 229 km, in a polar plane
E) none of the above
A) 69 742 km, in the equatorial plane
B) 35 016 km, in the equatorial plane
C) 42 443 km, in the equatorial plane
D) 45 229 km, in a polar plane
E) none of the above

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
78
The distance from the moon to the Earth at perigee is about 356 000 km and its distance at apogee is about 406 700 km. Find the eccentricity of the moon's orbit.
A) 0.066
B) 0.058
C) 0.051
D) 0.043
E) none of the above
A) 0.066
B) 0.058
C) 0.051
D) 0.043
E) none of the above

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
79
The angular velocity of a certain comet at perihelion is 10 times its angular velocity at aphelion. Find the eccentricity of the comet's orbit.
A)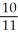
B)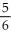
C)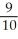
D)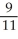
E) none of the above
A)
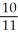
B)
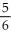
C)
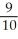
D)
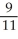
E) none of the above

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck
80
Find the radial and transverse components of the acceleration of a particle moving with constant speed v along the polar curve r = 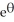 . Express your answer s as functions of .
. Express your answer s as functions of .
A)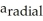 = -
= - 
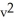
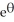 ,
, 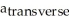 = -
= - 


B)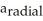 = -
= - 
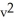
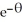 ,
, 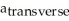 =
= 
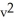
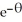
C)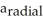 = -
= - 

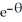 ,
,  =
= 
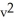
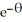
D)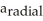 =
= 
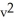
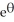 ,
,  =
= 
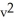
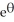
E) none of the above
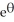 . Express your answer s as functions of .
. Express your answer s as functions of .A)
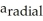 = -
= - 
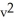
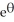 ,
, 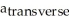 = -
= - 


B)
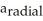 = -
= - 
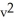
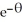 ,
, 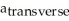 =
= 
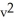
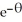
C)
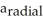 = -
= - 

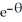 ,
,  =
= 
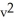
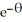
D)
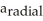 =
= 
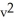
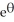 ,
,  =
= 
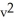
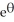
E) none of the above

Unlock Deck
Unlock for access to all 87 flashcards in this deck.
Unlock Deck
k this deck


