Deck 13: Sequences and Series
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/81
Play
Full screen (f)
Deck 13: Sequences and Series
1
Is the following sequence arithmetic, geometric, or neither?
arithmetic
2
Is the following sequence arithmetic, geometric, or neither?
neither
3
Is the following sequence arithmetic, geometric, or neither?
neither
4
For the arithmetic sequence , suppose . If , what is ?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
5
For the arithmetic sequence , suppose . What is ?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
6
For the arithmetic sequence , suppose , and the partial sum . What is ?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
7
A sequence can be defined by a recurrence relation, which gives the first term, , and a formula for finding in terms of the previous term, . For the recurrence relation below, what is ?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
8
A sequence can be defined by a recurrence relation, which gives the first term, , and a formula for finding in terms of the previous term, . For the recurrence relation below, what is the formula for the general term?
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
9
Is an arithmetic sequence?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
10
Consider the sequence , ...involving sums of odd integers.
What is the term of this sequence (i.e. the sum of the first 36 odd numbers)?
What is the term of this sequence (i.e. the sum of the first 36 odd numbers)?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
11
A person decides to walk for 11 minutes one day, and then increases his walks by 2.5 minutes each day for a month. Let be the sequence showing the length of time he walks each day, where is the length of time he walks on day . What is ?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
12
Let be an arithmetic sequence with and . What is ?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
13
Let be an arithmetic sequence with and . What is ?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
14
Let be a geometric sequence with and . What is ?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
15
If denotes your total earnings after weeks of work, is the amount you earned during the week given by ?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
16
Is the sequence arithmetic, geometric, neither, or both?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
17
Is the sequence arithmetic, geometric, neither, or both?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
18
What is the term of the sequence ? The sequence is either arithmetic or geometric.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
19
What is the term of the sequence ? The sequence is either arithmetic or geometric.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
20
What is the term of the sequence ? The sequence is either arithmetic or geometric.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
21
What is the sum of the first 22 positive even integers?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
22
The following table shows the enrollment in a private college for the years 1996 to 2005. Interpret these figures as partial sums, , of terms of a sequence, , where is the number of years since 1995 (so , etc.).
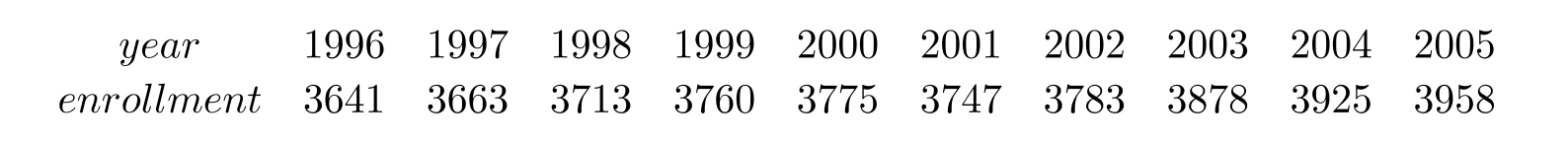
Which of the following gives the change in enrollment between 1999 and 2000 ?
A)
B)
C)
D)
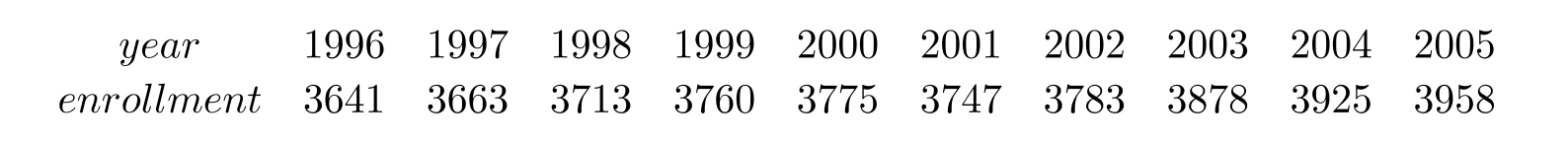
Which of the following gives the change in enrollment between 1999 and 2000 ?
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
23
The following table shows the enrollment in a private college for the years 1996 to 2005. Interpret these figures as partial sums, , of terms of a sequence, , where is the number of years since 1995 (so , etc.).
Which of the following gives the total enrollment in 2004?
A)
B)
C)
D)
Which of the following gives the total enrollment in 2004?
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
24
The following table shows the enrollment in a private college for the years 1996 to 2005. Interpret these figures as partial sums, , of terms of a sequence, , where is the number of years since 1995 (so , etc.).
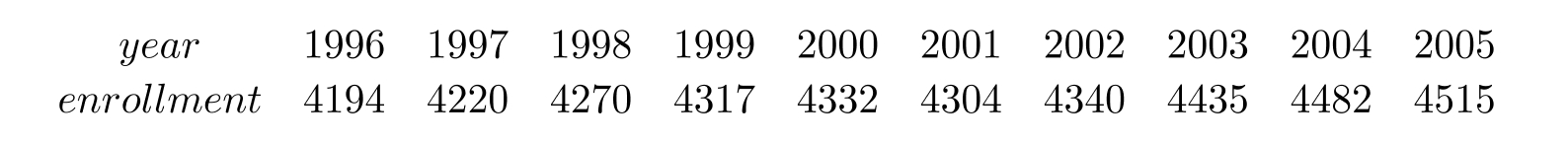
Which of the following gives the average enrollment between 1998 and 2001 ?
A)
B)
C)
D)
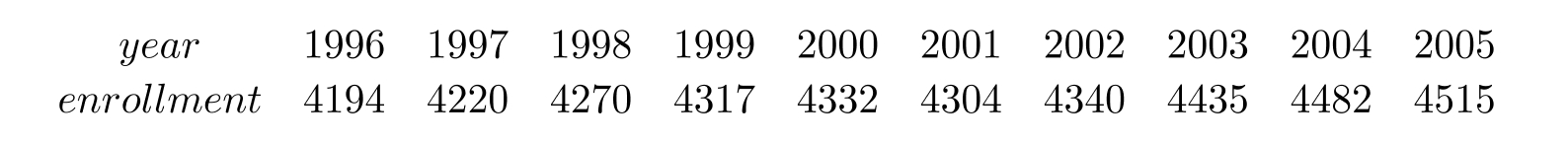
Which of the following gives the average enrollment between 1998 and 2001 ?
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
25
The following table shows the enrollment in a private college for the years 1996 to 2005. Interpret these figures as partial sums, , of terms of a sequence, , where is the number of years since 1995 (so , etc.).
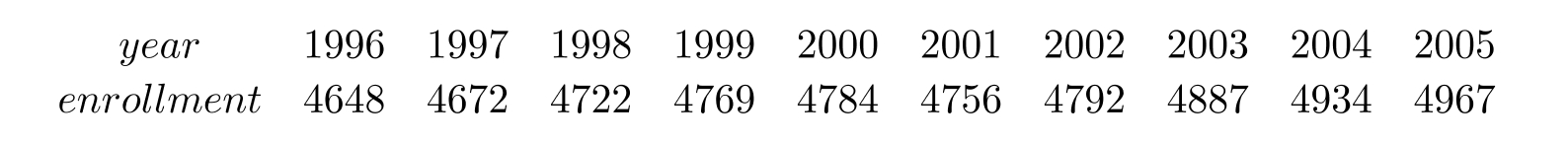
Which of the following gives the average change in enrollment between 2001 and 2005 ?
A)
B)
C)
D)
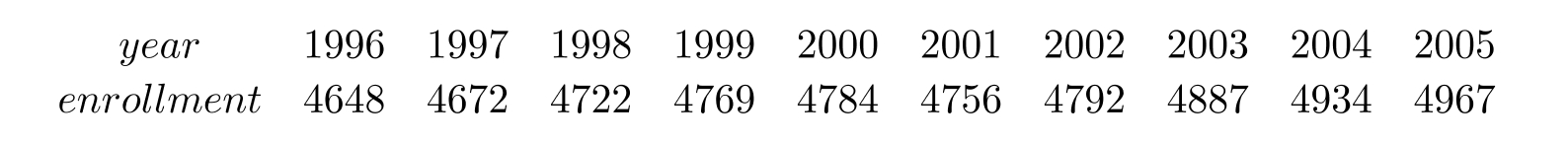
Which of the following gives the average change in enrollment between 2001 and 2005 ?
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
26
A person decides to walk for 18 minutes one day, and then increases his walks by 3 minutes each day for a month. Let be the sequence showing the length of time he walks each day, where is the length of time he walks on day , and let be the sequence of partial sums. What is ?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
27
Does

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
28
If , then , and

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
29
What is

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
30
Does ?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
31
A child building a tower with blocks places 17 blocks in the first row, 16 blocks in the second row, 15 blocks in the third row, and so forth. How many blocks are in the tower if it has 14 rows total?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
32
How many terms are there in the series ?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
33
If denotes your total earnings after weeks of work, what is the average amount of money you earned each week for the first 14 weeks?
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
34
The time it takes an employee to assemble a bicycle is 57 minutes the first time he does it. The second time, it take him 56 minutes; the third time it takes 55 minutes, and so on. If this trend continues, how many minutes will it take him to assemble the bicycle?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
35
The time it takes an employee to assemble a bicycle is 57 minutes the first time he does it. The second time, it take him 56 minutes; the third time it takes 55 minutes, and so on. If this trend continues and there are 11 bicycles to assemble, it should take him a total of ---------- hours and -----------minutes.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
36
Which of the following is the correct expansion of ?
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
37
Evaluate the following sum :

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
38
Find the sum of the first 400 odd integers.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
39
Write using sigma notation.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
40
Write using sigma notation.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
41
If you were to fill out a pedigree chart, the first column would have one name (yours), the second column would have two names (your parents), the third column would have 4 names (your grandparents), etc. How many names would be on the chart if there were 9 columns total?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
42
The repeating decimal 0.757575 . . can be written as an infinite geometric series Use this fact to find a fraction that is equivalent to 0.757575 . ... Your answer should be of the form "A/B".

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
43
If is a geometric series, give its ratio. If not, enter "not geometric"

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
44
Find to 3 decimal places.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
45
If , then , and

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
46
Is the series arithmetic, geometric, or neither?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
47
Is the series arithmetic, geometric, or neither?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
48
Is a geometric series?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
49
Find the sum of the series . Round to 3 decimal places if necessary.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
50
A new employee is offered two salary packages. Both start with an initial salary of $43,000. Package A offers a $2000 increase each year. Package B offers a 4% increase each year. After 11 years, the annual salary with package A would be ---------$ and the annual salary with package B would be$ -----------.Round both answers to the nearest dollar.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
51
Find the sum of the series . Round to 2 decimal places.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
52
Does ?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
53
Evaluate to 3 decimal places.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
54
To save for their child's college education, parents put at the beginning of each month into an account that pays annual interest, compounded monthly. How much will they have saved if they do this for 18 years? Round to the nearest cent.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
55
To save for their child's college education, parents put at the beginning of each month into an account that pays annual interest, compounded monthly. They do this for 18 years. How much money per month would they have had to save if they needed the same total amount in 18 years, but their account only paid annual interest, compunded monthly? Round to the nearest cent.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
56
A patient takes of a pain-killing drug twice a day. Every 12 hours, the patient's body metabolizes of the drug present. How many mg of the drug remain in the patient's body after 8 days (right after the dose)? Round to 2 decimal places.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
57
A patient takes of a pain-killing drug twice a day. Every 12 hours, the patient's body metabolizes of the drug present. If the patient stops taking the drug after 1 week, how many of the drug remain in the patient's body 2 days after that? Round to 2 decimal places.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
58
The population of Arizona rose from 5,130,635 in 2000 to 5,939,292 in 2005.
Assuming a constant percent annual growth rate, what is the population expected to be in 2021? Round to the nearest whole number.
Assuming a constant percent annual growth rate, what is the population expected to be in 2021? Round to the nearest whole number.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
59
The population of Arizona rose from 5,130,635 in 2000 to 5,939,292 in 2005. Assuming a constant percent growth rate, in what year is the population first expected to exceed 12 million?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
60
Does ?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
61
Does ?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
62
What is the present value of a lottery prize of 1 million dollars, paid out at a rate of per year for 50 years? Assume an annual interest rate of . Round to the nearest dollar.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
63
A patient takes of a pain-killing drug twice a day. Every 12 hours, the patient's body metabolizes of the drug present. If the patient continues to take the drug indefinitely, how many of the drug will remain in the patient's body in the long run? Round to 2 decimal places.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
64
Find the sum of the series , for .
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
65
What is ? Round to 2 decimal places.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
66
Subtract the following two equations and solve for :
Assume is not equal to 1 .
A)
B)
C)
D)
Assume is not equal to 1 .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
67
Let , with . As , what happens to ?
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
68
An athlete is offered a signing bonus of million per year for 10 years. If the interest rate is per year, compounded annually, what is the present value of this bonus? Round to the nearest dollar.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
69
A plaintiff is awarded a settlement of per year (paid at the beginning of the year) for the rest of his life. Assuming 3.6\% annual interest, how much money would the defendant have to put aside now in order to ensure that the settlement would be paid (no matter how many years the plaintiff lived)? In other words, what is the present value of the settlement if the plaintiff may live indefinitely? Round to the nearest dollar.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
70
Does the infinite geometric series converge or diverge?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
71
Write as a fraction.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
72
An employee is offered a salary of per year for 6 years. What is the present value of the contract on the date of the first payment if the interest rate is per year, compounded continuously. Round your answer to two decimal places.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
73
Write as a fraction or improper fraction.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
74
What is the present value of a bond which pays each year for 15 years, with the first payment one year from now? Assume the interest rate is 5.2\% per year compounded annually.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
75
What is the present value of a bond which pays each year for 15 years, with the first payment one year from now? Assume the interest rate is 5.5\% per year compounded annually.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
76
Find the sum . Round your answer to 3 decimal places.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
77
Your doctor tells you to take of a certain drug every 12 hours. It is known that only of the drug is still in the body at the end of 12 hours. What quantity of the drug is in the body right after taking the 11th dose? Give your answer correct to 3 decimal places.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
78
Your doctor tells you to take of a certain drug every 12 hours. It is known that only of the drug is still in the body at the end of 12 hours. Assuming you continue to take the drug, what happens to the drug level in the long run? Give your answer correct to 3 decimal places.

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
79
For what values of does the sum converge?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck
80
For what values of does the sum converge?

Unlock Deck
Unlock for access to all 81 flashcards in this deck.
Unlock Deck
k this deck


