Deck 13: One-Way Repeated Measures Anova
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/16
Play
Full screen (f)
Deck 13: One-Way Repeated Measures Anova
1
In a repeated measures ANOVA, the formula for calculating SSTotal is: 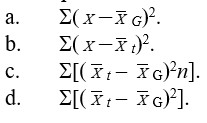
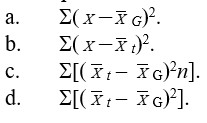
A
2
In a repeated measures ANOVA, the formula for calculating SSBetween is: 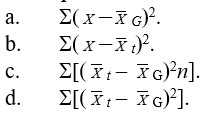
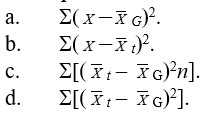
C
3
In a repeated measures ANOVA, the formula for calculating SSError is: 

B
4
In a repeated measures ANOVA, the formula for calculating SSSubjects is: 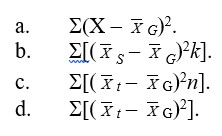
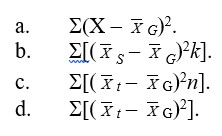

Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck
5
In a repeated measures ANOVA, the formula for calculating dfTotal is:
A) N - 1.
B) N - k.
C) k - 1.
D) N -(k - 1).
A) N - 1.
B) N - k.
C) k - 1.
D) N -(k - 1).

Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck
6
In a repeated measures ANOVA, the formula for calculating dfBetween is:
A) N - 1.
B) N - k.
C) k - 1.
D) N - (k - 1).
A) N - 1.
B) N - k.
C) k - 1.
D) N - (k - 1).

Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck
7
In a repeated measures ANOVA, the formula for calculating dfError is:
A) N - 1.
B) N - k.
C) k - 1.
D) (k - 1)(n -1).
A) N - 1.
B) N - k.
C) k - 1.
D) (k - 1)(n -1).

Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck
8
In a repeated measures ANOVA, the formula for calculating dfSubjects is:
A) n - 1.
B) n - k.
C) k - 1.
D) (k - 1)(n - 1).
A) n - 1.
B) n - k.
C) k - 1.
D) (k - 1)(n - 1).

Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck
9
In a repeated measures ANOVA, to calculate MSBetween, we divide _____ by _____.
A) SSTotal; dfTotal
B) SSBetween; dfBetween
C) SSWithin; dfWithin
D) SSBetween; dfWithin
A) SSTotal; dfTotal
B) SSBetween; dfBetween
C) SSWithin; dfWithin
D) SSBetween; dfWithin

Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck
10
In a repeated measures ANOVA, to calculate MSError, we divide _____ by _____.
A) SSTotal; dfTotal
B) SSBetween; dfBetween
C) SSError; dfError
D) SSBetween; dfWithin
A) SSTotal; dfTotal
B) SSBetween; dfBetween
C) SSError; dfError
D) SSBetween; dfWithin

Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck
11
In a repeated measures ANOVA, the final F-ratio is determined by dividing _____ by _____.
A) MSError; MSBetween
B) MSBetween; MSError
C) MSBetween; dfBetween
D) MSError; dfWithin
A) MSError; MSBetween
B) MSBetween; MSError
C) MSBetween; dfBetween
D) MSError; dfWithin

Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck
12
If a researcher reported an F-ratio with df = 3, 30 for a repeated measures ANOVA, then there were _____ conditions in the experiment and _____ total subjects.
A) 4; 10
B) 4; 11
C) 3; 10
D) 3; 11
A) 4; 10
B) 4; 11
C) 3; 10
D) 3; 11

Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck
13
If a researcher reported an F-ratio with df = 2, 24 for a repeated measures ANOVA, then there were _____ conditions in the experiment and _____ total subjects.
A) 2; 12
B) 2; 25
C) 3; 12
D) 3; 13
A) 2; 12
B) 2; 25
C) 3; 12
D) 3; 13

Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck
14
If a repeated measures ANOVA produced MSbetween = 20 and MSError = 5, then the F-ratio would be:
A) 20/5 = 4.00.
B) 5/20 = .025.
C) 20/25 = 0.80.
D) 25/5 = 5.00.
A) 20/5 = 4.00.
B) 5/20 = .025.
C) 20/25 = 0.80.
D) 25/5 = 5.00.

Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck
15
Calculate Tukey's HSD (honestly significant difference) and eta-squared for the following ANOVA.



Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck
16
The following ANOVA table corresponds to an experiment on pizza preference (0-10) based on the topping (plain, sausage, pepperoni, or everything). The mean preference by groups are as follows:
Plain: 8.5
Sausage: 6.8
Pepperoni: 6.5
Everything: 2.7
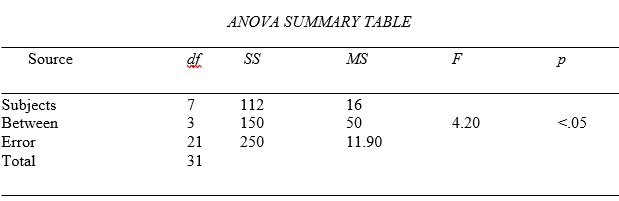 Fill in the table for a within-subjects design with a total of 8 subjects, draw the graph representing the data, calculate eta-squared and Tukey's HSD, and draw conclusions based on the ANOVA table and the means provided.
Fill in the table for a within-subjects design with a total of 8 subjects, draw the graph representing the data, calculate eta-squared and Tukey's HSD, and draw conclusions based on the ANOVA table and the means provided.
Plain: 8.5
Sausage: 6.8
Pepperoni: 6.5
Everything: 2.7
 Fill in the table for a within-subjects design with a total of 8 subjects, draw the graph representing the data, calculate eta-squared and Tukey's HSD, and draw conclusions based on the ANOVA table and the means provided.
Fill in the table for a within-subjects design with a total of 8 subjects, draw the graph representing the data, calculate eta-squared and Tukey's HSD, and draw conclusions based on the ANOVA table and the means provided.
Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck


