Deck 14: Multivariate Calculus
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/152
Play
Full screen (f)
Deck 14: Multivariate Calculus
1
Evaluate the function.
-Find when .
A) -3
B) -4
C) 5
D) 13
-Find when .
A) -3
B) -4
C) 5
D) 13
5
2
Evaluate the function.
-Find when .
A) 85
B) 88
C) 60
D) 56
-Find when .
A) 85
B) 88
C) 60
D) 56
60
3
Evaluate the function.
-Find when .
A) 9
B) 10
C)
D)
-Find when .
A) 9
B) 10
C)
D)
4
Evaluate the function.
-Find when .
A)
B)
C)
D)
-Find when .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
5
Evaluate the function.
-Find when .
A) 30
B) 370
C) 100
D) 1000
-Find when .
A) 30
B) 370
C) 100
D) 1000

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
6
Evaluate the function.
-Find when .
A)
B)
C)
D)
-Find when .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
7
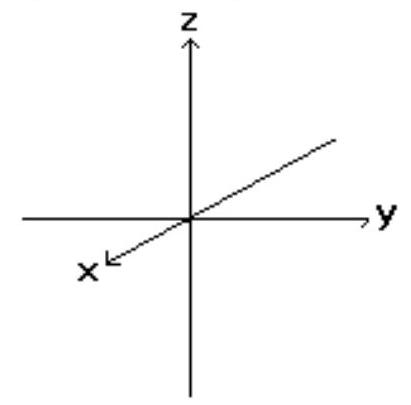
Sketch the indicated plane.
-
A)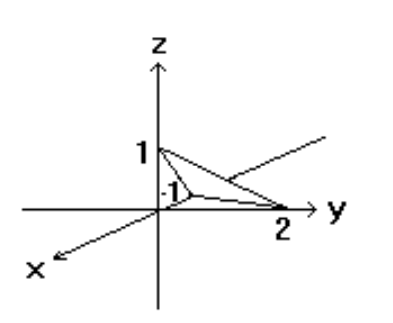
B)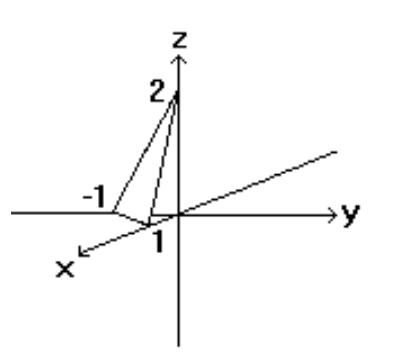
C)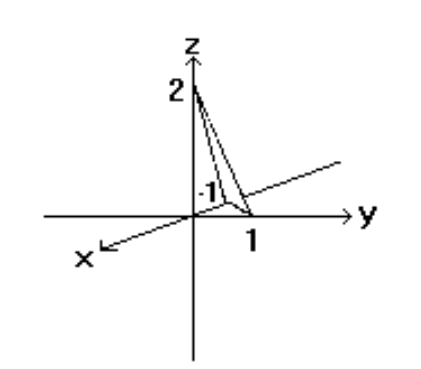
D)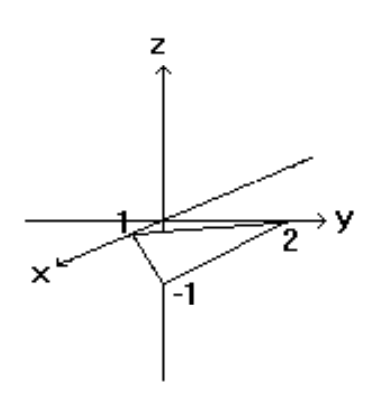
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
8
Sketch the indicated plane.
-

A)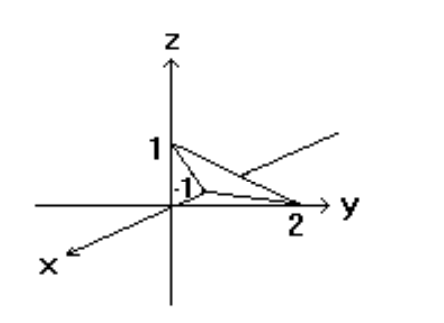
B)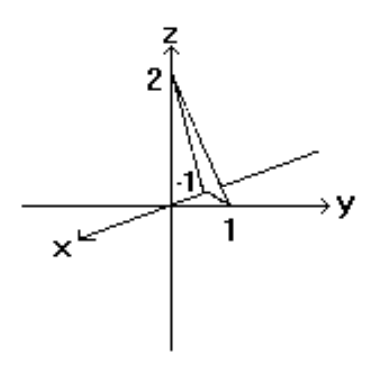
C)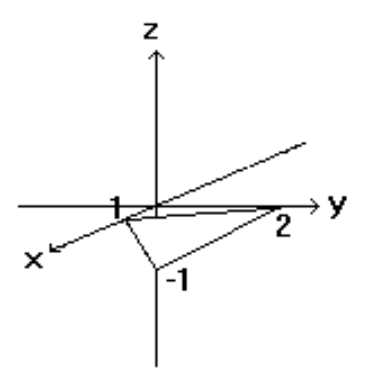
D)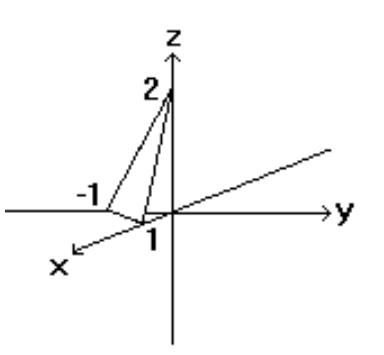
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
9
Sketch the indicated plane.
-
A)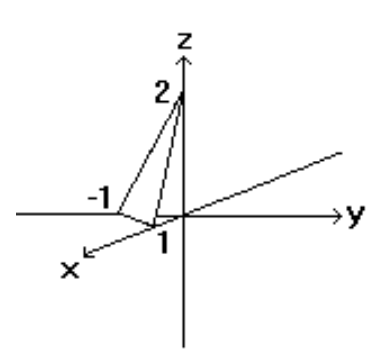
B)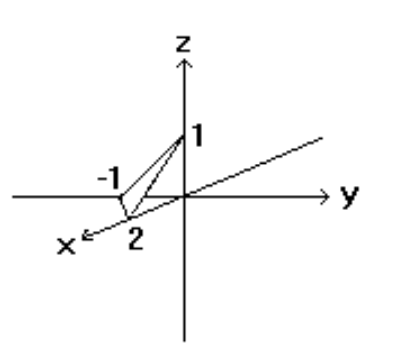
C)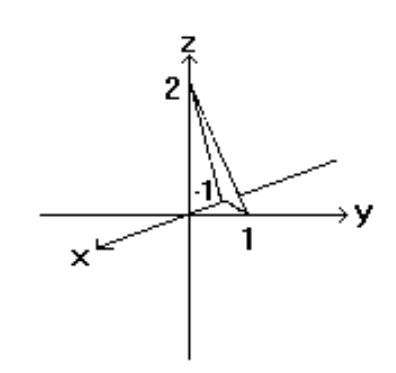
D)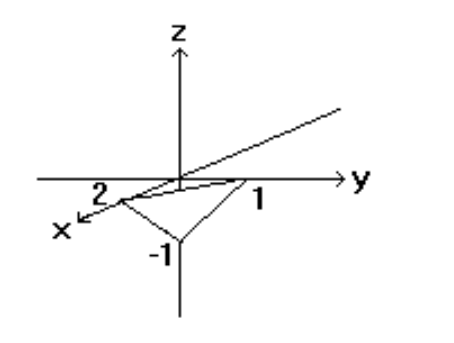
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
10
Sketch the indicated plane.
-

A)
B)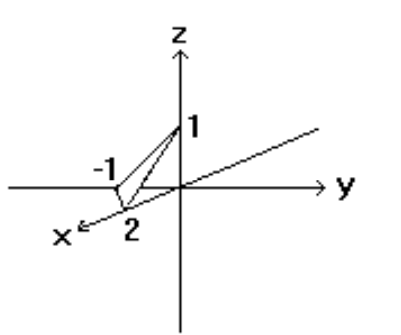
C)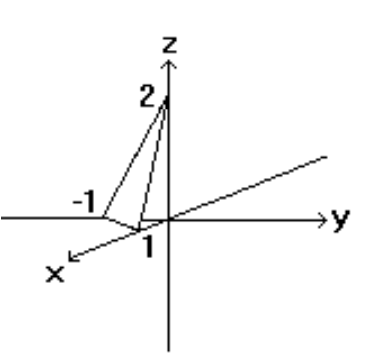
D)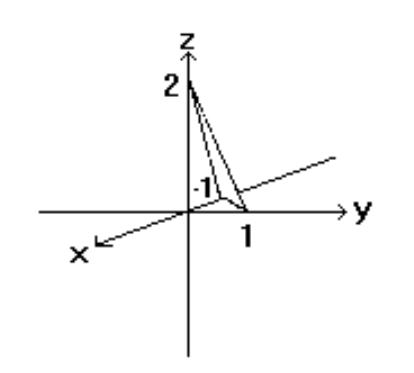
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
11
Sketch the indicated plane.
-
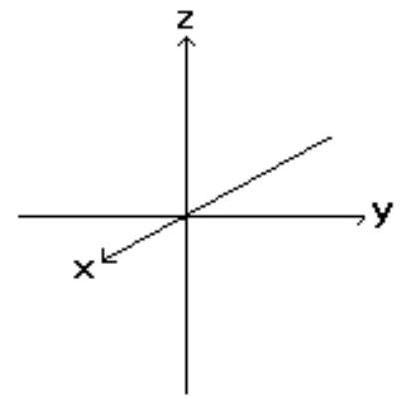
A)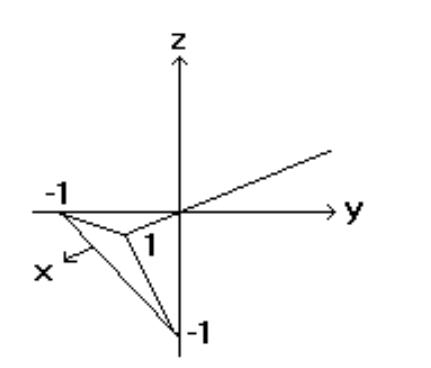
B)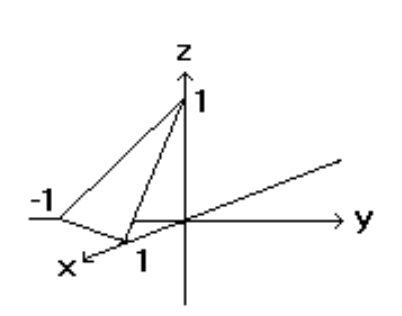
C)
D)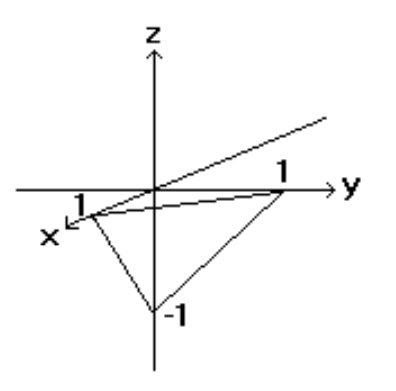
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
12
Sketch the indicated plane.
-

A)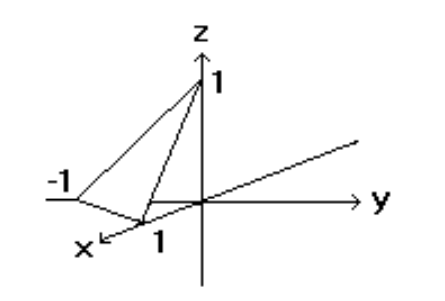
B)
C)
D)
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
13
Sketch the indicated plane.
-
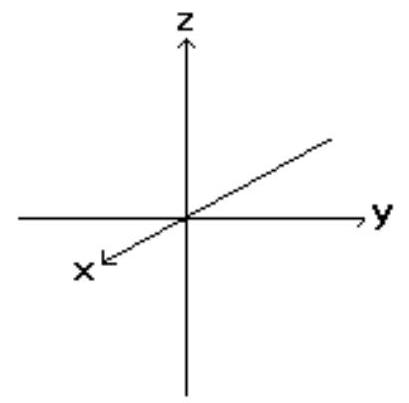
A)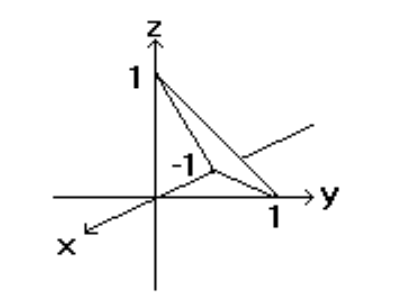
B)
C)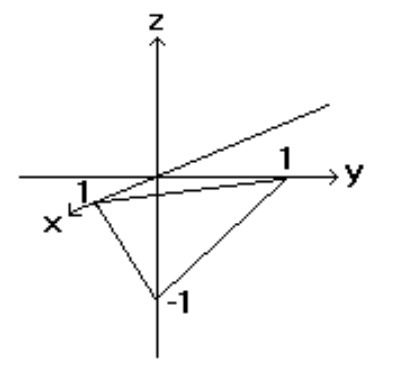
D)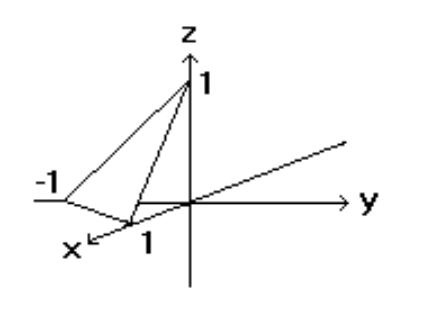
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
14
Sketch the indicated plane.
-
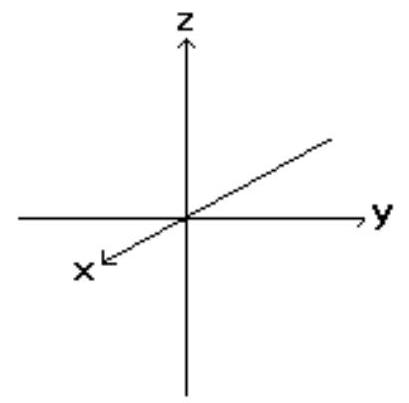
A)
B)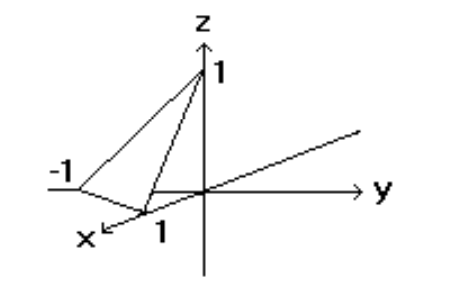
C)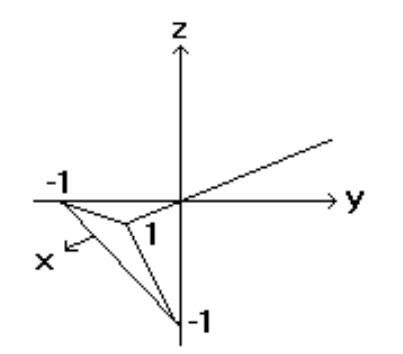
D)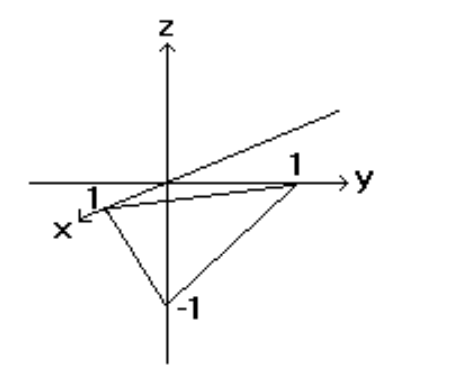
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
15
Graph the level curves in the first octant at heights of , and for the given equation.
-
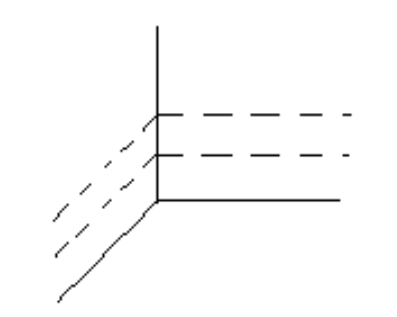
A)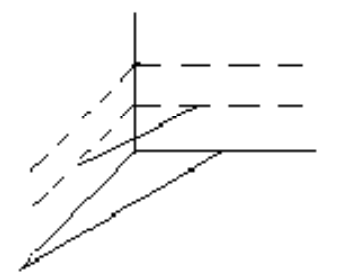
B)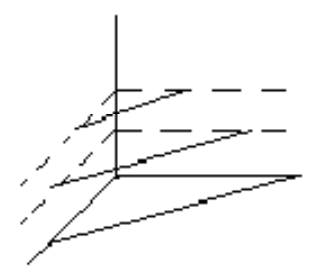
C)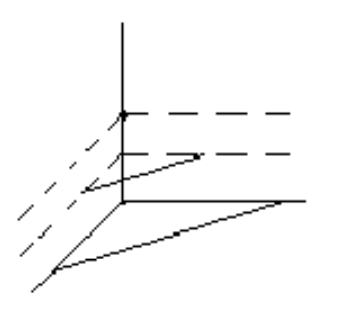
D)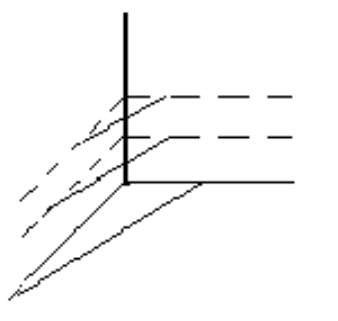
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
16
Graph the level curves in the first octant at heights of , and for the given equation.
-
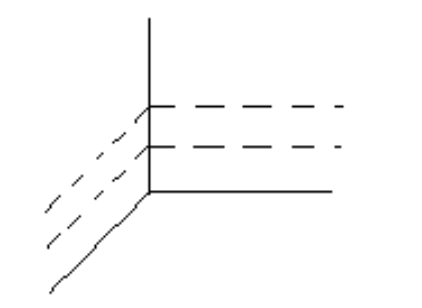
A)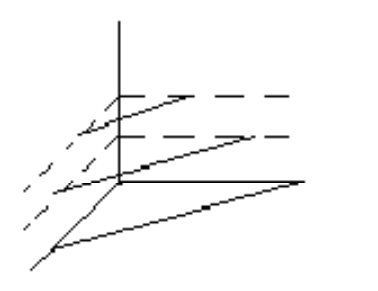
B)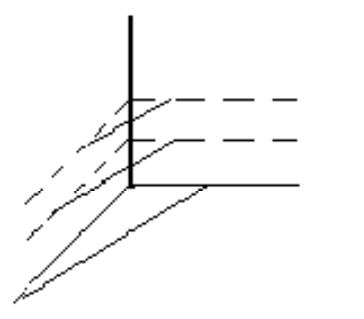
C)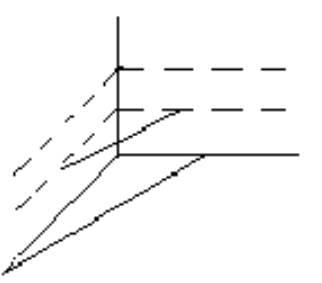
D)
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
17
Graph the level curves in the first octant at heights of , and for the given equation.
-
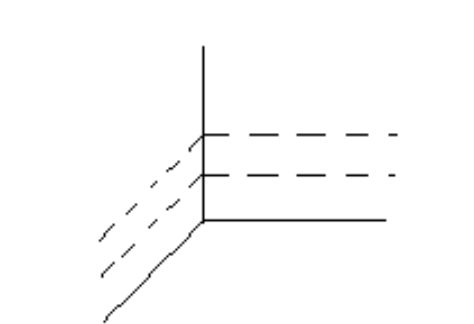
A)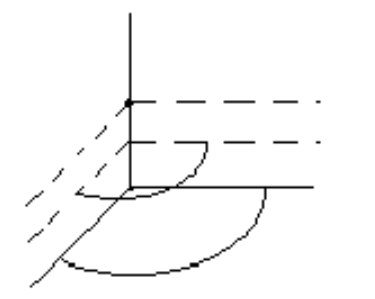
B)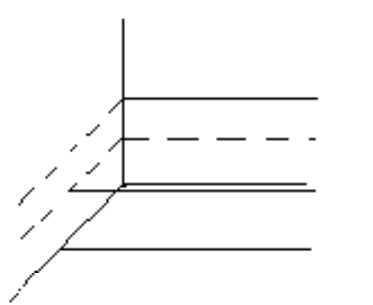
C)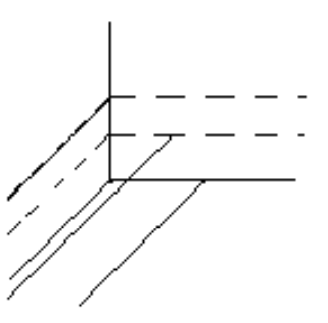
D)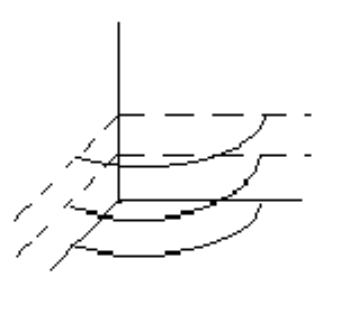
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
18
Graph the level curves in the first octant at heights of , and for the given equation.
-
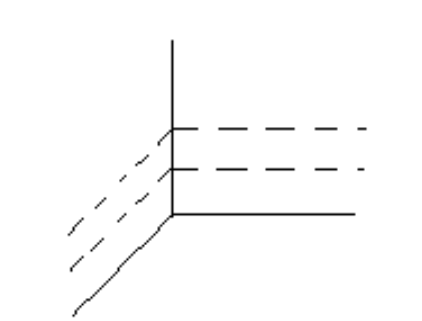
A)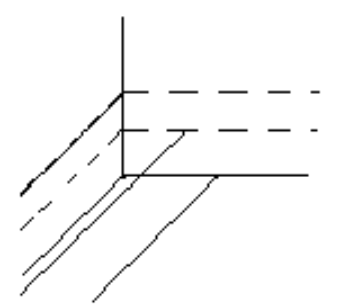
B)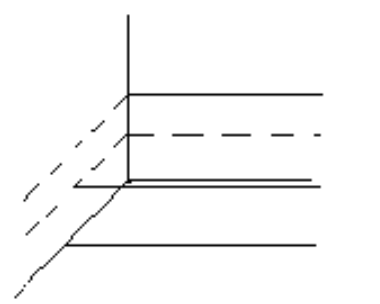
C)
D)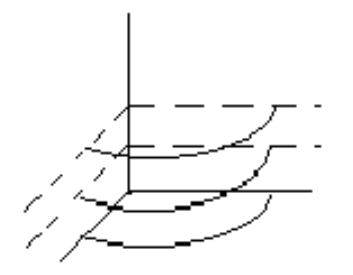
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
19
Graph the level curves in the first octant at heights of , and for the given equation.
-
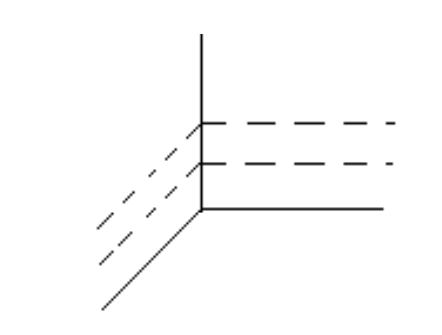
A)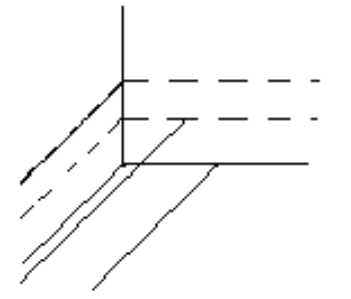
B)
C)
D)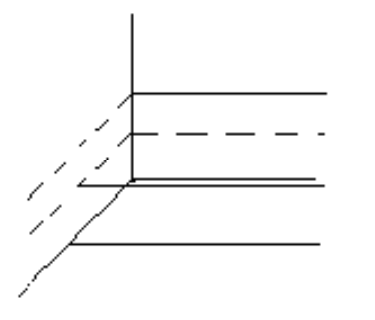
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
20
Graph the level curves in the first octant at heights of , and for the given equation.
-

A)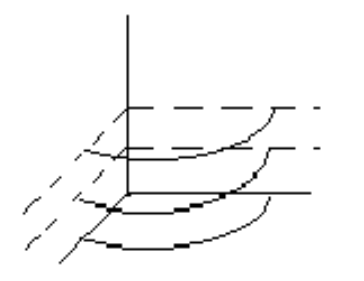
B)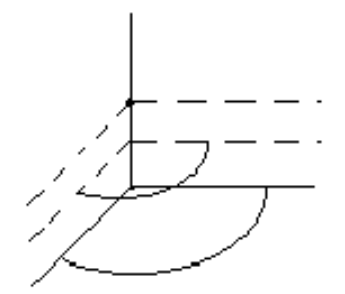
C)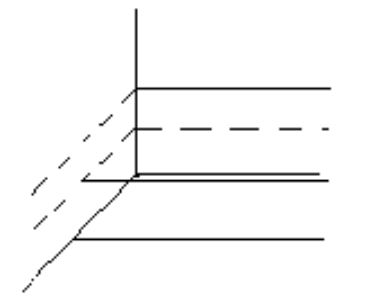
D)
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
21
Graph the level curves in the first octant at heights of , and for the given equation.
-
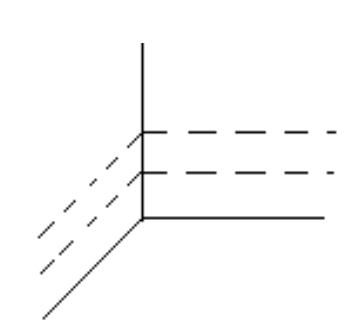
A)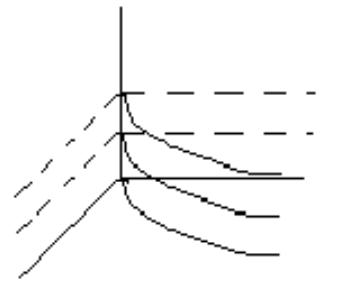
B)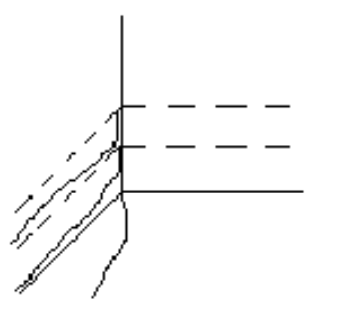
C)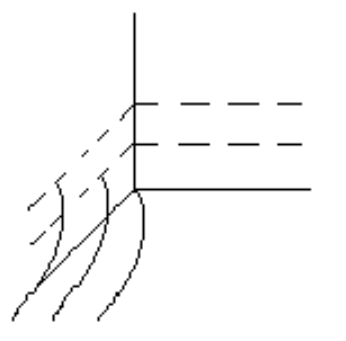
D)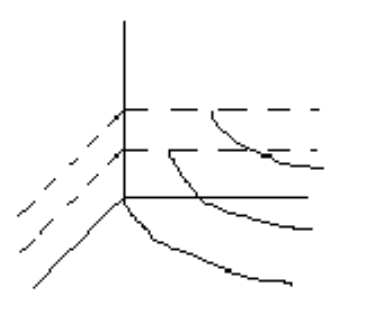
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
22
Graph the level curves in the first octant at heights of , and for the given equation.
-

A)
B)
C)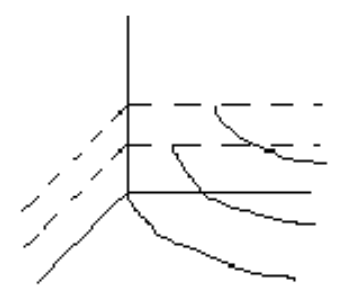
D)
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
23
Solve the problem.
-The following table shows the number (in thousands) of albums and t-shirts sold for three different months in the same year.
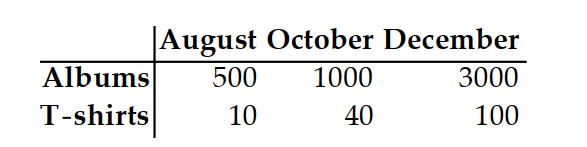
Model the number of -shirts sold as a function of the album sales and time using a linear function of the form constants) where represents thousands of -shirts sold to date, a represents thousands of albums sold to date, and represents time in months since April of that year.
A)
B)
C)
D)
-The following table shows the number (in thousands) of albums and t-shirts sold for three different months in the same year.
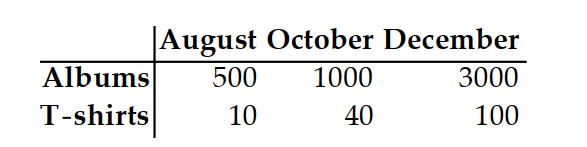
Model the number of -shirts sold as a function of the album sales and time using a linear function of the form constants) where represents thousands of -shirts sold to date, a represents thousands of albums sold to date, and represents time in months since April of that year.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
24
Solve the problem.
-Production of television sets is given by , where is work hours and is the amount of capital. If 64 work hours and 125 units of capital are used, what is the production output?
A) 456,356
B) 4630
C) 4564
D) 463,030
-Production of television sets is given by , where is work hours and is the amount of capital. If 64 work hours and 125 units of capital are used, what is the production output?
A) 456,356
B) 4630
C) 4564
D) 463,030

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
25
Solve the problem.
-The number of cows that can graze on a ranch is approximated by , where is the number of acres of grass and y the number of acres of alfalfa. If the ranch has 25 acres of alfalfa and 70 acres of grass, how many cows may graze?
A) 755 cows
B) 751 cows
C) 575 cows
D) 571 cows
-The number of cows that can graze on a ranch is approximated by , where is the number of acres of grass and y the number of acres of alfalfa. If the ranch has 25 acres of alfalfa and 70 acres of grass, how many cows may graze?
A) 755 cows
B) 751 cows
C) 575 cows
D) 571 cows

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
26
Solve the problem.
-The surface area of a human body (in square meters) is approximated by , where is the weight of the person in kilograms and is the height in meters. Find if and .
A)
B)
C)
D)
-The surface area of a human body (in square meters) is approximated by , where is the weight of the person in kilograms and is the height in meters. Find if and .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
27
Choose the graph of the equation.
-
A)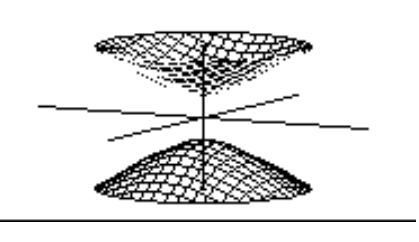
B)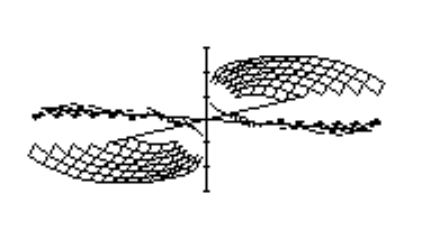
C)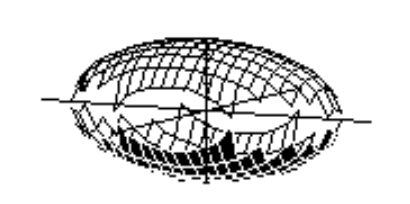
D)
-
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
28
Choose the graph of the equation.
-
A)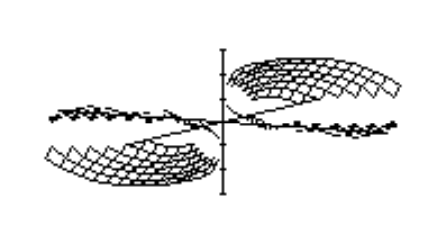
B)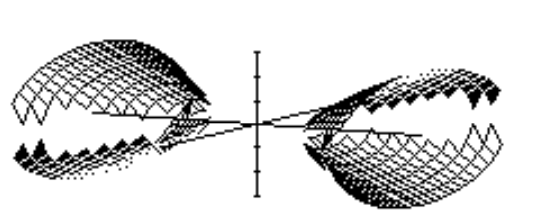
C)
D)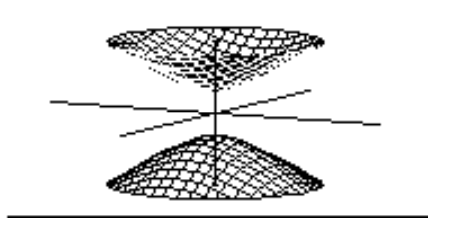
-
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
29
Choose the graph of the equation.
-
A)
B)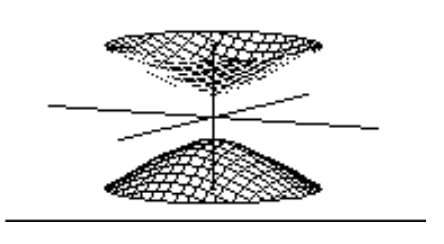
C)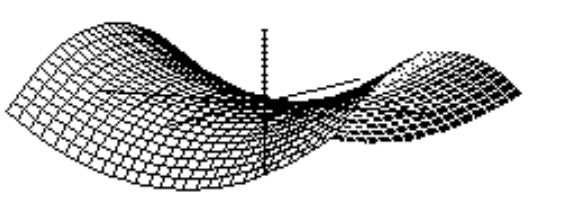
D)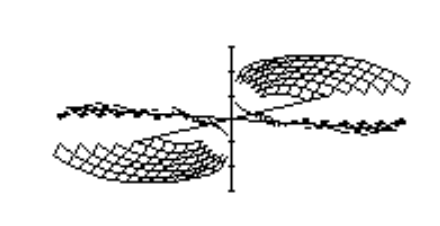
-
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
30
Choose the graph of the equation.
-
A)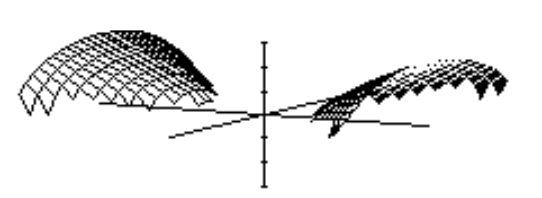
B)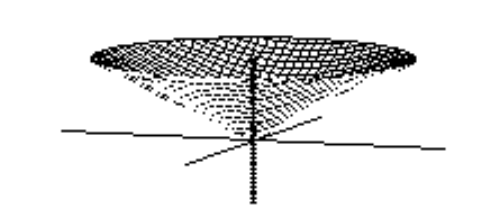
C)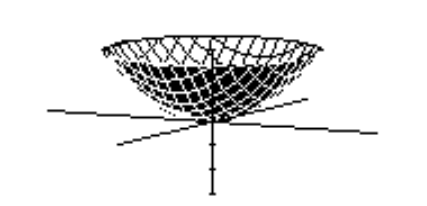
D)
-
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
31
Choose the graph of the equation.
-
A)
B)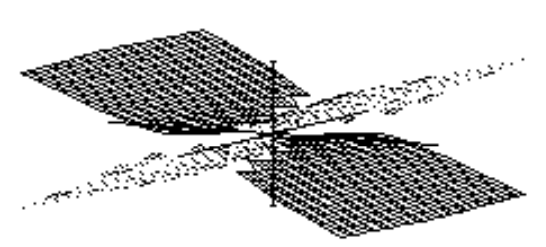
C)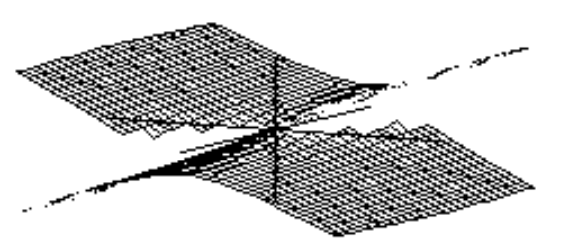
D)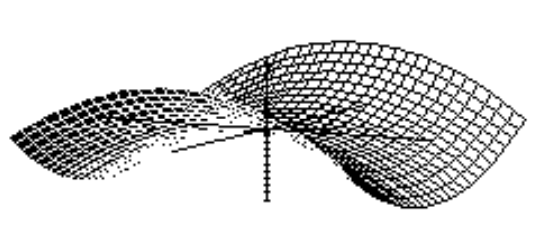
-
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
32
Choose the graph of the equation.
-
A)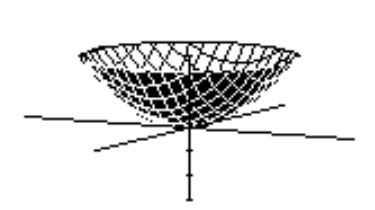
B)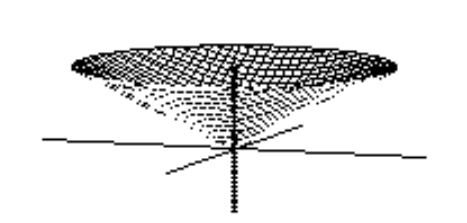
C)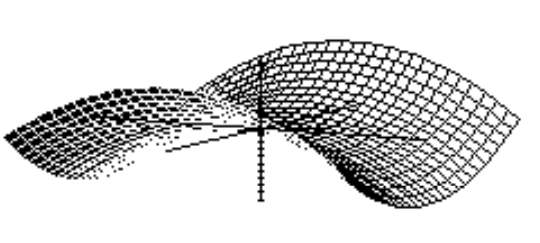
D)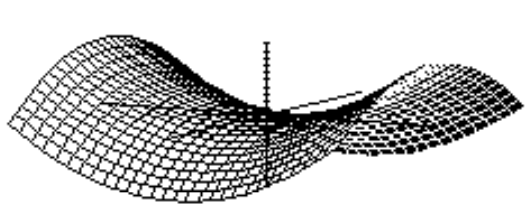
-
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
33
Choose the graph of the equation.
-
A)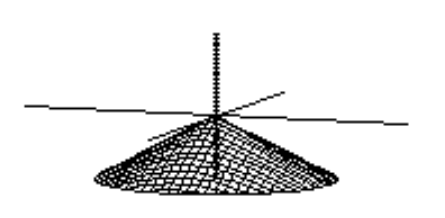
B)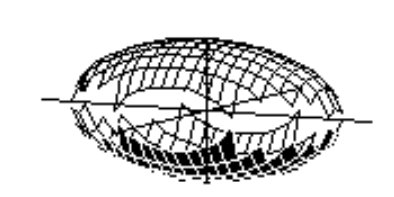
C)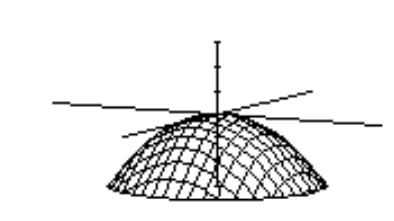
D)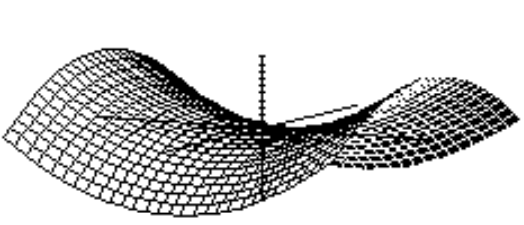
-
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
34
Choose the graph of the equation.
-
A)
B)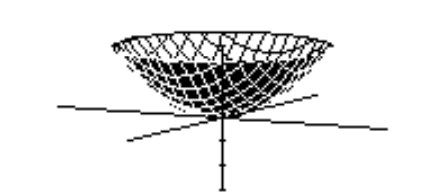
C)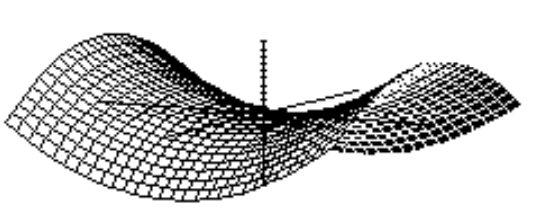
D)
-
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
35
Choose the graph of the equation.
-
A)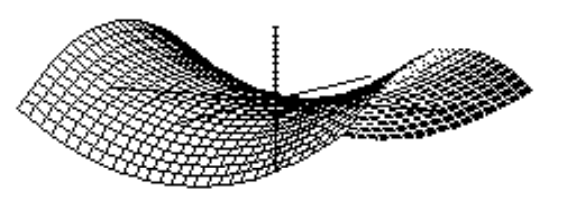
B)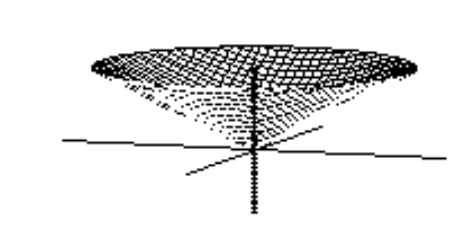
C)
D)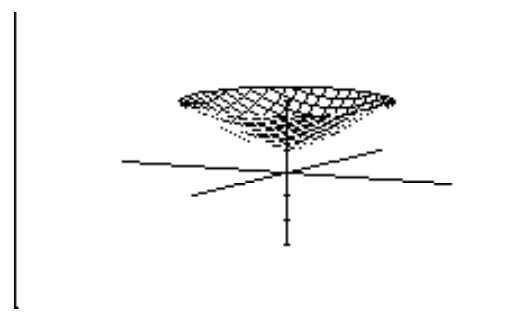
-
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
36
Choose the graph of the equation.
-
A)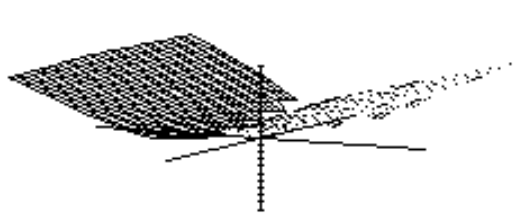
B)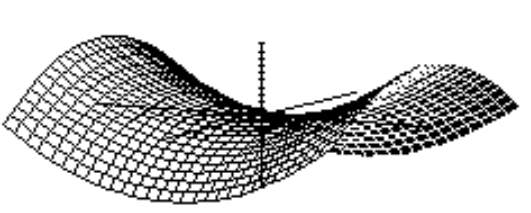
C)
D)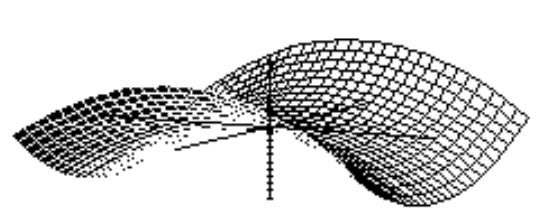
-
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
37
Find the partial derivative.
-Let . Find .
A)
B)
C)
D)
-Let . Find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
38
Find the partial derivative.
-Let . Find .
A)
B)
C)
D)
-Let . Find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
39
Find the partial derivative.
-Let . Find .
A)
B)
C)
D)
-Let . Find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
40
Find the partial derivative.
-Let . Find .
A)
B)
C)
D)
-Let . Find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
41
Find the partial derivative.
-Find when .
A) 45
B) -45
C) -162
D) 207
-Find when .
A) 45
B) -45
C) -162
D) 207

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
42
Find the partial derivative.
-Find when .
A) 129
B) -54
C) -45
D) 39
-Find when .
A) 129
B) -54
C) -45
D) 39

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
43
Find the partial derivative.
-Find when .
A)
B)
C)
D)
-Find when .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
44
Find the partial derivative.
-Find when .
A)
B)
C)
D)
-Find when .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
45
Find the partial derivative.
-Find when .
A)
B)
C)
D)
-Find when .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
46
Find the partial derivative.
-Find when .
A)
B)
C)
D)
-Find when .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
47
Find the second-order partial derivative.
-Find when .
A) -14
B)
C) -28
D)
-Find when .
A) -14
B)
C) -28
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
48
Find the second-order partial derivative.
-Find when .
A)
B)
C) -28
D) -14
-Find when .
A)
B)
C) -28
D) -14

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
49
Find the second-order partial derivative.
-Find when .
A)
B) -28
C) -14
D)
-Find when .
A)
B) -28
C) -14
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
50
Find the second-order partial derivative.
-Find when .
A) 48xy
B) -28
C) -14
D)
-Find when .
A) 48xy
B) -28
C) -14
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
51
Find the second-order partial derivative.
-Find gyy when .
A)
B)
C)
D)
-Find gyy when .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
52
Find the second-order partial derivative.
-Find when .
A)
B)
C)
D)
-Find when .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
53
Find the second-order partial derivative.
-Find when .
A)
B)
C)
D)
-Find when .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
54
Find the second-order partial derivative.
-Find when .
A)
B)
C)
D)
-Find when .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
55
Find the second-order partial derivative.
-Find when .
A)
B)
C)
D)
-Find when .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
56
Find the second-order partial derivative.
-Find when .
A)
B)
C)
D)
-Find when .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
57
Evaluate the required second order partial derivative.
-Find , if .
A) 542
B) -633
C) 1464
D) -825
-Find , if .
A) 542
B) -633
C) 1464
D) -825

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
58
Evaluate the required second order partial derivative.
-Find , if .
A) -96
B) 752
C) -192
D) -288
-Find , if .
A) -96
B) 752
C) -192
D) -288

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
59
Evaluate the required second order partial derivative.
-Find , if .
A)
B)
C)
D)
-Find , if .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
60
Evaluate the required second order partial derivative.
-Find , if .
A)
B)
C)
D)
-Find , if .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
61
Evaluate the required second order partial derivative.
-Find , if .
A) -0.404
B) -1.819
C) 0
D) -0.067
-Find , if .
A) -0.404
B) -1.819
C) 0
D) -0.067

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
62
Evaluate the required second order partial derivative.
-Find , if .
A)
B)
C)
D)
-Find , if .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
63
Evaluate the required second order partial derivative.
-Find , if .
A) -0.032
B) -0.011
C) 0.179
D) -0.043
-Find , if .
A) -0.032
B) -0.011
C) 0.179
D) -0.043

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
64
Evaluate the required second order partial derivative.
-Find , if .
A) -0.132
B) 0.364
C) -0.207
D) -0.074
-Find , if .
A) -0.132
B) 0.364
C) -0.207
D) -0.074

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
65
Evaluate the required second order partial derivative.
-Find , if .
A) 7.5
B) -2.5
C) -1.25
D) -1
-Find , if .
A) 7.5
B) -2.5
C) -1.25
D) -1

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
66
Evaluate the required second order partial derivative.
-Find , if .
A) 0.063
B) 3.75
C) 0.25
D) 0.5
-Find , if .
A) 0.063
B) 3.75
C) 0.25
D) 0.5

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
67
Find values of and such that both and .
-
A)
B)
C)
D)
-
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
68
Find values of and such that both and .
-
A)
B)
C)
D)
-
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
69
Find values of and such that both and .
-
A)
B)
C)
D)
-
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
70
Find values of and such that both and .
-
A)
B)
C)
D)
-
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
71
Find values of and such that both and .
-
A)
B)
C)
D)
-
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
72
Find values of and such that both and .
-
A)
B)
C)
D)
-
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
73
Find values of and such that both and .
-
A)
B)
C)
D)
-
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
74
Find values of and such that both and .
-
A)
B)
C)
D)
-
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
75
Find values of and such that both and .
-
A)
B)
C)
D)
-
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
76
Find values of and such that both and .
-
A)
B)
C)
D)
-
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
77
Find the indicated derivative.
-Find
A)
B)
C)
D)
-Find
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
78
Find the indicated derivative.
-Find
A)
B)
C)
D)
-Find
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
79
Find the indicated derivative.
-Find
A)
B)
C)
D)
-Find
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck
80
Find the indicated derivative.
-Find
A)
B)
C)
D)
-Find
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 152 flashcards in this deck.
Unlock Deck
k this deck


