Deck 10: Confidence Intervals
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/82
Play
Full screen (f)
Deck 10: Confidence Intervals
1
You are thinking of using a t table to find a 95 percent confidence interval for the mean μ of a population. The distribution of the population is normal and the population standard deviation is unknown. A random sample of size n is drawn from this population. You may use the t distribution only if the sample size n is small.
False
2
If the population is normal and its standard deviation σ is known, but the sample size is small, z distribution values may be used to determine interval estimates for the population mean.
False
3
If the population is normal and its standard deviation σ is known, and the sample size n is large (n ≥ 30), interval estimates for the population mean must be determined using z values.
True
4
In determining the interval estimates for a population proportion using the sample proportion, it is appropriate to use the z distribution.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
5
Given the error we are willing to tolerate, the sample size is determined by the mean µ of the population and the confidence level.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
6
Brian Vanecek, VP of Operations at Portland Trust Bank, is evaluating the service level provided to walk-in customers. Accordingly, his staff recorded the waiting times for 45 randomly selected walk-in customers, and calculated that their mean waiting time was 15 minutes. If Brian concludes that the average waiting time for all walk-in customers is 15 minutes, he is using a ___.
A) a range estimate
B) a statistical parameter
C) an interval estimate
D) a point estimate
E) an exact estimate
A) a range estimate
B) a statistical parameter
C) an interval estimate
D) a point estimate
E) an exact estimate

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
7
A market researcher computed a confidence interval for a population proportion using a 95% confidence level. Her boss decided that she wanted a 99% confidence level instead. The new interval with 99% confidence level will be wider than the original one with a 95% confidence level.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
8
If the degrees of freedom in a t distribution increase, difference between the t values and the z values will also increase.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
9
Suppose a random sample of 16 is selected from a population with a normal distribution with a known population standard deviation σ of 10. Assume that the sample mean is 4.2. Based on a 90% confidence interval for the population mean, we can conclude that 0.1 is a plausible number for the population mean μ.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
10
If the population is normal but its standard deviation σ is known, and the sample size n is large (n ≥ 30), z distribution values may be used to determine interval estimates for the population mean.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
11
To construct a confidence interval we first choose
A) an interval of interest.
B) a confidence level.
C) a confidence coefficient.
D) minimum and maximum values.
E) an exact estimate.
A) an interval of interest.
B) a confidence level.
C) a confidence coefficient.
D) minimum and maximum values.
E) an exact estimate.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
12
An assumption underlying the use of t statistic in sample-based estimation is that the population is normally distributed.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
13
Catherine Chao, Director of Marketing Research, is evaluating consumer acceptance of a new toothpaste package. Her staff reports that 17% of a random sample of 200 households prefers the new package to all other package designs. If Catherine concludes that 17% of all households prefer the new package, she is using ___.
A) a point estimate
B) a range estimate
C) a statistical parameter
D) an interval estimate
E) an exact estimate
A) a point estimate
B) a range estimate
C) a statistical parameter
D) an interval estimate
E) an exact estimate

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
14
A t distribution is similar to a normal distribution, but with flatter tails.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
15
When the population standard deviation σ is unknown. the sample standard deviation s is used in determining the interval estimate for the population mean.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
16
In estimating the sample size necessary to estimate a population mean, the error of estimation, E, is equal to the difference between the sample mean and the sample standard deviation.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
17
When a range of values is used to estimate a population parameter, it is called a range estimate.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
18
When a statistic calculated from sample data is used to estimate a population parameter, it is called a point estimate.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
19
Eugene Gates, Marketing Director of Mansfield Motors Manufacturers, Inc.'s Electrical Division, is leading a study to assess the relative importance of product features. An item on a survey questionnaire distributed to 100 of Mansfield's customers asked them to rate the importance of "ease of maintenance" on a scale of 1 to 10 (with 1 meaning "not important" and 10 meaning "highly important"). His staff assembled the following statistics:  If Eugene concludes that the average rate of "ease of maintenance" for all customers is 7.5, he is using ___.
If Eugene concludes that the average rate of "ease of maintenance" for all customers is 7.5, he is using ___.
A) a range estimate
B) a statistical parameter
C) a point estimate
D) an interval estimate
E) a guesstimate
 If Eugene concludes that the average rate of "ease of maintenance" for all customers is 7.5, he is using ___.
If Eugene concludes that the average rate of "ease of maintenance" for all customers is 7.5, he is using ___.A) a range estimate
B) a statistical parameter
C) a point estimate
D) an interval estimate
E) a guesstimate

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
20
In order to find values in the t distribution table, you must determine the appropriate degrees of freedom based on the sample sizes.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
21
James Desreumaux, VP of Human Resources of American First Banks (AFB), is reviewing the employee training programs of AFB banks. His staff randomly selected personnel files for 100 tellers in the Southeast Region and determined that their mean training time was 25 hours. Assume that the population standard deviation is 5 hours. The 92% confidence interval for the population mean of training times is ___.
A) 16.25 to 33.75
B) 24.30 to 25.71
C) 17.95 to 32.05
D) 24.12 to 25.88
E) 24.45 to 27.32
A) 16.25 to 33.75
B) 24.30 to 25.71
C) 17.95 to 32.05
D) 24.12 to 25.88
E) 24.45 to 27.32

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
22
The employees of Cybertronics Inc. need to complete a certification online. A random sample of 49 employees gives an average time for completion of all the coursework and passing the tests of 6 hours. Assume that the population standard deviation is 6 hours and the population of employees is fairly large. Construct a 95% confidence interval for the average time required to complete the certification.
A) 18.28 to 21.72
B) 18.32 to 21.68
C) 18.36 to 21.64
D) 18.40 to 21.60
E) 19.76 to 20.24
A) 18.28 to 21.72
B) 18.32 to 21.68
C) 18.36 to 21.64
D) 18.40 to 21.60
E) 19.76 to 20.24

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
23
The table t value associated with the upper 5% of the t distribution and 12 degrees of freedom is ___.
A) 2.179
B) 1.782
C) 1.356
D) 3.055
E) 3.330
A) 2.179
B) 1.782
C) 1.356
D) 3.055
E) 3.330

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
24
The z value associated with a two?sided 90% confidence interval is ___.
A) 1.28
B) 1.645
C) 1.96
D) 2.575
E) 2.33
A) 1.28
B) 1.645
C) 1.96
D) 2.575
E) 2.33

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
25
The z value associated with a two?sided 88% confidence interval is ___.
A) 1.28
B) 1.55
C) 1.17
D) 0.88
E) 1.90
A) 1.28
B) 1.55
C) 1.17
D) 0.88
E) 1.90

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
26
A researcher is interested in estimating the mean weight of a semi-tracker truck to determine the potential load capacity. She takes a random sample of 17 trucks and computes a sample mean of 20,000 pounds with sample standard deviation of 1,500. The 95% confidence interval for the population mean weight of a semi-tracker truck is ___.
A) 19,232 to 20,768
B) 19,365 to 20,635
C) 19,229 to 20,771
D) 19,367 to 20,633
E) 119.89 to 122.12
A) 19,232 to 20,768
B) 19,365 to 20,635
C) 19,229 to 20,771
D) 19,367 to 20,633
E) 119.89 to 122.12

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
27
James Desreumaux, VP of Human Resources of American First Banks (AFB), is reviewing the employee training programs of AFB banks. His staff randomly selected personnel files for 100 tellers in the Southeast Region and determined that their mean training time was 25 hours. Assume that the population standard deviation is 5 hours. The 88% confidence interval for the population mean of training times is ___.
A) 17.25 to 32.75
B) 24.23 to 25.78
C) 24.42 to 25.59
D) 19.15 to 30.85
E) 21.00 t0 32.00
A) 17.25 to 32.75
B) 24.23 to 25.78
C) 24.42 to 25.59
D) 19.15 to 30.85
E) 21.00 t0 32.00

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
28
Brian Vanecek, VP of Operations at Portland Trust Bank, is evaluating the service level provided to walk-in customers. Accordingly, his staff recorded the waiting times for 64 randomly selected walk-in customers and determined that their mean waiting time was 15 minutes. Assume that the population standard deviation is 4 minutes. The 90% confidence interval for the population mean of waiting times is ___.
A) 14.27 to 15.73
B) 14.18 to 15.82
C) 9.88 to 20.12
D) 13.86 to 16.14
E) 18.12 to 19.87
A) 14.27 to 15.73
B) 14.18 to 15.82
C) 9.88 to 20.12
D) 13.86 to 16.14
E) 18.12 to 19.87

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
29
The normal distribution is used to test about a population mean for large samples if the population standard deviation is known. "Large" is usually defined as ___.
A) at least 10
B) at least 5% of the population size
C) at least 30
D) at least 12
E) at least 100
A) at least 10
B) at least 5% of the population size
C) at least 30
D) at least 12
E) at least 100

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
30
The z value associated with a two-sided 95% confidence interval is ___.
A) 1.28
B) 1.645
C) 1.96
D) 2.575
E) 2.33
A) 1.28
B) 1.645
C) 1.96
D) 2.575
E) 2.33

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
31
If the standard deviation 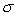 is known, the z distribution values may not be used to determine interval estimates for the population mean when
is known, the z distribution values may not be used to determine interval estimates for the population mean when
A) n < 30.
B) the distribution is not normal.
C) the distribution is skewed.
D) n is big.
E) n is small (<30) and the distribution is not normal.
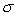 is known, the z distribution values may not be used to determine interval estimates for the population mean when
is known, the z distribution values may not be used to determine interval estimates for the population mean whenA) n < 30.
B) the distribution is not normal.
C) the distribution is skewed.
D) n is big.
E) n is small (<30) and the distribution is not normal.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
32
A random sample of 64 items is selected from a population of 400 items. The sample mean is 200. The population standard deviation is 48. From this data, a 90% confidence interval to estimate the population mean can be computed as ___.
A) 189.21 to 210.79
B) 188.24 to 211.76
C) 190.13 to 209.87
D) 190.94 to 209.06
E) 193.45 to 211.09
A) 189.21 to 210.79
B) 188.24 to 211.76
C) 190.13 to 209.87
D) 190.94 to 209.06
E) 193.45 to 211.09

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
33
A random sample of 64 items is selected from a population of 400 items. The sample mean is 200. The population standard deviation is 48. From this data, a 95% confidence interval to estimate the population mean can be computed as ___.
A) 189.21 to 210.79
B) 188.24 to 211.76
C) 190.13 to 209.87
D) 190.94 to 209.06
E) 193.45 to 211.09
A) 189.21 to 210.79
B) 188.24 to 211.76
C) 190.13 to 209.87
D) 190.94 to 209.06
E) 193.45 to 211.09

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
34
James Desreumaux, VP of Human Resources of American First Banks (AFB), is reviewing the employee training programs of AFB banks. His staff randomly selected personnel files for 100 tellers in the Southeast Region and determined that their mean training time was 25 hours. Assume that the population standard deviation is 5 hours. The 95% confidence interval for the population mean of training times is ___.
A) 15.20 to 34.80
B) 24.18 to 25.82
C) 24.02 to 25.98
D) 16.78 to 33.23
E) 23.32 to 35.46
A) 15.20 to 34.80
B) 24.18 to 25.82
C) 24.02 to 25.98
D) 16.78 to 33.23
E) 23.32 to 35.46

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
35
A researcher is interested in estimating the mean weight of a semi-tracker truck to determine the potential load capacity. She takes a random sample of 17 trucks and computes a sample mean of 20,000 pounds with sample standard deviation of 1,500. She decides to construct a 98% confidence interval to estimate the mean. The degrees of freedom associated with this problem are ___.
A) 18
B) 17
C) 16
D) 15
E) 20
A) 18
B) 17
C) 16
D) 15
E) 20

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
36
Brian Vanecek, VP of Operations at Portland Trust Bank, is evaluating the service level provided to walk-in customers. Accordingly, his staff recorded the waiting times for 64 randomly selected walk-in customers and determined that their mean waiting time was 15 minutes. Assume that the population standard deviation is 4 minutes. The 95% confidence interval for the population mean of waiting times is ___.
A) 14.02 to 15.98
B) 7.16 to 22.84
C) 14.06 to 15.94
D) 8.42 to 21.58
E) 19.80 to 23.65
A) 14.02 to 15.98
B) 7.16 to 22.84
C) 14.06 to 15.94
D) 8.42 to 21.58
E) 19.80 to 23.65

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
37
The z value associated with a two-sided 99% confidence interval is ___.
A) 1.28
B) 1.645
C) 1.96
D) 2.575
E) 2.33
A) 1.28
B) 1.645
C) 1.96
D) 2.575
E) 2.33

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
38
The table t value associated with the upper 5% of the t distribution and 14 degrees of freedom is ___.
A) 2.977
B) 2.624
C) 2.145
D) 1.761
E) 1.345
A) 2.977
B) 2.624
C) 2.145
D) 1.761
E) 1.345

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
39
The z value associated with a two?sided 80% confidence interval is ___.
A) 1.645
B) 1.28
C) 0.84
D) 0.29
E) 2.00
A) 1.645
B) 1.28
C) 0.84
D) 0.29
E) 2.00

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
40
The table t value associated with the upper 10% of the t distribution and 23 degrees of freedom is ___.
A) 1.319
B) 1.714
C) 2.069
D) 1.321
E) 2.332
A) 1.319
B) 1.714
C) 2.069
D) 1.321
E) 2.332

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
41
A random sample of 225 items from a population results in 60% possessing a given characteristic. Using this information, the researcher constructs a 90% confidence interval to estimate the population proportion. The resulting confidence interval is ___.
A) 0.546 to 0.654
B) 0.536 to 0.664
C) 0.596 to 0.604
D) 0.571 to 0.629
E) 0.629 to 0.687
A) 0.546 to 0.654
B) 0.536 to 0.664
C) 0.596 to 0.604
D) 0.571 to 0.629
E) 0.629 to 0.687

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
42
A large trucking company wants to estimate the proportion of its tracker truck population with refrigerated carrier capacity. A random sample of 200 tracker trucks is taken and 30% of the sample have refrigerated carrier capacity. The 95% confidence interval to estimate the population proportion is ___.
A) 0.53 to 0.67
B) 0.25 to 0.35
C) 0.24 to 0.36
D) 0.27 to 0.33
E) 0.28 to 0.34
A) 0.53 to 0.67
B) 0.25 to 0.35
C) 0.24 to 0.36
D) 0.27 to 0.33
E) 0.28 to 0.34

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
43
Elwin Osbourne, CIO at GFS, Inc., is studying employee use of GFS email for non-business communications. A random sample of 200 email messages was selected. Thirty of the messages were business related. The 90% confidence interval for the population proportion is ___.
A) 0.108 to 0.192
B) 0.153 to 0.247
C) 0.091 to 0.209
D) 0.145 to 0.255
E) 0.255 to 0.265
A) 0.108 to 0.192
B) 0.153 to 0.247
C) 0.091 to 0.209
D) 0.145 to 0.255
E) 0.255 to 0.265

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
44
A large national company is considering negotiating cellular phone rates for its employees. The Human Resource department would like to estimate the proportion of its employee population who own an Apple iPhone. A random sample of size 250 is taken and 40% of the sample own an iPhone. The 90% confidence interval to estimate the population proportion is ___.
A) 0.35 to 0.45
B) 0.34 to 0.46
C) 0.37 to 0.43
D) 0.39 to 0.41
E) 0.40 to 0.45
A) 0.35 to 0.45
B) 0.34 to 0.46
C) 0.37 to 0.43
D) 0.39 to 0.41
E) 0.40 to 0.45

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
45
The t score (or table value for Student's t distribution) associated with the upper 5% and 48 degrees of freedom is ___.
A) 1.677
B) 1.687
C) 1.697
D) 1.707
E) 1.717
A) 1.677
B) 1.687
C) 1.697
D) 1.707
E) 1.717

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
46
A large national company is considering negotiating cellular phone rates for its employees. The Human Resource department would like to estimate the proportion of its employee population who own an Apple iPhone. A random sample of size 250 is taken and 40% of the sample own an iPhone. The 95% confidence interval to estimate the population proportion is ___.
A) 0.35 to 0.45
B) 0.34 to 0.46
C) 0.37 to 0.43
D) 0.39 to 0.41
E) 0.40 to 0.42
A) 0.35 to 0.45
B) 0.34 to 0.46
C) 0.37 to 0.43
D) 0.39 to 0.41
E) 0.40 to 0.42

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
47
A researcher wants to estimate the proportion of the population which possesses a given characteristic. A random sample of size 1800 is taken resulting in 450 items which possess the characteristic. The point estimate for this population proportion is ___.
A) 0.55
B) 0.45
C) 0.35
D) 0.25
E) 0.15
A) 0.55
B) 0.45
C) 0.35
D) 0.25
E) 0.15

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
48
Elwin Osbourne, CIO at GFS, Inc., is studying employee use of GFS email for non-business communications. A random sample of 200 email messages was selected. Thirty of the messages were business related. The 95% confidence interval for the population proportion is ___.
A) 0.108 to 0.192
B) 0.153 to 0.247
C) 0.091 to 0.209
D) 0.101 to 0.199
E) 0.199 to 0.201
A) 0.108 to 0.192
B) 0.153 to 0.247
C) 0.091 to 0.209
D) 0.101 to 0.199
E) 0.199 to 0.201

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
49
The employees of Cybertronics Inc. need to complete a certification online. A random sample of 16 employees gives an average time for completion of all the coursework and passing the tests of 20 hours. The population standard deviation is unknown but the sample standard deviation is 6 hours. You can assume that the population of employees is fairly large. Construct a 95% confidence interval for the average time required to complete the certification.
A) 17.06 to 22.94
B) 17.37 to 22.63
C) 17.68 to 22.32
D) 17.99 to 22.01
E) 18.31 to 21.69
A) 17.06 to 22.94
B) 17.37 to 22.63
C) 17.68 to 22.32
D) 17.99 to 22.01
E) 18.31 to 21.69

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
50
A researcher wants to estimate the proportion of the population which possesses a given characteristic. A random sample of size 800 is taken resulting in 360 items which possess the characteristic. The point estimate for this population proportion is ___.
A) 0.55
B) 0.45
C) 0.35
D) 0.65
E) 0.70
A) 0.55
B) 0.45
C) 0.35
D) 0.65
E) 0.70

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
51
A researcher is interested in estimating the mean weight of a semi-tracker truck to determine the potential load capacity. She takes a random sample of 17 trucks and computes a sample mean of 20,000 pounds with sample standard deviation of 1,500. The 90% confidence interval for the population mean rod length is ___.
A) 19,365 to 20,635
B) 19,367 to 20,633
C) 19,514 to 20,486
D) 19,515 to 20,485
E) 18,500 to 21,500
A) 19,365 to 20,635
B) 19,367 to 20,633
C) 19,514 to 20,486
D) 19,515 to 20,485
E) 18,500 to 21,500

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
52
A large trucking company wants to estimate the proportion of its tracker truck population with refrigerated carrier capacity. A random sample of 200 tracker trucks is taken and 30% of the sample have refrigerated carrier capacity. The 90% confidence interval to estimate the population proportion is ___.
A) 0.53 to 0.67
B) 0.25 to 0.35
C) 0.24 to 0.36
D) 0.27 to 0.33
E) 0.33 to 0.39
A) 0.53 to 0.67
B) 0.25 to 0.35
C) 0.24 to 0.36
D) 0.27 to 0.33
E) 0.33 to 0.39

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
53
Elwin Osbourne, CIO at GFS, Inc., is studying employee use of GFS email for non-business communications. A random sample of 200 email messages was selected. Thirty of the messages were business related. The point estimate for this population proportion is ___.
A) 0.150
B) 0.300
C) 0.182
D) 0.667
E) 0.786
A) 0.150
B) 0.300
C) 0.182
D) 0.667
E) 0.786

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
54
Life tests performed on a sample of 13 batteries of a new model indicated: (1) an average life of 75 months, and (2) a standard deviation of 5 months. Other battery models, produced by similar processes, have normally distributed life spans. The 98% confidence interval for the population mean life of the new model is ___.
A) 63.37 to 86.63
B) 61.60 to 88.41
C) 71.77 to 78.23
D) 71.28 to 78.72
E) 79.86 to 81.28
A) 63.37 to 86.63
B) 61.60 to 88.41
C) 71.77 to 78.23
D) 71.28 to 78.72
E) 79.86 to 81.28

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
55
Elwin Osbourne, CIO at GFS, Inc., is studying employee use of GFS email for non-business communications. A random sample of 200 email messages was selected. Thirty of the messages were business related. The 98% confidence interval for the population proportion is ___.
A) 0.108 to 0.192
B) 0.153 to 0.247
C) 0.091 to 0.209
D) 0.145 to 0.255
E) 0.250 to 0.275
A) 0.108 to 0.192
B) 0.153 to 0.247
C) 0.091 to 0.209
D) 0.145 to 0.255
E) 0.250 to 0.275

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
56
Catherine Chao, Director of Marketing Research, is evaluating consumer acceptance of a new toothpaste package. She randomly selects a sample of 200 households. Forty households prefer the new package to all other package designs. The point estimate for this population proportion is ___.
A) 0.20
B) 0.25
C) 0.40
D) 0.45
E) 0.55
A) 0.20
B) 0.25
C) 0.40
D) 0.45
E) 0.55

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
57
For a new product, you need to determine the average diameter of a specialized electronic component, which will be a critical component of the new product. You measure the diameter in a sample of size 15 and find an average diameter of 0.24 mm, with a standard deviation of 0.02 mm. Other studies indicate that the diameter of similar products is normally distributed. The 99% confidence interval for the average diameter of this electronic component is ___.
A) 0.232 to 0.248
B) 0.230 to 0.250
C) 0.228 to 0.252
D) 0.226 to 0.254
E) 0.224 to 0.256
A) 0.232 to 0.248
B) 0.230 to 0.250
C) 0.228 to 0.252
D) 0.226 to 0.254
E) 0.224 to 0.256

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
58
A random sample of 225 items from a population results in 60% possessing a given characteristic. Using this information, the researcher constructs a 99% confidence interval to estimate the population proportion. The resulting confidence interval is ___.
A) 0.54 to 0.66
B) 0.59 to 0.61
C) 0.57 to 0.63
D) 0.52 to 0.68
E) 0.68 to 0.76
A) 0.54 to 0.66
B) 0.59 to 0.61
C) 0.57 to 0.63
D) 0.52 to 0.68
E) 0.68 to 0.76

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
59
Life tests performed on a sample of 13 batteries of a new model indicated: (1) an average life of 75 months, and (2) a standard deviation of 5 months. Other battery models, produced by similar processes, have normally distributed life spans. The 90% confidence interval for the population mean life of the new model is ___.
A) 66.78 to 83.23
B) 72.72 to 77.28
C) 72.53 to 77.47
D) 66.09 to 83.91
E) 73.34 to 76.25
A) 66.78 to 83.23
B) 72.72 to 77.28
C) 72.53 to 77.47
D) 66.09 to 83.91
E) 73.34 to 76.25

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
60
The weights of aluminum castings produced by a process are normally distributed. A random sample of 5 castings is selected; the sample mean weight is 2.21 pounds; and the sample standard deviation is 0.12 pound. The 98% confidence interval for the population mean casting weight is ___.
A) 1.76 to 2.66
B) 2.00 to 2.41
C) 2.08 to 2.34
D) 1.93 to 2.49
E) 2.49 to 2.67
A) 1.76 to 2.66
B) 2.00 to 2.41
C) 2.08 to 2.34
D) 1.93 to 2.49
E) 2.49 to 2.67

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
61
A researcher wants to estimate the percent of the population that uses the radio to stay informed on local news issues. The researcher wants to estimate the population proportion with a 90% level of confidence. He estimates from previous studies that no more than 30% of the population stay informed on local issues through the radio. The researcher wants the estimate to have an error of no more than.02. The necessary sample size is at least ___.
A) 29
B) 47
C) 298
D) 1421
E) 1500
A) 29
B) 47
C) 298
D) 1421
E) 1500

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
62
Elwin Osbourne, CIO at GFS, Inc., is studying employee use of GFS email for non-business communications. He plans to use a 95% confidence interval estimate of the proportion of email messages that are non-business; he will accept a 0.05 error. Previous studies indicate that approximately 30% of employee email is business related. Elwin should sample ___ email messages.
A) 323
B) 12
C) 457
D) 14
E) 100
A) 323
B) 12
C) 457
D) 14
E) 100

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
63
Catherine Chao, Director of Marketing Research, is evaluating consumer acceptance of a new toothpaste package. She plans to use a 95% confidence interval estimate of the proportion of households which prefer the new packages; she will accept a 0.05 error. Previous studies indicate that new packaging has an approximately 70% acceptance rate. The sample size should be at least ___.
A) 27
B) 59
C) 323
D) 427
E) 500
A) 27
B) 59
C) 323
D) 427
E) 500

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
64
A researcher wants to determine the sample size necessary to adequately conduct a study to estimate the population mean to within 5 points. The range of population values is 80 and the researcher plans to use a 90% level of confidence. The sample size should be at least ___.
A) 44
B) 62
C) 216
D) 692
E) 700
A) 44
B) 62
C) 216
D) 692
E) 700

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
65
A researcher wants to estimate the percent of the population that uses the internet to stay informed on world news issues. The researcher wants to estimate the population proportion with a 98% level of confidence. He estimates from previous studies that at least 65% of the population stay informed on world issues through the internet. He also wants the error to be no more than.03. The sample size should be at least ___.
A) 41
B) 313
C) 1677
D) 1373
E) 1500
A) 41
B) 313
C) 1677
D) 1373
E) 1500

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
66
A study is going to be conducted in which a population mean will be estimated using a 92% confidence interval. The estimate needs to be within 12 of the actual population mean. The population variance is estimated to be around 2500. The necessary sample size should be at least ___.
A) 15
B) 47
C) 54
D) 638
E) 700
A) 15
B) 47
C) 54
D) 638
E) 700

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
67
The manager of City Pizza wanted to estimate the mean amount spent per customer at his shop on Friday nights. From a sample of 50 Friday night receipts, he determined that the mean amount spent per customer was $15.50. Assume that the amount spent per customer on Friday nights is normally distributed with a standard deviation of $2.00. Construct a 98% confidence interval for the true mean amount each customer spent on Friday nights.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
68
An insurance company is interested in conducting a study to estimate the population proportion of teenagers who obtain a driving permit within 1 year of their 16th birthday. A level of confidence of 99% will be used and an error of no more than.04 is desired. There is no knowledge as to what the population proportion will be. The size of sample should be at least ___.
A) 1036
B) 160
C) 41
D) 259
E) 289
A) 1036
B) 160
C) 41
D) 259
E) 289

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
69
A researcher wants to estimate the proportion of manufacturing companies that use the Six Sigma method. For this purpose, she takes a random sample of 50 manufacturing companies and finds out that 28 of them employ this method. The point estimate for the proportion of the population that uses this method is ___.
A) 0.88
B) 0.56
C) 0.44
D) 0.28
E) 0.22
A) 0.88
B) 0.56
C) 0.44
D) 0.28
E) 0.22

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
70
A researcher wants to estimate the proportion of manufacturing companies that use the Six Sigma method. For this purpose, she takes a random sample of 50 manufacturing companies and finds out that 28 of them employ this method. The 95% confidence interval for the proportion of manufacturing companies that use Six Sigma is ___.
A) 0.462 to 0.628
B) 0.452 to 0.638
C) 0.442 to 0.648
D) 0.432 to 0.658
E) 0.422 to 0.668
A) 0.462 to 0.628
B) 0.452 to 0.638
C) 0.442 to 0.648
D) 0.432 to 0.658
E) 0.422 to 0.668

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
71
If you need to estimate the population mean within 2.5 units and with a confidence level of 95%, the minimum value observed is 15 and the maximum 65. What sample size would you use?
A) 9
B) 10
C) 81
D) 96
E) 97
A) 9
B) 10
C) 81
D) 96
E) 97

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
72
A sample of 120 adults from a large population has a mean IQ of 112 with a standard deviation of 10. Construct a 98% confidence interval for the true mean IQ of the population.

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
73
A researcher wants to estimate the proportion of millennials who regularly listen to podcasts (defined as at least two entire podcasts per week). Previous studies indicate that only 18% of millennials listen regularly to podcasts, and this researcher wants to estimate if that figure has increased. For this purpose, the researcher will use a 98% confidence level and wants to estimate the population proportion within 0.01 (i.e., 1 percentage point) of the actual value. The required sample size is at least ___.
A) 47
B) 48
C) 320
D) 321
E) 331
A) 47
B) 48
C) 320
D) 321
E) 331

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
74
Elwin Osbourne, CIO at GFS, Inc., is studying employee use of GFS email for non-business communications. He plans to use a 98% confidence interval estimate of the proportion of email messages that are non-business; he will accept a 0.05 error. Previous studies indicate that approximately 30% of employee email is business related. Elwin should sample ___ email messages.
A) 323
B) 12
C) 457
D) 14
E) 100
A) 323
B) 12
C) 457
D) 14
E) 100

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
75
A researcher wants to estimate the proportion of the manufacturing companies that use the Six Sigma method. For this purpose, she will take a random sample of 50 manufacturing companies and find out how many of them use this method. She estimates that the proportion will be anywhere from 0.4 to 0.55. Of all these proportions, which one would produce the narrowest 95% confidence interval for the population proportion?
A) 0.4
B) 0.45
C) 0.5
D) 0.55
E) 0.6
A) 0.4
B) 0.45
C) 0.5
D) 0.55
E) 0.6

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
76
A researcher wants to estimate the percent of the population that uses the radio to stay informed on local news issues. The researcher wants to estimate the population proportion with a 95% level of confidence. He estimates from previous studies that no more than 30% of the population stay informed on local issues through the radio. The researcher wants the estimate to have an error of no more than.03. The necessary sample size is at least ___.
A) 27
B) 188
C) 211
D) 897
E) 900
A) 27
B) 188
C) 211
D) 897
E) 900

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
77
A study is going to be conducted in which a mean of a lifetime of batteries produced by a certain method will be estimated using a 90% confidence interval. The estimate needs to be within +/- 2 hours of the actual population mean. The population standard deviation σ is estimated to be around 25. The necessary sample size should be at least ___.
A) 100
B) 21
C) 923
D) 35
E) 423
A) 100
B) 21
C) 923
D) 35
E) 423

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
78
In estimating the sample size necessary to estimate π, if there is no good approximation for the value of P available, the value of ___ should be used as an estimate of π in the formula.
A) 0.10
B) 0.50
C) 0.40
D) 1.96
E) 2.00
A) 0.10
B) 0.50
C) 0.40
D) 1.96
E) 2.00

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
79
Catherine Chao, Director of Marketing Research, is evaluating consumer acceptance of a new toothpaste package. She randomly selects a sample of 200 households. Forty households prefer the new package to all other package designs. The 90% confidence interval for the population proportion is ___.
A) 0.199 to 0.201
B) 0.153 to 0.247
C) 0.164 to 0.236
D) 0.145 to 0.255
E) 0.185 to 0.275
A) 0.199 to 0.201
B) 0.153 to 0.247
C) 0.164 to 0.236
D) 0.145 to 0.255
E) 0.185 to 0.275

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck
80
A researcher wants to estimate the proportion of manufacturing companies that use the Six Sigma method. For this purpose, she will take a random sample of 50 manufacturing companies and find out how many of them use this method. She estimates that the proportion will be anywhere from 0.4 to 0.55. Of all these proportions, which one would produce the widest 95% confidence interval for the population proportion?
A) 0.4
B) 0.45
C) 0.5
D) 0.55
E) 0.6
A) 0.4
B) 0.45
C) 0.5
D) 0.55
E) 0.6

Unlock Deck
Unlock for access to all 82 flashcards in this deck.
Unlock Deck
k this deck


