Deck 10: One-Sample Tests of Hypothesis
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/134
Play
Full screen (f)
Deck 10: One-Sample Tests of Hypothesis
1
What is the level of significance?
A) Probability of a Type II error
B) Probability of a Type I error
C) z-value of 1.96
D) Beta error
A) Probability of a Type II error
B) Probability of a Type I error
C) z-value of 1.96
D) Beta error
B
2
Type II error is the probability or risk of rejecting a null hypothesis when it is actually true.
False
3
The probability of a Type I error is also referred to as alpha.
True
4
A null hypothesis makes a claim about a
A) Population parameter
B) Sample statistic
C) Sample mean
D) Type II error
A) Population parameter
B) Sample statistic
C) Sample mean
D) Type II error

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
5
An alternate hypothesis is a statement about a population parameter that is accepted when the null hypothesis is rejected.

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
6
The researcher must decide on the level of significance before formulating a decision rule and collecting sample data.

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
7
An example of a hypothesis is: a person is innocent until proven guilty.

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
8
A p-value is the same as a stated significance level.

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
9
A test statistic is a value computed from sample information and used to test the null hypothesis.

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
10
Test at the 0.01 level the statement that 55% of those families who plan to purchase a vacation residence in Florida want a condominium. The null hypothesis is = 0.55 and the alternate is .55. A random sample of 400 families who planned to buy a vacation residence revealed that 228 families want a condominium. What decision should be made regarding the null hypothesis?
A) Do not reject it
B) Reject it
C) Cannot accept nor reject it based on the information given
D) Purchase a condominium.
A) Do not reject it
B) Reject it
C) Cannot accept nor reject it based on the information given
D) Purchase a condominium.

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
11
For a one-tailed test using the 0.05 level of significance, the critical value for the z test is 1.645, but for t it is 1.96.

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
12
If the null hypothesis is 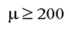 and the alternate hypothesis states that µ is less than 200, then, a two-tail test is being conducted.
and the alternate hypothesis states that µ is less than 200, then, a two-tail test is being conducted.
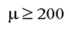 and the alternate hypothesis states that µ is less than 200, then, a two-tail test is being conducted.
and the alternate hypothesis states that µ is less than 200, then, a two-tail test is being conducted.
Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
13
The probability of a Type II error is represented by the Greek symbol, .

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
14
Assuming that the null hypothesis is true, a p-value is the probability of observing a sample value greater than and/or less than an observed sample observation.

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
15
If the null hypothesis is false and the researchers do not reject it, then a Type I error has been made.

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
16
Hypothesis testing is a procedure that uses sample evidence and probability theory to decide whether to reject or fail to reject a hypothesis.

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
17
A p-value is a probability.

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
18
A manufacturer wants to increase the shelf life of a line of cake mixes. Past records indicate that the average shelf life of the mix is 216 days. After a revised mix has been developed, a sample of nine boxes of cake mix gave these shelf lives (in days): 215, 217, 218, 219, 216, 217, 217, 218 and 218. At the 0.025 level, has the shelf life of the cake mix increased?
A) Yes, because computed t is greater than the critical value.
B) Yes, because computed t is less than the critical value.
C) No, because computed t lies in the region of acceptance.
D) No, because 217.24 is quite close to 216.
A) Yes, because computed t is greater than the critical value.
B) Yes, because computed t is less than the critical value.
C) No, because computed t lies in the region of acceptance.
D) No, because 217.24 is quite close to 216.

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
19
The level of significance is the risk of rejecting the null hypothesis when it is actually true.

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
20
The mean length of a small counter balance bar is 43 millimeters. There is concern that the settings of the machine producing the bars have changed. Test the claim at the 0.02 level that there has been no change in the mean length. The alternate hypothesis is that there has been a change. Twelve bars (n = 12) were selected at random and their lengths recorded. The lengths are (in millimeters) 42, 39, 42, 45, 43, 40, 39, 41, 40, 42, 43 and 42. The mean of the sample is 41.5 and the standard deviation 1.784. Computed t = -2.913. Has there been a statistically significant change in the mean length of the bars?
A) Yes, because the computed t lies in the rejection region.
B) No, because the information given is not complete.
C) No, because the computed t lies in the area to the right of -2.718.
D) Yes, because 43 is greater than 41.5.
A) Yes, because the computed t lies in the rejection region.
B) No, because the information given is not complete.
C) No, because the computed t lies in the area to the right of -2.718.
D) Yes, because 43 is greater than 41.5.

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
21
What are the two critical values for a two-tailed test with a 0.01 level of significance when n is large and the population standard deviation is known?
A) Above 1.96 and below -1.96
B) Above 1.65 and below -1.65
C) Above 2.58 and below -2.58
D) Above 1.00 and below -1.00
A) Above 1.96 and below -1.96
B) Above 1.65 and below -1.65
C) Above 2.58 and below -2.58
D) Above 1.00 and below -1.00

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
22
What is another name for the alternate hypothesis?
A) Null hypothesis
B) Hypothesis of no difference
C) Rejected hypothesis
D) Research hypothesis
A) Null hypothesis
B) Hypothesis of no difference
C) Rejected hypothesis
D) Research hypothesis

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
23
A manufacturer wants to increase the absorption capacity of a sponge. Based on past data, the average sponge could absorb 3.5 ounces. After the redesign, the absorption amounts of a sample of sponges were (in ounces): 4.1, 3.7, 3.3, 3.5, 3.8, 3.9, 3.6, 3.8, 4.0, and 3.9. What is the decision rule at the 0.01 level of significance to test if the new design increased the absorption amount of the sponge?
A) Do not reject null hypothesis if computed t is less than 2.580
B) Do not reject null hypothesis if computed t is less than 2.821
C) Reject null hypothesis if computed z is 1.96 or larger
D) Reject null hypothesis if computed t is less than 2.764
A) Do not reject null hypothesis if computed t is less than 2.580
B) Do not reject null hypothesis if computed t is less than 2.821
C) Reject null hypothesis if computed z is 1.96 or larger
D) Reject null hypothesis if computed t is less than 2.764

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
24
If the 1% level of significance is used and the z-test statistic is +6.00, what is our decision?
A) Do not reject H0
B) Reject H0
C) Reject H1
D) None of these
A) Do not reject H0
B) Reject H0
C) Reject H1
D) None of these

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
25
What do we call the statement that determines if the null hypothesis is rejected?
A) Decision rule
B) Test statistic
C) Alternate hypothesis
D) Critical value
A) Decision rule
B) Test statistic
C) Alternate hypothesis
D) Critical value

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
26
The number of trials and the population proportion are respectively represented by what symbols?
A) p and n
B)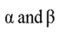
C) z and t
D) n and
A) p and n
B)
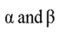
C) z and t
D) n and

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
27
What is a Type II error?
A) Accepting a false null hypothesis
B) Rejecting a false null hypothesis
C) Accepting a false alternate hypothesis
D) Rejecting a false alternate hypothesis
A) Accepting a false null hypothesis
B) Rejecting a false null hypothesis
C) Accepting a false alternate hypothesis
D) Rejecting a false alternate hypothesis

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
28
What is the probability of making a Type II error if the null hypothesis is actually true?
A)
B) 1
C) 0
D) 0.05
A)
B) 1
C) 0
D) 0.05

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
29
To conduct a test of hypothesis with a small sample, we need to be able to make an assumption that:
A) a larger computed value of t will be needed to reject the null hypothesis.
B) the region of acceptance will be wider than for large samples.
C) the confidence interval will be wider than for large samples.
D) the population is normally distributed.
A) a larger computed value of t will be needed to reject the null hypothesis.
B) the region of acceptance will be wider than for large samples.
C) the confidence interval will be wider than for large samples.
D) the population is normally distributed.

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
30
If the alternate hypothesis states that µ does not equal 4,000, what is the rejection region for the hypothesis test?
A) Both tails
B) Lower or left tail
C) Upper or right tail
D) Center
A) Both tails
B) Lower or left tail
C) Upper or right tail
D) Center

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
31
For a one-tailed hypothesis test, the critical z-value of the test statistic is -2.33. Which of the following is true about the hypothesis test?
A) = 0.05 for a lower-tailed test
B) = 0.01 for a lower-tailed test
C) = 0.05 for an upper-tailed test
D) = 0.01 for an upper-tailed test
A) = 0.05 for a lower-tailed test
B) = 0.01 for a lower-tailed test
C) = 0.05 for an upper-tailed test
D) = 0.01 for an upper-tailed test

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
32
Which symbol represents a test statistic used to test a hypothesis about a population mean?
A)
B)
C)
D) z
A)
B)
C)
D) z

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
33
For a hypothesis test with an alternative hypothesis: µ > 6,700, where is the rejection region for the hypothesis test located?
A) Both tails
B) Left or lower tail
C) Right or upper tail
D) Center
A) Both tails
B) Left or lower tail
C) Right or upper tail
D) Center

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
34
If we reject the null hypothesis, what can we conclude subject to the risk?
A) Null hypothesis is false
B) Alternative hypothesis is false
C) Null hypothesis is true
D) Both the null hypothesis and the alternative hypothesis are true
A) Null hypothesis is false
B) Alternative hypothesis is false
C) Null hypothesis is true
D) Both the null hypothesis and the alternative hypothesis are true

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
35
For a two-tailed test with a 0.05 significance level, what is the rejection region when n is large and the population standard deviation is known?
A) Between 1.96
B) Between 1.65
C) Greater than +1.96 and less than -1.96
D) Greater than +1.65 and less than -1.65
A) Between 1.96
B) Between 1.65
C) Greater than +1.96 and less than -1.96
D) Greater than +1.65 and less than -1.65

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
36
A machine is set to fill the small size packages of M&M candies with 56 candies per bag. A sample revealed: 3 bags of 56, 2 bags of 57, 1 bag of 55, and 2 bags of 58. How many degrees of freedom are there?
A) 9
B) 1
C) 8
D) 7
A) 9
B) 1
C) 8
D) 7

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
37
Using a 5% level of significance and a sample size of 25, what is the critical value for a one-tailed hypothesis test?
A) 1.708
B) 1.711
C) 2.060
D) 2.064
A) 1.708
B) 1.711
C) 2.060
D) 2.064

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
38
If the critical z-value for a test statistic equals 2.45, what value of the test statistic would provide the least chance of making a Type I error?
A) 3.74
B) 10,000
C) 2.46
D) 4.56
A) 3.74
B) 10,000
C) 2.46
D) 4.56

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
39
What are the critical z-values for a two-tailed hypothesis test if = 0.01?
A) 1.96
B) 2.33
C) 2.58
D) 1.65
A) 1.96
B) 2.33
C) 2.58
D) 1.65

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
40
A random sample of size 15 is selected from a normal population. The population standard deviation is unknown. Assume that a two-tailed test at the 0.10 significance level is to be used. For what value of t will the null hypothesis not be rejected?
A) To the left of -1.282 or to the right of 1.282
B) To the left of -1.345 or to the right of 1.345
C) Between -1.761 and 1.761
D) To the left of -1.645 or to the right of 1.645
A) To the left of -1.282 or to the right of 1.282
B) To the left of -1.345 or to the right of 1.345
C) Between -1.761 and 1.761
D) To the left of -1.645 or to the right of 1.645

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
41
Based on the Nielsen ratings, the local CBS affiliate claims its 11:00 PM newscast reaches 41% of the viewing audience in the area. In a survey of 100 viewers, 36% indicated that they watch the late evening news on this local CBS station. What is the null hypothesis?
A) = 0.36
B) = 0.41
C) 0.36
D) µ = 0.41
A) = 0.36
B) = 0.41
C) 0.36
D) µ = 0.41

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
42
Based on the Nielsen ratings, the local CBS affiliate claims its 11:00 PM newscast reaches 41% of the viewing audience in the area. In a survey of 100 viewers, 36% indicated that they watch the late evening news on this local CBS station. What is the critical value if the level of significance is 0.10?
A) -1.282
B) 1.65
C) -2.58
D) 2.58
A) -1.282
B) 1.65
C) -2.58
D) 2.58

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
43
In hypothesis testing, what is the level of significance?
A) Risk of rejecting the null hypothesis when it is true
B) Symbolized by the Greek letter " "
C) Value between 0 and 1
D) Selected before a decision rule can be formulated
E) All of these are true
A) Risk of rejecting the null hypothesis when it is true
B) Symbolized by the Greek letter " "
C) Value between 0 and 1
D) Selected before a decision rule can be formulated
E) All of these are true

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
44
Based on the Nielsen ratings, the local CBS affiliate claims its 11:00 PM newscast reaches 41% of the viewing audience in the area. In a survey of 100 viewers, 36% indicated that they watch the late evening news on this local CBS station. What is the sample proportion?
A) 0.41
B) 0.36%
C) 0.41%
D) 0.36
A) 0.41
B) 0.36%
C) 0.41%
D) 0.36

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
45
Which of the following is NOT one of the five steps in the hypothesis testing procedure?
A) Formulate a decision rule
B) State the null and alternate hypotheses
C) Select a level for
D) Identify the test statistic
A) Formulate a decision rule
B) State the null and alternate hypotheses
C) Select a level for
D) Identify the test statistic

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
46
The average cost of tuition, room and board at small private liberal arts colleges is reported to be $8,500 per term, but a financial administrator believes that the average cost is higher. A study conducted using 350 small liberal arts colleges showed that the average cost per term is $8,745 with a standard deviation of $1,200. Let = 0.05. Based on the computed test statistic or p-value, what is our decision about the average cost?
A) Equal to $8,500
B) Greater than $8,500
C) Less than $8,500
D) Not equal to $8,500
A) Equal to $8,500
B) Greater than $8,500
C) Less than $8,500
D) Not equal to $8,500

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
47
Based on the Nielsen ratings, the local CBS affiliate claims its 11:00 PM newscast reaches 41% of the viewing audience in the area. In a survey of 100 viewers, 36% indicated that they watch the late evening news on this local CBS station. What is your decision if = 0.01?
A) Fail to reject the null hypothesis and conclude the newscast reaches about 41% of the audience.
B) Reject the null hypothesis and conclude the newscast does not reach 41% of the audience.
C) Fail to reject the alternate and conclude the newscast does not reach 41% of the audience.
D) Reject the alternate and conclude the newscast reaches about 41% of the audience.
A) Fail to reject the null hypothesis and conclude the newscast reaches about 41% of the audience.
B) Reject the null hypothesis and conclude the newscast does not reach 41% of the audience.
C) Fail to reject the alternate and conclude the newscast does not reach 41% of the audience.
D) Reject the alternate and conclude the newscast reaches about 41% of the audience.

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
48
Based on the Nielsen ratings, the local CBS affiliate claims its 11:00 PM newscast reaches 41% of the viewing audience in the area. In a survey of 100 viewers, 36% indicated that they watch the late evening news on this local CBS station. What is the p-value?
A) 0.3461
B) 0.1539
C) 0.3078
D) 0.0100
A) 0.3461
B) 0.1539
C) 0.3078
D) 0.0100

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
49
The average cost of tuition, room and board at small private liberal arts colleges is reported to be $8,500 per term, but a financial administrator believes that the average cost is higher. A study conducted using 350 small liberal arts colleges showed that the average cost per term is $8,745 with a standard deviation of $1,200. Let = 0.05. What are the null and alternative hypotheses for this study?
A) Null: µ $9,000; alternative: µ > $9,000
B) Null: µ $9,000; alternative: µ < $9,000
C) Null: µ $8,500; alternative: µ > $8,500
D) Null: µ $8,500; alternative: µ < $8,500
A) Null: µ $9,000; alternative: µ > $9,000
B) Null: µ $9,000; alternative: µ < $9,000
C) Null: µ $8,500; alternative: µ > $8,500
D) Null: µ $8,500; alternative: µ < $8,500

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
50
The sample proportion is defined as:
A) n
B) x/n
C) n!
D)
A) n
B) x/n
C) n!
D)

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
51
Based on the Nielsen ratings, the local CBS affiliate claims its 11:00 PM newscast reaches 41% of the viewing audience in the area. In a survey of 100 viewers, 36% indicated that they watch the late evening news on this local CBS station. What is the alternate hypothesis?
A) = 0.36
B) = 0.41
C) 0.41
D) µ 0.41
A) = 0.36
B) = 0.41
C) 0.41
D) µ 0.41

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
52
It is claimed that in a bushel of peaches, less than ten percent are defective. A sample of 400 peaches is examined and 50 are found to be defective. What is the null hypothesis?
A) 0.10
B) 0.10
C) 0.10
D) < 0.10
A) 0.10
B) 0.10
C) 0.10
D) < 0.10

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
53
The average cost of tuition, room and board at small private liberal arts colleges is reported to be $8,500 per term, but a financial administrator believes that the average cost is higher. A study conducted using 350 small liberal arts colleges showed that the average cost per term is $8,745 with a standard deviation of $1,200. Let = 0.05. What is the test statistic for this test?
A) 3.82
B) 0.204
C) -3.82
D) +3.82
A) 3.82
B) 0.204
C) -3.82
D) +3.82

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
54
The average cost of tuition, room and board at small private liberal arts colleges is reported to be $8,500 per term, but a financial administrator believes that the average cost is higher. A study conducted using 350 small liberal arts colleges showed that the average cost per term is $8,745 with a standard deviation of $1,200. Let = 0.05. What is the p-value for this test?
A) 0.0000
B) 0.0124
C) 0.0500
D) 0.4938
A) 0.0000
B) 0.0124
C) 0.0500
D) 0.4938

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
55
Based on the Nielsen ratings, the local CBS affiliate claims its 11:00 PM newscast reaches 41% of the viewing audience in the area. In a survey of 100 viewers, 36% indicated that they watch the late evening news on this local CBS station. What is the critical value, if = 0.01?
A) 2.58
B) 2.33
C) 2.58
D) -2.33
A) 2.58
B) 2.33
C) 2.58
D) -2.33

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
56
Based on the Nielsen ratings, the local CBS affiliate claims its 11:00 PM newscast reaches 41% of the viewing audience in the area. In a survey of 100 viewers, 36% indicated that they watch the late evening news on this local CBS station. What is the z-statistic?
A) 1.02
B) 1.22
C) -1.02
D) -1.22
A) 1.02
B) 1.22
C) -1.02
D) -1.22

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
57
If 20 out of 50 students sampled live in a college dormitory, what is the estimated proportion of students at the University living in a dormitory?
A) 0.20
B) 0.40
C) 0.50
D) 0.60
A) 0.20
B) 0.40
C) 0.50
D) 0.60

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
58
The average cost of tuition, room and board at small private liberal arts colleges is reported to be $8,500 per term, but a financial administrator believes that the average cost is higher. A study conducted using 350 small liberal arts colleges showed that the average cost per term is $8,745 with a standard deviation of $1,200. Let = 0.05. What is the critical z-value for this test?
A) +1.96
B) -1.96
C) + 1.65
D) -1.65
A) +1.96
B) -1.96
C) + 1.65
D) -1.65

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
59
The claim that "40% of those persons who retired from an industrial job before the age of 60 would return to work if a suitable job was available," is to be investigated at the 0.02 level of risk. If 74 out of the 200 workers sampled said they would return to work, what is our decision?
A) Do not reject the null hypothesis because -0.866 lies in the region between 0 and -2.33
B) Do not reject the null hypothesis because -0.866 lies in the region between 0 and -2.58
C) Reject the null hypothesis because 37% is less than 40%
D) Do not reject the null hypothesis because 37% lies in the area between 0% and 40%
A) Do not reject the null hypothesis because -0.866 lies in the region between 0 and -2.33
B) Do not reject the null hypothesis because -0.866 lies in the region between 0 and -2.58
C) Reject the null hypothesis because 37% is less than 40%
D) Do not reject the null hypothesis because 37% lies in the area between 0% and 40%

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
60
A manufacturer of stereo equipment introduces new models in the fall. Retail dealers are surveyed immediately after the Christmas selling season regarding their stock on hand of each piece of equipment. It has been discovered that unless 40% of the new equipment ordered by the retailers in the fall had been sold by Christmas, immediate production cutbacks are needed. The manufacturer has found that contacting all of the dealers after Christmas by mail is frustrating as many of them never respond. This year 80 dealers were selected at random and telephoned regarding a new receiver. It was discovered that 38% of those receivers had been sold. Since 38% is less than 40%, does this mean that immediate production cutbacks are needed or can this difference of 2 percentage points be attributed to sampling? Test at the 0.05 level. Computed z = -0.37.
A) Cut back production.
B) Do not cut back production.
C) Increase production.
D) None of the above.
A) Cut back production.
B) Do not cut back production.
C) Increase production.
D) None of the above.

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
61
The probability of a Type II error is represented by
A)
B)
C) Type I error
D)
A)
B)
C) Type I error
D)

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
62
It is claimed that in a bushel of peaches, less than ten percent are defective. A sample of 400 peaches is examined and 50 are found to be defective. What is the z-statistic?
A) 0.025
B) 0.278
C) -1.65
D) 1.67
A) 0.025
B) 0.278
C) -1.65
D) 1.67

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
63
A hypothesis regarding the weight of newborn infants at a community hospital is that the mean is 6.6 pounds. A sample of seven infants is randomly selected and their weights at birth are recorded as 9.0, 7.3, 6.0, 8.8, 6.8, 8.4, and 6.6 pounds. What is the degree of freedom?
A) 7
B) 8
C) 6
D) 6.6
A) 7
B) 8
C) 6
D) 6.6

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
64
The probability of a Type II error is directly related to
A)
B) The standard deviation.
C) Type I error.
D) The difference between the hypothesized mean and the sample mean.
A)
B) The standard deviation.
C) Type I error.
D) The difference between the hypothesized mean and the sample mean.

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
65
It is claimed that in a bushel of peaches, less than ten percent are defective. A sample of 400 peaches is examined and 50 are found to be defective. If = 0.025, what will be the decision?
A) Fail to reject the null and conclude the defects are not greater than 10%
B) Reject the null and conclude the defects are not greater than 10%
C) Reject the null and conclude the defects are greater than 10%
D) Fail to reject the null and conclude the defects are not less than 10%
A) Fail to reject the null and conclude the defects are not greater than 10%
B) Reject the null and conclude the defects are not greater than 10%
C) Reject the null and conclude the defects are greater than 10%
D) Fail to reject the null and conclude the defects are not less than 10%

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
66
A hypothesis regarding the weight of newborn infants at a community hospital is that the mean is 6.6 pounds. A sample of seven infants is randomly selected and their weights at birth are recorded as 9.0, 7.3, 6.0, 8.8, 6.8, 8.4, and 6.6 pounds. What is the decision for a statistical significant change in average weights at birth at the 5% level of significance?
A) Fail to reject the null hypothesis and conclude the mean is 6.6 lb.
B) Reject the null hypothesis and conclude the mean is higher than 6.6 lb.
C) Reject the null hypothesis and conclude the mean is lower than 6.6 lb.
D) Cannot calculate because population standard deviation is unknown.
A) Fail to reject the null hypothesis and conclude the mean is 6.6 lb.
B) Reject the null hypothesis and conclude the mean is higher than 6.6 lb.
C) Reject the null hypothesis and conclude the mean is lower than 6.6 lb.
D) Cannot calculate because population standard deviation is unknown.

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
67
A hypothesis regarding the weight of newborn infants at a community hospital is that the mean is 6.6 pounds. A sample of seven infants is randomly selected and their weights at birth are recorded as 9.0, 7.3, 6.0, 8.8, 6.8, 8.4, and 6.6 pounds. The null hypothesis is
A) µ = 6.6
B) µ 6.6
C) µ > 7.6
D) µ 7.6
A) µ = 6.6
B) µ 6.6
C) µ > 7.6
D) µ 7.6

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
68
A hypothesis regarding the weight of newborn infants at a community hospital is that the mean is 6.6 pounds. A sample of seven infants is randomly selected and their weights at birth are recorded as 9.0, 7.3, 6.0, 8.8, 6.8, 8.4, and 6.6 pounds. What is the sample variance?
A) 1.177
B) 6.6
C) 1.385
D) 7.6
A) 1.177
B) 6.6
C) 1.385
D) 7.6

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
69
It is claimed that in a bushel of peaches, less than ten percent are defective. A sample of 400 peaches is examined and 50 are found to be defective. What is the critical value for = 0.025?
A) 1.96
B) 1.65
C) -1.96
D) -1.65
A) 1.96
B) 1.65
C) -1.96
D) -1.65

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
70
The mean gross annual incomes of certified welders are normally distributed with the mean of $20,000 and a standard deviation of $2,000. The ship building association wishes to find out whether their welders earn more or less than $20,000 annually. The alternate hypothesis is that the mean is not $20,000. If the level of significance is 0.10, what is the critical value?
A) 1.65
B) 1.28
C) 1.28
D) 1.65
A) 1.65
B) 1.28
C) 1.28
D) 1.65

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
71
A hypothesis regarding the weight of newborn infants at a community hospital is that the mean is 6.6 pounds. A sample of seven infants is randomly selected and their weights at birth are recorded as 9.0, 7.3, 6.0, 8.8, 6.8, 8.4, and 6.6 pounds. If = 0.05, what is the critical t value?
A) -2.365
B) 1.96
C) 2.365
D) 2.447
E) -2.447
A) -2.365
B) 1.96
C) 2.365
D) 2.447
E) -2.447

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
72
The mean gross annual incomes of certified welders are normally distributed with the mean of $20,000 and a standard deviation of $2,000. The ship building association wishes to find out whether their welders earn more or less than $20,000 annually. The alternate hypothesis is that the mean is not $20,000. Which of the following is the alternate hypothesis?
A) $20,000
B) µ $20,000
C) µ < $20,000
D) µ = $20,000
A) $20,000
B) µ $20,000
C) µ < $20,000
D) µ = $20,000

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
73
A hypothesis regarding the weight of newborn infants at a community hospital is that the mean is 6.6 pounds. A sample of seven infants is randomly selected and their weights at birth are recorded as 9.0, 7.3, 6.0, 8.8, 6.8, 8.4, and 6.6 pounds. What is the sample standard deviation?
A) 1.177
B) 6.6
C) 1.385
D) 7.6
A) 1.177
B) 6.6
C) 1.385
D) 7.6

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
74
A hypothesis regarding the weight of newborn infants at a community hospital is that the mean is 6.6 pounds. A sample of seven infants is randomly selected and their weights at birth are recorded as 9.0, 7.3, 6.0, 8.8, 6.8, 8.4, and 6.6 pounds. What is the decision for a significant increase in the average birthrate at a 5% level of significance?
A) Fail to reject the null hypothesis and conclude the mean is 6.6 lb.
B) Reject the null hypothesis and conclude the mean is lower than 6.6 lb.
C) Reject the null hypothesis and conclude the mean is greater than 6.6 lb.
D) Cannot calculate because population standard deviation is unknown.
A) Fail to reject the null hypothesis and conclude the mean is 6.6 lb.
B) Reject the null hypothesis and conclude the mean is lower than 6.6 lb.
C) Reject the null hypothesis and conclude the mean is greater than 6.6 lb.
D) Cannot calculate because population standard deviation is unknown.

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
75
It is claimed that in a bushel of peaches, less than ten percent are defective. A sample of 400 peaches is examined and 50 are found to be defective. What is the p-value?
A) 0.0250
B) 0.4525
C) 0.0475
D) 0.0500
A) 0.0250
B) 0.4525
C) 0.0475
D) 0.0500

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
76
A hypothesis regarding the weight of newborn infants at a community hospital is that the mean is 6.6 pounds. A sample of seven infants is randomly selected and their weights at birth are recorded as 9.0, 7.3, 6.0, 8.8, 6.8, 8.4, and 6.6 pounds. What is the sample mean?
A) 6.6
B) 7.6
C) 1.177
D) 2.447
A) 6.6
B) 7.6
C) 1.177
D) 2.447

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
77
A hypothesis regarding the weight of newborn infants at a community hospital is that the mean is 6.6 pounds. A sample of seven infants is randomly selected and their weights at birth are recorded as 9.0, 7.3, 6.0, 8.8, 6.8, 8.4, and 6.6 pounds. What is the alternate hypothesis?
A) µ = 6.6
B) µ 6.6
C) µ 6.6
D) µ > 7.6
A) µ = 6.6
B) µ 6.6
C) µ 6.6
D) µ > 7.6

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
78
It is claimed that in a bushel of peaches, less than ten percent are defective. A sample of 400 peaches is examined and 50 are found to be defective. What is the alternate hypothesis for a one-sided test?
A) 0.10
B) > 0.10
C) 0.10
D) < 0.10
A) 0.10
B) > 0.10
C) 0.10
D) < 0.10

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
79
It is claimed that in a bushel of peaches, less than ten percent are defective. A sample of 400 peaches is examined and 50 are found to be defective. What is the sample proportion?
A) 0.10
B) 0.125
C) 40
D) 0.40
A) 0.10
B) 0.125
C) 40
D) 0.40

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck
80
The mean gross annual incomes of certified welders are normally distributed with the mean of $20,000 and a standard deviation of $2,000. The ship building association wishes to find out whether their welders earn more or less than $20,000 annually. The alternate hypothesis is that the mean is not $20,000. If the level of significance is 0.10, what is the decision rule?
A) Do not reject the null hypothesis if computed z lies between -1.65 and +1.65; otherwise, reject it
B) Do not reject the null hypothesis if computed z is greater than 1.65; otherwise, reject it
C) Do not reject the null hypothesis if computed z lies between -1.96 and +1.96; otherwise, reject it
D) Reject the null hypothesis if computed z is below -1.96; otherwise, reject it
A) Do not reject the null hypothesis if computed z lies between -1.65 and +1.65; otherwise, reject it
B) Do not reject the null hypothesis if computed z is greater than 1.65; otherwise, reject it
C) Do not reject the null hypothesis if computed z lies between -1.96 and +1.96; otherwise, reject it
D) Reject the null hypothesis if computed z is below -1.96; otherwise, reject it

Unlock Deck
Unlock for access to all 134 flashcards in this deck.
Unlock Deck
k this deck


