Deck 9: Interest Rate Risk II

Question 1

Duration measures the average life of a financial asset.

Answer 1

True

Question 2

For a given maturity fixed-income asset, duration increases as the promised interest payment declines.

Answer 2

False

Question 3

A key assumption of Macaulay duration is that the yield curve is flat so that all cash flows are discounted at the same discount rate.

Answer 3

True

Question 4

For a given maturity fixed-income asset, duration decreases as the market yield increases.

Question 5

Duration normally is less than the maturity for a fixed income asset.

Question 6

Duration considers the timing of all the cash flows of an asset by summing the product of the cash flows and the time of occurrence.

Question 7

Duration is the weighted-average present value of the cash flows using the timing of the cash flows as weights.

Question 8

The economic meaning of duration is the interest elasticity of a financial assets price.

Question 9

Duration is equal to maturity when at least some of the cash flows are received upon maturity of the asset.

Question 10

In most countries FIs report their balance sheet using market value accounting.

Question 11

Duration increases with the maturity of a fixed-income asset at a decreasing rate.

Question 12

The difference between the changes in the market value of the assets and market value of liabilities for a given change in interest rates is, by definition, the change in the FI's net worth.

Question 13

In duration analysis, the times at which cash flows are received are weighted by the relative importance in present value terms of the cash flows arriving at each point in time.

Question 14

Marking-to-market accounting is a market value accounting method that reflects the purchase prices of assets and liabilities.

Question 15

As interest rates rise, the duration of a consol bond decreases.

Question 16

Duration is related to maturity in a linear manner through the interest rate of the asset.

Question 17

Larger coupon payments on a fixed-income asset cause the present value weights of the cash flows to be lower in the duration calculation.

Question 18

Duration is related to maturity in a nonlinear manner through the current yield to maturity of the asset.

Question 19

Duration of a fixed-rate coupon bond will always be greater than one-half of the maturity.

Question 20

Duration of a zero coupon bond is equal to the bond's maturity.

Question 21

Perfect matching of the maturities of the assets and liabilities will always achieve perfect immunization for the equity holders of an FI against interest rate risk.

Question 22

Matching the maturities of assets and liabilities is not a perfect method of immunizing the balance sheet because the timing of the cash flows is likely to differ between the assets and liabilities.

Question 23

The immunization of a portfolio against interest rate risk means that the portfolio will neither gain nor lose value when interest rates change.

Question 24

The larger the interest rate shock, the smaller the interest rate risk exposure of an FI.

Question 25

Investing in a zero-coupon asset with a maturity equal to the desired investment horizon is one method of immunizing against changes in interest rates.

Question 26

For given changes in interest rates, the change in the market value of net worth of an FI is equal to the difference between the changes in the market value of the assets and market value of the liabilities.

Question 27

Buying a fixed-rate asset whose duration is exactly equal to the desired investment horizon immunizes against interest rate risk.

Question 28

Using a fixed-rate bond to immunize a desired investment horizon means that the reinvested coupon payments are not affected by changes in market interest rates.

Question 29

The leverage adjusted duration of a typical depository institution is positive.

Question 30

One method of changing the positive leverage adjusted duration gap for the purpose of immunizing the net worth of a typical depository institution is to increase the duration of the assets and to decrease the duration of the liabilities.

Question 31

The duration of a portfolio of assets can be found by calculating the book value weighted average of the durations of the individual assets.

Question 32

An FI can immunize its portfolio by matching the maturity of its asset with its liabilities.

Question 33

For a given change in required yields, short-duration securities suffer a smaller capital loss or receive a smaller capital gain than do long-duration securities.

Question 34

Immunizing the balance sheet of an FI against interest rate risk requires that the leverage adjusted duration gap (D_A-kD_L) should be set to zero.

Question 35

The value for duration describes the percentage increase in the price of an asset for a given increase in the required yield or interest rate.

Question 36

Deep discount bonds are semi-annual fixed-rate coupon bonds that sell at a market price that is less than par value.

Question 37

Immunization of an FIs net worth requires the duration of the liabilities to be adjusted for the amount of leverage on the balance sheet.

Question 38

Investing in a zero-coupon asset with a maturity equal to the desired investment horizon removes interest rate risk from the investment management process.

Question 39

Setting the duration of the assets higher than the duration of the liabilities will exactly immunize the net worth of an FI from interest rate shocks.

Question 40

The smaller the leverage adjusted duration gap, the more exposed the FI is to interest rate shocks.

Question 41

The use of duration to predict changes in bond prices for given changes in interest rate changes will always underestimate the amount of the true price change.

Question 42

The error from using duration to estimate the new price of a fixed-income security will be less as the amount of convexity increases.

Question 43

The fact that the capital gain effect for rate decreases is greater than the capital loss effect for rate increases is caused by convexity in the yield-price relationship.

Question 44

The larger the size of an FI, the larger the _________ from any given interest rate shock.

A)duration mismatch
B)immunization effect
C)net worth exposure
D)net interest income
E)risk of bankruptcy

Question 45

The cost in terms of both time and money to restructure the balance sheet of large and complex FIs has decreased over time.

Question 46

Attempts to satisfy the objectives of shareholders and regulators requires the bank to use the same duration match in the protection of net worth from interest rate risk.

Question 47

Immunizing the net worth ratio requires that the duration of the assets be set equal to the duration of the liabilities.

Question 48

The duration of a consol bond is

A)less than its maturity.
B)infinity.
C)30 years.
D)more than its maturity.
E)given by the formula D = 1/(1-R).

Question 49

The rate of change in duration values is less than the rate of change in maturity.

Question 50

Managers can achieve the results of duration matching by using these to hedge interest rate risk.

A)Rate sensitive assets.
B)Rate sensitive liabilities.
C)Coupon bonds.
D)Consol bonds.
E)Derivatives.

Question 51

Convexity is a desirable effect to a portfolio manager because it is easy to measure and price.

Question 52

The duration of all floating rate debt instruments is

A)equal to the time to maturity.
B)less than the time to repricing of the instrument.
C)time interval between the purchase of the security and its sale.
D)equal to time to repricing of the instrument.
E)infinity.

Question 53

All fixed-income assets exhibit convexity in their price-yield relationships.

Question 54

Which of the following is indicated by high numerical value of the duration of an asset?

A)Low sensitivity of an asset price to interest rate shocks.
B)High interest inelasticity of a bond.
C)High sensitivity of an asset price to interest rate shocks.
D)Lack of sensitivity of an asset price to interest rate shocks.
E)Smaller capital loss for a given change in interest rates.

Question 55

Which of the following statements about leverage adjusted duration gap is true?

A)It is equal to the duration of the assets minus the duration of the liabilities.
B)Larger the gap in absolute terms, the more exposed the FI is to interest rate shocks.
C)It reflects the degree of maturity mismatch in an FI's balance sheet.
D)It indicates the dollar size of the potential net worth.
E)Its value is equal to duration divided by (1 + R).

Question 56

Immunizing the balance sheet to protect equity holders from the effects of interest rate risk occurs when

A)the maturity gap is zero.
B)the repricing gap is zero.
C)the duration gap is zero.
D)the effect of a change in the level of interest rates on the value of the assets of the FI is exactly offset by the effect of the same change in interest rates on the liabilities of the FI.
E)after-the-fact analysis demonstrates that immunization coincidentally occurred.

Question 57

For small change in interest rates, market prices of bonds move in an inversely proportional manner according to the size of the

A)equity.
B)asset value.
C)liability value.
D)duration value.
E)Answers A and B only.

Question 58

The greater is convexity, the more insurance a portfolio manager has against interest rate increases and the greater potential gain from rate decreases.

Question 59

As the investment horizon approaches, the duration of an unrebalanced portfolio that originally was immunized will be less than the time remaining to the investment horizon.

Question 60

Immunizing net worth from interest rate risk using duration matching requires that the duration match must be realigned periodically as the maturity horizon approaches.

Question 61

Fully amortizing loan cash flows:
$$\begin{array} { | l | l | l | l | l | } \hline \mathrm { PV } = 30,000,000 & \mathrm { FV } = 0 & \mathrm { I } = 6 & \mathrm {~N} = 2 & \mathbf { P M T } = \mathbf { 1 6 , 3 6 3 , 1 0 7 } \\\hline\end{array}$$ Macaulay's Duration $$D = \frac { \sum _ { t = 1 } ^ { N } P V _ { t } \times t } { \sum _ { t = 1 } ^ { N } P _ { t } } = \frac { \left[ \frac { 16,363,107 } { ( 1.06 ) ^ { 1 } } \times 1 \right] + \left[ \frac { 16,363,107 } { ( 1.06 ) ^ { 2 } } \times 2 \right] } { 30,000,000 } = 1.4854$$

-Calculate the modified duration of a two-year corporate loan paying 6 percent interest annually. The $40,000,000 loan is 100 percent amortizing, and the current yield is 9 percent annually.

A)2 years.
B)1.91 years.
C)1.94 years.
D)1.49 years.
E)1.36 years.

Question 62

The following information is about current spot rates for Second Duration Savings' assets (loans) and liabilities (CDs). All interest rates are fixed and paid annually $$\begin{array} { | c | c | } \hline \text { Assets } & \text { Liabilities } \\\hline \text { 1-year loan rate: } 7.50 \text { percent } & \text { 1-year CD rate: } 6.50 \text { percent } \\\hline \text { 2-year loan rate: } 8.15 \text { percent } & \text { 2-year CD rate: } 6.65 \text { percent } \\\hline\end{array}$$

-What is the duration of the two-year loan (per $100 face value) if it is selling at par?

A)2.00 years
B)1.92 years
C)1.96 years
D)1.00 year
E)0.91 years

Question 63

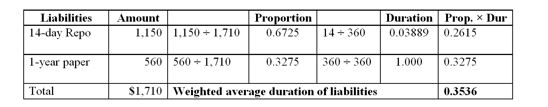
What is the leverage-adjusted duration gap?

A)0.605 years.
B)0.956 years.
C)0.360 years.
D)0.436 years.
E)0.189 years.

Question 64

Calculate the duration of a two-year corporate loan paying 6 percent interest annually, selling at par. The $30,000,000 loan is 100 percent amortizing with annual payments.

A)2 years.
B)1.89 years.
C)1.94 years.
D)1.49 years.
E)1.73 years.

Question 65

Macaulay's Duration
$$D = \frac { \sum _ { t = 1 } ^ { N } P V _ { t } \times t } { \sum _ { t = 1 } ^ { N } P _ { t } } = \frac { \left[ \frac { 100 } { ( 1.10 ) ^ { 5 } } \times 5 \right] } { \left[ \frac { 100 } { ( 1.10 ) ^ { 5 } } \right] } = \frac { 310.46 } { 62.09 } = 5.00 \text { years }$$ The duration of a zero coupon bond is equal to its maturity.

-Calculating modified duration involves

A)dividing the value of duration by the change in the market interest rate.
B)dividing the value of duration by 1 plus the interest rate.
C)dividing the value of duration by discounted change in interest rates.
D)multiplying the value of duration by discounted change in interest rates.
E)dividing the value of duration by the curvature effect.

Question 66

Modified Duration, semi-annual
$$M D = \frac { D } { \left( 1 + \frac { 1 } { 2 } R \right) } = \frac { 7.50 } { ( 1.05 ) } = 7.143$$ Dollar Duration
$$\text { Dollar Duration } = M D \times P = 7.143 \times 100,000 = 714,300$$ Change in Price
$$\Delta P = - \text { Dollar Duration } \times \Delta R = 714,300 \times 0.04 = - 28,572$$ New Price
$$100,000 - 28,572 = \$ 71,428$$

-What is the duration of an 8 percent annual payment two-year note that currently sells at par?

A)2 years.
B)1.75 years.
C)1.93 years.
D)1.5 years.
E)1.97 years.

Question 67

Duration of commercial loans $$D = \frac { \sum _ { t = 1 } ^ { N } P V _ { t } \times t } { \sum _ { t = 1 } ^ { N } P _ { t } } = \frac { \left[ \frac { 40,000,000 } { ( 1.10 ) ^ { 1 } } \times 1 \right] + \left[ \frac { 40,000,000 + 400,000,000 } { ( 1.10 ) ^ { 2 } } \times 2 \right] } { 400,000,000 } = 1.9114$$

-What is the FI's leverage-adjusted duration gap?

A)0.91 years.
B)0.83 years.
C)0.73 years.
D)0.50 years.
E)0 years.

Question 68

First Duration, a securities dealer, has a leverage-adjusted duration gap of 1.21 years, $60 million in assets, 7 percent equity to assets ratio, and market rates are 8 percent.
What is the impact on the dealer's market value of equity per $100 of assets if the change in all interest rates is an increase of 0.5 percent [i.e., ΔR = 0.5 percent]

A)+$336,111.
B)-$0.605.
C)-$336,111.
D)+$0.605.
E)-$363,000.

Question 69

When does "duration" become a less accurate predictor of expected change in security prices?

A)As interest rate shocks increase in size.
B)As interest rate shocks decrease in size.
C)When maturity distributions of an FI's assets and liabilities are considered.
D)As inflation decreases.
E)When the leverage adjustment is incorporated.

Question 70

The following information is about current spot rates for Second Duration Savings' assets (loans) and liabilities (CDs). All interest rates are fixed and paid annually $$\begin{array} { | c | c | } \hline \text { Assets } & \text { Liabilities } \\\hline \text { 1-year loan rate: } 7.50 \text { percent } & \text { 1-year CD rate: } 6.50 \text { percent } \\\hline \text { 2-year loan rate: } 8.15 \text { percent } & \text { 2-year CD rate: } 6.65 \text { percent } \\\hline\end{array}$$

-What is the interest rate risk exposure of the optimal transaction in the previous question over the next 2 years?

A)The risk that interest rates will rise since the FI must purchase a 2-year CD in one year.
B)The risk that interest rates will rise since the FI must sell a 1-year CD in one year.
C)The risk that interest rates will fall since the FI must sell a 2-year loan in one year.
D)The risk that interest rates will fall since the FI must buy a 1-year loan in one year.
E)There is no interest rate risk exposure.

Question 71

The following information is about current spot rates for Second Duration Savings' assets (loans) and liabilities (CDs). All interest rates are fixed and paid annually $$\begin{array} { | c | c | } \hline \text { Assets } & \text { Liabilities } \\\hline \text { 1-year loan rate: } 7.50 \text { percent } & \text { 1-year CD rate: } 6.50 \text { percent } \\\hline \text { 2-year loan rate: } 8.15 \text { percent } & \text { 2-year CD rate: } 6.65 \text { percent } \\\hline\end{array}$$

-If rates do not change, the balance sheet position that maximizes the FI's returns is

A)a positive spread of 15 basis points by selling 1-year CDs to finance 2-year CDs.
B)a positive spread of 100 basis points by selling 1-year CDs to finance 1-year loans.
C)a positive spread of 85 basis points by financing the purchase of a 1-year loan with a 2-year CD.
D)a positive spread of 165 basis points by selling 1-year CDs to finance 2-year loans.
E)a positive spread of 150 basis points by selling 2-year CDs to finance 2-year loans.

Question 72

First Duration Bank has the following assets and liabilities on its balance sheet $$\begin{array} { | l | l | l | l | l | l | } \hline{ \text { Assets } } & { \begin{array} { c } \text { Par } \\\text { Amount }\end{array} } & \text { Rate } & \text { Liabilities } & \begin{array} { c } \text { Par } \\\text { Amount }\end{array} & \text { Rate } \\\hline \begin{array} { l } \text { 2-year commercial } \\\text { loans, annual fixed } \\\text { rate, at par }\end{array} & \$ 400 \text { million } & 10 \% & \begin{array} { l } \text { 1-year CDs, } \\\text { annual fixed } \\\text { rate, at par }\end{array} & \$ 450 \text { million } & 7 \% \\\hline \text { 1-year Treasury bills } & \$ 100 \text { million } & & \text { Net Worth } & \$ 50 \text { million } & \\\hline\end{array}$$

-What is the duration of the commercial loans?

A)1.00 years.
B)2.00 years.
C)1.73 years.
D)1.91 years.
E)1.50 years.

Question 73

$$\left[ D _ { A } - D _ { L } k \right] = \left[ 0.955 - 0.354 \times \left( \frac { 1,710 } { 1,730 } \right) \right] = [ 0.955 - 0.350 ] = 0.605$$ Consider a five-year, 8 percent annual coupon bond selling at par of $1,000.

-What is the duration of this bond?

A)5 years.
B)4.31 years.
C)3.96 years.
D)5.07 years.
E)Not enough information to answer.

Question 74

$$\begin{array} { | l | r | l | l | l | l | l | } \hline{ \text { Assets } } & \text { Amount } & & \text { Proportion } & & \text { Duration } & \text { Prop. } \times \text { Dur } \\\hline \text { 30-day T-bills } & 300 & 300 \div 1,730 & 0.1734 & 30 + 360 & 0.083 & 0.144442 \\\hline \text { 90-day T-bills } & 550 & 550 \div 1.730 & 0.3179 & 90 \div 360 & 0.250 & 0.079475 \\\hline \text { 2-year T-notes } & 700 & 700 \div 1,730 & 0.4046 & 720 \div 360 & 2.000 & 0.8092 \\\hline \text { 180-day munis } & 180 & 180 \div 1,730 & 0.1041 & 180 \div 360 & 0.500 & 0.05205 \\\hline \text { Total } & \$ 1,730 & \text { Weighted average duration of assets } & & &&\mathbf { 0 . 9 5 5 2 } \\\hline\end{array}$$

-What is the duration of the liabilities?

A)0.708 years.
B)0.354 years.
C)0.350 years.
D)0.955 years.
E)0.519 years.

Question 75

An FI has financial assets of $800 and equity of $50. If the duration of assets is 1.21 years and the duration of all liabilities is 0.25 years, what is the leverage-adjusted duration gap?

A)0.9000 years.
B)0.9600 years.
C)0.9756 years.
D)0.8844 years.
E)Cannot be determined.

Question 76

Leverage-adjusted duration gap $$- \left[ D _ { A } - D _ { L } k \right] = - \left[ 1.21 - 0.25 \times \left( \frac { 800 - 50 } { 800 } \right) \right] = - [ 1.21 - 0.234375 ] = - 0.9756$$

-Calculate the duration of a two-year corporate bond paying 6 percent interest annually, selling at par. Principal of $20,000,000 is due at the end of two years.

A)2 years.
B)1.91 years.
C)1.94 years.
D)1.49 years.
E)1.75 years.

Question 77

Macaulay's Duration $$D = \frac { \sum _ { t = 1 } ^ { N } P V _ { t } \times t } { \sum _ { t = 1 } ^ { N } P _ { t } } = \frac { \left[ \frac { 8 } { ( 1.08 ) ^ { 1 } } \times 1 \right] + \left[ \frac { 8 + 100 } { ( 1.08 ) ^ { 2 } } \times 2 \right] } { 100 } = 1.9259 \text { years }$$

-What is the duration of a 5-year par value zero coupon bond yielding 10 percent annually?

A)0.50 years.
B)2.00 years.
C)4.40 years.
D)5.00 years.
E)4.05 years.

Question 78

Immunization of a portfolio implies that changes in _____ will not affect the value of the portfolio.

A)book value of assets
B)maturity
C)market prices
D)interest rates
E)duration

Question 79

$1,000 face value, 9% annual coupon, 5 year maturity, 10% rate $$\begin{array} { | l | l | l | l | l | } \hline \mathrm { PV } = 962.09 & \mathrm { FV } = 1,000 & \mathrm { I } = 10 & \mathrm {~N} = 5 & \text { PMT } = 90 \\\hline\end{array}$$

-What is the duration of the bond?

A)4.677 years.
B)5.000 years.
C)4.674 years.
D)4.328 years.
E)4.223 years

Question 80

$$D = \frac { \left[ \frac { 80 } { ( 1.08 ) ^ { 1 } } \times 1 \right] + \left[ \frac { 80 } { ( 1.08 ) ^ { 2 } } \times 2 \right] \left[ \frac { 80 } { ( 1.08 ) ^ { 3 } } \times 3 \right] \left[ \frac { 80 } { ( 1.08 ) ^ { 4 } } \times 4 \right] \left[ \frac { 80 + 1,000 } { ( 1.08 ) ^ { 5 } } \times 5 \right] } { 1,000 } = 4.312$$

-If interest rates increase by 20 basis points, what is the approximate change in the market price using the duration approximation?

A)-$7.985
B)-$7.941
C)-$3.990
D)+$3.990
E)+$7.949